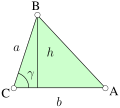
邊 (幾何)
|
Read other articles:
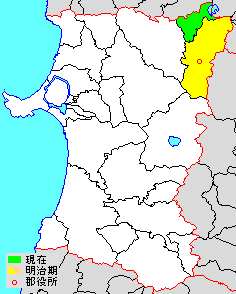
Lokasi Distrik Kazuno di Prefektur Akita. Lokasi munisipalitas yang ada di Distrik Kazuno, Prefektur Akita1. – Kosakawarna hijau - cakupan wilayah distrik saat iniwarna kuning - bekas wilayah distrik pada awal zaman Meiji Distrik Kazuno (鹿角郡code: ja is deprecated , Kazuno-gun) adalah sebuah distrik yang terletak di Prefektur Akita, Jepang. Per 1 Oktober 2020, distrik ini memiliki estimasi jumlah penduduk sebesar 4.780 jiwa dan kepadatan penduduk sebesar 23,70 orang per km². Distrik i...

Jinshi Hanzi tradisional: 進士 Hanzi sederhana: 进士 Alih aksara Mandarin - Hanyu Pinyin: jìnshì - Wade-Giles: chin⁴-shih⁴ Jinshi (Hanzi: 進士; Pinyin: jìnshì) adalah tingkat tertinggi dan terakhir dalam ujian kenegaraan pada masa Kekaisaran Tiongkok.[1][2] Ujian ini biasanya diadakan di ibu kota, di dalam istana, sehinga disebut juga Ujian Ibu kota. Mereka yang berhasil lulus ujian tingkat akhir ini dinamakan jinshi, terkadang diterjemahkan menjadi Sarj...

Public university in Charlottesville, Virginia, US UVa redirects here. For other uses, see Uva. University of VirginiaTypePublic research universityEstablishedJanuary 25, 1819; 205 years ago (January 25, 1819)[1]FounderThomas JeffersonAccreditationSACSAcademic affiliationsAAUORAUSCHEVURASea-grantSpace-grantEndowment$13.6 billion (2022)[2]Budget$1.91 billion (2020)[3][a]PresidentJames E. RyanProvostIan BaucomAcademic staff3,265 (Fall 2019)[...

This article does not cite any sources. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Eska TV – news · newspapers · books · scholar · JSTOR (February 2024) (Learn how and when to remove this template message) Television channel Eska TVCountryPolandOwnershipOwnerZPR Media Group (formerly), Telewizja Polsat (Present)Sister channelsEska TV ExtraEska Rock TVHistoryLa...

Commune in Hauts-de-France, FranceSeclinCommuneSeclin Town Hall Coat of armsLocation of Seclin SeclinShow map of FranceSeclinShow map of Hauts-de-FranceCoordinates: 50°32′56″N 3°01′49″E / 50.5489°N 3.0303°E / 50.5489; 3.0303CountryFranceRegionHauts-de-FranceDepartmentNordArrondissementLilleCantonFaches-ThumesnilIntercommunalityMétropole Européenne de LilleGovernment • Mayor (2020–2026) François-Xavier Cadart[1]Area117.42 k...

This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Inter Nashville FC – news · newspapers · books · scholar · JSTOR (April 2018) (Learn how and when to remove this message) This article needs to be updated. Please help update this article to reflect recent events or newly available information. (May 2022) Socce...

Shoe with a tall, thin heel For other uses, see Stiletto (disambiguation). This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Stiletto heel – news · newspapers · books · scholar · JSTOR (April 2019) (Learn how and when to remove this message) Stiletto heels can be found on almost every type of shoes, such as th...

Disambiguazione – Indiana Jones and the Temple of Doom rimanda qui. Se stai cercando il videogioco tratto dal film, vedi Indiana Jones and the Temple of Doom (videogioco). Indiana Jones e il tempio maledettoShorty (Ke Huy Quan), Willie (Kate Capshaw) e Indiana Jones (Harrison Ford) in una scena del filmTitolo originaleIndiana Jones and the Temple of Doom Lingua originaleinglese Paese di produzioneStati Uniti d'America Anno1984 Durata119 min Rapporto2,35:1 Genereavventura, azion...

ХристианствоБиблия Ветхий Завет Новый Завет Евангелие Десять заповедей Нагорная проповедь Апокрифы Бог, Троица Бог Отец Иисус Христос Святой Дух История христианства Апостолы Хронология христианства Раннее христианство Гностическое христианство Вселенские соборы Н...

Stasiun Abeyamakōen安部山公園駅Stasiun Abeyamakōen pada Agustus 2007Lokasi4 Yugawa-shinmachi, Kokuraminami, Kitakyushu, Fukuoka(北九州市小倉南区湯川新町4丁目)JepangKoordinat33°50′39″N 130°54′15″E / 33.84417°N 130.90417°E / 33.84417; 130.90417Koordinat: 33°50′39″N 130°54′15″E / 33.84417°N 130.90417°E / 33.84417; 130.90417Operator JR KyushuJalur■ Jalur Utama NippōSejarahDibuka1987PenumpangFY20...

Scottish novelist, surgeon, critic and playwright, 1721–1771 This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Tobias Smollett – news · newspapers · books · scholar · JSTOR (October 2017) (Learn how and when to remove this message) Tobias SmollettBornTobias George Smollett(1721-03-19)19 March 1721Dalquhurn (...

United States historic placeCrow Creek SiteU.S. National Register of Historic PlacesU.S. National Historic Landmark Aerial view of the siteShow map of South DakotaShow map of the United StatesNearest cityChamberlain, South DakotaCoordinates43°58′48″N 99°19′54″W / 43.98000°N 99.33167°W / 43.98000; -99.33167Builtcirca 1100 ADNRHP reference No.66000710Significant datesAdded to NRHPOctober 15, 1966[1]Designated NHLJuly 19, 1964[2]...

Baseball player Zack CoxCox with the Arkansas RazorbacksThird basemanBorn: (1989-05-09) May 9, 1989 (age 34)Campbellsville, KentuckyBats: LeftThrows: Right Zackary Kendrick Cox (born May 9, 1989) is an American retired professional baseball third baseman. Amateur career Cox was born in Campbellsville, Kentucky. He attended Pleasure Ridge Park High School in the Pleasure Ridge Park neighborhood of Louisville, and played for the school's baseball team. Cox was selected in the 20th round o...

World Weightlifting Championship Main article: 2021 World Weightlifting Championships 2021 World Weightlifting ChampionshipsMenWomen55 kg45 kg61 kg49 kg67 kg55 kg73 kg59 kg81 kg64 kg89 kg71 kg96 kg76 kg102 kg81 kg109 kg87 kg+109 kg+87 kgvte The men's 96 kilograms competition at the 2021 World Weightlifting Championships was held on 13 and 14 December 2021.[1] Schedule Date Time Event 13 December 2021 21:30 Group D 14 December 2021 08:30 Group C 13:00 Group B 16:00 Group A Medalists Ev...

This article needs to be updated. Please help update this article to reflect recent events or newly available information. (April 2019) The Ecuadorian Constitution requires that all children attend school until they achieve a “basic level of education,” which is estimated at nine school years. The Human Rights Measurement Initiative (HRMI)[1] finds that Ecuador is fulfilling only 83.4% of what it should be fulfilling for the right to education based on the country's level of inco...

Angelique KerberAngelique Kerber nel 2021Nazionalità Germania Polonia Altezza173 cm Peso68 kg Tennis Carriera Singolare1 Vittorie/sconfitte 673 – 363 (64.96%) Titoli vinti 14 Miglior ranking 1ª (12 settembre 2016) Ranking attuale ranking Risultati nei tornei del Grande Slam Australian Open V (2016) Roland Garros QF (2012, 2018) Wimbledon V (2018) US Open V (2016) Altri tornei Tour Finals F (2016) Giochi olimpici (2016) Doppio1 Vittorie/sconfit...

Metro station in Chongqing, China Cuiyun翠云General informationLocationYubei District, ChongqingChinaOperated byChongqing Rail Transit Corp., LtdLine(s) Line 3Platforms2 side platformsConstructionStructure typeElevatedOther informationStation code3/34HistoryOpened8 October 2011 (2011-10-08)Services Preceding station Chongqing Rail Transit Following station The EXPO Gardentowards Yudong Line 3 Changfulutowards Terminal 2 of Jiangbei Airport Cuiyun ...

Study of the timing of biological events Not to be confused with Phrenology, Phonology, or Phenomenology. Phenology is the study of periodic events in biological life cycles and how these are influenced by seasonal and interannual variations in climate, as well as habitat factors (such as elevation).[1] Examples include the date of emergence of leaves and flowers, the first flight of butterflies, the first appearance of migratory birds, the date of leaf colouring and fall in deciduous...

Orang Han (漢族 atau 汉族)Zhou GongGong FuziLaoziQu YuanZhuge LiangWang XizhiTang TaizongLi BaiQin ShihuangHan WudiWu ZetianYue FeiWen TianxiangQin LiangyuKoxingaSun Yat-senJumlah populasi1.310.000.000 (satu miliar tiga ratus sepuluh juta)19,73% populasi dunia(estimasi)Daerah dengan populasi signifikan Tiongkok1.317.541.842[1] Taiwan22.575.365[2] Hong Kong7.393.410[3] Singapura2.984.936[4] Makau433.641[5] Thailand9.053.240[6] Malaysia7.590.500[7] Am...

Lok Sabha constituency in West Bengal Purulia WB-35Lok Sabha constituencyInteractive Map Outlining Purulia Lok Sabha ConstituencyConstituency detailsCountryIndiaRegionEast IndiaStateWest BengalAssembly constituenciesBaghmundiManbazarBalarampurKashipurParaJoypurPuruliaEstablished1957Total electors18,23,120 (2024)[1]ReservationNoneMember of Parliament18th Lok SabhaIncumbent Jyotirmoy Singh Mahato Party Bharatiya Janata PartyElected year2024 Purulia Lok Sabha constituency is one of the p...