三角学
|
Read other articles:

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Januari 2023. Hutan Rekreasi Soga PerdanaMelayu: Hutan Lipur Soga Perdanacode: ms is deprecated GeografiLokasiBatu Pahat, Johor, MalaysiaKoordinat1°50′53.6″N 102°57′37.8″E / 1.848222°N 102.960500°E / 1.848222; 102.960500Koordi...

Dharmavaram RamakrishnamacharyuluLahir1853Dharmavaram, distrik AnantapurMeninggal1912PekerjaanPengacaraKebangsaanIndiaKewarganegaraanIndiaPendidikanMatriculation, F.A.GenrePenulis drama, penyair, pemeranKarya terkenalChitranaliyamPenghargaanAndhra Nataka PithamahaKerabatBellary Raghava, Dharmavaram Gopalacharyulu Dharmavaram Ramakrishnamacharyulu (1853 – 30 November 1912)[1] adalah seorang dramawan dan penulis drama Telugu dari Bellary. Ia dikenal sebagai Andhra Nataka Pithamah...

Artikel ini sudah memiliki daftar referensi, bacaan terkait, atau pranala luar, tetapi sumbernya belum jelas karena belum menyertakan kutipan pada kalimat. Mohon tingkatkan kualitas artikel ini dengan memasukkan rujukan yang lebih mendetail bila perlu. (Pelajari cara dan kapan saatnya untuk menghapus pesan templat ini) Fabulous BoysDari kiri ke kanan. Hwang In Deok, Lyan Cheng, Jiro Wang dan Evan YoGenreRomansa KomediPemeranLyan ChengJiro WangHwang In DeokEvan YoLagu pembukaPromise (約定) o...

Warwickshire RingTwo boats entering lock 44 of the Hatton flight, the third from the topSpecificationsLength116 miles (187 km)Locks105StatusCanal ringNavigation authorityCanal & River Trust vteWarwickshire Ring Legend Fazeley Junction Coventry Canal Drayton Manor Theme Park Curdworth Locks (11) Tamworth Locks (2) M6 Toll motorway 57 yd Curdworth Tunnel Tamworth M42 motorway Minworth Locks (3) Birmingham and Fazeley Canal Salford JunctionNechells/Erdington Garrison Locks (5...

North-south avenue in Manhattan, New York Template:Attached KML/Seventh Avenue (Manhattan)KML is from Wikidata Seventh AvenueSeventh Avenue South (south of 11th St)Fashion Avenue (26th–42nd Sts)Adam Clayton Powell Jr. Boulevard (north of 110th St)Seventh Avenue heading north to Greenwich Village and Central ParkNamesakeGarment District and Adam Clayton Powell Jr.OwnerCity of New YorkMaintained byNYCDOTLength5.3 mi (8.5 km)[1][2]LocationManhattan, New York CitySouth...

Study of the microorganisms that inhibit, create, or contaminate food Food safety Terms Foodborne illness Good manufacturing practice (GMP) Hazard analysis and critical control points (HACCP) Hazard analysis and risk-based preventive controls (HARPC) Critical control point Critical factors FAT TOM pH Water activity (aw) Bacterial pathogens Clostridium botulinum Escherichia coli Listeria Salmonella Vibrio cholerae Cronobacter spp Viral pathogens Enterovirus Hepatitis A Norovirus Rotavirus Para...

この記事は検証可能な参考文献や出典が全く示されていないか、不十分です。出典を追加して記事の信頼性向上にご協力ください。(このテンプレートの使い方)出典検索?: コルク – ニュース · 書籍 · スカラー · CiNii · J-STAGE · NDL · dlib.jp · ジャパンサーチ · TWL(2017年4月) コルクを打ち抜いて作った瓶の栓 コルク(木栓、�...

Village in Telangana, IndiaAdibatlaVillageAdibatlaLocation in Telangana, IndiaShow map of TelanganaAdibatlaAdibatla (India)Show map of IndiaCoordinates: 17°14′N 78°33′E / 17.233°N 78.550°E / 17.233; 78.550Country IndiaStateTelanganaPopulation (2017) • Total3,000Languages • OfficialTeluguTime zoneUTC+5:30 (IST)Telephone code040Vehicle registrationTS 07Sex ratio1:1(approx) ♂/♀ Adibatla is a village in Ranga Reddy district ne...

Opera by Richard Strauss Richard Strauss Guntram (Op. 25) is an opera in three acts by Richard Strauss with a German libretto written by the composer. The second act of the opera was composed in Ramacca, Sicily.[1] It was Strauss' first opera and shows a strong Wagnerian influence. The music of Guntram is quoted in Strauss's tone poem Ein Heldenleben. The composer revised the score in 1940. Performance history The opera was not very successful, and was only staged a few times during S...

Toyota FX-1Toyota FX-1 all'AutoRAI 1985Descrizione generaleCostruttore Toyota Tipo principalecoupé 2+2 Produzionenel 1983 Esemplari prodotti1 La Toyota FX-1 è una concept car realizzata dalla Toyota nel 1983. Il contesto Presentata presso il salone dell'automobile di Tokyo del 1983, la FX-1 si poneva come la possibile erede della Toyota 2000GT. La carrozzeria venne progettata per offrire solo 0,25 CX di coefficiente di resistenza aerodinamica, mentre la configurazione della vettura era...

Fairchild AviationJenisAircraft manufacturerDidirikan1925PendiriSherman FairchildDitutup2003KantorpusatSan Antonio, Texas, USAAnakusahaFairchild Aircraft Ltd. Ranger Aircraft Engine Division/Fairchild Engine DivisionSitus webhttp://www.m7aerospace.com Fairchild adalah nama sebuah perusahaan manufaktur pesawat dan kedirgantaraan yang berbasis di Farmingdale, New York, Hagerstown, Maryland, dan San Antonio, Texas. The Fairchild plant at Jamaica, NY, in 1941. Fairchild Argus III, 1944 Fairchild ...

Частина серії проФілософіяLeft to right: Plato, Kant, Nietzsche, Buddha, Confucius, AverroesПлатонКантНіцшеБуддаКонфуційАверроес Філософи Епістемологи Естетики Етики Логіки Метафізики Соціально-політичні філософи Традиції Аналітична Арістотелівська Африканська Близькосхідна іранська Буддій�...

This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Pusser's – news · newspapers · books · scholar · JSTOR (January 2009) (Learn how and when to remove this message) A derelict sign promoting Pusser's Rum Pusser's Rum is a brand name of rum produced by Pusser's Rum Ltd., based in the British Virgin Islands. Nine...
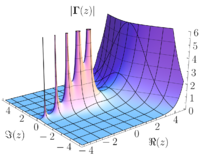
Complex-differentiable (mathematical) function For Zariski's theory of holomorphic functions on an algebraic variety, see formal holomorphic function. Holomorphism redirects here. Not to be confused with Homomorphism. A rectangular grid (top) and its image under a conformal map f (bottom). Mathematical analysis → Complex analysisComplex analysis Complex numbers Real number Imaginary number Complex plane Complex conjugate Unit complex number Complex functions Complex-valued function Analyti...

Federal governmental agency within the United States Administrator of the Environmental Protection AgencySeal of the Environmental Protection AgencyIncumbentMichael S. Regansince March 11, 2021Member ofCabinetInaugural holderWilliam D. RuckelshausFormation1970Websitewww.epa.gov The administrator of the Environmental Protection Agency is the head of the United States Environmental Protection Agency (EPA), and is thus responsible for enforcing the nation's Clean Air and Clean Water Acts, a...

Egyptian activist Asmaa Mahfouzأسماء محفوظMahfouz in 2011Born (1985-02-01) 1 February 1985 (age 39)Cairo, EgyptNationalityEgyptianAlma materCairo UniversityKnown for2011 Egyptian revolution Asmaa Mahfouz (Egyptian Arabic: أسماء محفوظ, pronounced [ʔæsˈmæːʔ mɑħˈfuːz, ˈʔæsmæ-], born 1 February 1985) is an Egyptian activist and one of the founders of the April 6 Youth Movement.[1] She has been credited by journalist Mona Eltahawy an...

New Zealand professional men's basketball team New Zealand Breakers 2023–24 New Zealand Breakers seasonLeagueNBLFounded2003; 21 years ago (2003)HistoryNew Zealand Breakers2003–presentArenaSpark ArenaCapacity9,740LocationAuckland, New ZealandTeam coloursPink, blue, white, black Main sponsorBank of New ZealandCEOLisa EdserGeneral managerSimon EdwardsHead coachVacantTeam captainThomas AbercrombieOwnershipBreakers Basketball LtdChampionships4 (2011,...

US Open 2015Singolare femminileSport Tennis Vincitrice Flavia Pennetta Finalista Roberta Vinci Punteggio7–6(4), 6-2 Tornei Singolare uomini (q) donne (q) ragazzi ragazze Doppio uomini donne misto ragazzi ragazze Singolare carrozzina uomini donne quad 2014 2016 Voce principale: US Open 2015. Serena Williams era la detentrice del titolo, ma è stata eliminata in semifinale da Roberta Vinci. Flavia Pennetta si è aggiudicata il torneo sconfiggendo in finale Roberta Vinci per 7–6(4), 6...

議会調査局ロゴ 議会調査局(ぎかいちょうさきょく、英:Congressional Research Service)は1970年からアメリカ議会図書館に置かれる立法補佐機関[1]。前身の立法レファレンス局(英語 Legislative Reference Service)は1914年に設置された[1][2]。略称CRS[1]。議員や議会委員会などの立法活動を助ける情報提供や調査依頼への回答などを行う[1]。 年間予算配...

Resistance to the Russian invasion of Ukraine in Belarus and Russia It has been suggested that content related to Russia be split out and merged into the article titled Attacks in Russia during the Russian invasion of Ukraine, which already exists. (Discuss) (March 2024) It has been suggested that content related to Belarus be split out and merged into the article titled Belarusian partisan movement (2020–present), which already exists. (Discuss) (January 2024) Belarusian and Russia...






































![{\displaystyle {\frac {a-b}{a+b}}={\frac {\tan \left[{\tfrac {1}{2}}(A-B)\right]}{\tan \left[{\tfrac {1}{2}}(A+B)\right]}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a1da4e06eb6f25cd7f7fc1a7784a11a82ae53f9f)





















