Stochastic matrix
|
Read other articles:

Gereja Santo Matthew, Maubisse, Timor Leste Mayoritas penduduk Timor Leste beragama Katolik, dan Gereja Katolik adalah institusi keagamaan yang dominan.[1] Ada juga sebagian kecil komunitas Protestan dan Muslim.[1] Sejarah Agama di Timor Leste (sensus 2015) Kristen Katolik (97.57%) Kristen Protestan (1.96%) Islam (0.24%) Agama Tradisional (0.08%) Agama Buddha (0.05%) Agama Hindu (0.02%) Lainnya (0.08...

Lazare Lévy Lazare Lévy, juga disebut sebagai Lazare-Lévy,[1] (18 Januari 1882 – 20 September 1964) adalah seorang pianis, organis, komponis dan pedagog Prancis berpengaruh. Sebagai pianis virtuoso, ia berkeliling ke seluruh penjuru Eropa, Afrika Utara, Israel, Uni Soviet dan Jepang. Ia mengajar selama beberapa tahun di Paris Conservatoire. Sejumlah pianis telah menjadi muridnya, antara lain Agnelle Bundervoët,[2] Jean Langlais,[3] Clara Haskil,[...

Cet article est une ébauche concernant le chemin de fer. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. L'agglomération nantaise (Nantes Métropole) compte 16 gares ouvertes au trafic voyageurs[1], dont treize sont en dehors de la ville de Nantes (voir l'article Liste des gares à Nantes) mais les territoires des communes faisant partie de la métropole. Elles sont toutes desservies par les TER Pays de la Loi...

Indian revolutionary (1890–1942) Sachindra Nath SanyalSachindra Nath SanyalBorn(1890-04-03)3 April 1890Benares, Benares State, British IndiaDied7 February 1942(1942-02-07) (aged 51)Gorakhpur, United Provinces, British IndiaOccupationRevolutionaryOrganization(s)Anushilan Samiti, Ghadar Party, Hindustan Socialist Republican AssociationNotable workA Life of Captivity (Bandi Jeevan)MovementIndian revolutionary movementCriminal penaltyCapital punishmentCriminal statusJailedRelativesSanjeev ...

Namakura Gatanaなまくら刀 Film animeDurasi2 menit Portal anime dan manga Namakura Gatana (なまくら刀code: ja is deprecated ) atau Hanawa Hekonai meitō no maki (塙凹内名刀之巻code: ja is deprecated ) adalah sebuah anime Jepang pendek yang dibuat oleh Jun'ichi Kouchi pada 1917. Film tersebut ditemukan di sebuah toko barang antik di Osaka di tengah Jepang pada Maret 2008.[1] Referensi ^ Two Nine-Decade-Old Anime Films Discovered (Updated). Anime News Network.&...

Table tennis at the 2006 Asian GamesVenueAl-Arabi Indoor HallDates29 November – 7 December 2006Competitors144 from 22 nations← 20022010 → Table Tennis was contested by men and women at the 2006 Asian Games in Doha, Qatar from November 29 to December 7. It was one of six sports to begin prior to the Opening Ceremonies on December 1. Singles, Doubles, and Team events were held with all competition taking place at the Al-Arabi Indoor Hall. Schedule P ...

Dra.Lucy Kurniasari Anggota Dewan Perwakilan RakyatRepublik IndonesiaPetahanaMulai menjabat 18 Mei 2018Pengganti Antar Waktu hingga 30 September 2019Perolehan suara28.378 (2019)PendahuluFandi UtomoPenggantiPetahanaDaerah pemilihanJawa Timur IMasa jabatan1 Oktober 2009 – 30 September 2014Perolehan suara40.555 (2009)Daerah pemilihanJawa Timur I Informasi pribadiLahir4 Februari 1968 (umur 56)Surabaya, IndonesiaKebangsaanIndonesiaPartai politikPartai DemokratAnakLaras Ayu Kart...
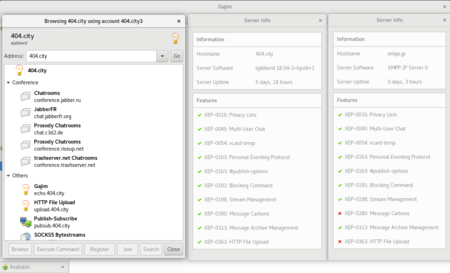
Free instant messaging client for the XMPP protocol Not to be confused with Gaim. GajimGajim main window (version 1.8.2)Developer(s)Gajim DevelopersInitial releaseMay 21, 2004[1]Stable release1.8.4[2] / 26 November 2023 Repositorydev.gajim.org/gajim/gajimWritten inPythonOperating systemBSD, Linux, macOS, Microsoft WindowsStandard(s)XMPPAvailable inMulti language[3]TypeInstant messaging clientLicenseGPL-3.0-onlyWebsitegajim.org Gajim /ɡɛˈʒiːm/[4] is a...

German academic publisher LIT VerlagFounded1980Country of originGermanyHeadquarters locationMünsterPublication typesBooksOfficial websitewww.lit-verlag.de LIT Verlag is a German academic[1] publisher founded in 1980.[2] Its managing director is Wilhelm Hopf.[2] Its principal place of publication is Münster; further publishing offices are located in Berlin, Vienna, Hamburg, London, Zurich, and New York City. It publishes approximately 800 books per year. It generally ...

English language daily newspaper Typical New Times front page.TypeDaily newspaperFoundedSeptember 1995Websitewww.newtimes.co.rw The New Times is a national English language newspaper in Rwanda. It was established in 1995 shortly after the Rwandan genocide. They also used to have a Kinyarwanda-language weekly called Izuba Rirashe.[1] The New Times is published in Kigali from Monday to Saturday, with its sister paper the Sunday Times, appearing on Sundays. The New Times Online was launc...

Эту статью предлагается удалить.Пояснение причин и соответствующее обсуждение вы можете найти на странице Википедия:К удалению/13 января 2021.Пока процесс обсуждения не завершён, статью можно попытаться улучшить, однако следует воздерживаться от переименований или немот...

Peta Rupt-sur-Moselle. Rupt-sur-Moselle merupakan sebuah komune di departemen Vosges yang terletak pada sebelah timur laut Prancis. Lihat pula Komune di departemen Vosges Referensi INSEE lbsKomune di departemen Vosges Les Ableuvenettes Ahéville Aingeville Ainvelle Allarmont Ambacourt Ameuvelle Anglemont Anould Aouze Arches Archettes Aroffe Arrentès-de-Corcieux Attignéville Attigny Aulnois Aumontzey Autigny-la-Tour Autreville Autrey Auzainvilliers Avillers Avrainville Avranville Aydoilles B...

「俄亥俄」重定向至此。关于其他用法,请见「俄亥俄 (消歧义)」。 俄亥俄州 美國联邦州State of Ohio 州旗州徽綽號:七葉果之州地图中高亮部分为俄亥俄州坐标:38°27'N-41°58'N, 80°32'W-84°49'W国家 美國加入聯邦1803年3月1日,在1953年8月7日追溯頒定(第17个加入联邦)首府哥倫布(及最大城市)政府 • 州长(英语:List of Governors of {{{Name}}}]]) •&...

此條目介紹的是来自威斯康星州的美国参议员(1947–57)。关于其他叫麦卡锡的人,请见「麦卡锡」。 本條目存在以下問題,請協助改善本條目或在討論頁針對議題發表看法。 此條目需要补充更多来源。 (2018年11月7日)请协助補充多方面可靠来源以改善这篇条目,无法查证的内容可能會因為异议提出而被移除。致使用者:请搜索一下条目的标题(来源搜索:约瑟夫·雷�...
Men's 10 metre platformat the Games of the XXII OlympiadMedalists Falk Hoffmann East Germany Vladimir Aleynik Soviet Union David Ambartsumyan Soviet Union← 19761984 → Diving at the1980 Summer Olympics3 m springboardmenwomen10 m platformmenwomenvte The men's 10 metre platform, also reported as platform diving, was one of four diving events on the Diving at the 1980 Summer Olympics programme.[1] The competition was split into two phases: Prelimin...

De Rietvink, NijetrijneDe Rietvink under repair, May 2009.OriginMill nameDe RietvinkMill locationVeendijk 6, 8481 JC NijetrijneCoordinates52°51′16″N 5°54′44″E / 52.85444°N 5.91222°E / 52.85444; 5.91222Operator(s)PrivateYear built1855InformationPurposeDrainage millTypeSmock millStoreysTwo storey smockBase storeysSingle storey baseSmock sidesEight sidesNo. of sailsFour sailsType of sailsCommon sailsWindshaftCast ironWindingTailpole and winchType of pumpArchim...

Brazilian judge You can help expand this article with text translated from the corresponding article in Portuguese. (February 2015) Click [show] for important translation instructions. View a machine-translated version of the Portuguese article. Machine translation, like DeepL or Google Translate, is a useful starting point for translations, but translators must revise errors as necessary and confirm that the translation is accurate, rather than simply copy-pasting machine-translated tex...

Gustave F. PernaGeneral Gustave F. Perna pada 2016Lahir1960 (umur 63–64)PengabdianAmerika SerikatDinas/cabangAngkatan Darat Amerika SerikatLama dinas1981–kiniPangkatJenderalKomandanUnited States Army Materiel CommandJoint Munitions CommandDefense Supply Center Philadelphia4th Sustainment Brigade64th Forward Support BattalionPerang/pertempuranPerang IrakPenghargaanArmy Distinguished Service Medal (3)Defense Superior Service Medal (2)Legion of MeritBronze Star Medal (2) Gustav...

Pour les articles homonymes, voir Route 699. Cet article est une ébauche concernant la route. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Route nationale 699 L'ancienne borne départementale entre Haute-Vienne et Dordogne. Historique Déclassement D 699 Caractéristiques Longueur 153 km Direction est / ouest Extrémité est N 21 à Séreilhac (chez Quinque) Intersections D 901D 675 �...

A tool used to install electrical wires in tubes This article relies largely or entirely on a single source. Relevant discussion may be found on the talk page. Please help improve this article by introducing citations to additional sources.Find sources: Fish tape – news · newspapers · books · scholar · JSTOR (June 2018) A 75-foot (23 m) steel fish tapeA comparison of nylon (top) and steel (bottom) fish tapes A fish tape (also called a draw wire, d...


![{\displaystyle P=\left[{\begin{matrix}P_{1,1}&P_{1,2}&\dots &P_{1,j}&\dots &P_{1,\alpha }\\P_{2,1}&P_{2,2}&\dots &P_{2,j}&\dots &P_{2,\alpha }\\\vdots &\vdots &\ddots &\vdots &\ddots &\vdots \\P_{i,1}&P_{i,2}&\dots &P_{i,j}&\dots &P_{i,\alpha }\\\vdots &\vdots &\ddots &\vdots &\ddots &\vdots \\P_{\alpha ,1}&P_{\alpha ,2}&\dots &P_{\alpha ,j}&\dots &P_{\alpha ,\alpha }\\\end{matrix}}\right].}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c6200446103959c8994075edaddeddf91abb8166)

















![{\displaystyle [0,1,0,0,0]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a7e509a68d531c11b6675c081f7266e5f5225503)
![{\displaystyle {\boldsymbol {\tau }}=[0,1,0,0],\qquad T={\begin{bmatrix}0&0&{\frac {1}{2}}&0\\0&0&1&0\\{\frac {1}{4}}&{\frac {1}{4}}&0&{\frac {1}{4}}\\0&0&{\frac {1}{2}}&0\end{bmatrix}},}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e4a73af368f0021cc7df30b0adad0e2ccefba6b9)



![{\displaystyle E[K]={\boldsymbol {\tau }}\left(I+T+T^{2}+\cdots \right){\boldsymbol {1}}={\boldsymbol {\tau }}(I-T)^{-1}{\boldsymbol {1}}=4.5.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/379877a01b5d3a2bdb6a93a911e89c1ca693e33c)
![{\displaystyle E[K(K-1)\dots (K-n+1)]=n!{\boldsymbol {\tau }}(I-{T})^{-n}{T}^{n-1}\mathbf {1} \,.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9916c8baf401c9d1a9ad2ed475ab33e36ac92c2f)