Subset
|
Read other articles:

Donauinsel yang memisahkan Donau Baru (kiri) dengan Sungai Donau (kanan). Pemandangan dari Kahlenberg. Donauinsel (Pulau Donau) adalah sebuah pulau yang panjang yang terletak di kota Wina, Austria. Pulau ini terletak di antara Sungai Donau dengan kanal Neue Donau (Donau Baru). Panjang pulau ini mencapai 21,1 km, tetapi lebarnya hanya 70–210 m.[1] Pulau ini adalah pulau buatan yang terbentuk dari proyek penggalian kanal Donau Baru yang berlangsung dari tahun 1972 hingga 1988. Tu...

LukunDesaNegara IndonesiaProvinsiRiauKabupatenKepulauan MerantiKecamatanTebing Tinggi TimurKode pos28753Kode Kemendagri14.10.07.2001 Luas... km²Jumlah penduduk... jiwaKepadatan... jiwa/km² Lukun merupakan salah satu desa yang ada di kecamatan Tebing Tinggi Timur, Kabupaten Kepulauan Meranti, provinsi Riau, Indonesia. Perekonomian penduduk Desa Lukun terdapat pada sektor Perkebunan dan perikanan. Pada perkebunan meliputi menyadap karet, mengolah hasil tanaman sagu dan tanaman kelapa. Se...

الدوري المنغولي لكرة القدم 1998 تفاصيل الموسم الدوري المنغولي لكرة القدم البلد منغوليا البطل نادي إركيم الدوري المنغولي لكرة القدم 1997 الدوري المنغولي لكرة القدم 1999 تعديل مصدري - تعديل الدوري المنغولي لكرة القدم 1998 هو موسم من الدوري المنغولي لكرة القدم. �...

Town in Saxony-Anhalt, Germany Town in Saxony-Anhalt, GermanyWernigerode TownView over Wernigerode with its castle Coat of armsLocation of Wernigerode within Harz district Wernigerode Show map of GermanyWernigerode Show map of Saxony-AnhaltCoordinates: 51°50′6″N 10°47′7″E / 51.83500°N 10.78528°E / 51.83500; 10.78528CountryGermanyStateSaxony-AnhaltDistrictHarz Government • Mayor (2022–29) Tobias Kascha[1] (SPD)Area • Tot...

Jupiter trojan asteroid 617 PatroclusHubble Space Telescope image composite of Patroclus and its companion Menoetius, taken in 2018Discovery [1]Discovered byA. KopffDiscovery siteHeidelberg Obs.Discovery date17 October 1906DesignationsMPC designation(617) PatroclusPronunciation/pəˈtroʊkləs/[2]Named afterΠάτροκλος Patroklos(Greek mythology)[3]Alternative designations1906 VY · 1941 XC1962 NBMinor planet categoryJu...

Tencent QQ Tangkapan layar Tampilan utama QQ2008TipeManajemen hubungan pelanggan, layanan jejaring sosial, situs web, aplikasi seluler dan komunitas daring Versi pertama11 Februari 1999 Versi stabilDaftarmacOS: 6.9.33 (15 April 2024)Linux: 3.2.7 (15 April 2024)iOS, iPadOS, WatchOS: 9.0.35 (15 April 2024)Android: 9.0.35 (16 April 2024)Microsoft Windows: 9.9.9 (15 April 2024) GenrePengirim pesan instanModel bisnisfreemium BahasaTionghoa Daftar bahasa Tionghoa, Inggris Klasifikasi Alexa6 EponimI...
Video camera connected to a computer or network A Logitech-branded webcam attached to a laptop. A webcam is a video camera which is designed to record or stream to a computer or computer network. They are primarily used in video telephony, live streaming and social media, and security. Webcams can be built-in computer hardware or peripheral devices, and are commonly connected to a device using USB or wireless protocols. Webcams have been used on the Internet as early as 1993, and the first wi...

Questa voce o sezione sull'argomento Brani musicali non cita le fonti necessarie o quelle presenti sono insufficienti. Puoi migliorare questa voce aggiungendo citazioni da fonti attendibili secondo le linee guida sull'uso delle fonti. Segui i suggerimenti del progetto di riferimento. Il silenzioScreenshot tratto dal videoclip del branoArtistaNini Rosso Autore/iTrascriz.: N. Rosso - W. Brezza FeaturingWilly Brezza, la sua Orch. e Coro GenerePop-jazzMusica leggeraMusica strumentale[1&...

Russian-French microbiologist (1860–1930) Waldemar Mordechai HaffkineBorn15 March 1860 (1860-03-15)Odessa, Kherson Governorate, Russian Empire (now Ukraine)Died26 October 1930 (1930-10-27) (aged 70)Lausanne, SwitzerlandCitizenshipRussian EmpireFrance (later) BritishAlma materOdessa UniversityKnown forVaccines against cholera and bubonic plagueAwardsCameron Prize for Therapeutics of the University of Edinburgh (1900)Scientific careerFieldsBacteriology, protozoologyIn...

Anandha ThandavamSutradaraA.R.Gandhi KrishnaProduserViswanathan RavichandranDitulis olehA.R.Gandhi Krishna Sujatha RangarajanPemeranTamannaahSiddharth VenugopalRukmini VijayakumarRishiPenata musikG. V. Prakash KumarSinematograferJeeva ShankarPenyuntingV. T. VijayanDistributorAascar Film Pvt. LtdTanggal rilis 10 April 2009 (2009-04-10) NegaraIndiaBahasaTamil Ananda Thandavam adalah sebuah adaptasi film berbahasa Tamil tahun 2009 dari novel terserialisasi Sujatha Rangarajan Pirivom S...

German association football club Football club1. FC Schweinfurt 05Full name1. Fussball-Club Schweinfurt 1905, Verein für Leibesübungen e.V.Nickname(s)Die Schnüdel Die Grün-WeißenFounded5 May 1905; 119 years ago (1905-05-05)GroundSachs-StadionCapacity15,060[1] (860 seated)ChairmanMarkus WolfManagerMarc ReitmaierLeagueRegionalliga Bayern (IV)2022–236thWebsiteClub website Home colours Away colours Third colours 1. Fussball-Club Schweinfurt 1905, Verein für Leibe...

Radio station in BristolHeart West CountryBristolBroadcast areaBristol, Somerset, Bath, West Dorset and WiltshireFrequencyFM: Bristol North Somerset and South Gloucestershire: 96.3 (Bristol, Yate, Nailsea, Keynsham, Portishead and Thornbury), Somerset 96.5 (Taunton) 97.1 (Crewkerne, Chard & Beaminster) 102.6 (Somerset, Wells Glastonbury Bridgwater Shepton Mallet & Burnham-on-Sea) 103.0 (Weston-super-Mare Stroud and Bath) Wiltshire 96.5 (Marlborough) 97.2 (Swindon and Witney) 102.2 (C...

Israeli professor of history (born 1951) Avner Ben-AmosBorn (1951-08-21) 21 August 1951 (age 72)Tel Aviv, IsraelNationalityIsraeliOccupation(s)Historian, academic, scholarAcademic backgroundAlma materHebrew University of Jerusalem, University of California, BerkeleyAcademic workInstitutionsTel Aviv UniversityMain interestsHistory of political rituals and national memory in Israel and France Avner Ben-Amos (born 1951) is an Israeli historian of education, an emeritus professor at Tel Aviv...

Former autonomous corporate entity in Scotland and Northern England For other uses, see Burgh (disambiguation). The Royal Burgh of Culross in Fife A burgh (/ˈbʌrə/ BURR-ə) is an autonomous municipal corporation in Scotland, usually a city, town, or toun in Scots. This type of administrative division existed from the 12th century, when King David I created the first royal burghs. Burgh status was broadly analogous to borough status, found in the rest of the United Kingdom. Following local ...

American silversmith and Patriot in the American Revolution This article is about the 18th-century American activist and artisan. For other uses, see Paul Revere (disambiguation). Not to be confused with Paul Rivière. Paul RevereJohn Singleton Copley, Portrait of Paul Revere. c. 1768–1770, Museum of Fine Arts, BostonBorn(1735-01-01)January 1, 1735(O.S.: December 21, 1734)North End, Boston, Massachusetts Bay, British AmericaDiedMay 10, 1818(1818-05-10) (aged 83)Boston, Massachusetts, U...

Pistol cartridge designed by SIG Sauer and Federal Premium Ammunition .357 SIG.357 SIG jacketed flat point cartridgeTypePistolPlace of originSwitzerlandUnited StatesProduction historyDesignerSIGARMS / Federal Premium AmmunitionDesigned1994Produced1994–presentSpecificationsParent case10mm AutoCase typeRimless, bottleneckBullet diameter9.02 mm (0.355 in)Land diameter8.71 mm (0.343 in)Neck diameter9.68 mm (0.381 in)Shoulder ...

Bank PrancisBanque de FranceKantor pusatParis, PrancisDidirikan18 Januari 1800GubernurFrançois Villeroy de GalhauNegaraPrancisSitus webwww.banque-france.fr1 Banque de France masih ada tetapi banyak fungsi yang diambil alih oleh ECB. Bank Prancis (bahasa Prancis: Banque de France), berkantor pusat di Paris, adalah bank sentral Prancis. Bank Prancis adalah lembaga independen, anggota Eurosystem sejak 1999. Tiga misi utamanya, sesuai dengan statusnya sebagai bank sentral, adalah untuk menggerak...

Theatre in London, home to the English National Opera This article is about the London theatre in St. Martin's Lane. For the former building to the east of Regent's Park, see London Colosseum. For other uses, see Coliseum Theatre (disambiguation). London ColiseumThe Coliseum TheatreLondon Coliseum Theatre of VarietiesLondon Coliseum in 2004AddressSt Martin's LaneLondon, WC2United KingdomCoordinates51°30′35″N 0°07′35″W / 51.509722°N 0.126389°W / 51.509722; -...

Oscillating flow effect resulting from fluid passing over a blunt body This article focuses only on one specialized aspect of the subject. Please help improve this article by adding general information and discuss at the talk page. (October 2019) Vortex shedding behind a circular cylinder. In this animation, the flows on the two sides of the cylinder are shown in different colors, to show that the vortices from the two sides alternate. Courtesy, Cesareo de La Rosa Siqueira. Vortex sheddi...

この記事のほとんどまたは全てが唯一の出典にのみ基づいています。 他の出典の追加も行い、記事の正確性・中立性・信頼性の向上にご協力ください。出典検索?: 獣戦士ガルキーバ – ニュース · 書籍 · スカラー · CiNii · J-STAGE · NDL · dlib.jp · ジャパンサーチ · TWL (2016年6月) 獣戦士ガルギーバ ジャンル アクション 漫画 原作�...







































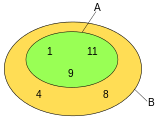
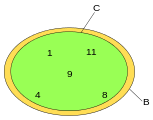
















![{\displaystyle [A]^{k}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/0fe283baada639c7008fb3a8612464d214ee1e5d)




![{\displaystyle [n]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a26847bfc29bbeb4d6ef62ac3fd076378c0fd1db)

![{\displaystyle [a]\subseteq [b].}](https://wikimedia.org/api/rest_v1/media/math/render/svg/86c0b0cba950301eacc0a11b017b876d2460a33f)
