Gonality of an algebraic curve
|
Read other articles:

Kegubernuran Ninawa Ibu kota Mosul Kota terbesar Kepala pemerintah Luas (km²) 37.323 Populasi (jiwa) 2.600.000 Kepadatan (/km²) 69 Bahasa Arab Kurdi Assyria Turkmen Shabaqi Komposisi etnis Kegubernuran Ninawa (Arab: نینوى, Kurdi: Neynewa) merupakan sebuah kegubernuran di Irak. Kegubernuran ini terletak di bagian baratlaut di negara itu. Kegubernuran ini memiliki luas wilayah 37.323 km² dengan memiliki jumlah penduduk 2.600.000 jiwa (2003). Ibu kotanya ialah Mosul. Distrik Akra A...
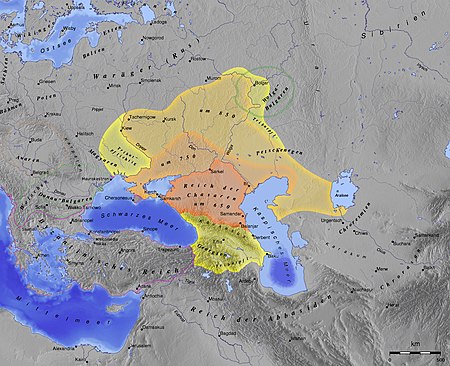
UkrainaУкраїнаUkrajina Flagga Statsvapen Valspråk: Volja, Zlahoda, Dobro(ukrainska för Frihet, enighet, godhet) Nationalsång: Ще не вмерла Україна (Sjtje ne vmerla Ukraina)(svenskaː Ännu lever Ukraina)Ukrainas nationalsång (instrumentalisk) läge Huvudstad(även största stad) Kiev Officiellt språk Ukrainska Statsskick Republik - President Volodymyr Zelenskyj - Premiärminister Denys Sjmyhal Självständighet Från Sovjetunione...

Untuk petinju Olimpiade Pakistan, lihat Ahmed Rashid (petinju). Ahmed RashidAhmed Rashid berpidato dalam sebuah acara di Chatham House pada Januari 2014Lahir1948 (umur 75–76)Rawalpindi, PakistanPekerjaanWartawan, penulis Ahmed Rashid (Urdu:احمد رشید; kelahiran 1948 di Pakistan) adalah seorang wartawan dan penulis banyak buku berpenjualan terbaik tentang kebijakan luar negeri di Afghanistan, Pakistan, dan Asia Tengah. Ahmed Rashid telah menikah, memiliki dua anak, dan tingga...

Mountain in Oregon, United States Steens MountainSteens Mountain near Andrews, OregonHighest pointElevation9,738 ft (2,968 m) NAVD 88[1]Prominence4,373 ft (1,333 m)[1]ListingOregon county high pointsNorth America isolated peaks 106thCoordinates42°38′11″N 118°34′36″W / 42.636418°N 118.576717°W / 42.636418; -118.576717[1]NamingEtymologyEnoch SteenGeographySteens MountainLocation in Oregon LocationHarn...

Berbagi berkas (Inggris: File sharing) adalah kegiatan mendistribusikan atau menyediakan akses ke media digital, seperti program komputer, multimedia (audio, gambar, dan video), dokumen-dokumen atau buku elektronik. Praktik distribusi berkas dapat dilakukan dalam beberapa cara. Metode yang umum digunakan adalah penyimpanan, transmisi dan penyebaran termasuk metode manual dengan memanfaatkan media penyimpanan portabel, server pada jaringan komputer, World Wide Web berbasis dokumen hyperlin...

Sabuk vulkanik Trans-Meksiko di Meksiko. Sabuk vulkanik adalah tempat aktif vulkanik yang besar, istilah lain digunakan untuk area aktivitas yang lebih kecil seperti lapangan vulkanik.[1] Sejak berdirinya teori lempeng tektonik, banyak ahli telah menetapkan model vulkanik global berdasarkan teori lempeng, mereka percaya bahwa sebagian besar gunung berapi tersebar pada batas lempeng tektonik dan beberapa di dalam lempeng.[2] Sabuk vulkanik ditemukan di atas zona suhu sangat tin...

ليك تشارلز الإحداثيات 30°12′53″N 93°12′31″W / 30.214722222222°N 93.208611111111°W / 30.214722222222; -93.208611111111 [1] تاريخ التأسيس 7 مارس 1861 تقسيم إداري البلد الولايات المتحدة[2][3] التقسيم الأعلى مقاطعة كالكاسيو خصائص جغرافية المساحة 116.301535 كيلومتر مرب...

Hansol Korea Open 2011 Sport Tennis Data 19 - 25 settembre Edizione 8ª Superficie Cemento Campioni Singolare María José Martínez Sánchez Doppio Natalie Grandin / Vladimíra Uhlířová 2010 2012 L' Hansol Korea Open 2011 è stato un torneo di tennis giocato sul cemento all'aperto. È stata l'8ª edizione del torneo che fa parte della categoria International nell'ambito del WTA Tour 2011. Il torneo si è giocato dal 19 al 25 settembre all' Olympic Park Tennis Center a Seul, Corea del sud...

Galaxy in the constellation Triangulum NGC 769SDSS image of NGC 769Observation data (J2000 epoch)ConstellationTriangulumRight ascension01h 59m 35.915s[1]Declination+30° 54′ 35.65″[1]Redshift0.0148Heliocentric radial velocity4404 km/sDistance177 Mly (54.2 Mpc)[2]Apparent magnitude (B)13.4[3]CharacteristicsTypeSc[4]Other designationsUGC 1467, MCG +05-05-037, PGC 7537[3] NGC 769 is a spiral galaxy located i...

Gereja Kristen Oikoumene di IndonesiaLogo GKOPenggolonganProtestanPemimpinPdt. Hermanus La Elu, Th.D.WilayahIndonesiaDidirikan29 Juli 1979 Depok, Jawa BaratSitus web resmihttps://sinodegko.org/ Gereja Kristen Oikoumene di Indonesia (disingkat GKO; bahasa Inggris: The Synod of The Ecumenical Christian Church in Indonesia) adalah kelompok gereja Kristen Protestan di Indonesia, yang didirikan dan berkantor pusat di Provinsi Jawa Barat. Sejarah GKO pertama kali didirikan di Depok tanggal 29 Juli ...

此條目可参照英語維基百科相應條目来扩充。 (2021年5月6日)若您熟悉来源语言和主题,请协助参考外语维基百科扩充条目。请勿直接提交机械翻译,也不要翻译不可靠、低品质内容。依版权协议,译文需在编辑摘要注明来源,或于讨论页顶部标记{{Translated page}}标签。 约翰斯顿环礁Kalama Atoll 美國本土外小島嶼 Johnston Atoll 旗幟颂歌:《星條旗》The Star-Spangled Banner約翰斯頓環礁�...

الانتداب الفرنسي على سوريا ولبنان الإحتلال الفرنسي على سوريا ولبنان 1920 – 1946 الانتداب الفرنسي على سوريا ولبنانعلم الانتداب الفرنسي على سورية ولبنان عاصمة دمشق، بيروت. نظام الحكم الانتداب الفرنسي اللغة الرسمية الفرنسية اللغة الفرنسية، العربية المندوب ال�...

Chiesa di San DomenicoStato Italia RegionePiemonte LocalitàTorino Coordinate45°04′28.56″N 7°40′55.13″E45°04′28.56″N, 7°40′55.13″E Religionecattolica di rito romano TitolareDomenico di Guzmán Arcidiocesi Torino Stile architettonicogotico Inizio costruzioneXIII secolo CompletamentoXV secolo Modifica dati su Wikidata · Manuale La chiesa di San Domenico è una chiesa ubicata nel centro di Torino, all'angolo dell'omonima via all'incrocio con via Milano. La facciata...

Main-belt asteroid 150 Nuwa3D convex shape model of 150 NuwaDiscoveryDiscovered byJ. C. WatsonDiscovery date18 October 1875DesignationsMPC designation(150) NuwaPronunciation/ˈnjuːwɑː/[citation needed]Named afterNüwaAlternative designationsA875 UA; 1908 AL;2002 JR70Minor planet categoryMain beltOrbital characteristics[1]Epoch 31 July 2016 (JD 2457600.5)Uncertainty parameter 0Observation arc116.94 yr (42714 d)Aphelion3.3586 AU (502.44 Gm)Perihe...

Australian politician (born 1970) The HonourablePeter DuttonMPDutton in 2023Leader of the OppositionIncumbentAssumed office 30 May 2022Prime MinisterAnthony AlbaneseDeputySussan LeyPreceded byAnthony AlbaneseLeader of the Liberal PartyIncumbentAssumed office 30 May 2022DeputySussan LeyPreceded byScott MorrisonMinister for DefenceIn office30 March 2021 – 23 May 2022Prime MinisterScott MorrisonDeputyAndrew HastiePreceded byLinda ReynoldsSucceeded byRichard MarlesLeader of the...

关于其他條目說明,請見「途易航空 (消歧义)」。 德国途易航空TUI fly Deutschland IATA ICAO 呼号 X3 TUI TUIJET 創立於1972年[1]以赫伯罗特航空之名重點機場汉诺威-朗根哈根机场 科隆-波恩机场 杜塞尔多夫机场 法兰克福机场 汉堡机场 慕尼黑机场 斯图加特机场飛行常客奖励计划老友薯条(曾用「蓝色里程」)机队数量38通航城市36母公司途易股份公司总部 德国�...

1986 United States Supreme Court caseBethel School District v. FraserSupreme Court of the United StatesArgued March 3, 1986Decided July 7, 1986Full case nameBethel School District No. 403 v. Matthew N. Fraser, a Minor, et al.Citations478 U.S. 675 (more)106 S. Ct. 3159; 92 L. Ed. 2d 549; 1986 U.S. LEXIS 139; 54 U.S.L.W. 5054Case historyPriorJudgment for plaintiff; affirmed, 755 F.2d 1356 (9th Cir. 1985); cert. granted, 474 U.S. 814 (1985).HoldingThe First Amendment, as applied through...
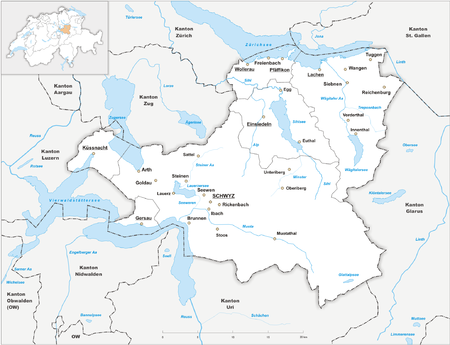
Canton of Switzerland CantonCanton of Schwyz Kanton Schwyz (German)Canton FlagCoat of armsBrandmarkLocation in Switzerland Map of Schwyz Coordinates: 47°4′N 8°45′E / 47.067°N 8.750°E / 47.067; 8.750CapitalSchwyzLargest cityFreienbachSubdivisions30 municipalities, 6 districtsGovernment • ExecutiveRegierungsrat (7) • LegislativeKantonsrat (100)Area[1] • Total907.89 km2 (350.54 sq mi)Population (...

Galaxy group in the constellation Antlia NGC 2997 group of galaxiesObservation data (Epoch )Constellation(s)Hydra / AntliaRight ascension09h 43m 54.7s[1]Declination−31° 31′ 10″[1]Distance24.8 millionNotable featuresNGC 2997 The NGC 2997 group is a group of galaxies about 24.8 million light-years from Earth containing NGC 2997 as a member. It is a group in the Local Supercluster along with the Local Group. References ^ a b NAME NGC 2997 Group. SIMBA...

Pour les articles homonymes, voir Allison. George Allison Biographie Nom George Frederick Allison Nationalité Anglais Naissance 24 octobre 1883 Hurworth-on-Tees (Angleterre) Décès 13 mars 1957 Londres (Angleterre) Parcours senior1 SaisonsClubsM (B.) Arsenal FC Parcours entraîneur AnnéesÉquipe Stats 1934-1947 Arsenal FC 1 Matchs de championnat uniquement. modifier George Allison, né le 24 octobre 1883 à Hurworth-on-Tees (Angleterre), mort le 13 mars 1957 à Londres (Anglete...