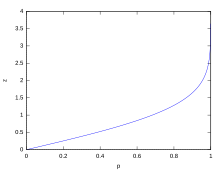
正規分布
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Read other articles:

Mourning GraveSutradaraOh In-chunProduserLee Jong-ho Ju Seong-ho Bang Mi-jeong Lee Suk-joonPemeranKang Ha-neul Kim So-eunSinematograferKwon Sang-junPenyuntingKim Chang-ju Kim Woo-hyeonPerusahaanproduksiGhost PicturesJupiter FilmsDistributorLittle Big Pictures9ers Entertainment (international)Tanggal rilis 3 Juli 2014 (2014-07-03) Durasi90 menitNegaraKorea SelatanBahasaKorea Mourning Grave (Hangul: 소녀괴담; RR: Sonyeogoedam; lit. Girl Ghost Story[1]) ada...

Elang harpy Harpia harpyja Status konservasiHampir terancamIUCN22695998 TaksonomiKerajaanAnimaliaFilumChordataKelasAvesOrdoAccipitriformesFamiliAccipitridaeGenusHarpiaSpesiesHarpia harpyja (Linnaeus, 1758) Tata namaSinonim takson Vultur harpyja Linnaeus, 1758 ProtonimVultur harpyja DistribusiThe harpy eagle is rare throughout its range, which extends from Mexico to Brazil (throughout its territory)[1] and Argentina (Only the north). lbs Elang harpy (Harpia harpyja) adalah spesies...

Karta med standardtidszoner i världen. En tidszon är ett område på jorden med samma normaltid, så kallad zontid. Eftersom jorden roterar kring sin egen axel, kan det aldrig bli samma klocktid eller soltid över hela klotet. Lokaltiden är bara gemensam för de platser som ligger på samma meridian. Platser som ligger längre österut och längre västerut har lokaltid som ligger före respektive efter. För att hålla samma tid inom länder eller områden har jorden blivit indelad i ett ...

Pour les articles homonymes, voir Brunhes. Jacques Brunhes Fonctions Secrétaire d'État au Tourisme 23 octobre 2001 – 6 mai 2002 (6 mois et 13 jours) Président Jacques Chirac Premier ministre Lionel Jospin Gouvernement Jospin Prédécesseur Michelle Demessine Successeur Gilles de Robien Maire de Gennevilliers 22 septembre 1987 – octobre 2001 (14 ans et 13 jours) Réélection 1989, 1995 Prédécesseur Lucien Lanternier Successeur Jacques Bourgoin Conseiller général...

Kubok Ukraïny 2012-2013Кубок України Competizione Kubok Ukraïny Sport Calcio Edizione 22ª Date dal 25 luglio 2012al 22 maggio 2013 Luogo Ucraina Partecipanti 55 Risultati Vincitore Šachtar(9º titolo) Secondo Čornomorec' Semi-finalisti Dnipro Sevastopol' Statistiche Miglior marcatore Luiz Adriano (4) Alex Teixeira (4) Incontri disputati 53 Gol segnati 148 (2,79 per incontro) Cronologia della competizione 2011-2012 2013-2014 Manu...

Jet airliner, produced 1965-1982 DC-9 A Northwest Airlines DC-9 in 2007. Role Narrow-body jet airlinerType of aircraft National origin United States Manufacturer Douglas Aircraft CompanyMcDonnell Douglas (from Aug. 1967) First flight February 25, 1965 Introduction December 8, 1965, with Delta Air Lines Status In limited service for cargo transport and private use Primary users USA Jet Airlines (historical)Aeronaves TSM Everts Air Cargo Northwest Airlines (historical) Produced 1965–1982...

Filipino artist (1912–1969) In this Philippine name, the middle name or maternal family name is Villaluz and the surname or paternal family name is Francisco. Botong FranciscoFrancisco on a 2012 stamp of the PhilippinesBornCarlos Modesto Villaluz Francisco[1](1912-11-04)November 4, 1912Angono, Rizal, Insular Government of the Philippine Islands, U.S.DiedMarch 31, 1969(1969-03-31) (aged 56)Angono, Rizal, PhilippinesResting placeAngono Catholic CemeteryNationalityFilipin...
Kypello Ellados 1954-1955 Competizione Coppa di Grecia Sport Calcio Edizione 13ª Date ? 1954 - 12 luglio 1955 Luogo Grecia Risultati Vincitore Panathīnaïkos(3º titolo) Secondo PAOK Cronologia della competizione 1953-1954 1955-1956 Manuale La Coppa di Grecia 1954-1955 è stata la 13ª edizione del torneo. La competizione è terminata il 12 luglio 1955. Il Panathinaikos ha vinto il trofeo per la terza volta, battendo in finale il PAOK. Indice 1 Ottavi di finale 2 Quarti d...

Lockheed XP-49 (Model perusahaan 522) adalah kemajuan pada P-38 Lightning untuk pejuang dalam menanggapi US Army Air Corps usulan 39-775. Dimaksudkan untuk penggunaan mesin Pratt & Whitney X-1800 baru 24-silinder, proposal ini, yang untuk pesawat besar serupa dengan P-38, ditugaskan penunjukan XP-49, sementara pesaingnya Grumman Model G-46 dianugerahi tempat kedua dan ditunjuk XP-50. Referensi Green, William. War Planes of the Second World War, Volume Four: Fighters. London: MacDonald &a...

Si ce bandeau n'est plus pertinent, retirez-le. Cliquez ici pour en savoir plus. Cet article ne cite pas suffisamment ses sources (juillet 2018). Si vous disposez d'ouvrages ou d'articles de référence ou si vous connaissez des sites web de qualité traitant du thème abordé ici, merci de compléter l'article en donnant les références utiles à sa vérifiabilité et en les liant à la section « Notes et références ». En pratique : Quelles sources sont attendues ? C...

本條目存在以下問題,請協助改善本條目或在討論頁針對議題發表看法。 此條目需要編修,以確保文法、用詞、语气、格式、標點等使用恰当。 (2013年8月6日)請按照校對指引,幫助编辑這個條目。(幫助、討論) 此條目剧情、虛構用語或人物介紹过长过细,需清理无关故事主轴的细节、用語和角色介紹。 (2020年10月6日)劇情、用語和人物介紹都只是用於了解故事主軸,輔助�...

Netball at the 2018 Commonwealth GamesVenueGold Coast Convention and Exhibition CentreCoomera Indoor Sports CentreDates5–15 April 2018Competitors144 from 12 nationsMedalists England Australia Jamaica← Glasgow 2014Birmingham 2022 → Netball was one of the ten core sports at the 2018 Commonwealth Games, which was held on the Gold Coast in Australia. This was the sixth staging of netball at the Commonwealth Games since...

Namma Metro's Green Line metro station Jalahalli Namma Metro stationJalahalli Metro Station Entrance AGeneral informationLocationBengaluru – Mangaluru Highway, Peenya, Bengaluru, Karnataka 560058Coordinates13°02′22″N 77°31′11″E / 13.039491°N 77.519703°E / 13.039491; 77.519703Owned byBangalore Metro Rail Corporation Ltd (BMRCL)Operated byNamma MetroLine(s)Green LinePlatformsSide platform Platform-1 → Nagasandra Platform-2 → Silk InstituteTracks2Connect...

Kejuaraan Dunia BWF 2025Informasi turnamenEdisike-29LevelInternasionalTempatpenyelenggaraanParis, Prancis ← Kopenhagen 2023 India 2026 → Kejuaraan Dunia BWF 2025 adalah turnamen bulu tangkis yang akan diadakan di Paris, Prancis. Pilihan kota tuan rumah Paris dianugerahi acara tersebut pada November 2018 saat pengumuman 18 tuan rumah acara bulutangkis utama dari 2019 hingga 2025.[1] Ringkasan medali Klasemen medali Klasemen medali Kejuaraan Dunia BWF 2025PeringkatNegaraEmasPerakPer...

Urban development in locations generating cost savings This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article is written like a personal reflection, personal essay, or argumentative essay that states a Wikipedia editor's personal feelings or presents an original argument about a topic. Please help improve it by rewriting it in an encyclopedic style. (January 2018) (Learn how and whe...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada November 2022. Artikel atau sebagian dari artikel ini mungkin diterjemahkan dari Top Spin (film) di en.wikipedia.org. Isinya masih belum akurat, karena bagian yang diterjemahkan masih perlu diperhalus dan disempurnakan. Jika Anda menguasai bahasa aslinya, harap pert...

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (فبراير 2020) الأسماء النائبة أو أسماء الحيز هي كلمات يمكنها الإشارة إلى ما لم يكن له اسم بعد، أو إلى ما كان اسمه منسيًا...

Senior Member of the Spanish Government Second Deputy Prime Minister of SpainVicepresidencia Segunda del Gobierno de EspañaCoat of arms of SpainIncumbentYolanda Díazsince 12 July 2021Government of SpainCouncil of MinistersStyleExcelentísimo/a Señor/aMember ofCabinetResidencePalacio de la MoncloaSeatMadrid, SpainNominatorPrime MinisterAppointerMonarchCountersigned by the Prime Minister of SpainTerm lengthNo fixed termNo term limits are imposed on the office.Constituting instrumentOrga...

1995 studio album by Tim SparksGuitar BazaarStudio album by Tim SparksReleased1995Recorded1995GenreFolk, worldLength47 minutes LabelAcoustic Music Records (31910802)ProducerTim Sparks, Jay M. FlemingTim Sparks chronology The Nutcracker Suite(1993) Guitar Bazaar(1995) One String Leads to Another(1999) Guitar Bazaar is the second solo recording by Tim Sparks on the Acoustic Music Records label, released in 1995.[1] History After a journey to Hungary, Yugoslavia and Bosnia in the...

2018 single by Calvin Harris featuring PartyNextDoorNuh Ready Nuh ReadySingle by Calvin Harris featuring PartyNextDoorReleased8 February 2018 (2018-02-08)StudioGod's Eyes Studios (Los Angeles, CA)JA Recording Studios (Santa Rosa, CA)GenreDancehall[1]Length3:04LabelSonySongwriter(s)Jahron BrathwaiteAdam WilesProducer(s)Calvin HarrisCalvin Harris singles chronology The Weekend (Funk Wav Remix) (2017) Nuh Ready Nuh Ready (2018) One Kiss (2018) PartyNextDoor singles chr...













![{\displaystyle E[(X-\mu )^{n}]={\begin{cases}0,&{\text{if }}n{\text{ is odd}}\\[1ex](n-1)!!\,\sigma ^{n},&{\text{if }}n{\text{ is even}}\end{cases}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/f71efddc3c16a51bfe5fd1e7f72682584adccfe4)


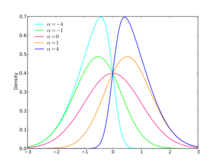

![{\displaystyle \Phi (x)=\int _{-\infty }^{x}\phi (t)\,dt={\frac {1}{2}}\left[1+\operatorname {erf} \left({\frac {x}{\sqrt {2}}}\right)\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/24d883a46c36e91409c002ec0a2b43dc9772e28a)