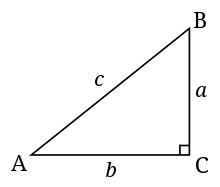
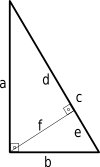
直角三角形
| |||||||||||||||||
Read other articles:

Pintu Madrasah Sankore Madrasah Sankoré, Universitas Sankoré, atau Masjid Sankore adalah pusat pembelajaran tertua yang terletak di Timbuktu, Mali, Afrika Barat. Pada tahun 1300, wanita Tuareg lokal, mendanai pembangunan Masjid Sankore. Di bawah kekuasaan Mandinka, masjid ini menarik pembelajar dari seluruh dunia muslim. Pranala luar Sankore Manuscript Project Diarsipkan 2010-11-27 di Wayback Machine. Ancient Manuscripts from the Desert Libraries of Timbuktu, Library of Congress — exhibit...

Disambiguazione – Se stai cercando altri significati, vedi Anversa (disambigua). Anversacomune(NL) Antwerpen(FR) Anvers Anversa – VedutaUna veduta di Anversa. LocalizzazioneStato Belgio Regione Fiandre Provincia Anversa ArrondissementAnversa AmministrazioneSindacoBart De Wever (N-VA) TerritorioCoordinate51°13′N 4°23′E / 51.216667°N 4.383333°E51.216667; 4.383333 (Anversa)Coordinate: 51°13′N 4°23′E / 51.216667°N 4.383333°E...

Gunung KīlaueaKerucut Retakan 8 di Kīlauea meletus pada pagi hari tanggal 28 Juni 2018Titik tertinggiKetinggian4.091 ft (1.247 m)[1]Puncak50 ft (15 m)[2]Koordinat19°25′16″N 155°17′12″W / 19.421097472°N 155.286762433°W / 19.421097472; -155.286762433Koordinat: 19°25′16″N 155°17′12″W / 19.421097472°N 155.286762433°W / 19.421097472; -155.286762433 [1]GeografiKīlaueaLetakHaw...
PemberitahuanTemplat ini mendeteksi bahwa artikel bahasa ini masih belum dinilai kualitasnya oleh ProyekWiki Bahasa dan ProyekWiki terkait dengan subjek. Perhatian: untuk penilai, halaman pembicaraan artikel ini telah diisi sehingga penilaian akan berkonflik dengan isi sebelumnya. Harap salin kode dibawah ini sebelum menilai. {{PW Bahasa|importance=|class=}} Terjadi false positive? Silakan laporkan kesalahan ini. 01.12, Kamis, 21 Maret, 2024 (UTC) • hapus singgahan Sebanyak ...

Perbandingan antara kosakata yang digunakan dalam basa Budak dengan ragam bahasa lainnya (tatakrama basa) dalam bahasa Sunda Basa budak atau basa Lemes keur budak (aksara Sunda: ᮘᮞ ᮘᮥᮓᮊ᮪, dapat diterjemahkan menjadi bahasa budak,[a] kadang hanya disebut sebagai Lemes Budak[2]) adalah sebuah istilah dalam bahasa Sunda untuk sejumlah kosakata yang digunakan oleh orang dewasa ketika berkomunikasi dengan anak-anak maupun sebaliknya.[3][4][5]&#...

Communist ideology developed by Joseph Stalin This article is about the political philosophy and state ideology. For countries governed by Marxist–Leninist parties, see Communist state. For the means of governing and related policies implemented by Joseph Stalin, see Stalinism. For Lenin's ideology in the form that existed in Lenin's own lifetime, see Leninism. Part of a series onMarxism–Leninism Concepts Administrative-command system Aggravation of class struggle under socialism Anti-imp...

1971 1989 Élections sénatoriales de 1980 en Ille-et-Vilaine 4 (+1) sièges de Sénateurs Le 28 septembre 1980 Type d’élection Élections sénatoriales Corps électoral et résultats Population 749 764 Inscrits au 1er tour 1 658 Votants au 1er tour 1 655 99,82 % Votes exprimés au 1er tour 1 641 Inscrits au 2d tour 1 658 Votants au 2d tour 1 654 99,76 % Votes exprimés au 2d tour 1 616 Sénateurs Sortant Élu Louis de La...

ロバート・デ・ニーロRobert De Niro 2011年のデ・ニーロ生年月日 (1943-08-17) 1943年8月17日(80歳)出生地 アメリカ合衆国・ニューヨーク州ニューヨーク市身長 177 cm職業 俳優、映画監督、映画プロデューサージャンル 映画、テレビドラマ活動期間 1963年 -配偶者 ダイアン・アボット(1976年 - 1988年)グレイス・ハイタワー(1997年 - )主な作品 『ミーン・ストリート』(1973年)...

Online encyclopedia Jewish Virtual LibraryType of siteReference work encyclopediaAvailable inEnglishOwnerMitchell Bard (via the American-Israeli Cooperative Enterprise)URLwww.jewishvirtuallibrary.orgCommercialNoLaunched1997; 27 years ago (1997)Current statusOnline The Jewish Virtual Library (JVL, formerly known as JSOURCE)[1] is an online encyclopedia published by the American foreign policy analyst Mitchell Bard's non-profit organization American–Israeli...

American newspaper publisher and politician (1773–1839) Solomon Southwick1804 portrait by Ezra Ames. Albany Institute of History & Art.Anti-Masonic Party Nominee for Governor of New YorkIn office1828Preceded byNoneSucceeded byFrancis GrangerPostmaster of Albany, New YorkIn office1821–1822Preceded byGerrit L. DoxSucceeded bySolomon Van RensselaerMember of the Board of Regents of the University of the State of New YorkIn office1812–1823Preceded byPeter GansevoortSucceeded byPeter Wend...

Politics of Sierra Leone Constitution Human rights Executive President (list) Julius Maada Bio Vice-President Mohamed Juldeh Jalloh Chief Minister (list) Jacob Jusu Saffa Cabinet Legislative Parliament Speaker: Abass Bundu Members of Parliament Judiciary Supreme Court Chief Justice: A. H. Charm Human rights Elections Political parties Recent elections General: 201220182023 Administrative divisions Provinces Districts Chiefdoms Foreign relations Ministry of Foreign Affairs and International Co...

Sceaux 行政国 フランス地域圏 (Région) イル=ド=フランス地域圏県 (département) オー=ド=セーヌ県郡 (arrondissement) アントニー郡小郡 (canton) 小郡庁所在地INSEEコード 92071郵便番号 92330市長(任期) フィリップ・ローラン(2008年-2014年)自治体間連合 (fr) メトロポール・デュ・グラン・パリ人口動態人口 19,679人(2007年)人口密度 5466人/km2住民の呼称 Scéens地理座標 北緯48度4...

André DeflineFonctionInspecteur général des carrièresBiographieNaissance 19 avril 1876Bruay-sur-l'EscautDécès 8 mars 1945 (à 68 ans)Billy-MontignyNom de naissance André Louis Joseph Ghislain DeflineNationalité françaiseFormation École polytechniqueÉcole nationale supérieure des mines de ParisActivités Ingénieur, chef d'entrepriseParentèle Pierre Ceyrac (petit-fils)Autres informationsDistinction Officier de la Légion d'honneur (1922)modifier - modifier le code - m...

GER Class R24 LNER Class J67On a foggy January Saturday, 18 January 1947, Holden J67 0-6-0T No. 8590 of 1899, is struggling across a turntable with a string of dead locomotives (D15 'Claud' 4-4-0 No. 2509, J15 0-6-0 No. 5374 and D16/3 'Super Claud' 4-4-0 No. 2608).Type and originPower typeSteamDesignerJames HoldenBuilderStratford WorksBuild date1890–1901Total produced140SpecificationsConfiguration: • Whyte0-6-0T • UICC n2tGauge4 ft 8+1⁄2 ...

Voce principale: Christopher Lee. Christopher Lee ai Women's World Awards 2009. Christopher Lee è stato un attore e cantante britannico. Nella quasi settantennale carriera, ha ricoperto molti ruoli, principalmente quello dell'antagonista. Tra i personaggi più noti da lui interpretati, vi sono Dracula (che ha interpretato per dodici volte), il Conte Dooku nella trilogia prequel di Guerre stellari, e lo stregone Saruman il Bianco nelle trilogie de Il Signore degli Anelli e de Lo Hobbit. Ha i...

2016年美國總統選舉 ← 2012 2016年11月8日 2020 → 538個選舉人團席位獲勝需270票民意調查投票率55.7%[1][2] ▲ 0.8 % 获提名人 唐納·川普 希拉莉·克林頓 政党 共和黨 民主党 家鄉州 紐約州 紐約州 竞选搭档 迈克·彭斯 蒂姆·凱恩 选举人票 304[3][4][註 1] 227[5] 胜出州/省 30 + 緬-2 20 + DC 民選得票 62,984,828[6] 65,853,514[6]...

Overview of Oregon river drainage basins This is a partial listing of rivers in the state of Oregon, United States. This list of Oregon rivers is organized alphabetically and by tributary structure. The list may also include streams known as creeks, brooks, forks, branches and prongs, as well as sloughs and channels. A list of rivers of the Americas and a list of Pacific Ocean coast rivers of the Americas are also available, as is a list of Oregon lakes. Alphabetical listing Abiqua Creek Age...

American educator and activist (1886–1972) Frank Porter GrahamFrank Porter Graham, 1945United States Senatorfrom North CarolinaIn officeMarch 29, 1949 – November 26, 1950Appointed byW. Kerr ScottPreceded byMelville BroughtonSucceeded byWillis SmithPresident of the University of North Carolina SystemIn office1930–1949Preceded byHarry Woodburn ChaseSucceeded byGordon Gray Personal detailsBorn(1886-10-14)October 14, 1886Fayetteville, North Carolina, U.S.DiedFebruary 16, 1972(1972-...

Photographer and historian (1913 –1995) Helmut GernsheimFelix H. Man (1945) Portrait of Helmut GernsheimBorn1 March 1913Munich, GermanyDied20 July 1995 (aged 82)SwitzerlandEducationLudwig Maximilian University of Munich, State School of Photography, MunichOccupation(s)photographer and photo historianKnown forphotography collectionSpouses Alison Eames Irene Guenin Helmut Erich Robert Kuno Gernsheim (1 March 1913 – 20 July 1995)[1] was a historian of photography, a collector an...

American jazz composer and bandleader (1914–1993) This article is about the jazz musician, Sun Ra. For the hip hop artist also known as Sun R. A., see Sun Rise Above. Sun RaSun Ra, c. 1973Background informationBirth nameHerman Poole BlountAlso known asLe Sony'r Ra (legal name)Born(1914-05-22)May 22, 1914Birmingham, Alabama, U.S.DiedMay 30, 1993(1993-05-30) (aged 79)Birmingham, Alabama, U.S.Genres Jazz experimental space[1] Occupation(s) Bandleader composer arranger artist ...