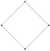
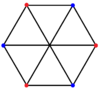
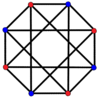
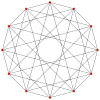
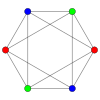
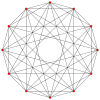
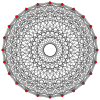
Cross-polytope

Cross-polytopes of dimension 2 to 5
A 2-dimensional cross-polytope A 3-dimensional cross-polytope
2 dimensions
square 		3 dimensions
octahedron
A 4-dimensional cross-polytope A 5-dimensional cross-polytope
4 dimensions
16-cell 		5 dimensions
5-orthoplex

In geometry, a cross-polytope,[1] hyperoctahedron, orthoplex,[2] staurotope,[3] or cocube is a regular, convex polytope that exists in n-dimensional Euclidean space. A 2-dimensional cross-polytope is a square, a 3-dimensional cross-polytope is a regular octahedron, and a 4-dimensional cross-polytope is a 16-cell. Its facets are simplexes of the previous dimension, while the cross-polytope's vertex figure is another cross-polytope from the previous dimension.

The vertices of a cross-polytope can be chosen as the unit vectors pointing along each co-ordinate axis – i.e. all the permutations of (±1, 0, 0, ..., 0). The cross-polytope is the convex hull of its vertices. The n-dimensional cross-polytope can also be defined as the closed unit ball (or, according to some authors, its boundary) in the ℓ1-norm on Rn:

In 1 dimension the cross-polytope is simply the line segment [−1, +1], in 2 dimensions it is a square (or diamond) with vertices {(±1, 0), (0, ±1)}. In 3 dimensions it is an octahedron—one of the five convex regular polyhedra known as the Platonic solids. This can be generalised to higher dimensions with an n-orthoplex being constructed as a bipyramid with an (n−1)-orthoplex base.

The cross-polytope is the dual polytope of the hypercube. The 1-skeleton of an n-dimensional cross-polytope is the Turán graph T(2n, n) (also known as a cocktail party graph [4]).

4 dimensions

The 4-dimensional cross-polytope also goes by the name hexadecachoron or 16-cell. It is one of the six convex regular 4-polytopes. These 4-polytopes were first described by the Swiss mathematician Ludwig Schläfli in the mid-19th century.

Higher dimensions

The cross-polytope family is one of three regular polytope families, labeled by Coxeter as βn, the other two being the hypercube family, labeled as γn, and the simplex family, labeled as αn. A fourth family, the infinite tessellations of hypercubes, he labeled as δn.[5]

The n-dimensional cross-polytope has 2n vertices, and 2n facets ((n − 1)-dimensional components) all of which are (n − 1)-simplices. The vertex figures are all (n − 1)-cross-polytopes. The Schläfli symbol of the cross-polytope is {3,3,...,3,4}.

The dihedral angle of the n-dimensional cross-polytope is . This gives: δ2 = arccos(0/2) = 90°, δ3 = arccos(−1/3) = 109.47°, δ4 = arccos(−2/4) = 120°, δ5 = arccos(−3/5) = 126.87°, ... δ∞ = arccos(−1) = 180°.

The hypervolume of the n-dimensional cross-polytope is

For each pair of non-opposite vertices, there is an edge joining them. More generally, each set of k + 1 orthogonal vertices corresponds to a distinct k-dimensional component which contains them. The number of k-dimensional components (vertices, edges, faces, ..., facets) in an n-dimensional cross-polytope is thus given by (see binomial coefficient):

[6]

The extended f-vector for an n-orthoplex can be computed by (1,2)n, like the coefficients of polynomial products. For example a 16-cell is (1,2)4 = (1,4,4)2 = (1,8,24,32,16).

There are many possible orthographic projections that can show the cross-polytopes as 2-dimensional graphs. Petrie polygon projections map the points into a regular 2n-gon or lower order regular polygons. A second projection takes the 2(n−1)-gon petrie polygon of the lower dimension, seen as a bipyramid, projected down the axis, with 2 vertices mapped into the center.

Cross-polytope elements
n βn
k11 		Name(s)
Graph 		Graph
2n-gon 		Schläfli Coxeter-Dynkin
diagrams 		Vertices Edges Faces Cells 4-faces 5-faces 6-faces 7-faces 8-faces 9-faces 10-faces
0 β0 Point
0-orthoplex 		. ( )
1                    
1 β1 Line segment
1-orthoplex 		{ }
2 1                  
2 β2
−111 		Square
2-orthoplex
Bicross 		{4}
2{ } = { }+{ }
4 4 1                
3 β3
011 		Octahedron
3-orthoplex
Tricross 		{3,4}
{31,1}
3{ }

6 12 8 1              
4 β4
111 		16-cell
4-orthoplex
Tetracross 		{3,3,4}
{3,31,1}
4{ }

8 24 32 16 1            
5 β5
211 		5-orthoplex
Pentacross 		{33,4}
{3,3,31,1}
5{ }

10 40 80 80 32 1          
6 β6
311 		6-orthoplex
Hexacross 		{34,4}
{33,31,1}
6{ }

12 60 160 240 192 64 1        
7 β7
411 		7-orthoplex
Heptacross 		{35,4}
{34,31,1}
7{ }

14 84 280 560 672 448 128 1      
8 β8
511 		8-orthoplex
Octacross 		{36,4}
{35,31,1}
8{ }

16 112 448 1120 1792 1792 1024 256 1    
9 β9
611 		9-orthoplex
Enneacross 		{37,4}
{36,31,1}
9{ }

18 144 672 2016 4032 5376 4608 2304 512 1  
10 β10
711 		10-orthoplex
Decacross 		{38,4}
{37,31,1}
10{ }

20 180 960 3360 8064 13440 15360 11520 5120 1024 1
...
n βn
k11 		n-orthoplex
n-cross 		{3n − 2,4}
{3n − 3,31,1}
n{} 		...
...
... 		2n 0-faces, ... k-faces ..., 2n (n−1)-faces

The vertices of an axis-aligned cross polytope are all at equal distance from each other in the Manhattan distance (L1 norm). Kusner's conjecture states that this set of 2d points is the largest possible equidistant set for this distance.[7]

Generalized orthoplex

Regular complex polytopes can be defined in complex Hilbert space called generalized orthoplexes (or cross polytopes), βp
n = 2{3}2{3}...2{4}p, or ... Real solutions exist with p = 2, i.e. β2
n = βn = 2{3}2{3}...2{4}2 = {3,3,..,4}. For p > 2, they exist in . A p-generalized n-orthoplex has pn vertices. Generalized orthoplexes have regular simplexes (real) as facets.[8] Generalized orthoplexes make complete multipartite graphs, βp
2 make Kp,p for complete bipartite graph, βp
3 make Kp,p,p for complete tripartite graphs. βp
n creates Kpn. An orthogonal projection can be defined that maps all the vertices equally-spaced on a circle, with all pairs of vertices connected, except multiples of n. The regular polygon perimeter in these orthogonal projections is called a petrie polygon.

Generalized orthoplexes
p = 2 p = 3 p = 4 p = 5 p = 6 p = 7 p = 8

2{4}2 = {4} =
K2,2
2{4}3 =
K3,3
2{4}4 =
K4,4
2{4}5 =
K5,5
2{4}6 =
K6,6
2{4}7 =
K7,7
2{4}8 =
K8,8

2{3}2{4}2 = {3,4} =
K2,2,2
2{3}2{4}3 =
K3,3,3
2{3}2{4}4 =
K4,4,4
2{3}2{4}5 =
K5,5,5
2{3}2{4}6 =
K6,6,6
2{3}2{4}7 =
K7,7,7
2{3}2{4}8 =
K8,8,8

2{3}2{3}2
{3,3,4} =
K2,2,2,2
2{3}2{3}2{4}3

K3,3,3,3
2{3}2{3}2{4}4

K4,4,4,4
2{3}2{3}2{4}5

K5,5,5,5
2{3}2{3}2{4}6

K6,6,6,6
2{3}2{3}2{4}7

K7,7,7,7
2{3}2{3}2{4}8

K8,8,8,8

2{3}2{3}2{3}2{4}2
{3,3,3,4} =
K2,2,2,2,2
2{3}2{3}2{3}2{4}3

K3,3,3,3,3
2{3}2{3}2{3}2{4}4

K4,4,4,4,4
2{3}2{3}2{3}2{4}5

K5,5,5,5,5
2{3}2{3}2{3}2{4}6

K6,6,6,6,6
2{3}2{3}2{3}2{4}7

K7,7,7,7,7
2{3}2{3}2{3}2{4}8

K8,8,8,8,8

2{3}2{3}2{3}2{3}2{4}2
{3,3,3,3,4} =
K2,2,2,2,2,2
2{3}2{3}2{3}2{3}2{4}3

K3,3,3,3,3,3
2{3}2{3}2{3}2{3}2{4}4

K4,4,4,4,4,4
2{3}2{3}2{3}2{3}2{4}5

K5,5,5,5,5,5
2{3}2{3}2{3}2{3}2{4}6

K6,6,6,6,6,6
2{3}2{3}2{3}2{3}2{4}7

K7,7,7,7,7,7
2{3}2{3}2{3}2{3}2{4}8

K8,8,8,8,8,8

Cross-polytopes can be combined with their dual cubes to form compound polytopes:

  • In two dimensions, we obtain the octagrammic star figure {⁠8/2⁠},
  • In three dimensions we obtain the compound of cube and octahedron,
  • In four dimensions we obtain the compound of tesseract and 16-cell.

See also

Citations

  1. ^ Coxeter 1973, pp. 121–122, §7.21. illustration Fig 7-2B.
  2. ^ Conway, J. H.; Sloane, N. J. A. (1991). "The Cell Structures of Certain Lattices". In Hilton, P.; Hirzebruch, F.; Remmert, R. (eds.). Miscellanea Mathematica. Berlin: Springer. pp. 89–90. doi:10.1007/978-3-642-76709-8_5. ISBN 978-3-642-76711-1.
  3. ^ McMullen, Peter (2020). Geometric Regular Polytopes. Cambridge University Press. p. 92. ISBN 978-1-108-48958-4.
  4. ^ Weisstein, Eric W. "Cocktail Party Graph". MathWorld.
  5. ^ Coxeter 1973, pp. 120–124, §7.2.
  6. ^ Coxeter 1973, p. 121, §7.2.2..
  7. ^ Guy, Richard K. (1983), "An olla-podrida of open problems, often oddly posed", American Mathematical Monthly, 90 (3): 196–200, doi:10.2307/2975549, JSTOR 2975549.
  8. ^ Coxeter, Regular Complex Polytopes, p. 108

References

  • Coxeter, H.S.M. (1973). Regular Polytopes (3rd ed.). New York: Dover.
    • pp. 121-122, §7.21. see illustration Fig 7.2B
    • p. 296, Table I (iii): Regular Polytopes, three regular polytopes in n-dimensions (n≥5)
Wikimedia Commons has media related to Cross-polytope graphs.
Fundamental convex regular and uniform polytopes in dimensions 2–10
Family An Bn I2(p) / Dn E6 / E7 / E8 / F4 / G2 Hn
Regular polygon Triangle Square p-gon Hexagon Pentagon
Uniform polyhedron Tetrahedron Octahedron • Cube Demicube Dodecahedron • Icosahedron
Uniform polychoron Pentachoron 16-cell • Tesseract Demitesseract 24-cell 120-cell • 600-cell
Uniform 5-polytope 5-simplex 5-orthoplex • 5-cube 5-demicube
Uniform 6-polytope 6-simplex 6-orthoplex • 6-cube 6-demicube 122 • 221
Uniform 7-polytope 7-simplex 7-orthoplex • 7-cube 7-demicube 132 • 231 • 321
Uniform 8-polytope 8-simplex 8-orthoplex • 8-cube 8-demicube 142 • 241 • 421
Uniform 9-polytope 9-simplex 9-orthoplex • 9-cube 9-demicube
Uniform 10-polytope 10-simplex 10-orthoplex • 10-cube 10-demicube
Uniform n-polytope n-simplex n-orthoplex • n-cube n-demicube 1k2 • 2k1 • k21 n-pentagonal polytope
Topics: Polytope families • Regular polytope • List of regular polytopes and compounds

Read other articles:

羅生門 (電影)

本條目存在以下問題，請協助改善本條目或在討論頁針對議題發表看法。 此條目需要补充更多来源。 (2018年3月17日)请协助補充多方面可靠来源以改善这篇条目，无法查证的内容可能會因為异议提出而被移除。致使用者：请搜索一下条目的标题（来源搜索：羅生門 (電影) — 网页、新闻、书籍、学术、图像），以检查网络上是否存在该主题的更多可靠来源（判定指引）。 �...

 

روفوس هل جودي في قاعة كارنيجي

هذه مقالة غير مراجعة. ينبغي أن يزال هذا القالب بعد أن يراجعها محرر؛ إذا لزم الأمر فيجب أن توسم المقالة بقوالب الصيانة المناسبة. يمكن أيضاً تقديم طلب لمراجعة المقالة في الصفحة المخصصة لذلك. (فبراير 2021) هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة �...

 

Élections législatives françaises de 1820

1819 1824 Élections législatives françaises de 1820 434 députés 4 et 13 novembre 1820 Type d’élection Élections législatives Corps électoral et résultats Votants 94 000 Doctrinaires – Armand-Emmanuel du Plessis de Richelieu Voix 42 300 44,70 %   8 Députés élus 194  58 Ultraroyalistes – Jean-Baptiste de Villèle Voix 34 780 36,87 %   1,2 Députés élus 160  68 Libéraux – Benjam...

Mesin pecok

Jackson 6700 Plasser & Theurer 09-16 CSM Mesin pecok adalah mesin yang digunakan untuk memecok (atau memadatkan) balast di bawah rel kereta api agar rel lebih tahan lama. Sebelum pemecok mekanik diperkenalkan, tugas pemecokan dilakukan oleh para pekerja dengan bantuan alat pemadat. Karena lebih cepat, lebih akurat, lebih efisien, dan lebih hemat tenaga, mesin pecok berperan penting untuk penggunaan bantalan rel beton karena terlalu berat (biasanya lebih dari 250 kg (551 pon)) ji...

 

2020 South Dakota Senate election

2020 South Dakota Senate election ← 2018 November 3, 2020 2022 → All 35 seats in the South Dakota Senate18 seats needed for a majorityTurnout64.31%[1]   Majority party Minority party   Leader Kris Langer(retiring) Troy Heinert Party Republican Democratic Leader's seat 25th district 26th district Seats before 30 5 Seats won 32 3 Seat change 2 2 Popular vote 271,017 78,110 Percentage 72.83% 20.99% Results by gains and holds Resul...

 

العلاقات الكونغوية المدغشقرية

العلاقات الكونغوية المدغشقرية مدغشقر   جمهورية الكونغو   مدغشقر تعديل مصدري - تعديل   العلاقات الكونغوية المدغشقرية هي العلاقات الثنائية التي تجمع بين جمهورية الكونغو ومدغشقر.[1][2][3][4][5] مقارنة بين البلدين هذه مقارنة عامة ومرجعية للدولتين...

Bendera Maroko

Bendera Maroko Perbandingan 2:3 Dipakai 17 November 1915 Bendera Maroko terdiri dari sebuah bintang berwarna hijau pada sebuah latar belakang berwarna merah. Bendera baru ini ditampilkan pada hari kemerdekaan Maroko pada tahun 1956. Pada abad ke-17 bendera ini digunakan oleh Dinasti Alawi. Bendera bersejarah Bendera Maroko pada masa Dinasti Idrisid Bendera Maroko pada masa Dinasti Almoravid Bendera Maroko pada masa Kekhalifahan Almohad Bendera Maroko pada masa Dinasti Marinid Bendera Maroko ...

 

Staines West railway station

Former railway station in Surrey, England Staines WestMain building seen from the south in 2017General informationLocationStaines-upon-Thames, SpelthorneEnglandGrid referenceTQ033718Platforms1Other informationStatusDisusedHistoryOriginal companyStaines & West Drayton RailwayPre-groupingGreat Western RailwayPost-groupingGreat Western RailwayKey dates2 November 1885 (1885-11-02)Opened as Staines26 September 1949Renamed Staines West29 March 1965 (1965-03-29)Clos...

 

Billy Bathgate (film)

1991 film by Robert Benton Billy BathgateOriginal Theatrical PosterDirected byRobert BentonScreenplay byTom StoppardBased onBilly Bathgateby E.L. DoctorowProduced byRobert F. ColesburyArlene DonovanStarring Dustin Hoffman Nicole Kidman Steven Hill Loren Dean Bruce Willis CinematographyNéstor AlmendrosEdited byAlan HeimDavid RayRobert M. ReitanoMusic byMark IshamProductioncompanyTouchstone PicturesDistributed byBuena Vista Pictures DistributionRelease date November 1, 1991 (199...

Exhaustion of remedies

This article includes a list of references, related reading, or external links, but its sources remain unclear because it lacks inline citations. Please help improve this article by introducing more precise citations. (November 2020) (Learn how and when to remove this message) Administrative law of the United States General Rulemaking Notice of proposed rulemaking Adjudication Administrative law judge Code of Federal Regulations Federal Register Statutory framework Administrative Procedure Ac...

 

Auguste Bouché-Leclercq

Pour les articles homonymes, voir Bouché et Leclercq. Auguste Bouché-LeclercqAuguste Bouché-Leclercq.BiographieNaissance 30 juillet 1842FrancièresDécès 19 juillet 1923 (à 80 ans)Nogent-sur-MarneNationalité françaiseFormation Faculté des lettres de ParisActivités Historien, érudit classique, professeur d'universitéEnfant Emile Bouché-Leclercq (d)Autres informationsA travaillé pour Université de MontpellierFaculté des lettres de ParisMembre de Académie des inscriptions e...

 

Binary operation

Mathematical operation with two operands Not to be confused with Bitwise operation. A binary operation ∘ {\displaystyle \circ } is a rule for combining the arguments x {\displaystyle x} and y {\displaystyle y} to produce x ∘ y {\displaystyle x\circ y} In mathematics, a binary operation or dyadic operation is a rule for combining two elements (called operands) to produce another element. More formally, a binary operation is an operation of arity two. More specifically, a binary o...

الفرقاطة العثمانية أرطغرل

رحلة الفرقاطة أرطغرل إلى اليابان بقيادة أمير اللواء (بالتركية: Mirliva)‏ علي عثمان باشا. اللوحة محفوظة في متحف إسطنبول البحري (بالتركية: İstanbul Deniz Müzesi)‏ في بشكطاش (بالتركية: Beşiktaş)‏ باسطنبول. اللوحة من رسم خيال العميد بحري عثمان نوري باشا (1839-1906م). أمير اللواء (بالتركية: Mirliva)‏ عل...

 

María de las Mercedes, Princess of Asturias

Princess of Asturias (1880–1904) María de las MercedesPrincess of AsturiasBorn(1880-09-11)11 September 1880Royal Palace of Madrid, Madrid, SpainDied17 October 1904(1904-10-17) (aged 24)MadridBurialEl EscorialSpouse Prince Carlos of the Two Sicilies ​ ​(m. 1901)​Issue Infante Alfonso Infante Fernando Isabel Alfonsa, Countess Zamoyska NamesMaría de las Mercedes Isabel Teresa Cristina AlfonsaHouseBourbonFatherAlfonso XII of SpainMotherMaria Christina o...

 

Veneziana (dolce)

VenezianaVeneziana monoporzione con granelli di zuccheroOriginiLuogo d'origine Italia RegioneLombardia DettagliCategoriadolce Ingredienti principali farina zucchero uova burro Varianti con o senza arancia candita o aroma di arancia con copertura di granella di zucchero, glassa di mandorle o crema pasticcera lievito di birra al posto della pasta madre miele nell'impasto La veneziana è un dolce a impasto lievitato della cucina milanese, ricoperto da granella di zucchero[1] e/o gla...

Список округов Кентукки

Карта округов Кентукки Американский штат Кентукки административно разделён на 120 округов, которые создавались с 1780 по 1912 год. По данным на 2020 год[1] население штата составляло 4 505 836 человек, то есть в одном округе в среднем проживало 37 549 человек. Площадь штата �...

 

シダックス

この項目では、持株会社について説明しています。カラオケボックスのシダックスについては「シダックス・コミュニティー」を、タレントのしだっくすについては「B2takes!!」を、かつてシダックス(sidux)と呼ばれていたLinuxディストリビューションについては「aptosid」をご覧ください。 シダックス株式会社SHiDAX CORPORATION種類 株式会社市場情報 東証スタンダード 48372001...

 

استثمار بديل

الاستثمار البديل أو صندوق الاستثمار البديل (AIF) هو استثمار أو صندوق يستثمر في فئات الأصول البديلة عن الأسهم والسندات و النقدية المعادلة. إن المصطلح واسع نسبياً ويتضمن أصول ملموسة مثل المعادن النفيسة والأعمال الفنية والنبيذ والتحف والعملات المعدنية أو حتى الطوابع وبعض الأ...

パロディ

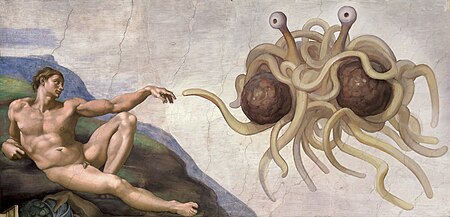
星野源の楽曲については「夢の外へ」を、ジャニーズWESTの楽曲については「しあわせの花」をご覧ください。 ミケランジェロ作『アダムの創造』のパロディ作品。パロディ宗教（英語版）の空飛ぶスパゲッティ・モンスター教を象徴する[1]。 パロディ（英語: parody、ギリシア語: παρωδία）とは、他者によって創作された文学や音楽、美術、演説などを模...

 

École nationale du génie de l'eau et de l'environnement de Strasbourg

École nationale du génie de l'eau et de l'environnement de StrasbourgTypePublicEstablished1952LocationStrasbourg, France48°34′51″N 7°45′31″E / 48.58084507°N 7.75859931°E / 48.58084507; 7.75859931AffiliationsConférence des Grandes ÉcolesWebsiteengees.unistra.frLocation in StrasbourgShow map of StrasbourgÉcole nationale du génie de l'eau et de l'environnement de Strasbourg (Alsace)Show map of AlsaceÉcole nationale du génie de l'eau et de l'environneme...