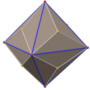
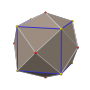
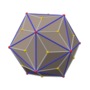
四角化菱形三十面體
| ||||||||||||||||||||||||||||||||||||||||||||||||||||
Read other articles:

Republik Persatuan Myanmarပြည်ထောင်စု သမ္မတ မြန်မာနိုင်ငံတော် Pyidaunzu Thanmăda Myănma Nainngandaw (Burma) Bendera Lambang Semboyan: —Lagu kebangsaan: ကမ္ဘာမကျေ Kambha Ma Kye (Indonesia: Hingga Dunia Berakhir) Perlihatkan BumiPerlihatkan peta ASEANPerlihatkan peta BenderaLokasi Myanmar (hijau)di ASEAN (abu-abu tua) – [Legenda]Ibu kotaNaypyida...

Pirate who operates along a river The USS Panay, a United States Navy river gunboat, part of the brown-water navy, which served on the Yangtze Patrol, hunting for river pirates and Chinese insurgents, on the Yangtze River, in China. The Imperial Japanese Army ultimately sunk the Panay in 1937, known as the Panay Incident. A river pirate is a pirate who operates along a river. The term has been used to describe many different kinds of pirate groups who carry out riverine attacks in Asia, Afric...

Klasemen medaliOlimpiade Musim Panas 1936LokasiBerlin, JermanIkhtisarPerolehan medali emas terbanyak Jerman (101)Perolehan jumlah medali terbanyak Jerman (38)← 1932 Klasemen medali1948 → Klasemen medali Olimpiade Musim Panas 1936 adalah klasemen medali selama pelaksanaan Olimpiade Musim Panas 1936. Olimpiade ini berlangsung di Berlin, Jerman sejak 1 hingga 16 Agustus 1936. Terdapat 3.963 atlet dari 49 Komite Olimpiade Nasional (KON) yang bertanding dalam 129 no...

Cet article est une ébauche concernant l’histoire, la Nouvelle-France et le Québec. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Seigneurie de MontarvilleGéographiePays CanadaProvince QuébecRégion administrative MontérégieTerritoire équivalent agglomération de LongueuilVille Saint-Bruno-de-MontarvilleCoordonnées 45° 32′ 00″ N, 73° 21′ 00″ OFonctionneme...

WW2 German Army division 24th Panzer Division24. Panzer-DivisionUnit insignia 1943Active28 November 1941 – 8 May 1945Country Nazi GermanyBranch German ArmyTypePanzerRoleArmoured warfareSizeDivisionGarrison/HQWehrkreis III: Frankfurt an der OderAnniversariesin former East GermanyEngagementsWorld War II Case Blue Battle of Stalingrad CommandersNotablecommandersGeneral of the Cavalry (Germany) Kurt FeldtInsigniaInsignia 1941–1942Insignia 1943–1945Military unit The 24th Panzer Div...

Об экономическом термине см. Первородный грех (экономика). ХристианствоБиблия Ветхий Завет Новый Завет Евангелие Десять заповедей Нагорная проповедь Апокрифы Бог, Троица Бог Отец Иисус Христос Святой Дух История христианства Апостолы Хронология христианства Ран�...

British Lions & Scotland international rugby union player Rugby playerSirIan McGeechanOBEDate of birth (1946-10-30) 30 October 1946 (age 77)Place of birthHeadingley, Leeds, Yorkshire, EnglandUniversityCarnegie Physical Training CollegeOccupation(s)Teacher Director of rugby, Head coachRugby union careerPosition(s) Fly-half, Outside centreSenior careerYears Team Apps (Points)1964–1979 Headingley ()International careerYears Team Apps (Points)1972–1979 Scotland 32 (21)1974 & 1977...

Romanian national radio station This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article is in list format but may read better as prose. You can help by converting this article, if appropriate. Editing help is available. (May 2018) This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may ...

Pleione in the Pleiades is a shell star. A shell star is a star having a spectrum that shows extremely broad absorption lines, plus some very narrow absorption lines. They typically also show some emission lines, usually from the Balmer series but occasionally of other lines. The broad absorption lines are due to rapid rotation of the photosphere, the emission lines from an equatorial disk, and the narrow absorption lines are produced when the disc is seen nearly edge-on. Shell stars have spe...

Ini adalah nama Korea; marganya adalah Kim. Kim Sung-ryungKim pada April 2018Nama asal김성령Lahir8 Februari 1967 (umur 57)Seoul, Korea SelatanNama lainKim Sung-ryoungPendidikanInstitut Teknologi InhaUniversitas Kyung HeeUniversitas Kajian Luar Negeri HankukPekerjaanPemeranTahun aktif1988–sekarangTinggi168 m (551 ft 2 in)GelarMiss Korea 1988Suami/istriLee Ki-soo (m. 1996)Anak2 Pemenang kontes kecantikanJumlah film16 (pada A...

Elezioni parlamentari in Ucraina del 1998 Stato Ucraina Data 29 marzo Assemblea Verchovna Rada Liste KPU NRU SPU - SelPU Voti 6.550.35324,65% 2.498.2629,40% 2.273.7888,56% Seggi 122 / 450 46 / 450 35 / 450 Distribuzione del voto per collegio 1994 2002 Le elezioni parlamentari in Ucraina del 1998 si tennero il 29 marzo. I 450 deputati furono eletti mediante un sistema misto: la metà dei seggi fu assegnata mediante sistema proporzionale, fra le liste che avessero superato il 4% dei voti...

Questa voce o sezione sull'argomento pallacanestro non cita le fonti necessarie o quelle presenti sono insufficienti. Puoi migliorare questa voce aggiungendo citazioni da fonti attendibili secondo le linee guida sull'uso delle fonti. Segui i suggerimenti del progetto di riferimento. FIBA EuroBasket 2025 Sport Pallacanestro Zona FIBA FIBA Europe Edizione 42ª Paese ospitante Lettonia Cipro Finlandia Polonia Periodo 27 agosto-14 settembre 2025 Squadre 24 (da 49 f...

莎拉·阿什頓-西里洛2023年8月,阿什頓-西里洛穿著軍服出生 (1977-07-09) 1977年7月9日(46歲) 美國佛羅里達州国籍 美國别名莎拉·阿什頓(Sarah Ashton)莎拉·西里洛(Sarah Cirillo)金髮女郎(Blonde)职业記者、活動家、政治活動家和候選人、軍醫活跃时期2020年—雇主內華達州共和黨候選人(2020年)《Political.tips》(2020年—)《LGBTQ國度》(2022年3月—2022年10月)烏克蘭媒�...

Football match2013 DFL-SupercupMatch programme coverEventDFL-Supercup Borussia Dortmund Bayern Munich 4 2 Date27 July 2013VenueSignal Iduna Park, DortmundRefereeJochen Drees (Münster-Sarmsheim)Attendance80,645← 2012 2014 → The 2013 DFL-Supercup was the fourth DFL-Supercup, an annual football match contested by the winners of the previous season's Bundesliga and DFB-Pokal competitions. It featured Bayern Munich, winners of the 2012–13 Bundesliga and the 2012–13 DFB-Pokal, and...

Berkas:BetaThetaPiCrest.jpgLogo Beta Theta Pi Fraternity Beta Theta Pi (ΒΘΠ) adalah sebuah perhimpunan mahasiswa (Inggris: fraternity / persaudaraan) yang dibentuk di Miami University di kota Oxford, Ohio, Amerika Serikat (AS). Nama singkatnya adalah Beta dan merupakan perhimpunan mahasiswa pertama yang dibentuk bukan di pantai timur AS. Saat ini Beta memiliki lebih dari 120 cabang dan anak cabang di berbagai universitas di AS dan Kanada. Lebih dari 175.000 anggota telah diterima di seluru...

This is the talk page for discussing improvements to the Cartoon Network template. Put new text under old text. Click here to start a new topic. New to Wikipedia? Welcome! Learn to edit; get help. Assume good faith Be polite and avoid personal attacks Be welcoming to newcomers Seek dispute resolution if needed Archives: 1 This template does not require a rating on Wikipedia's content assessment scale.It is of interest to the following WikiProjects:Cartoon Network Cartoon Network portalThis t...

Mutiu AdepojuNazionalità Nigeria Altezza180 cm Peso76 kg Calcio RuoloCentrocampista CarrieraGiovanili 1986-1987 Femo Scorpions Squadre di club1 1988 Shooting Stars? (?)1989 Julius Berger? (?)1989-1992 Castilla65 (27)1992-1996 Racing Santander123 (24)1996-2000 Real Sociedad89 (8)2000-2001 Al-Ittihād? (?)2001-2002 Salamanca14 (0)2002-2003 Samsunspor8 (0)2003-2004 AEL Limassol5 (1)2004-2005 Eldense? (?)2005-2006 Cobeña? (?) Nazi...

Temple on the Athenian Acropolis, Greece Temple of Athena redirects here. For other uses, see Parthenon (disambiguation) and Temple of Athena (disambiguation). Not to be confused with Pantheon, Rome. ParthenonΠαρθενώναςThe Parthenon in 1978General informationTypeTempleArchitectural styleClassicalLocationAthens, GreeceCoordinates37°58′17″N 23°43′36″E / 37.9715°N 23.7266°E / 37.9715; 23.7266Construction started447 BC[1][2]Comple...

Range of physical processes For other uses, see Scattering (disambiguation). ScatteringFeynman diagram of scattering between two electrons by emission of a virtual photon Bragg diffraction Brillouin Compton Dynamic light Kikuchi lines Light scattering by particles Mie Neutron Powder diffraction Raman Rayleigh Rutherford Small-angle Tyndall Thomson Wolf effect X-ray crystallography vte Wine glass in LCD projectors light beam makes the beam scatter. In physics, scattering is a wide range of phy...

Pour l’article homonyme, voir Thomas Dobrée (1810-1895). Thomas DobréeFonctionConseiller municipal de Nantes1813-1830BiographieNaissance 6 avril 1781NantesDécès 15 décembre 1828 (à 47 ans)NantesSépulture Cimetière MiséricordeSurnom Thomas I DobréeNationalité françaiseActivités Armateur, industriel, négociantFamille Famille Dobrée (d)Père Pierre-Frédéric Dobrée (d)Conjoint Frédérique Möller (d)Enfant Thomas DobréeParentèle Bonamy DobréeAutres informationsMembre...