Rational sieve
|
Read other articles:

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Oktober 2022. Gramatur adalah istilah untuk menunjuk ukuran berat kertas yang beredar di pasaran. Satuan yang dipergunakan untuk menghitung berat kertas adalah gram per square meter (gsm) atau (g/m2). Terdapat beberapa kelompok gramatur kertas, yakni 70-80 gsm, 100-...

Singapore Telecommunications LimitedKantor pusat Singtel Di SingapuraJenisPublikIndustriTelekomunikasiDidirikanSingapura (1879)KantorpusatSingapuraTokohkunciSimon Israel, (Ketua) Chua Sock Koong, (CEO) Jeann Low, CFOProdukJasa telepon genggam Internet Jasa jaringan permanenPendapatan$15.34 juta SGD (2022)Karyawan>25,000Situs webSingtel Singapore Telecommunications Limited (SGX: T48) (disingkat Singtel) adalah perusahaan telekomunikasi terbesar di Singapura. Bila pelanggan dari anak perusah...

English charter of 1217 The Charter of the Forest, 1225 reissue, held by the British Library The Charter of the Forest of 1217 (Latin: Carta de Foresta or Charta Forestæ) is a charter that re-established for free men rights of access to the royal forest that had been eroded by King William the Conqueror and his heirs. Many of its provisions were in force for centuries afterwards.[1] It was originally sealed in England by the young King Henry III, acting under the regency of William M...

Nigerian CubeSat Nigeria EduSat-1Nigeria EduSat-1 at deploymentNamesBird NNMission typeTechnology demonstrationEarth observationOperatorFederal University of Technology AkureCOSPAR ID1998-067MY SATCAT no.42824Mission duration24 months (planned)22 months, 5 days (achieved) Spacecraft propertiesSpacecraft type1U CubeSatManufacturerFederal University of Technology AkureLaunch mass1 kgDimensions10 × 10 × 10 cmPowerwatts Start of missionLaunch date3 June 2017, 21:07:38 UTC[1]Ro...

فلوريان ألبرت (بالمجرية: Albert Flórián) معلومات شخصية الميلاد 15 سبتمبر 1941(1941-09-15) الوفاة 31 أكتوبر 2011 (عن عمر ناهز 70 عاماً)بودابست سبب الوفاة مضاعفات ناجمة عن جراحة الطول 1.81 م (5 قدم 11 1⁄2 بوصة) مركز اللعب مهاجم الجنسية المجر أبناء فلوريان ألبرت جونيور&...

Public debate in Australia over British colonialism Not to be confused with the Canadian history wars. This article is part of a series on theHistory of Australia Timeline and periods Prehistory European exploration (sea) European exploration (land) 1788–1850 1851–1900 1901–1945 1945–present Topics Abortion Agriculture Antisemitism Banking Capital punishment Civil rights Cinema Constitution Diplomacy Economics Federation Immigration Labour LGBT Military Monarchy Sports Telecommunicati...

Questa voce o sezione sull'argomento Giappone è stata parzialmente tradotta dalla lingua inglese. Puoi contribuire terminando la traduzione o usando altre fonti. Non usare programmi di traduzione automatica. Usa {{Tradotto da}} quando hai terminato. Se nella pagina di modifica trovi del testo nascosto, controlla che sia aggiornato servendoti dei collegamenti «in altre lingue» in fondo alla colonna di sinistra. Segui i suggerimenti del progetto di riferimento....

Dalam nama Tionghoa ini, nama keluarganya adalah Chen. Annie ChenLahir28 April 1989 (umur 34)Taichung, TaiwanPekerjaanPemeran, peraga busanaTahun aktif2007-kini Annie Chen Hanzi tradisional: 陳庭妮 Hanzi sederhana: 陈庭妮 Alih aksara Mandarin - Hanyu Pinyin: Chén Tíngnī Karier musikNama lainChen Ting-ni Annie Chen (Hanzi: 陳庭妮; lahir 28 April 1989)[1] adalah seorang pemeran dan peraga busana asal Taiwan. Ia adalah orang pertama yang memenangkan kontes perag...

البرانسمعلومات عامةالبلد إسبانيا فرنسا أندورا المكان القائمة ... أقطانية الجديدة — قسطانية — منطقة إقليم الباسك — أَرَغـُون — منطقة نبرة — كتالونيا جزء من حزام ألبي الجغرافياالإحداثيات 42°40′N 1°00′E / 42.67°N 1°E / 42.67; 1 قمة جبل أنيتو الارتفاع 3٬404 متر ا�...
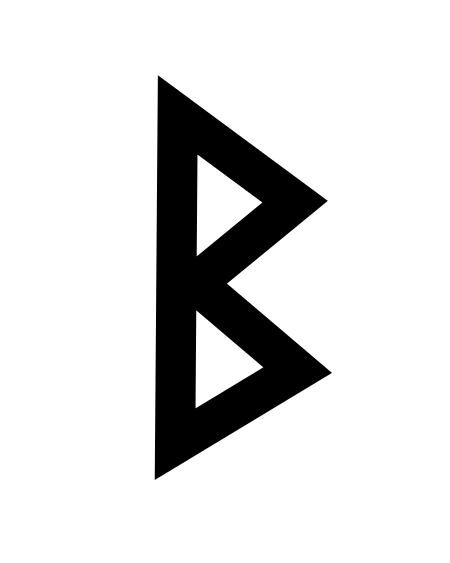
此條目介紹的是拉丁字母中的第2个字母。关于其他用法,请见「B (消歧义)」。 提示:此条目页的主题不是希腊字母Β、西里尔字母В、Б、Ъ、Ь或德语字母ẞ、ß。 BB b(见下)用法書寫系統拉丁字母英文字母ISO基本拉丁字母(英语:ISO basic Latin alphabet)类型全音素文字相关所属語言拉丁语读音方法 [b][p][ɓ](适应变体)Unicode编码U+0042, U+0062字母顺位2数值 2歷史發...

Citizens or residents of Iraq This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Iraqis – news · newspapers · books · scholar · JSTOR (October 2023) (Learn how and when to remove this message) IraqisالعراقيونMap of the Iraqi diaspora in the world including descendantsTotal population50+ million worldwi...

Questa voce o sezione sull'argomento scrittori statunitensi non cita le fonti necessarie o quelle presenti sono insufficienti. Puoi migliorare questa voce aggiungendo citazioni da fonti attendibili secondo le linee guida sull'uso delle fonti. Segui i suggerimenti del progetto di riferimento. Morgan Robertson Morgan Robertson (Oswego, 30 settembre 1861 – Atlantic City, 24 marzo 1915) è stato uno scrittore e inventore statunitense. Indice 1 Biografia 2 Morte 3 Note 4 Voci correlate 5 A...

Unincorporated community in Florida, United StatesAlligator PointUnincorporated communityThe Marina at Alligator Point during the 1960sAlligator PointCoordinates: 29°54′18″N 84°25′01″W / 29.905°N 84.417°W / 29.905; -84.417CountryUnited StatesStateFloridaCountyFranklinGovernment • BodyFranklin County, Florida • District 2 CommissionerBert B. Bolt IIPopulation (2012) • Total447 Rough Estimate; Not Conducted by th...

This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Tamil Nadu Civil Service – news · newspapers · books · scholar · JSTOR (September 2019) (Learn how and when to remove this message) Tamil Nadu Administrative Serviceதமிழ்நாடு நிர்வாக சேவைTamil Nadu logoAgency overviewForm...

UK charitable organisation This article contains content that is written like an advertisement. Please help improve it by removing promotional content and inappropriate external links, and by adding encyclopedic content written from a neutral point of view. (February 2017) (Learn how and when to remove this message) Christians Against PovertyFounded1996FounderJohn KirkbyTypeChristian CharityPurposeDebt counselling[1]Origins United KingdomWebsitecapuk.org Christians Against Povert...

1968 studio album by Frankie LaineTo Each His OwnStudio album by Frankie LaineReleased1968LabelABCProducerBob ThieleFrankie Laine chronology I Wanted Someone to Love(1967) To Each His Own(1968) Take Me Back to Laine Country(1968) Professional ratingsReview scoresSourceRatingAllMusic[1]BillboardPositive[2] To Each His Own is a studio album by Frankie Laine released in 1968 on ABC Records.[3] Track listing Side oneNo.TitleWriter(s)Length1.To Each His OwnJ. Living...

Surgical transplant procedure Heart transplantationland illustrating the placement of a donor heart in an orthotopic procedure. Notice how the back of the patient's left atrium and great vessels are left in place.SpecialtycardiologyICD-9-CM37.51MeSHD016027MedlinePlus003003[edit on Wikidata] A heart transplant, or a cardiac transplant, is a surgical transplant procedure performed on patients with end-stage heart failure or severe coronary artery disease when other medical or surgical treat...

French fairy-tale This article is about the fairy-tale. For other uses, see Beauty and the Beast (disambiguation). La Belle et la Bête(Beauty and the Beast)Beauty releases the prince from his beastly curse. Artwork from Europa's Fairy Book, by John Batten.Folk taleNameLa Belle et la Bête(Beauty and the Beast)Also known asLa Bèlla e la Bèstia,Die Schöne und das BiestAarne–Thompson groupingATU 425C (Beauty and the Beast)RegionFrancePublished inLa jeune américaine, et les contes marins (...

Category 5 Atlantic hurricane in 2007 This article is about the Atlantic hurricane of 2007. For other storms of the same name, see List of storms named Dean. Hurricane Dean Dean at peak intensity making landfall on August 21Meteorological historyFormedAugust 13, 2007Remnant lowAugust 23, 2007DissipatedAugust 27, 2007Category 5 major hurricane1-minute sustained (SSHWS/NWS)Highest winds175 mph (280 km/h)Lowest pressure905 mbar (hPa); 26.72 inHgOverall effectsFatalities4...

Concorso a squadre femminileHelsinki 1952 Informazioni generaliLuogoTöölö Sports Hall, Helsinki Periodo22 - 23 luglio 1952 Partecipanti16 squadre nazionali Podio Oro Unione Sovietica Argento Ungheria Bronzo Cecoslovacchia Edizione precedente e successiva Londra 1948 Melbourne 1956 Voce principale: Ginnastica ai Giochi della XV Olimpiade. Ginnastica a Helsinki 1952 Concorso a squadre uomini donne Attrezzi a squadre donne Concorso individuale uomini don...














