Independent and identically distributed random variables
|
Read other articles:

Opera by Wolfgang Amadeus Mozart For other uses, see The Marriage of Figaro (disambiguation). The Marriage of FigaroOpera by W. A. MozartEarly 19th-century engraving depicting Count Almaviva and Susanna in act 3Native titleLe nozze di FigaroLibrettistLorenzo Da PonteLanguageItalianBased onLa folle journée, ou le Mariage de Figaroby Pierre BeaumarchaisPremiere1 May 1786 (1786-05-01)Burgtheater, Vienna The Marriage of Figaro (Italian: Le nozze di Figaro, pronounced [le ˈnɔ...

Sporting event delegationSeychelles at the2017 World Aquatics ChampionshipsFlag of SeychellesFINA codeSEYNational federationSeychelles Swimming Associationin Budapest, HungaryCompetitors4 in 1 sportMedals Gold 0 Silver 0 Bronze 0 Total 0 World Aquatics Championships appearances197319751978198219861991199419982001200320052007200920112013201520172019202220232024 Seychelles competed at the 2017 World Aquatics Championships in Budapest, Hungary from 14 July to 30 July. Swimming Main article: Swi...

Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: Ladakh – berita · surat kabar · buku · cendekiawan · JSTOR Ladakh Ladakh, yang terletak di bagian timur pemerintahan Jammu dan Kashmir adalah salah satu daerah tempat tinggal tertinggi di muka bumi. Suat...

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (ديسمبر 2018) لمعانٍ أخرى، طالع مسجد النور (توضيح). مسجد النور معلومات عامة القرية أو المدينة الموصل الدولة ا�...

Para otros usos de este término, véase Lérida (desambiguación). Lérida Lleida Municipio y ciudad de EspañaBanderaEscudo De izquierda a derecha y de arriba abajo: panorámica de la ciudad desde el río Segre, la Estación de Lérida Pirineos, Palacio de la Paeria, Iglesia de San Juan, la Lonja de Lérida, Puente de Príncipe de Viana, el conjunto de la Seo Vieja y el Aeropuerto de Lérida-Alguaire. LéridaUbicación de Lérida en España LéridaUbicación de Lérida en la provincia de L...

Men's national volleyball team representing Brazil BrazilNickname(s)CanarinhosGalacticBest of All TimesAssociationCBVConfederationCSVHead coachBernardo Rezende[1]FIVB ranking5 (as of 2 December 2023)Uniforms Home Away Third Summer OlympicsAppearances14 (First in 1964)Best result (1992, 2004, 2016)World ChampionshipAppearances17 (First in 1956)Best result (2002, 2006, 2010)World CupAppearances12 (First in 1969)Best result (2003, 2007, 2019)www.cbv.com.br (in Portuguese) Honours Event 1...

此條目可参照英語維基百科相應條目来扩充。 (2021年5月6日)若您熟悉来源语言和主题,请协助参考外语维基百科扩充条目。请勿直接提交机械翻译,也不要翻译不可靠、低品质内容。依版权协议,译文需在编辑摘要注明来源,或于讨论页顶部标记{{Translated page}}标签。 约翰斯顿环礁Kalama Atoll 美國本土外小島嶼 Johnston Atoll 旗幟颂歌:《星條旗》The Star-Spangled Banner約翰斯頓環礁�...

穆罕默德·达乌德汗سردار محمد داود خان 阿富汗共和國第1任總統任期1973年7月17日—1978年4月28日前任穆罕默德·查希爾·沙阿(阿富汗國王)继任穆罕默德·塔拉基(阿富汗民主共和國革命委員會主席團主席) 阿富汗王國首相任期1953年9月7日—1963年3月10日君主穆罕默德·查希爾·沙阿 个人资料出生(1909-07-18)1909年7月18日 阿富汗王國喀布尔逝世1978年4月28日(...
2020年夏季奥林匹克运动会波兰代表團波兰国旗IOC編碼POLNOC波蘭奧林匹克委員會網站olimpijski.pl(英文)(波兰文)2020年夏季奥林匹克运动会(東京)2021年7月23日至8月8日(受2019冠状病毒病疫情影响推迟,但仍保留原定名称)運動員206參賽項目24个大项旗手开幕式:帕维尔·科热尼奥夫斯基(游泳)和马娅·沃什乔夫斯卡(自行车)[1]闭幕式:卡罗利娜·纳亚(皮划艇)&#...

Santa LuciaIl sepolcro in piazza S. Lucia alla borgata Stato Italia Regione Sicilia Provincia Siracusa CittàSiracusa Santa Lucia (A Buggata in dialetto siracusano) è il secondo quartiere storico di Siracusa (fino al 2018 dotato di una propria circoscrizione) storicamente e popolarmente definito anche Borgata, bagnata a sud-est dalle acque del porto piccolo o porto marmoreo in cui in passato era attivo il collegamento marittimo Borgata-Ortigia e viceversa con il buzzetto, una imbar...
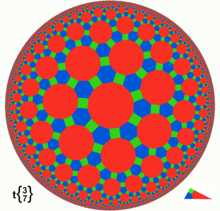
Model of hyperbolic geometry Poincaré disk with hyperbolic parallel lines Poincaré disk model of the truncated triheptagonal tiling. In geometry, the Poincaré disk model, also called the conformal disk model, is a model of 2-dimensional hyperbolic geometry in which all points are inside the unit disk, and straight lines are either circular arcs contained within the disk that are orthogonal to the unit circle or diameters of the unit circle. The group of orientation preserving isometries of...

City in South Dakota, United StatesFort PierreCityMain and Deadwood streets in Fort Pierre, South DakotaMotto: Where The West BeginsLocation in Stanley County and the state of South DakotaCoordinates: 44°22′04″N 100°22′59″W / 44.36778°N 100.38306°W / 44.36778; -100.38306CountryUnited StatesStateSouth DakotaCountyStanleyFounded1867IncorporatedJune 2, 1890[1]Government • MayorGloria HansonArea[2] • Total3.17 s...

Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: Nida Ria – berita · surat kabar · buku · cendekiawan · JSTOR Nida Ria GroupGenreQasidahTahun aktif1980 - sekarang Nida Ria Group adalah sebuah group Qasidah Modern yang didirikan oleh anak pendiri Nasida...

British linguist and writer David CrystalOBE FBA FLSW FCILCrystal in 2017Born (1941-07-06) 6 July 1941 (age 82)Lisburn, Northern IrelandNationalityBritishAlma materUniversity College LondonSpouseHilary CrystalChildrenBen CrystalScientific careerFieldsLinguistics Websitedavidcrystal.com David Crystal, OBE, FBA, FLSW, FCIL (born 6 July 1941) is a British linguist who works on the linguistics of the English language. Crystal studied English at Univer...

Sports event 2013 Canadian Olympic Curling TrialsHost cityWinnipeg, ManitobaArenaMTS CentreDatesDecember 1–8Men's winner Brad JacobsCurling clubSoo CA, Sault Ste. MarieSkipBrad JacobsThirdRyan FrySecondE. J. HarndenLeadRyan HarndenAlternateCaleb FlaxeyCoachTom CoultermanFinalist John MorrisWomen's winner Jennifer JonesCurling clubSt. Vital CC, WinnipegSkipJennifer JonesThirdKaitlyn LawesSecondJill OfficerLeadDawn McEwenAlternateKirsten WallCoachJanet ArnottFinalist Sherry Middaugh« 20...

SananDesaPeta lokasi Desa SananNegara IndonesiaProvinsiJawa TengahKabupatenWonogiriKecamatanGirimartoKode pos57683Kode Kemendagri33.12.22.2013 Luas5,71 km²Jumlah penduduk3.255 jiwa (2012)Kepadatan570,05 jiwa per km² (2012) Sanan adalah desa di kecamatan Girimarto, Kabupaten Wonogiri, provinsi Jawa Tengah, Indonesia. Pembagian wilayah Desa Sanan terdiri dari 8 dusun:[1] Brenggolo Gandon Mongsari Sanan Semagarledok Sempon Sinlonggong Tampakan Pendidikan Lembaga pendidikan formal ...

Pemilihan umum Wali Kota Pematangsiantar 2015201020209 Desember 2015Kandidat Calon Hulman Sitorus Wesly Silalahi Teddy Robinson Siahaan Partai Demokrat PDI-P NasDem Pendamping Hefriansyah Sailanto Zainal Purba Suara rakyat 59.445 25.899 19.282 Persentase 54,49% 23,74% 17,67% Calon Sujito Partai Independen Pendamping Djumadi Suara rakyat 19.282 Persentase 17,67% Peta persebaran suara Peta lokasi Pematangsiantar Wali Kota petahanaHulman Sitorus Demokrat Wali Kota terpil...

L'emisfero occidentale geografico della Terra indicato in giallo. Emisfero occidentale in riferimento alle Americhe (o al Nuovo Mondo). L'emisfero occidentale è un termine geografico utilizzato per indicare la metà della Terra che si trova ad ovest del meridiano di Greenwich (che attraversa Greenwich a Londra, nel Regno Unito), l'altra metà è l'emisfero orientale.[1] Inoltre viene utilizzato in modo specifico in riferimento alle Americhe (o il Nuovo Mondo) e le acque adiacenti...

Italian middle-distance runner Ala ZoghlamiAla Zoghlami at the 2020 Olympics.Personal informationNational team ItalyBorn (1994-06-19) 19 June 1994 (age 30)Tunis, TunisiaHeight1.80 m (5 ft 11 in)Weight57 kg (126 lb)SportSportAthleticsEvent(s)Middle-distance running3000 metres steeplechaseClubCus PalermoCoached byGaspare PolizziAchievements and titlesPersonal best 3000 m steeplechase 8:24.98 (2021) Ala Zoghlami (Arabic: علاء الزغلامي; born 19 June ...

Cet article est une ébauche concernant le catholicisme. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Diocèse de Carpentras(la) Dioecesis Carpentoractensis Cathédrale Saint-Siffrein de Carpentras Informations générales Pays France Église catholique Rite liturgique romain Type de juridiction diocèse Suppression 1790 / 1801 Province ecclésiastique Arles puis Avignon Siège Carpentras Diocèses suffragant...


























