Rangkap
|
Read other articles:

Jurina MatsuiJurina Matsui pada tahun 2014Informasi latar belakangNama lahirJurina MatsuiNama lainJurina (じゅりなcode: ja is deprecated )Lahir8 Maret 1997 (umur 27)AsalPrefektur Aichi, Jepang.GenrePopPekerjaan Penyanyi Pemeran Tahun aktif2008 - 2021Artis terkait SKE48 AKB48 Jurina Matsui (松井 珠理奈code: ja is deprecated , Matsui Jurina, lahir 8 Maret 1997) adalah idola Jepang anggota idola Tim S SKE48. Sebagai anggota SKE48, ia secara berturut-turut diikutsertakan sebag...

Artikel ini adalah bagian dari seri:Permainan video Pelantar Dingdong Konsol permainan Konsol video rumah Permainan elektronik Konsol genggam Permainan ponsel Permainan daring Permainan PC Linux Mac Genre Laga Berhantam Bertarung Arung pelantar Bertahan hidup Siluman Bertahan hidup horor Petualangan Bermain peran Bermain peran laga Bermain peran taktik Simulasi Konstruksi dan manajemen Simulasi kehidupan Olahraga Kendaraan Strategi Bertarung daring banyak pemain Strategi waktu nyata Taktik wa...

Syd Barker redirects here. For his son, see Syd Barker Jr. Australian rules footballer Australian rules footballer Syd Barker Personal informationFull name Sydney Quinton Barker Sr.Date of birth (1887-11-26)26 November 1887Place of birth Collingwood, VictoriaDate of death 23 March 1930(1930-03-23) (aged 42)Place of death Abbotsford, VictoriaHeight 183 cm (6 ft 0 in)Weight 95 kg (209 lb)Position(s) RuckmanPlaying career1Years Club Games (Goals)1906–1907 Ess...

2013 video gameMIND≒0North American cover art featuring Kei Takanashi, the game's protagonistDeveloper(s)Acquire, ZeroDivPublisher(s)JP: GungHo Online EntertainmentNA/EU: Aksys GamesComposer(s)Zizz StudioPlatform(s)PlayStation Vita, Microsoft WindowsReleasePlayStation VitaJP: August 1, 2013NA: May 27, 2014[1]EU: May 28, 2014[1]Microsoft WindowsWW: March 8, 2016Genre(s)Role-playing, dungeon crawlerMode(s)Single-player Mind Zero, stylized MIND≒0,[2] (Japanese: マイ...

Miriam Giovanelli ai Premi Goya 2017 Miriam Giovanelli (Roma, 28 aprile 1989) è un'attrice e modella italiana naturalizzata spagnola. Indice 1 Biografia 2 Filmografia 2.1 Cinema 2.2 Televisione 3 Doppiatrici italiane 4 Altri progetti 5 Collegamenti esterni Biografia Nasce a Roma da padre italiano e madre spagnola; dall'età di 10 anni vive a Madrid. La sua carriera inizia come modella, lavorando per numerose campagne pubblicitarie e spot televisivi. Come attrice inizia a interpretare alcuni ...

Former museum in New York City KGB Espionage MuseumLocation within ManhattanFormer nameKGB Spy MuseumEstablishedJanuary 17, 2019 (2019-01-17)Location245 West 14th St., New York, New York, United StatesCoordinates40°44′14″N 73°59′51″W / 40.737358°N 73.997451°W / 40.737358; -73.997451TypeHistory MuseumCollection sizeOver 3,900 ObjectsWebsitehttps://kgbespionagemuseum.org/ The KGB Espionage Museum was a museum dedicated to the unbiased presenta...

Scottish Episcopal ChurchLambang Gereja Episkopal Skotlandia, menggambarkan dua mitra dan crosierPenggolonganProtestan (dengan beragam identitas teologi dan doktrin, termasuk Anglo-Katolik, Liberal dan Evangelikal)OrientasiAnglikanKitab suciAlkitabTeologiDoktrin AnglikanBentukpemerintahanEpiskopalPrimusMark StrangePerhimpunanDewan Gereja-Gereja Sedunia,Komuni Anglikan,Komuni Porvoo,Action of Churches Together in ScotlandWilayahSkotlandiaKantor pusatEdinburgh, SkotlandiaDidirikanConcordat of L...

Minor Upanishad of Hinduism Annapurna UpanishadThe Upanishad discusses meditation and spiritual liberationDevanagariअन्नपूर्णाIASTAnnapūrṇāTitle meansAbundance of foodTypeSamanya (general)[1]Linked VedaAtharvaveda[1]Chapters5[2]Verses337[2]PhilosophyVedanta[1] The Annapurna Upanishad (Sanskrit: अन्नपूर्णा उपनिषद्, IAST: Annapūrṇā Upaniṣad) is a Sanskrit text and one of the minor Upanishads ...

Polish politician and activist This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Julian Marchlewski – news · newspapers · books · scholar · JSTOR (December 2010) (Learn how and when to remove this message) Julian MarchlewskiBorn(1867-05-17)17 May 1867Włocławek, Congress Poland, Russian EmpireDied22 March 192...

National Research Nuclear University MEPhI (Moscow Engineering Physics Institute)Former namesMoscow Mechanical Institute of Munitions (1942–1953), Moscow Engineering Physics Institute (1953–2009)TypePublicEstablished1942RectorMikhail Nikolaevich StrikhanovLocationMoscow, Russia55°38′59″N 37°39′52″E / 55.64972°N 37.66444°E / 55.64972; 37.66444Websitehttp://eng.mephi.ruBuildingBuilding detailsMain corpus building University rankingsRegional – OverallQS ...

Six gay pornographic actors during the 2010 Blatino Erotica Awards This is a list of notable men who have appeared in gay pornographic films. Pornography has become more mainstream and as of 2009 was a $13 billion industry in the United States; globally consumers spent more than US$3000 on porn every second of every day, in 2009.[1] The gay market is estimated to be five to ten percent of the overall adult market.[2] Gay pornographic films trace their origins to the Athl...

此條目需要补充更多来源。 (2021年7月4日)请协助補充多方面可靠来源以改善这篇条目,无法查证的内容可能會因為异议提出而被移除。致使用者:请搜索一下条目的标题(来源搜索:美国众议院 — 网页、新闻、书籍、学术、图像),以检查网络上是否存在该主题的更多可靠来源(判定指引)。 美國眾議院 United States House of Representatives第118届美国国会众议院徽章 众议院旗...

American novelist James Branch CabellJames Branch Cabell photographed by Carl Van Vechten, 1935Born(1879-04-14)April 14, 1879Richmond, Virginia, U.S.DiedMay 5, 1958(1958-05-05) (aged 79)Richmond, Virginia, U.S.Resting placeHollywood Cemetery, Richmond, Virginia, U.S.OccupationAuthorAlma materCollege of William and MaryGenreFantasy fiction James Branch Cabell (/ˈkæbəl/; April 14, 1879 – May 5, 1958) was an American author of fantasy fiction and belles-lettres. Cabell was w...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Februari 2023. Sony Alpha a99 adalah satu dari beberapa kamera DSLR [1] yang diproduksi oleh Sony. Sony Alpha a99 adalah kamera full-frame pertama yang ada di dunia yang mengusung teknologi Translucent Mirror.[2] yakni sebuah teknologi cermin pantul ...

French national day (14 July) This article is about the French national holiday. For other uses, see Bastille Day (disambiguation). Fête nationale française redirects here. For other French language fêtes nationales, see Fête nationale. Bastille DayThe Patrouille de France with nine Alpha Jets over the Champs-Élysées in Paris in 2017, during the Bastille Day military paradeAlso calledFrench National Day(Fête nationale)The Fourteenth of July(Quatorze juillet)Observed byFranceTypeNa...

Pour les articles homonymes, voir Marilyn Manson et Manson. Marilyn Manson Marilyn Manson sur scène à Moscou, en Russie, en 2012.Informations générales Pays d'origine États-Unis Genre musical Metal industriel, rock industriel, metal alternatif, glam rock, shock rock Années actives Depuis 1989 Labels Nothing Records, Interscope Records (1989–2011), Cooking Vinyl (2012-2015), Loma Vista Recordings, Caroline international (2017-2021) Site officiel www.marilynmanson.com Composition du gr...

Auto racing factory team by Škoda This article needs to be updated. Please help update this article to reflect recent events or newly available information. (October 2018) Škoda MotorsportFull nameŠkoda MotorsportBase Plazy, Czech RepublicTeam principal(s)Michal HrabánekChassis Škoda Fabia RS Rally2 ŠKODA Motorsport is the sports factory team of the car manufacturer Škoda Auto, currently focusing on the development and construction of racing cars. The latest model from Škoda Motorspor...

Program allowing for online play of video games XLink KaiDeveloper(s)Team XLinkInitial releaseMarch 13, 2003; 21 years ago (2003-03-13)Stable release7.4.45 / 02 November 2023 Written inC++Operating systemWindows, Linux, macOS, Raspberry Pi OSAvailable in11 languagesList of languagesChinese (Simplified), Chinese (Traditional), Dutch, English, French, German, Japanese, Korean, Polish, Russian, SpanishLicenseProprietaryWebsiteOfficial website XLink Kai is a program developed by...
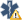
تقرح حول ظفري التهاب حول الظفر معلومات عامة الاختصاص طب الجلد من أنواع أمراض الأظافر، ومرض الإدارة حالات مشابهة داحس تعديل مصدري - تعديل التهاب حول الظفر[1][2][3][4] أو التهاب حتار الظفر[5] أو داحس[6] (بالإنجليزية: Paronychia) هو التهاب يصيب ...

German physicist Jürgen EhlersAt the award ceremony for the Charles University Medal in Potsdam, September 2007Born(1929-12-29)29 December 1929Hamburg, GermanyDied20 May 2008(2008-05-20) (aged 78)Potsdam, Brandenburg, GermanyNationalityGermanAlma materUniversity of HamburgKnown forGeneral relativityMathematical physicsAwardsMax Planck Medal (2002)Scientific careerFieldsPhysicsInstitutionsUniversity of HamburgMax Planck Institute for AstrophysicsMax Planck Institute for Gravit...



