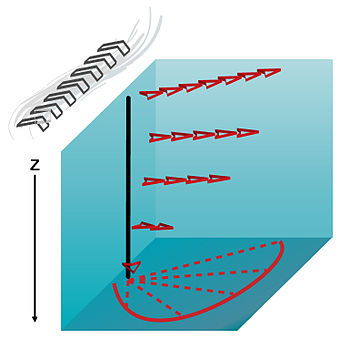
Ekman layer
|
Read other articles:

At-TajuriLingkunganNegara Arab SaudiProvinsiProvinsi MadinahKotaMadinahZona waktuUTC+3 (EAT) • Musim panas (DST)UTC+3 (EAT) At-Tajuri adalah sebuah lingkungan di kota suci Madinah di Provinsi Madinah, tepatnya di sebelah barat Arab Saudi.[1] Referensi ^ National Geospatial-Intelligence Agency. GeoNames database entry. (search Diarsipkan 2017-03-18 di Wayback Machine.) Accessed 12 May 2011. lbsLingkungan sekitar Masjid Nabawi, Madinah, Arab Saudi • Al-Jumu'ah • -Mu...

'Gagak api terbang' (shen huo fei ya), bom roket bersayap aerodinamis dari Huolongjing Huolongjing (Hanzi tradisional: 火龍經; Hanzi sederhana: 火龙经; Pinyin: Huǒ Lóng Jīng; Wade-Giles: Huo Lung Ching: Huo Lung Ching ; diterjemahkan dalam bahasa Inggris sebagai Fire Drake Manual atau Fire Dragon Manual), juga dikenal sebagai Huoqitu (gambaran Senjata Api), adalah risalah militer abad 14 yang disusun dan diedit oleh Jiao Yu dan Liu Bowen dari dinasti Ming awal (1368–168...

Chronologie de la France ◄◄ 1585 1586 1587 1588 1589 1590 1591 1592 1593 ►► Chronologies 1er août : Jacques Clément assassine le roi à coup de couteau.Données clés 1586 1587 1588 1589 1590 1591 1592Décennies :1550 1560 1570 1580 1590 1600 1610Siècles :XIVe XVe XVIe XVIIe XVIIIeMillénaires :-Ier Ier IIe IIIe Chronologies thématiques Art Architecture, Arts plastiques (Dessin, Gravure, Peinture et Sculptur...

2022 single by GloRilla BlessedSingle by GloRillafrom the EP Anyways, Life's Great ReleasedAugust 31, 2022Length3:36LabelCMGInterscopeSongwriter(s)Gloria WoodsMario MimsAntonio Anderson, Jr.Producer(s)Macaroni ToniGloRilla singles chronology Just Say That (Remix) (2022) Blessed (2022) F.N.F. (Let's Go) (Remix) (2022) Yo Gotti singles chronology Big League(2022) Blessed(2022) Brown Liquor(2022) Music videoBlessed on YouTube Blessed is a song by American rapper GloRilla, released on Aug...

Artikel ini membutuhkan rujukan tambahan agar kualitasnya dapat dipastikan. Mohon bantu kami mengembangkan artikel ini dengan cara menambahkan rujukan ke sumber tepercaya. Pernyataan tak bersumber bisa saja dipertentangkan dan dihapus.Cari sumber: Nyoman Rudana – berita · surat kabar · buku · cendekiawan · JSTOR Nyoman Rudana - Juni 2008 Nyoman Rudana (lahir 17 September 1948) adalah seorang kolektor seni, wirausahawan, dan anggota Dewan Perwakilan Dae...

Pour les articles homonymes, voir George Washington (homonymie) et Lafayette. Georges Washington de La Fayette Georges Washington de La Fayette à onze ans, peint sur Le serment de La Fayette à la fête de la Fédération (14 juillet 1790).(musée de la Révolution française) Fonctions Député 1815, 1827-1848 Groupe politique Libéral (1815)Aide-toi, le ciel t'aidera (1827-1830)Gauche (1830)Républicain (1831-1848) Biographie Date de naissance 24 décembre 1779 à Paris, Royaume de France...

Miguel Layún Layún con la maglia del Messico nel 2017. Nazionalità Messico Altezza 178 cm Peso 69 kg Calcio Ruolo Difensore, centrocampista Termine carriera 2024 CarrieraGiovanili 2003-2006 VeracruzSquadre di club1 2006-2009 Veracruz58 (1)2009-2010 Atalanta2 (0)2010-2015 América111 (13)[1]2015 Watford20 (1)2015-2018 Porto50 (6)2018→ Siviglia16 (2)2018-2019 Villarreal8 (0)2019-2021 Monterrey73 (3)2021-2024 América68 (1)Na...

His Excellency赫瓦贾·纳齐姆丁爵士খাজা নাজিমুদ্দীন خواجہ ناظِمُ الدّینCIE, KCIE摄于1948年第2任巴基斯坦總理任期1951年10月17日—1953年4月17日君主佐治六世伊莉沙白二世总督古拉姆·穆罕默德前任利雅卡特·阿里·汗继任Mohammad Ali Bogra(英语:Mohammad Ali Bogra)第2任巴基斯坦總督(英语:Governor-General of Pakistan)任期1948年9月14日—1951年10月17日君�...

此條目需要补充更多来源。 (2021年7月4日)请协助補充多方面可靠来源以改善这篇条目,无法查证的内容可能會因為异议提出而被移除。致使用者:请搜索一下条目的标题(来源搜索:美国众议院 — 网页、新闻、书籍、学术、图像),以检查网络上是否存在该主题的更多可靠来源(判定指引)。 美國眾議院 United States House of Representatives第118届美国国会众议院徽章 众议院旗...

此條目需要补充更多来源。 (2021年7月4日)请协助補充多方面可靠来源以改善这篇条目,无法查证的内容可能會因為异议提出而被移除。致使用者:请搜索一下条目的标题(来源搜索:美国众议院 — 网页、新闻、书籍、学术、图像),以检查网络上是否存在该主题的更多可靠来源(判定指引)。 美國眾議院 United States House of Representatives第118届美国国会众议院徽章 众议院旗...

カナダの政治家サー・ウィルフリッド・ローリエSir Wilfrid Laurier 生年月日 1841年11月20日出生地 カナダ、ケベック州サン・ラン没年月日 (1919-02-17) 1919年2月17日(77歳没)所属政党 自由党サイン 第8代首相在任期間 1896年7月11日 - 1911年10月5日テンプレートを表示 サー・ウィルフリッド・ローリエ(Sir Wilfrid Laurier フランス語: [wilfʁid loʁje] 英語: [ˈlɒrieɪ]、1841年11月20�...

American baseball player (born 1990) Baseball player Zack WheelerWheeler with the Philadelphia Phillies in 2022Philadelphia Phillies – No. 45Starting pitcherBorn: (1990-05-30) May 30, 1990 (age 34)Smyrna, Georgia, U.S.Bats: LeftThrows: RightMLB debutJune 18, 2013, for the New York MetsMLB statistics (through June 3, 2024)Win–loss record94–66Earned run average3.38Strikeouts1,492 Teams New York Mets (2013–2014, 2017–2019) Philadelphia Phillies (2020–present) Care...

У этого термина существуют и другие значения, см. Волхов (значения). ГородВолхов Флаг Герб 59°54′02″ с. ш. 32°21′10″ в. д.HGЯO Страна Россия Субъект Федерации Ленинградская область Муниципальный район Волховский Городское поселение город Волхов Глава МО Арутюнян ...

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (مارس 2016) كأس أمريكا الجنوبية 1967معلومات عامةموسم لـ كوبا أمريكا الرياضة كرة القدم البلد الأوروغواي المكان ملعب سنتي...

У Вікіпедії є статті про інші значення цього терміна: Ягода (значення). Чотири типи дійсних ягід; за годинниковою стрілкою справа: виноград, хурма, аґрус, порічки (верх). Кілька типів «ягід» у звичайному розумінні, жодна з яких не є ягодою з ботанічної точки зору; згори дон...

Untuk radikal Italia bernama sama, lihat Gregorio Fontana (radikal). Gregorio FontanaLahir(1735-12-07)7 Desember 1735Nogaredo, ItaliaMeninggal24 Agustus 1803(1803-08-24) (umur 67)Milan, ItaliaKebangsaanItaliaDikenal ataskoordinat kutubKarier ilmiahBidangGeometriMahasiswa ternamaPietro Paoli Gregorio Fontana, bernama lahir Giovanni Battista Lorenzo Fontana (7 Desember 1735 – 24 Agustus 1803) adalah seorang matematikawan dan anggota keagamaan ordo Piaris asal Italia. Ia men...

Article principal : Aviron aux Jeux olympiques d'été de 2016. Deux sans barreur féminin(W2-)aux Jeux olympiquesd'été de 2016 Généralités Sport Aviron Organisateur(s) CIO Éditions 27e Lieu(x) Rio de Janeiro Date du 7 août 2016 au 12 août 2016 Nations 15 Participants 30 Site(s) Lagoa Rodrigo de Freitas Palmarès Tenant du titre Glover / Stanning Vainqueur Glover / Stanning Deuxième Behrent / Scown Troisième Rasmussen / Andersen Navigation Londres 2012 Tokyo 2020 modifier L'ép...

County Jefferson, WisconsinJefferson County courthouseMap of Wisconsin highlighting County JeffersonLokasi di negara bagian WisconsinLokasi negara bagian Wisconsin di Amerika SerikatDidirikan1839Asal namaThomas JeffersonSeatJeffersonKota terbesarWatertownWilayah • Keseluruhan583 sq mi (1.510 km2) • Daratan556 sq mi (1.440 km2) • Perairan26 sq mi (67 km2), 4.5Distrik kongreske-5Zona waktuTengahSitus webwww.jeffers...

فورغ فورگ - قرية - تقسيم إداري البلد إيران[1] المحافظة خراسان الجنوبية المقاطعة مقاطعة درميان الناحية الناحية المركزية القسم الريفي قسم درمیان الريفي خصائص جغرافية إحداثيات 32°50′42″N 59°56′59″E / 32.845°N 59.94972°E / 32.845; 59.94972 الارتفاع 1789 متر[2]...
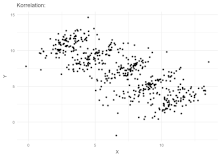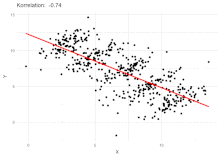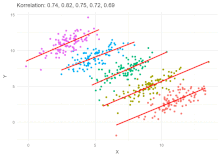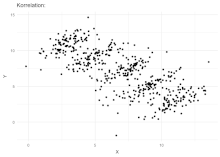
Error in statistical reasoning with groups Simpson's paradox for quantitative data: a positive trend ( , ) appears for two separate groups, whereas a negative trend ( ) appears when the groups are combined. Visualization of Simpson's paradox on data resembling real-world variability indicates that risk of misjudgment of true causal relationship can be hard to spot. Simpson's paradox is a phenomenon in probability and statistics in which a trend appears in several groups of dat...
![{\displaystyle {\begin{aligned}-fv&=-{\frac {1}{\rho _{o}}}{\frac {\partial p}{\partial x}}+K_{m}{\frac {\partial ^{2}u}{\partial z^{2}}},\\[5pt]fu&=-{\frac {1}{\rho _{o}}}{\frac {\partial p}{\partial y}}+K_{m}{\frac {\partial ^{2}v}{\partial z^{2}}},\\[5pt]0&=-{\frac {1}{\rho _{o}}}{\frac {\partial p}{\partial z}},\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b414923e6bbccd402f4da50e38b19f783ef876e0)
















![{\displaystyle {\begin{aligned}u&=u_{g}+{\frac {\sqrt {2}}{\rho _{o}fd}}e^{z/d}\left[\tau ^{x}\cos(z/d-\pi /4)-\tau ^{y}\sin(z/d-\pi /4)\right],\\[5pt]v&=v_{g}+{\frac {\sqrt {2}}{\rho _{o}fd}}e^{z/d}\left[\tau ^{x}\sin(z/d-\pi /4)+\tau ^{y}\cos(z/d-\pi /4)\right],\\[5pt]d&={\sqrt {2K_{m}/|f|}}.\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/fd8c7721137c50a08bef42bcd4c0b55ce89263e9)








![{\displaystyle w={\frac {1}{f\rho _{o}}}\left[-\left({\frac {\partial \tau ^{x}}{\partial x}}+{\frac {\partial \tau ^{y}}{\partial y}}\right)e^{z/d}\sin(z/d)+\left({\frac {\partial \tau ^{y}}{\partial x}}-{\frac {\partial \tau ^{x}}{\partial y}}\right)(1-e^{z/d}\cos(z/d))\right].}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7300fc583368544b2d93fa68b4c97d6430129a17)

![{\displaystyle \ \left[\nu \right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9f42e24bd3af001fe2dd599781fc7cef71f07e14)

