Atom (measure theory)
|
Read other articles:

Mascot of the San Antonio Spurs The Coyote showing a sign to the crowd during a time-out at a San Antonio Spurs game. The Coyote is the official mascot of the San Antonio Spurs, a professional basketball team in the National Basketball Association (NBA). First introduced in 1983, he was inducted into the Mascot Hall of Fame in 2007.[1] History The Coyote was first introduced in public at a San Antonio Spurs game on April 13, 1983.[1] He's known for his slapstick comedy routine...

Basilika Tuhan Yesus BaikBasilika Minor Tuhan Yesus BaikPortugis: Santuário Basílica Bom Jesus do Livramentocode: pt is deprecated Basilika Tuhan Yesus BaikLokasiLiberdadeNegara BrasilDenominasiGereja Katolik RomaArsitekturStatusBasilika minorStatus fungsionalAktif Basilika Tuhan Yesus Baik (Portugis: Santuário Basílica Bom Jesus do Livramentocode: pt is deprecated ) adalah sebuah gereja basilika minor Katolik yang terletak di Liberdade, Brasil. Basilika ini ditetapkan statusnya pada...

Questa voce o sezione sull'argomento edizioni di competizioni calcistiche non cita le fonti necessarie o quelle presenti sono insufficienti. Puoi migliorare questa voce aggiungendo citazioni da fonti attendibili secondo le linee guida sull'uso delle fonti. Segui i suggerimenti del progetto di riferimento. Allsvenskan 1999 Competizione Allsvenskan Sport Calcio Edizione 75ª Organizzatore SvFF Date dal 10 aprile 1999al 30 ottobre 1999 Luogo Svezia Partecipanti 14 Formula Gir...

Об экономическом термине см. Первородный грех (экономика). ХристианствоБиблия Ветхий Завет Новый Завет Евангелие Десять заповедей Нагорная проповедь Апокрифы Бог, Троица Бог Отец Иисус Христос Святой Дух История христианства Апостолы Хронология христианства Ран�...

Eriskirch Lambang kebesaranLetak Eriskirch di Bodenseekreis NegaraJermanNegara bagianBaden-WürttembergWilayahTübingenKreisBodenseekreisPemerintahan • MayorMarkus SpiethLuas • Total14,58 km2 (563 sq mi)Ketinggian400 m (1,300 ft)Populasi (2021-12-31)[1] • Total4.967 • Kepadatan3,4/km2 (8,8/sq mi)Zona waktuWET/WMPET (UTC+1/+2)Kode pos88097Kode area telepon07541Pelat kendaraanFNSitus webwww.eriskirch.de E...

Voce principale: Sportverein Wehen 1926 Taunusstein. Sportverein Wehen 1926 TaunussteinStagione 2009-2010Sport calcio Squadra Wehen Allenatore Hans-Werner Moser (1ª-22ª) Gino Lettieri (23ª-38ª) All. in seconda Steffen Vogler 3. Liga15º posto Coppa di GermaniaPrimo turno Maggiori presenzeCampionato: Reinert, Ziemer (35)Totale: Reinert, Ziemer (36) Miglior marcatoreCampionato: Öztürk, Ziemer (7)Totale: Öztürk, Bohl, Ziemer (7) StadioBRITA-Arena Maggior numero di spettatori8 ...

Jean RenoJean Reno at Cannes 2002LahirJuan Moreno y Herrera JiménezTahun aktif1980 - sekarangSuami/istriZofia Borucka Jean Reno (lahir 30 Juni 1948) merupakan seorang aktor berkebangsaan Prancis. Dia lebih banyak berkarya film di Prancis dan Inggris. Dia dilahirkan di Casablanca, Maroko. Dia berkarier di dunia film sejak tahun 1980. Dilahirkan dengan nama Juan Moreno y Herrera Jiménez. Filmografi Tahun Judul Sebagai Catatan 1980 The Moroccan Stallion 1982 La Passante du Sans-Souci 198...

Cruz latina. Cruz monumental en el Valle de los Caídos, en España. La cruz latina es una cruz formada por dos segmentos de diversa medida que se intersecan en ángulo recto, donde el segmento menor tiene una proporción de tres cuartos respecto del más largo. Refiere a la forma del crucifijo de la tradición cristiana. Arquitectura En arquitectura hay edificios con una forma de planta denominada de cruz latina: corresponde al diseño utilizado en las iglesias en las que la nave mayor tiene...

Great Officer of State for England Not to be confused with the Lord Chamberlain. Lord Great Chamberlain of EnglandIncumbentRupert Carington, 7th Baron Carringtonsince 8 September 2022 Joint hereditary officeholders Cholmondeley share: The 7th Marquess of Cholmondeley (50%) Ancaster share: The 28th Baroness Willoughby de Eresby (25%) Carrington share: The 10th Earl of Albemarle (5%) Nicholas Llewellen Palmer (5%) Harry Legge-Bourke (5%) Lorraine Wilson (1.66%) Tatiana Dent (1.66%) Ines Ga...

Флаг гордости бисексуалов Бисексуальность Сексуальные ориентации Бисексуальность Пансексуальность Полисексуальность Моносексуальность Сексуальные идентичности Би-любопытство Гетерогибкость и гомогибкость Сексуальная текучесть Исследования Шк...

Untuk kegunaan lain, lihat Isin (disambiguasi). IsinLokasi di IraqLokasiIshan al-Bahriyat, Kegubernuran Al-Qādisiyyah, IrakWilayahMesopotamiaKoordinat31°53′06″N 45°16′07″E / 31.88500°N 45.26861°E / 31.88500; 45.26861Koordinat: 31°53′06″N 45°16′07″E / 31.88500°N 45.26861°E / 31.88500; 45.26861JenisPermukiman Isin (Sumerian: 𒉌𒋛𒅔𒆠, translit: I3-si-inkicode: sux is deprecated ,[1] Bahasa Arab modern:...

Логотип ІКОМОСМіжнародна рада з охорони пам'яток та історичних місць (ІКОМОС, англ. ICOMOS — англ. International Council on Monuments and Sites) — міжнародна асоціація професіоналів, метою діяльності якої є збереження та захист культурної спадщини в усьому світі. Заснована 1965 року у Варша...

Eight-thousander and 10th-highest mountain on Earth, located in Nepal This article is about the individual mountain. For the mountain range, see Annapurna (mountain range). For other uses, see Annapurna (disambiguation). For the Hindu goddess, see Annapurna (goddess). AnnapurnaSouth face of Annapurna I (Main)Highest pointElevation8,091 m (26,545 ft)Ranked 10thProminence2,984 m (9,790 ft)[1][2]Ranked 94thParent peakCho OyuListingEight-thousanderUltraCoo...

朴島 朴島の空中写真。2019年4月29日撮影。国土交通省 国土地理院 地図・空中写真閲覧サービスの空中写真を基に作成所在地 日本 宮城県塩竈市所在海域 松島湾所属諸島 浦戸諸島座標 北緯38度21分04秒 東経141度07分26秒 / 北緯38.35111度 東経141.12389度 / 38.35111; 141.12389面積 0.15 km²海岸線長 2.2 km最高標高 23 m朴島朴島 (宮城県)宮城県の地図を表示朴島朴島 (�...

Questa voce sull'argomento attori statunitensi è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti del progetto di riferimento. Clem Bevans (1940) Clem Guy Bevans (Cozaddale, 16 ottobre 1880 – Woodland Hills, 11 agosto 1963) è stato un attore statunitense. Indice 1 Filmografia parziale 1.1 Cinema 1.2 Televisione 2 Altri progetti 3 Collegamenti esterni Filmografia parziale Cinema Cuori incatenati (Way Down East), regia di Henry King...

Pulau Opak BesarNegaraIndonesiaGugus kepulauanKepulauan SeribuProvinsiDKI JakartaKabupatenKepulauan SeribuLuas- km²Populasi- Pulau Opak Besar adalah sebuah pulau yang terletak di Kepulauan Seribu di Daerah Khusus Ibukota Jakarta, Indonesia. pulau opak besar termasuk dalam gugusan kelurahan kepulauan Harapan Lihat pula Kabupaten Administratif Kepulauan Seribu Kepulauan Seribu Pranala luar Situs resmi Kabupaten Administratif Kepulauan Seribu Diarsipkan 2017-02-22 di Wayback Machine. lbsPu...

Keyword assigned to information Not to be confused with Markup language or HTML element tags. Not to be confused with Hashtag or Mention (blogging). A tag cloud with terms related to Web 2.0 In information systems, a tag is a keyword or term assigned to a piece of information (such as an Internet bookmark, multimedia, database record, or computer file). This kind of metadata helps describe an item and allows it to be found again by browsing or searching.[1] Tags are generally chosen i...
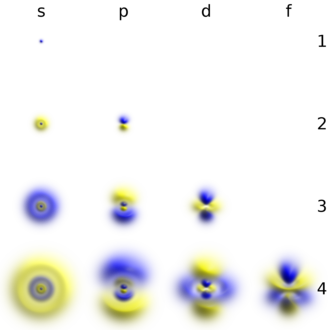
Tabular arrangement of the chemical elements ordered by atomic number This article is about the table used in chemistry and physics. For other uses, see Periodic table (disambiguation). Periodic table of the chemical elements showing the most or more commonly named sets of elements (in periodic tables), and a traditional dividing line between metals and nonmetals. The f-block actually fits between groups 2 and 3; it is usually shown at the foot of the table to save horizontal space. Part of a...

Mojito dalam sebuah gelas Mojito (IPA: /moʊˈhiːtoʊ/; pelafalan Spanyol: [moˈxito]) adalah sebuah minuman beralkohol khas Kuba. Sebuah mojito biasanya dibuat dari lima bahan: rum putih, gula (biasanya gula tebu), limun, air soda, dan daun mint. Kombinasi antara rasa manis, limun yang segar dan mint dimaksudkan untuk menutupi rum yang efeknya keras. Dengan itu minuman ini sangat populer sebagai minuman musim panas di Dunia Barat. Dalam membuat mojito, air perasan limun ditambahkan pada gul...

The Song of Tentomushiてんとう虫の歌(Tentōmushi no Uta) MangaPengarangNoboru KawasakiPenerbitShogakukanImprintTentōmushi ComicsMajalahShogakukan no Gakushō ZasshiDemografiMangaTerbit1973 – 1975Volume4 Seri animeSutradaraHiroshi SasagawaSkenarioShigeru YanagigawaTakao KoyamaMusikShunsuke KikuchiStudioTatsunoko ProductionSaluranasliFuji TVTayang 6 October 1974 – 26 September 1976Episode104 Portal anime dan manga The Song of Tentomushi (てんとう虫の歌 Tentōmushi n...








![{\displaystyle [A]:=\{B\in \Sigma :\mu (A\Delta B)=0\},}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d38c677a5ffffc62e0fd34c64c9b2b1ceddd81c6)

![{\displaystyle [A]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/79eaa334597b1861f1b08ca0c8fecb3858ebcb12)














![{\displaystyle X=[0,1]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/96de4495b54d28b3439ca59802d8730adcb3a588)












![{\displaystyle S:[0,c]\to \Sigma }](https://wikimedia.org/api/rest_v1/media/math/render/svg/d6b4a336ebd0f9d7be72dec0166191097ea77241)
![{\displaystyle \mu :\Sigma \to [0,c].}](https://wikimedia.org/api/rest_v1/media/math/render/svg/70aef2928a40a315e370c117f762e91bb7a3fe7e)




![{\displaystyle \Gamma :=\{S:D\to \Sigma \;:\;D\subseteq [0,c],\,S\;\mathrm {monotone} ,{\text{ for all }}t\in D\;(\mu (S(t))=t)\},}](https://wikimedia.org/api/rest_v1/media/math/render/svg/27d93764d6a7ff4948007ac23370a7b46e935af6)



![{\displaystyle [0,c],}](https://wikimedia.org/api/rest_v1/media/math/render/svg/590285386c104e35d623a0f2ed3652516ae9d400)