Read other articles:

Nila Djuwita MoeloekPotret resmi Nila Moeloek sebagai Menteri Kesehatan Indonesia (2014). Menteri Kesehatan Indonesia ke-18Masa jabatan27 Oktober 2014 – 20 Oktober 2019PresidenJoko WidodoWakil PresidenJusuf Kalla PendahuluNafsiah MboiPenggantiTerawan Agus Putranto Informasi pribadiLahir11 April 1949 (umur 74)Jakarta, IndonesiaPartai politikIndependenSuami/istriFaried Anfasa MoeloekHubunganAbdul Moeloek (mertua)Nukman Helwi Moeloek (ipar)Anak3Alma materUniversitas Indonesia...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada November 2022. Enílton Menezes de MirandaInformasi pribadiTanggal lahir 11 Oktober 1977 (umur 46)Tempat lahir BrasilPosisi bermain PenyerangKarier senior*Tahun Tim Tampil (Gol)2007 Omiya Ardija * Penampilan dan gol di klub senior hanya dihitung dari liga domes...
هذه المقالة بحاجة لصندوق معلومات. فضلًا ساعد في تحسين هذه المقالة بإضافة صندوق معلومات مخصص إليها. حي القرابيص هو حي يقع شمال مدينة حمص السورية. يقع الحي بالقرب من منطقة البساتين، ويعد هذا الحي عقدة مواصلات هامة في المدينة، وهو من الأحياء التي شهدت حراكاً شعبياً كبيراً منا�...
This article does not cite any sources. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Przemyśl Voivodeship – news · newspapers · books · scholar · JSTOR (May 2023) (Learn how and when to remove this template message) Przemyśl VoivodeshipWojewództwo przemyskieVoivodeship of Poland1975–1998Location within PolandCapitalPrzemyślArea • 19984,437 ...

Japanese actor Taiji Tonoyama殿山 泰司Born(1915-10-17)October 17, 1915DiedApril 30, 1989(1989-04-30) (aged 73)OccupationActor Taiji Tonoyama (殿山 泰司, Tonoyama Taiji, October 17, 1915 – April 30, 1989) was a Japanese character actor who made many appearances in films and on television from 1939 to 1989.[1] He was a close friend of Kaneto Shindo and one of his regular cast members.[2] He was also an essayist. In 1950 he helped form the film company Kindai E...

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (أبريل 2019) تيرينو ادرياتيكو 2007 تفاصيل السباقسلسلة42. تيرينو أدرياتيكومنافسةبرو تور 2007مراحل7التواريخ14 – 20 مارس 2007الم...

Mohamed Kalil Traoré Nazionalità Guinea Calcio Ruolo Attaccante Squadra Stade Beaucairois CarrieraSquadre di club1 2020-2021 Kamsar? (?)2021-2022 Hapoel Tel Aviv17 (0)2023- Stade Beaucairois11 (0)Nazionale 2021- Guinea6 (0) 1 I due numeri indicano le presenze e le reti segnate, per le sole partite di campionato.Il simbolo → indica un trasferimento in prestito. Statistiche aggiornate al 21 agosto 2023 Modifica dati su Wikidata · Manuale Mohamed Kalil T...

Species of crustacean Panulirus homarus Conservation status Least Concern (IUCN 3.1)[1] Scientific classification Domain: Eukaryota Kingdom: Animalia Phylum: Arthropoda Class: Malacostraca Order: Decapoda Suborder: Pleocyemata Family: Palinuridae Genus: Panulirus Species: P. homarus Binomial name Panulirus homarus(Linnaeus, 1758) Subspecies[2] P. h. homarus (Linnaeus, 1758) P. h. megasculpta (Pesta, 1915) P. h. rubellus Berry, 1974 Synonyms[3]...
2020年夏季奥林匹克运动会波兰代表團波兰国旗IOC編碼POLNOC波蘭奧林匹克委員會網站olimpijski.pl(英文)(波兰文)2020年夏季奥林匹克运动会(東京)2021年7月23日至8月8日(受2019冠状病毒病疫情影响推迟,但仍保留原定名称)運動員206參賽項目24个大项旗手开幕式:帕维尔·科热尼奥夫斯基(游泳)和马娅·沃什乔夫斯卡(自行车)[1]闭幕式:卡罗利娜·纳亚(皮划艇)&#...

Para catherinette di Paris, rue de la Paix, pada tahun 1932 Catherinette adalah sebuah label Prancis tradisional untuk para gadis berusia dua puluh lima tahun yang masih belum menikah pada Perayaan Santa Katarina (25 November). Sebuah perayaan khusus ditawarkan kepada mereka pada hari itu, di mana setiap orang mengharapkan mereka mengakhiri masa lajang mereka. Pranala luar Lihat entri catherinette di kamus bebas Wiktionary. Wikimedia Commons memiliki media mengenai Catherinettes. Legend of th...

Eparki KairoEparchia Cahirensis ChaldaeorumLokasiNegara MesirMetropolitSubyek Langsung PatriatkPopulasi- Katolik(per 2004)500InformasiRitusRitus KaldeaKatedralKatedral Bunda dari FatimaKepemimpinan kiniUskuptahta lowong Eparki Katolik Kaldea Kairo (bahasa Prancis: Le Caire, bahasa Latin: Eparchia Cahirensis Chaldaeorum) adalah sebuah eparki yang terletak di kota Kairo, Mesir. Eparki tersebut didirikan pada 23 April 1980 Referensi GCatholic.org Catholic Hierarchy lbsKeus...

This is a list of places in Iraq. Governorates of Iraq lists the governorates, and Districts of Iraq lists the subdivisions of those governorates. Modern cities and towns Main article: List of cities in Iraq Iraq Map Afak (عفك) Samarra Al `Awja (العوجا) Baghdad (ܒܓܕܐܕ) (بغداد) Kadhimiya(الكاظمية) Sadr City (مدينة الصدر) Green Zone (المنطقة الخضراء) List of neighborhoods and districts in Baghdad Baghdadi Bayji (بيجي) Balad (بلد) Ba`qub...

أديب بن حسن الشيشكلي رئيس الجمهورية السورية العاشر في المنصب11 يوليو 1953 – 25 فبراير 1954 فوزي السلو هاشم الأتاسي رئيس وزراء سوريا في المنصب19 يوليو 1953 – 1 مارس 1954 معلومات شخصية الميلاد 1909حماة، سوريا العثمانية الوفاة 27 سبتمبر 1964 (55 سنة)غوياس، البرازيل سبب الوفاة إصابة بعيار �...

Sculpture from ancient Greece and Rome Leochares: Belvedere Apollo. Roman copy of 130–140 AD after a Greek bronze original of 330–320 BC. Vatican Museums Classical sculpture (usually with a lower case c) refers generally to sculpture from Ancient Greece and Ancient Rome, as well as the Hellenized and Romanized civilizations under their rule or influence, from about 500 BC to around 200 AD. It may also refer more precisely a period within Ancient Greek sculpture from around 500 BC to the o...

Volkswagen Transporter (T4)Volkswagen Caravelle GLInformasiProdusenVolkswagen Commercial VehiclesJuga disebutVolkswagen Eurovan (Amerika Utara)Volkswagen CaravelleMasa produksi1990–2003PerakitanHannover, JermanPoznań, PolandiaJakarta, Indonesia (Garuda Mataram Motor)Taoyuan, TaiwanBodi & rangkaKelasKendaraan komersial ringanBentuk kerangka4-pintu van5-pintu van6-pintu van2-pintu pickup platform 3-pintu pickup platform4-pintu pickup platform4-pintu campervanTata letakMesin dep...

AllsvenskanSäsong2009Nya lag för säsongenIF Brommapojkarna Örgryte IS BK HäckenVinnareAIK(5:e allsvenska titeln)(11:e SM-titeln)NedflyttadeÖrgryte IS Hammarby IFChampions LeagueAIKEuropa LeagueIFK Göteborg IF Elfsborg Kalmar FFStatistikTotalt antal mål584 (2,43 per match)Bästa målgörareTobias Hysén (18) Wanderson (18)Största hemmavinst6 - 0 (IFKG - DIF)Största bortavinst1 - 5 (ÖIS - GAIS)Högsta åskådarantal26 241 (AIK - DIF)Lägsta åskåda...

此條目没有列出任何参考或来源。 (2013年7月21日)維基百科所有的內容都應該可供查證。请协助補充可靠来源以改善这篇条目。无法查证的內容可能會因為異議提出而被移除。 伊塔瓜伊Itaguaí市镇伊塔瓜伊在巴西的位置坐标:22°51′07″S 43°46′30″W / 22.8519°S 43.775°W / -22.8519; -43.775国家巴西州里约热内卢州面积 • 总计272 平方公里(105 平方英�...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Desember 2023. Ini adalah daftar maskapai penerbangan yang saat ini beroperasi di Seychelles. Maskapai penerbangan IATA ICAO Tanda panggil Air Seychelles HM SEY SEYCHELLES Lihat pula Daftar maskapai penerbangan Artikel bertopik maskapai penerbangan ini adalah sebuah...

Sporting event delegationLaos at the2017 World Aquatics ChampionshipsFlag of LaosFINA codeLAONational federationLaos Swimming Federationin Budapest, HungaryCompetitors3 in 1 sportMedals Gold 0 Silver 0 Bronze 0 Total 0 World Aquatics Championships appearances197319751978198219861991199419982001200320052007200920112013201520172019202220232024 Laos competed at the 2017 World Aquatics Championships in Budapest, Hungary from 14 July to 30 July. Swimming Main article: Swimming at the 2017 World A...
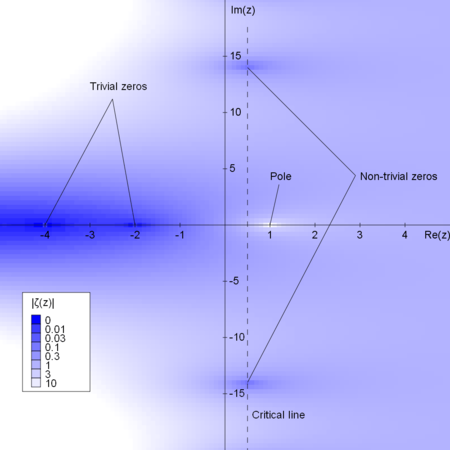
Conjecture on zeros of the zeta function For the musical term, see Riemannian theory. This plot of Riemann's zeta (ζ) function (here with argument z) shows trivial zeros where ζ(z) = 0, a pole where ζ(z) = ∞ {\displaystyle \infty } , the critical line of nontrivial zeros with Re(z) = 1/2 and slopes of absolute values.In mathematics, the Riemann hypothesis is the conjecture that the Riemann zeta function has its zeros only at the negative even integers and complex numbers with real p...