수학에서 평면에 주어진 점과 같은 평면에 있는 닫힌 곡선의 감김 수(영어: Winding number) 또는 감김 지수(영어: winding index)는 곡선이 점 주위에서 시계 반대 방향으로 감는 총 횟수를 나타내는 정수다. 즉, 곡선이 주어진 점 주위를 몇 번 회전하였는지를 나타낸다. 김김 수는 곡선이 점을 감는 방향에 따라 다르다. 시계 반대 방향으로 감는 경우를 양수를 부여하고, 시계 방향으로 점 주위를 감는 경우 음수를 부여한다.

감김 수는 대수적 위상수학의 기본적 대상이며 벡터 미적분학, 복소해석학, 기하학적 위상수학, 미분기하학뿐만 아니라, 수리물리학, 이론물리학(예: 끈 이론)에서도 중요한 역할을 한다.

평면에 방향이 주어진 닫힌 곡선이 있다고 가정하자. 곡선을 한 점 ${\displaystyle A}$가 이동하는 자취라고 생각하자. 그러면, 곡선의 감김 수는 점이 원점을 중심으로 시계 반대 방향으로 회전하는 총 수와 같다.

총 회전 수를 계산할 때 시계 반대 방향은 양수로, 시계 방향은 음수로 계산한다. 예를 들어 점 ${\displaystyle A}$가 처음에 시계 반대 방향으로 원점을 네 번 돌고 그 다음 시계 방향으로 원점을 한 번 돌면 곡선이 점을 감은 횟수는 세 번이다.

이 방식에 따르면, 원점 주위를 전혀 이동하지 않는 곡선은 감김 횟수가 0인 반면, 원점 주위를 시계 방향으로 이동하는 곡선은 음의 감김 횟수를 갖는다. 따라서 곡선의 감김 수는 정수가 될 수 있다. 다음 그림은 −2와 3 사이의 감김 수가 있는 곡선을 보여준다.

${\displaystyle \cdots }$
	− 2	− 1	0
				${\displaystyle \cdots }$
	1	2	3

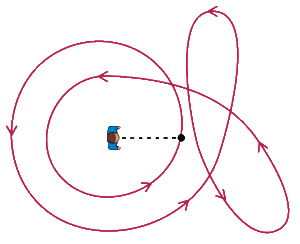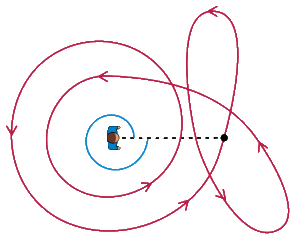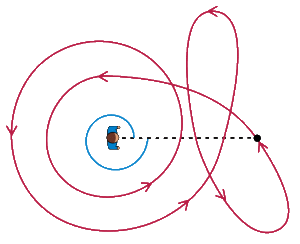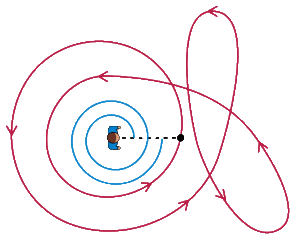
${\displaystyle \gamma :[0,1]\to \mathbb {C} \setminus \{a\}}$를 한 점 ${\displaystyle a}$를 뺀 평면 위에 있는 닫힌 곡선이라 하자. ${\displaystyle \gamma }$가 ${\displaystyle a}$ 주위를 감는 수는

${\displaystyle {\text{wind}}(\gamma ,a)=s(1)-s(0)}$

이다. 여기서 ${\displaystyle (\rho ,s)}$는 극좌표로 표현된 곡선, 즉, 다음 덮개 사상을 통해 들어올려진 곡선이다.

${\displaystyle p:\mathbb {R} _{>0}\times \mathbb {R} \to \mathbb {C} \setminus \{a\}:(\rho _{0},s_{0})\mapsto a+\rho _{0}e^{i2\pi s_{0}}.}$

감김 수는 들어 올려진 경로의 존재성과 유일성(덮개 공간의 시작점 지정)과 모든 올이 ${\displaystyle p}$ 형식이다 ${\displaystyle \rho _{0}\times (s_{0}+\mathbb {Z} )}$ (따라서 위의 표현은 시작점의 선택에 의존하지 않는다). 곡선이 닫혀 있기 때문에 정수이다.

감김 수는 종종 수학의 다양한 부분에서 다른 방식들으로 정의된다. 아래의 모든 정의는 위에 주어진 정의와 동일하다.

1865년 아우구스트 페르디난트 뫼비우스는 감김 수를 정의하기 위한 간단한 조합 규칙을 제안했으며^[1] 1928년 제임스 워델 알렉산더가 다시 독립적으로 제안했다.^[2] 모든 곡선은 평면을 여러 연결 성분들로 분할하며, 그 중 하나는 유계가 아니다. 같은 연결 성분에 있는 두 점 주위의 곡선의 감김 수는 동일하다. 유계가 아닌 연결 성분 안의 임의의 점에 대한 감김 수는 0이다. 마지막으로, 인접한 두 연결 성분의 감김 수는 정확히 1만큼 다르다. 더 큰 감김 수를 가진 연결 성분이 곡선의 왼쪽에 나타난다.

미분기하학에서 매개변수 방정식은 일반적으로 미분 가능(또는 적어도 부분적으로 미분 가능)하다고 가정한다. 이 경우 극좌표 ${\displaystyle \theta }$는 다음 방정식에 의해 데카르트 좌표 ${\displaystyle x,y}$와 관련된다.

${\displaystyle d\theta ={\frac {1}{r^{2}}}\left(x\,dy-y\,dx\right)\quad {\text{where }}r^{2}=x^{2}+y^{2}.}$

이는 ${\displaystyle \theta }$에 대한 다음 정의를 미분하여 찾을 수 있다.

${\displaystyle \theta (t)=\arctan {\bigg (}{\frac {y(t)}{x(t)}}{\bigg )}}$

미적분학의 기본정리에 의해 ${\displaystyle \theta }$의 전체 변화는 ${\displaystyle d\theta }$의 적분과 같다. 따라서 미분 가능한 곡선의 감김 수를 선적분으로 표현할 수 있다.

${\displaystyle {\text{wind}}(\gamma ,0)={\frac {1}{2\pi }}\oint _{\gamma }\,\left({\frac {x}{r^{2}}}\,dy-{\frac {y}{r^{2}}}\,dx\right).}$

제 1형식 ${\displaystyle d\theta }$(원점의 여집합에서 정의됨)는 닫힌 형식이지만 완전 형식은 아니며 구멍이 뚫린 평면의 첫 번째 드람 코호몰로지 군을 생성한다. 특히, ${\displaystyle \omega }$가 원점의 여집합에 정의된 닫힌 제 1미분형식인 경우 폐곡선을 따라 ${\displaystyle \omega }$를 적분하면 감김 수의 배수가 된다.

감김 수는 복소해석학에서 아주 중요한 역할을 한다(유수 정리 설명 참조). 복소해석학의 맥락에서 폐곡선 ${\displaystyle \gamma }$의 감김 수를 복소평면에서 복소 좌표 ${\displaystyle z=x+yi}$로 표현 할 수 있다. 구체적으로, ${\displaystyle z=re^{i\theta }}$라고 쓰면,

${\displaystyle dz=e^{i\theta }dr+ire^{i\theta }d\theta }$

따라서,

${\displaystyle {\frac {dz}{z}}={\frac {dr}{r}}+i\,d\theta =d[\ln r]+i\,d\theta .}$

${\displaystyle \gamma }$가 폐곡선이고 총 변화량은 ${\displaystyle \ln(r)}$ 0이므로 ${\textstyle {\frac {dz}{z}}}$를 적분한 값은 ${\displaystyle i}$에 ${\displaystyle \theta }$의 총 변화량을 곱한 값과 같다. 따라서, 원점에 대한 폐곡선 ${\displaystyle \gamma }$의 감김 수는^[3]

${\displaystyle {\frac {1}{2\pi i}}\oint _{\gamma }{\frac {dz}{z}}\,.}$

일반적으로, ${\displaystyle \gamma }$가 ${\displaystyle t\in [\alpha ,\beta ]}$에 의해 매개변수화된 닫힌 곡선이라 하자. ${\displaystyle z_{0}}$에 대한 ${\displaystyle \gamma }$ 의 감김 수 또는 ${\displaystyle z_{0}}$ 대한 ${\displaystyle \gamma }$의 지표는 복소수${\displaystyle z_{0}\notin \gamma ([\alpha ,\beta ])}$에 대해 다음과 같이 정의된다:^[4]

${\displaystyle \mathrm {Ind} _{\gamma }(z_{0})={\frac {1}{2\pi i}}\oint _{\gamma }{\frac {d\zeta }{\zeta -z_{0}}}={\frac {1}{2\pi i}}\int _{\alpha }^{\beta }{\frac {\gamma '(t)}{\gamma (t)-z_{0}}}dt.}$

이것은 유명한 코시 적분 공식의 특별한 경우이다.

복소 평면에서 감김 수의 기본 성질들 중 일부는 다음 정리에 의해 제공된다.^[5]

정리. ${\displaystyle \gamma :[\alpha ,\beta ]\to \mathbb {C} }$가 닫힌 곡선이고 ${\displaystyle \Omega }$가 . ${\displaystyle \gamma }$의 여집합이라고 하자. 즉, ${\displaystyle \Omega :=\mathbb {C} \setminus \gamma ([\alpha ,\beta ])}$. 그러면 ${\displaystyle z}$의 지수는 ${\displaystyle \gamma }$에 대하여,$${\displaystyle \mathrm {Ind} _{\gamma }:\Omega \to \mathbb {C} ,\ \ z\mapsto {\frac {1}{2\pi i}}\oint _{\gamma }{\frac {d\zeta }{\zeta -z}},}$$$${\displaystyle \mathrm {Ind} _{\gamma }:\Omega \to \mathbb {C} ,\ \ z\mapsto {\frac {1}{2\pi i}}\oint _{\gamma }{\frac {d\zeta }{\zeta -z}}}$$는 (i) 정수 값, 즉, 모든 ${\displaystyle z\in \Omega }$에 대해 ${\displaystyle \mathrm {Ind} _{\gamma }(z)\in \mathbb {Z} }$; (ii) ${\displaystyle \Omega }$의 각 연결 성분에 대해 상수; (iii) ${\displaystyle z}$가 유계가 아닌 ${\displaystyle \Omega }$의 연결 성분에 있으면 0.

즉각적인 결과로서 이 정리는 점 ${\displaystyle z}$에 대한 원형 곡선 ${\displaystyle \gamma }$의 감김 수를 제공한다. 예상대로 감김 횟수는 시계 반대 방향으로 ${\displaystyle z}$ 주위를 도는 고리 ${\displaystyle \gamma }$들의 수를 세는 것이다:

따름정리. ${\displaystyle \gamma }$가 ${\displaystyle \gamma (t)=a+re^{int},\ \ 0\leq t\leq 2\pi ,\ \ n\in \mathbb {Z} }$로 정의된 곡선이라 하자. 그러면, ${\displaystyle \mathrm {Ind} _{\gamma }(z)={\begin{cases}n,&|z-a|<r;\\0,&|z-a|>r.\end{cases}}}$

위상수학에서 감김 수는 브라우어르 차수의 다른 이름이다.

위의 한 점을 중심으로 하는 곡선의 예는 간단한 위상수학적 의미를 갖는다. 평면에서 한 점의 여집합은 원과 동등한 호모토피이므로 원에서 그 자체로의 사상이 실제로 고려해야 할 전부이다. 그러한 각각의 사상은 표준 사상 ${\displaystyle S^{1}\to S^{1}:s\mapsto s^{n}}$들 중 하나로 연속적으로 변형될 수 있음을 보여줄 수 있다(호모토픽하다). 여기서 원의 곱셈은 복소 평면에서 단위 원 상의 복소수 ${\displaystyle e^{i\theta }}$들의 곱으로 정의된다. 원에서 위상 공간으로 가는 사상의 호모토피 동치류 집합은 군을 형성하며, 이를 첫 번째 호모토피 군 또는 해당 공간의 기본군이라고 한다. 원의 기본군은 정수 군 ${\displaystyle \mathbb {Z} }$이다. 일반적 곡선의 감김 수는 이의 호모토피 동치류일 뿐이다.

3차원 구면에서 그 자체로의 사상은 감김 수 또는 때때로 폰트랴긴 지수라고도 하는 정수로 분류된다.

또한 곡선의 접벡터와 관련하여 곡선의 감김 수를 고려할 수 있다. 이는 곡선의 접벡터가 만드는 곡선의 원점에 대한 감김 수이다. 이 경우 이 문서의 시작 부분에 설명된 예에서는 작은 고리가 계산되기 때문에 감김 번호가 3이다.

이것은 몰입된 곡선에 대해서만 정의되며(즉, 도함수가 사라지지 않는 미분 가능한 경로에 대해) 접선 가우스 사상의 차수이다.

이것을 선회수, 회전수,^[6] 회전 지수^[7] 또는 곡선의 지수라고 하며, 전곡률을 π로 나눈 값으로 계산할 수 있다.

다각형에서 회전 수는 다각형 밀도라고 한다. 볼록 다각형 및 보다 일반적으로 단순한 다각형 (자기 교차하지 않음)의 경우 조르당 곡선 정리에 따라 밀도는 1이다. 대조적으로 정칙 별 모양 다각형 {p/q}의 경우 밀도는 q이다.

각도에는 일치하는 치수가 필요하므로 3차원 공간 안에 있는 일반적 곡선에 대해 회전 수를 정의할 수 없다. 그러나 국소적으로 볼록하고 닫힌 곡선의 경우 접선 회전 기호를 ${\displaystyle (-1)^{d}}$로 정의할 수 있다. 여기서 ${\displaystyle d}$는 탄젠트 표시의 입체 사영의 회전 수이다. 그것의 두 값은 국소적으로 볼록한 곡선의 두 비퇴화 호모토피 동치류에 해당한다.^[8][9]

감김 수는 (2+1) 차원 연속 하이젠베르크 강자성체 방정식과 이 방정식의 적분가능한 확장: 이시모리 방정식 등 마지막 방정식의 해는 감김 수 또는 위상 전하 (위상 불변량 및 위상 양자 수)로 분류된다.

다각형에 대한 점의 감김 수는 다각형 안의 점 문제를 해결하는 데 사용할 수 있다. 즉, 점이 다각형 내부에 있는지 여부를 결정하는 데 사용할 수 있다.

일반적으로 레이 캐스팅 알고리듬 은 감김 수 알고리즘과 달리 삼각 함수가 필요하지 않기 때문에 PIP 문제에 대한 더 나은 대안이다. 그럼에도 불구하고 감김 수 알고리듬은 삼각 함수와 관련된 계산이 필요하지 않도록 속도를 높일 수 있다.^[10] 단순하지 않은 다각형도 고려해야 하는 경우 선데이 알고리듬이라고도 하는 알고리듬의 속도 향상 버전을 권장한다.

• 편각 원리
• 연환수
• Nonzero Winding Rule
• 밀도 (다포체)
• 슐레플리 기호
• 위상수학적 양자 수
• 윌슨 고리