分解 (ホモロジー代数)
|
Read other articles:

Town in Massachusetts, United StatesLeicester, MassachusettsTownLeicester Town Hall SealLocation in Worcester County and the state of Massachusetts.Coordinates: 42°14′45″N 71°54′33″W / 42.24583°N 71.90917°W / 42.24583; -71.90917CountryUnited StatesStateMassachusettsCountyWorcesterSettled1713Incorporated1714Government • TypeOpen town meeting • Town AdministratorDavid A. Genereux • Board of SelectmenAllen Phillips (Chair) (...

Mitsubishi Ki-21Mitsubishi Ki-21 dari Sekolah Pengebom HamamatsuTipePengebom mediumTerbang perdana18 Desember 1936Diperkenalkan1938Pengguna utamaJepangPengguna lainThailandJumlah produksi2.064 units (tidak termasuk Ki-57)VarianMitsubishi Ki-57 Mitsubishi Ki-21 (九七式重爆撃機code: ja is deprecated , Kyūnana-shiki jūbakugekiki) (Nama sandi sekutu: Sally /Gwen) adalah pesawat pengebom Jepang selama Perang Dunia II. Pesawat ini memulai operasi tempurnya pada Perang Perbatasan Soviet-Jep...

English filmmaker This article's use of external links may not follow Wikipedia's policies or guidelines. Please improve this article by removing excessive or inappropriate external links, and converting useful links where appropriate into footnote references. (June 2023) (Learn how and when to remove this message) Jennings in 2008 Garth Jennings is an English director, screenwriter and actor. Films he has directed include The Hitchhiker's Guide to the Galaxy, Son of Rambow, Sing, and Sing 2....

4,310 acres in West Virginia (US) managed by the National Park Service Bluestone National Scenic RiverIUCN category V (protected landscape/seascape)[1]Bluestone Gorge near the upstream end of the National Scenic River section.Location of Bluestone National Scenic River in West VirginiaShow map of West VirginiaBluestone National Scenic River (the United States)Show map of the United StatesLocationWest Virginia, United StatesCoordinates37°32′30″N 80°59′57″W / 3...
2020年夏季奥林匹克运动会科索沃代表團科索沃国旗IOC編碼KOSNOC科索沃奧林匹克委員會網站www.noc-kosovo.org(英文)(阿爾巴尼亞文)(塞爾維亞文)2020年夏季奥林匹克运动会(東京)2021年7月23日至8月8日(受2019冠状病毒病疫情影响推迟,但仍保留原定名称)運動員11參賽項目6个大项旗手开幕式:阿基爾·賈科瓦(英语:Akil Gjakova)和瑪琳達·開爾門蒂(柔道)[1]闭幕式�...

Chemical compound containing two hydroxyl (–OH) groups Ethylene glycol, a common diol A diol is a chemical compound containing two hydroxyl groups (−OH groups).[1] An aliphatic diol is also called a glycol.[2] This pairing of functional groups is pervasive, and many subcategories have been identified. They are used as protecting groups of carbonyl groups, making them essential in synthesis of organic chemistry.[3] The most common industrial diol is ethylene glycol....

Department of the Ohio state government Department of Job and Family ServicesDepartment overviewFormedJuly 1, 2000 (2000-07-01)Preceding agenciesOhio Department of Human ServicesOhio Bureau of Employment ServicesJurisdictionOhioDepartment executiveMatt Damschroder, Interim directorWebsitejfs.ohio.gov The Ohio Department of Job and Family Services (ODJFS) is the administrative department of the Ohio state government[1] responsible for supervising the state's public assis...
Type of webcomic A panel of a hypothetical sprite comic featuring graphics from The Battle for Wesnoth Sprite comics are webcomics that consist primarily of computer sprites from video games. Art assets are ripped from various classic games such as Mega Man and Sonic the Hedgehog, are edited and combined by amateur cartoonists, and are posted on the internet. Popularized by Bob and George in the early 2000s, the style is considered relatively easy for beginning cartoonists to get involved in,...

Men's singlesat the VII Olympic Winter GamesDates29 January-1 FebruaryCompetitors16 from 11 nationsMedalists Hayes Alan Jenkins United States Ronald Robertson United States David Jenkins United States← 19521960 → Figure skating at the Olympics Figure skating at the1956 Winter OlympicsSinglesmenladiesPairsmixedvte The men's figure skating competition at the 1956 Winter Olympics took place at the Olympic Ice Stadium in Cortina d'Ampezzo, Italy. Th...
Aletshausen. Aletshausen adalah kota yang terletak di distrik Günzburg di Bavaria, Jerman. Kota Aletshausen memiliki luas sebesar 17.66 km² . Aletshausen pada tahun 2006, memiliki penduduk sebanyak 1.133 jiwa. lbsKota dan kotamadya di GünzburgAichen | Aletshausen | Balzhausen | Bibertal | Breitenthal | Bubesheim | Burgau | Burtenbach | Deisenhausen | Dürrlauingen | Ebershausen | Ellzee | Gundremmingen | Günzburg |...

American baseball player (born 1954) Baseball player Ken SchromPitcherBorn: (1954-11-23) November 23, 1954 (age 69)Grangeville, Idaho, U.S.Batted: RightThrew: RightMLB debutAugust 8, 1980, for the Toronto Blue JaysLast MLB appearanceOctober 3, 1987, for the Cleveland IndiansMLB statisticsWin–loss record51–51Earned run average4.81Strikeouts372 Teams Toronto Blue Jays (1980, 1982) Minnesota Twins (1983–1985) Cleveland Indians (1986–1987) Career highlig...

Map all coordinates using OpenStreetMap Download coordinates as: KML GPX (all coordinates) GPX (primary coordinates) GPX (secondary coordinates) This is a list of historic places in Essex County, Ontario, containing heritage sites listed on the Canadian Register of Historic Places (CRHP), all of which are designated as historic places either locally, provincially, territorially, nationally, or by more than one level of government. List of historic places Name Address Coordinates Government r...

本條目存在以下問題,請協助改善本條目或在討論頁針對議題發表看法。 此条目也许具备关注度,但需要可靠的来源来加以彰显。(2022年10月8日)请协助補充可靠来源以改善这篇条目。 此條目過於依赖第一手来源。 (2022年10月8日)请補充第二手及第三手來源,以改善这篇条目。 國立內埔高級農工職業學校國立內埔高級農工職業學校地址912 屏東縣內埔鄉水門村成功路83號经纬...

Church in Cambridge, England Church in Cambridge, EnglandSt Bene't's Church, CambridgeSt Bene't's Church with its Anglo-Saxon tower52°12′13.0″N 0°07′06.0″E / 52.203611°N 0.118333°E / 52.203611; 0.118333LocationBene't Street , CambridgeCountryEnglandDenominationChurch of EnglandWebsitehttps://www.stbenetschurch.orgHistoryStatusActiveFoundedc.1020ArchitectureFunctional statusParish churchHeritage designationGrade I listedSpecificationsTower height70ftBells6Te...

This article is part of a series aboutGeorge W. Bush Political positions Electoral history Early life Professional life Family Public image Honors 46th Governor of Texas Governorship 43rd President of the United States Presidency timeline Transition Inaugurations first second Policies Domestic Economic Foreign Bush Doctrine international trips Space Climate change Legislation and programs Pardons Appointments Cabinet Judiciary Roberts Miers Alito First term September 11 attacks War on terror...

American politician Anna Belle Clement O'Brien in August 2008. Anna Belle Clement O'Brien (May 6, 1923 – August 31, 2009) was a Tennessee politician, nicknamed the first lady of Tennessee politics.[1] She served as the governor's chief of staff from 1963 to 1967, was a member of the Tennessee House of Representatives in the 89th General Assembly, from 1975 to 1977, and a Tennessee State Senator in the 90th to 99th General Assemblies, from 1977 to 1996.[2] While she was not t...

Promozione 1971-72 Competizione Promozione Sport Calcio Edizione 5ª Organizzatore L.N.D.Comitati Regionali Luogo Italia Formula 26 gironi all'italiana Cronologia della competizione 1970-1971 1972-1973 Manuale Nella stagione 1971-1972, la Promozione era il quinto livello del calcio italiano (il primo livello regionale). Il campionato è strutturato in vari gironi all'italiana su base regionale, gestiti dai Comitati Regionali di competenza. Promozioni alla categoria superiore e retroces...

Maurois Harcourt nel 1936 André Maurois, pseudonimo di Émile Salomon Wilhelm Herzog (Elbeuf, 26 luglio 1885 – Neuilly-sur-Seine, 9 ottobre 1967), è stato un romanziere, saggista e storico francese. Fu eletto membro dell'Académie française il 23 giugno 1938, al seggio 26, successore di René Doumic. Indice 1 Biografia 2 Opere 3 Filmografia parziale 3.1 Sceneggiatore o autore del soggetto 4 Note 5 Altri progetti 6 Collegamenti esterni Biografia Tomba di André Maurois, al vecchio cimiter...
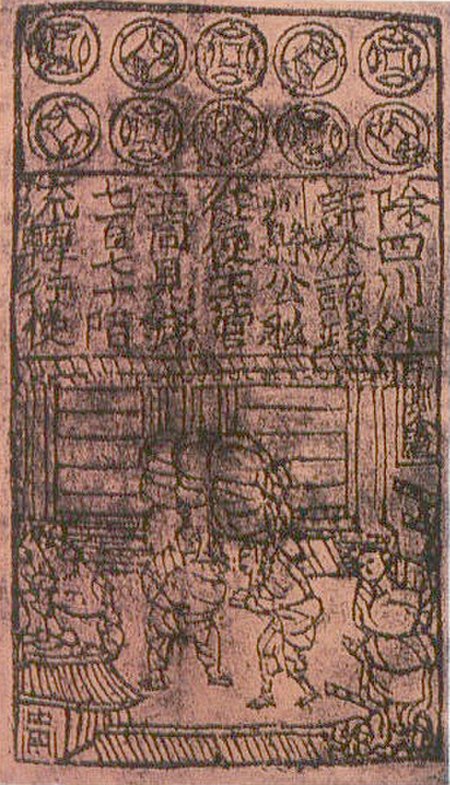
Currency not backed by any commodity Yuan dynasty banknotes are a medieval form of fiat money. Part of a series onEconomics History Outline Index Branches and classifications Applied Econometrics Heterodox International Micro / Macro Mainstream Mathematical Methodology Political JEL classification codes Concepts, theory and techniques Economic systems Economic growth Market National accounting Experimental economics Computational economics Game theory Operations research Middle income trap In...

جونجو شيلفي (بالإنجليزية: Jonjo Shelvey) معلومات شخصية الميلاد 27 فبراير 1992 (العمر 32 سنة)[1]رومفورد الطول 6 قدم 0 بوصة (1.84 م)[2][2] مركز اللعب وسط[3] الجنسية المملكة المتحدة معلومات النادي النادي الحالي نوتنجهام فورست الرقم 6 مسيرة الشباب سنوات فريق 200...















