Rétraction
|
Read other articles:

Artikel ini memiliki beberapa masalah. Tolong bantu memperbaikinya atau diskusikan masalah-masalah ini di halaman pembicaraannya. (Pelajari bagaimana dan kapan saat yang tepat untuk menghapus templat pesan ini) Netralitas artikel ini dipertanyakan. Diskusi terkait dapat dibaca pada the halaman pembicaraan. Jangan hapus pesan ini sampai kondisi untuk melakukannya terpenuhi. (Pelajari cara dan kapan saatnya untuk menghapus pesan templat ini) Artikel ini sudah memiliki referensi, tetapi tidak di...

WikifunctionsURLhttps://www.wikifunctions.org/ Eponimwiki dan fungsi TipeWikiensiklopediaPerdagangan ?TidakSubjek utamafungsi, Antarmuka pemrograman aplikasi dan Pemrograman fungsional SloganWikifunctions is a free library of functions that (soon) anyone can edit. Languebanyak bahasa LisensiCreative Commons Atribusi-BerbagiSerupa 4.0 Internasional, CC0 dan Apache License, Version 2.0 (en) Bagian dariAbstract Wikipedia (en) PemilikWikimedia FoundationPembuatDenny VrandečićWeb DeveloperW...

Erzurum Administration Pays Turquie Région Anatolie orientale Province Erzurum District Erzurum Maire Mandat Mehmet Sekmen (AKP) 2019-2024 Préfet Celalettin Güvenç2004 Indicatif téléphonique international +(90) Plaque minéralogique 25 Démographie Population 561 874 hab. Densité 23 hab./km2 Géographie Coordonnées 39° 54′ nord, 41° 16′ est Altitude 1 945 m Superficie 2 474 100 ha = 24 741 km2 Local...

United States admiral William H. Standley4th United States Ambassador to the Soviet Union In office14 April 1942 – 19 September 1943PresidentFranklin D. RooseveltPreceded byLaurence SteinhardtSucceeded byW. Averell Harriman6th Chief of Naval OperationsIn office1 July 1933 – 1 January 1937PresidentFranklin D. RooseveltPreceded byWilliam V. PrattSucceeded byWilliam D. Leahy Personal detailsBorn(1872-12-18)18 December 1872Ukiah, California, U.S.Died25 October 1963(1963-...

Public debate in Australia over British colonialism Not to be confused with the Canadian history wars. This article is part of a series on theHistory of Australia Timeline and periods Prehistory European exploration (sea) European exploration (land) 1788–1850 1851–1900 1901–1945 1945–present Topics Abortion Agriculture Antisemitism Banking Capital punishment Civil rights Cinema Constitution Diplomacy Economics Federation Immigration Labour LGBT Military Monarchy Sports Telecommunicati...

American college basketball season 2023–24 Tulane Green Wave men's basketballConferenceAmerican Athletic ConferenceRecord14–17 (5–13 AAC)Head coachRon Hunter (5th season)Associate head coachRay McCallumAssistant coaches Claude Pardue Sean Mock Darryl LaBarrie Home arenaDevlin FieldhouseSeasons← 2022–232024–25 → 2023–24 American Athletic Conference men's basketball standings vte Conf Overall Team W L PCT W L PCT South...

追晉陸軍二級上將趙家驤將軍个人资料出生1910年 大清河南省衛輝府汲縣逝世1958年8月23日(1958歲—08—23)(47—48歲) † 中華民國福建省金門縣国籍 中華民國政党 中國國民黨获奖 青天白日勳章(追贈)军事背景效忠 中華民國服役 國民革命軍 中華民國陸軍服役时间1924年-1958年军衔 二級上將 (追晉)部队四十七師指挥東北剿匪總司令部參謀長陸軍�...
ХристианствоБиблия Ветхий Завет Новый Завет Евангелие Десять заповедей Нагорная проповедь Апокрифы Бог, Троица Бог Отец Иисус Христос Святой Дух История христианства Апостолы Хронология христианства Раннее христианство Гностическое христианство Вселенские соборы Н...

Title in the Peerage of England Baronies of HastingsFirst creation (1290)Third creation (1461)(abeyant)Arms of Hastings (used as quartering by later barons), Barons Hastings: Or, a maunch gules [hr 1][1]Creation date1290 (first creation)(dormant 1389-1841, abeyant 1542-1841)1299 (second creation) 1461 (third creation) (abeyant 1868-71, 1960-) Created byEdward I (first creation)Edward I (second creation) Edward IV (third creation) PeeragePeerage of EnglandFirst holderJohn Hasti...

Early form of progressive rock Proto-progStylistic origins Rock progressive psychedelic rock[1] Cultural originsMid-1960sOther topics Progressive pop progressive rock post-progressive Proto-prog (short for proto-progressive[1]) is the earliest work associated with the first wave of progressive rock music,[2][3] known then as progressive pop.[4] Such musicians were influenced by modern classical and other genres usually outside of traditional rock influe...

Ця стаття потребує додаткових посилань на джерела для поліпшення її перевірності. Будь ласка, допоможіть удосконалити цю статтю, додавши посилання на надійні (авторитетні) джерела. Зверніться на сторінку обговорення за поясненнями та допоможіть виправити недоліки. Мат...

此条目序言章节没有充分总结全文内容要点。 (2019年3月21日)请考虑扩充序言,清晰概述条目所有重點。请在条目的讨论页讨论此问题。 哈萨克斯坦總統哈薩克總統旗現任Қасым-Жомарт Кемелұлы Тоқаев卡瑟姆若马尔特·托卡耶夫自2019年3月20日在任任期7年首任努尔苏丹·纳扎尔巴耶夫设立1990年4月24日(哈薩克蘇維埃社會主義共和國總統) 哈萨克斯坦 哈萨克斯坦政府...

Aviva MongilloLahirAviva Chiara Mongillo6 Februari 1998 (umur 26)Markham, Ontario, KanadaPendidikanSekolah Menengah Atas UnionvillePekerjaanPemeransekarangTahun aktif2016–sekarangTelevisiBackstage Workin' MomsKarier musikNama lainCarysGenrePopInstrumenVokalLabelWarner Music Canada Aviva Chiara Mongillo (lahir 6 Februari 1998), juga dikenal sebagai Carys, adalah pemeran dan penyanyi asal Kanada. Ia paling dikenal untuk perannya sebagai Alya Kendrick dalam drama Family Channel Back...

Waterway connecting the straits of Juan de Fuca and Georgia in northwest Washington, USA 48°30′N 122°45′W / 48.5°N 122.75°W / 48.5; -122.75 Rosario Strait connects the Strait of Juan de Fuca and the Strait of Georgia, east of the main San Juan Islands. This map follows the USGS definition of the strait. Major islands are named. Rosario Strait is a strait in northern Washington state, separating San Juan County and Skagit and Whatcom Counties. It extends from th...
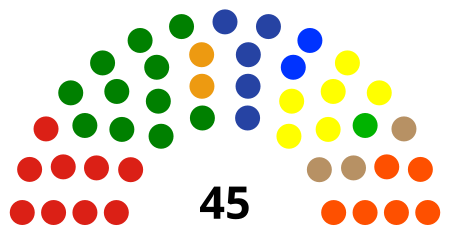
Dewan Perwakilan Rakyat Daerah Kabupaten TrenggalekDewan Perwakilan RakyatKabupaten Trenggalek2019-2024JenisJenisUnikameral Jangka waktu5 tahunSejarahSesi baru dimulai26 Agustus 2019PimpinanKetuaH. Samsul Anam, S.H., M.M., M.Hum. (PKB) sejak 28 September 2019 Wakil Ketua IDoding Rahmadi, S.T. (PDI-P) sejak 28 September 2019 Wakil Ketua IIHj. Arik Sri Wahyuni, S.E., M.M. (Golkar) sejak 28 September 2019 Wakil Ketua IIIAgus Cahyono, S.H.I., M.H.I. (PKS) sejak 28 September 2019 K...

Boat which is powered by an engine Motorboating redirects here. For other uses, see Motorboating (disambiguation). Speedboat redirects here. For the novel, see Speedboat (novel). Not to be confused with Motor ship. This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Motorboat – news · newspapers · books · scholar ...

Public park in the Bronx, New York Bridge ParkSouthern end of the parkTypeUrban parkLocationMorris Heights and Highbridge, Bronx, New York CityArea7.16 acres (2.90 ha)Opened2015Operated byNYC Parks Bridge Park is a park in the Bronx, New York, created as part of a larger vision of creating connected waterfront parks along both sides of the Harlem River. The park's name references three large arch bridges linking Manhattan and the Bronx: Alexander Hamilton Bridge, Washington Bridge, ...

Overview of music traditions in the U.S. state of Illinois This article relies largely or entirely on a single source. Relevant discussion may be found on the talk page. Please help improve this article by introducing citations to additional sources.Find sources: Music of Illinois – news · newspapers · books · scholar · JSTOR (June 2014) Music of the United States AK AL AR AS AZ CA CO CT DC DE FL GA GU HI IA ID IL IN KS KY LA MA MD ME MI MN MO MP MS MT...

Disambiguazione – Se stai cercando altri significati, vedi Bosco (disambigua). «Troverai più nei boschi che nei libri. Gli alberi e le rocce ti insegneranno cose che nessun maestro ti dirà.» (Bernardo di Chiaravalle, Epistola 106 n.2) Pineta di pini marittimi Il bosco (o selva) è un'area di superficie terrestre con estensione minima di 0,2 ettari, formata da un'associazione vegetale di alberi selvatici di alto fusto e di arbusti ed erbe che costituiscono il sottobosco.[1] Quan...

سفارة دولة فلسطين لدى إيطاليا فلسطين إيطاليا الإحداثيات 41°52′57″N 12°29′25″E / 41.8824°N 12.4902°E / 41.8824; 12.4902 البلد إيطاليا المكان روما الاختصاص إيطاليا السَفير عبير عودة الموقع الالكتروني الموقع الرسمي تعديل مصدري - تعديل سفارة دولة فلسطين لدى إيطالي�...
![{\displaystyle F:X\times [0,1]\to X}](https://wikimedia.org/api/rest_v1/media/math/render/svg/00930f3e4f9e733d5819981419fb759b0faa3cea)

![{\displaystyle \forall t\in [0,1],\forall a\in A,~F(a,t)=a.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b013081826210ab79d82252ae055d95161af1849)