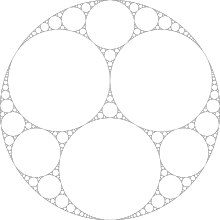
Kleinian group
|
Read other articles:

Si ce bandeau n'est plus pertinent, retirez-le. Cliquez ici pour en savoir plus. Cet article ne cite pas suffisamment ses sources (août 2013). Si vous disposez d'ouvrages ou d'articles de référence ou si vous connaissez des sites web de qualité traitant du thème abordé ici, merci de compléter l'article en donnant les références utiles à sa vérifiabilité et en les liant à la section « Notes et références » En pratique : Quelles sources sont attendues ? Comm...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Januari 2023. MetaMask adalah sebuah dompet mata uang kripto yang digunakan untuk berinteraksi dengan rantai blok Ethereum. MetaMask memungkinkan penggunanya untuk mengakses dompet Ethereum melalui ekstensi peramban ataupun melalui aplikasi seluler sehingga dapat be...

Austrian astronomer and mathematician (1514–1576) RheticusBorn16 February 1514Feldkirch, Archduchy of Austria, Holy Roman Empire(present-day Austria)Died4 December 1574(1574-12-04) (aged 60)Kassa, Kingdom of Hungary, Habsburg monarchy(present-day Slovakia)Alma materUniversity of WittenbergKnown forTrigonometric tables[2]Scientific careerFieldsMathematician and astronomerInstitutionsUniversity of Wittenberg (1536–42)University of Leipzig (1542–51)[1]Academi...

العلاقات البليزية الطاجيكستانية بليز طاجيكستان بليز طاجيكستان تعديل مصدري - تعديل العلاقات البليزية الطاجيكستانية هي العلاقات الثنائية التي تجمع بين بليز وطاجيكستان.[1][2][3][4][5] مقارنة بين البلدين هذه مقارنة عامة ومرجعية للدولتين: وج�...

مسجد السيد عزيز الله إحداثيات 35°40′31″N 51°25′20″E / 35.675138888889°N 51.422111111111°E / 35.675138888889; 51.422111111111 معلومات عامة القرية أو المدينة طهران، محافظة طهران الدولة إيران تاريخ الافتتاح الرسمي القرن 19[1] أبعاد المبنى التفاصيل التقنية المواد المستخدمة طابوق&#...

1861 battle in the American Civil War Battle of PlacitoPart of the American Civil War, Apache WarsArizona militia during the Civil War.DateSeptember 8, 1861LocationPlacitas, New Mexico Territory (USA), Arizona Territory (CSA);now in Lincoln County, New MexicoResult Confederate victoryBelligerents Confederate States ApacheCommanders and leaders John Pulliam UnknownStrength ~20 UnknownCasualties and losses Unknown 5 killed vteApache Wars Jicarilla War Point of Rocks Wagon Mound Bell's Fig...

2009 American filmOutrageDirected byKirby DickWritten byKirby DickProduced byAmy Ziering[1]CinematographyThaddeus Wadleigh[1]Edited byDouglas BlushMatthew Clarke[1]Music byPeter Golub[1]ProductioncompanyChain Camera PicturesDistributed byMagnolia PicturesRelease dates April 24, 2009 (2009-04-24) (Tribeca Film Festival) May 8, 2009 (2009-05-08) (United States) Running time89 minutesCountryUnited StatesLanguageEnglishBox offi...

Neighbourhood of Manama, Bahrain Neighborhood in Capital Governorate, BahrainSalmaniyaNeighborhoodCountryBahrainGovernorateCapital Governorate Salmaniya is a district located in the city of Manama, Bahrain. Healthcare A road intersection in Salmaniya, flooded in 2024. The largest public hospital in Bahrain, Salmaniya Medical Complex, and the country's only public Psychiatric Hospital (Bahrain). Other private hospitals operate within the vicinity such as the Royal Bahrain Hospital[1]. History ...

Italian film producer (1912–2007) For other uses, see Carlo Ponti (disambiguation). This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Carlo Ponti – news · newspapers · books · scholar · JSTOR (September 2023) (Learn how and when to remove this message) Carlo PontiOMRIPonti in 1951BornCarlo Fortunato Pietro P...

This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) The topic of this article may not meet Wikipedia's general notability guideline. Please help to demonstrate the notability of the topic by citing reliable secondary sources that are independent of the topic and provide significant coverage of it beyond a mere trivial mention. If notability cannot be shown, the article is likely to be merged,...

Vous lisez un « bon article » labellisé en 2021. L'Affaire Tournesol 18e album de la série Les Aventures de Tintin Haut de couverture de l'album L'Affaire Tournesol Auteur Hergé Genre(s) Bande dessinée franco-belgeAventure Personnages principaux TintinMilouCapitaine HaddockProfesseur TournesolBianca Castafiore Lieu de l’action Belgique Suisse France Bordurie Syldavie Langue originale Français Éditeur Casterman Première publication 1956 Nombre de pages 62 Pr...

Teatro Municipale Giuseppe VerdiLa facciata del Teatro con l'ingressoUbicazioneStato Italia LocalitàSalerno IndirizzoPiazza Matteo Luciani Dati tecniciTipoteatro all'italiana su pianta mistilinea (ovale raccordato al boccascena mediante due ali rette) con 71 palchi divisi in quattro ordini ed una galleria Fossapresente Capienzacirca 610 posti RealizzazioneCostruzione1863 Inaugurazione1872 ArchitettoAntonio D’AmoraGiuseppe Menichini Sito ufficiale Modifica dati su Wikidata · Manu...

UFC flagship event in 2015 UFC 192: Cormier vs. GustafssonThe poster for UFC 192: Cormier vs. GustafssonInformationPromotionUltimate Fighting ChampionshipDateOctober 3, 2015 (2015-10-03)VenueToyota CenterCityHouston, TexasAttendance14,622[1]Total gate$1,859,000[1]Buyrate250,000[2]Event chronology UFC Fight Night: Barnett vs. Nelson UFC 192: Cormier vs. Gustafsson UFC Fight Night: Holohan vs. Smolka UFC 192: Cormier vs. Gustafsson was a mixed martial arts...

Digital content of people who passed away A recent extension to the cultural relationship with death is the increasing number of people who die having created a large amount of digital content, such as social media profiles, that will remain after death. This may result in concern and confusion, because of automated features of dormant accounts (e.g. birthday reminders), uncertainty of the deceased's preferences that profiles be deleted or left as a memorial, and whether information that may ...

Le vorticisme est un mouvement artistique britannique du début du XXe siècle, s'épanouissant entre 1913 et 1915 et regroupant des plasticiens, des poètes et des théoriciens. Il est considéré comme le seul mouvement britannique significatif de cette période, et s'inscrit pour partie dans le prolongement du cubisme. Le terme « vortex » fait allusion aux théories de Umberto Boccioni, affirmant que l'art trouve sa source dans le tourbillon (du latin vortex) des émotions...

Not to be confused with Tennessean or Tennessee. E117 and Uus redirect here. For the E-road, see European route E117. For other uses, see UUS (disambiguation). Chemical element, symbol Ts and atomic number 117Tennessine, 117TsTennessinePronunciation/ˈtɛnəsiːn/[1] (TEN-ə-seen)Appearancesemimetallic (predicted)[2]Mass number[294]Tennessine in the periodic table Hydrogen Helium Lithium Beryllium Boron Carbon Nitrogen Oxygen Fluorine Neon Sodium Magnesium Alu...

American politician and judge George L. YapleMember of the U.S. House of Representativesfrom Michigan's 4th districtIn officeMarch 4, 1883 – March 3, 1885Preceded byJulius C. BurrowsSucceeded byJulius C. Burrows Personal detailsBorn(1851-02-20)February 20, 1851Leonidas, MichiganDiedDecember 16, 1939(1939-12-16) (aged 88)Mendon, MichiganResting placeMendon CemeteryPolitical partyDemocraticEducationNorthwestern University George Lewis Yaple (February 20, 1851 – Dec...

American chemist Stephen LippardLippard in 2017BornStephen James Lippard (1940-10-12) October 12, 1940 (age 83)[1]Pittsburgh, Pennsylvania, U.S.NationalityAmericanAlma materHaverford College (B.S.) (1962)Massachusetts Institute of Technology (Ph.D) (1965)AwardsWilliam H. Nichols Medal (1995)National Medal of Science (2004)Linus Pauling Award (2009)Priestley Medal (2014)Welch Award in Chemistry (2016)American Institute of Chemists Gold Medal (2017)Scientific careerFields Inor...

Historic house in Kansas, United States United States historic placeHenry J. Allen HouseU.S. National Register of Historic Places The house in late 2013Show map of KansasShow map of the United StatesLocation255 N. Roosevelt St.,Wichita, KansasCoordinates37°41′24″N 97°17′33″W / 37.69000°N 97.29250°W / 37.69000; -97.29250Area1 acre (0.40 ha)Built1917ArchitectFrank Lloyd WrightArchitectural stylePrairie SchoolNRHP reference No.73000775[...

Derby della CapitaleLokasiKota atau daerahRoma, ItaliaPertemuan pertama8 Desember 1929Tim terlibatA.S. RomaS.S. LazioData dan rekor Derby della Capitale (Indonesia: Derby ibu kota), juga dikenal sebagai Derby Capitolino dan Derby del Cupolone, adalah sebuah derby sepak bola di Roma, Italia, antara dua klub besar kota tersebut, A.S. Roma dan S.S. Lazio. Pranala luar Wikimedia Commons memiliki media mengenai Derby di Roma. Five classic Rome derbies over the past fifteen years di Goal.com Il Der...