Paley–Wiener integral

In mathematics, the Paley–Wiener integral is a simple stochastic integral. When applied to classical Wiener space, it is less general than the Itō integral, but the two agree when they are both defined.

The integral is named after its discoverers, Raymond Paley and Norbert Wiener.

Definition

Let $i:H\to E$ be an abstract Wiener space with abstract Wiener measure $\gamma$ on $E$ . Let $j:E^{*}\to H$ be the adjoint of $i$ . (We have abused notation slightly: strictly speaking, $j:E^{*}\to H^{*}$ , but since $H$ is a Hilbert space, it is isometrically isomorphic to its dual space $H^{*}$ , by the Riesz representation theorem.)

It can be shown that $j$ is an injective function and has dense image in $H$ .^{[citation needed]} Furthermore, it can be shown that every linear functional $f\in E^{*}$ is also square-integrable: in fact,

\|f\|_{L^{2}(E,\gamma ;\mathbb {R} )}=\|j(f)\|_{H}

This defines a natural linear map from $j(E^{*})$ to $L^{2}(E,\gamma ;\mathbb {R} )$ , under which $j(f)\in j(E^{*})\subseteq H$ goes to the equivalence class $[f]$ of $f$ in $L^{2}(E,\gamma ;\mathbb {R} )$ . This is well-defined since $j$ is injective. This map is an isometry, so it is continuous.

However, since a continuous linear map between Banach spaces such as $H$ and $L^{2}(E,\gamma ;\mathbb {R} )$ is uniquely determined by its values on any dense subspace of its domain, there is a unique continuous linear extension $I:H\to L^{2}(E,\gamma ;\mathbb {R} )$ of the above natural map $j(E^{*})\to L^{2}(E,\gamma ;\mathbb {R} )$ to the whole of $H$ .

This isometry $I:H\to L^{2}(E,\gamma ;\mathbb {R} )$ is known as the Paley–Wiener map. $I(h)$ , also denoted $\langle h,x\rangle ^{\sim }$ , is a function on $E$ and is known as the Paley–Wiener integral (with respect to $h\in H$ ).

It is important to note that the Paley–Wiener integral for a particular element $h\in H$ is a function on $E$ . The notation $\langle h,x\rangle ^{\sim }$ does not really denote an inner product (since $h$ and $x$ belong to two different spaces), but is a convenient abuse of notation in view of the Cameron–Martin theorem. For this reason, many authors^{[citation needed]} prefer to write $\langle h,-\rangle ^{\sim }(x)$ or $I(h)(x)$ rather than using the more compact but potentially confusing $\langle h,x\rangle ^{\sim }$ notation.

References

Park, Chull; Skoug, David (1988), "A Note on Paley-Wiener-Zygmund Stochastic Integrals", Proceedings of the American Mathematical Society, 103 (2): 591–601, doi:10.1090/S0002-9939-1988-0943089-8, JSTOR 2047184
Elworthy, David (2008), MA482 Stochastic Analysis (PDF), Lecture Notes, University of Warwick (Section 6)

v t e Analysis in topological vector spaces
Basic concepts	Abstract Wiener space Classical Wiener space Bochner space Convex series Cylinder set measure Infinite-dimensional vector function Matrix calculus Vector calculus
Derivatives	Differentiable vector–valued functions from Euclidean space Differentiation in Fréchet spaces Fréchet derivative Total Functional derivative Gateaux derivative Directional Generalizations of the derivative Hadamard derivative Holomorphic Quasi-derivative
Measurability	Besov measure Cylinder set measure Canonical Gaussian Classical Wiener measure Measure like set functions infinite-dimensional Gaussian measure Projection-valued Vector Bochner / Weakly / Strongly measurable function Radonifying function
Integrals	Bochner Direct integral Dunford Gelfand–Pettis/Weak Regulated Paley–Wiener
Results	Cameron–Martin theorem Inverse function theorem Nash–Moser theorem Feldman–Hájek theorem No infinite-dimensional Lebesgue measure Sazonov's theorem Structure theorem for Gaussian measures
Related	Crinkled arc Covariance operator
Functional calculus	Borel functional calculus Continuous functional calculus Holomorphic functional calculus
Applications	Banach manifold (bundle) Convenient vector space Choquet theory Fréchet manifold Hilbert manifold

Functional analysis (topics – glossary)

Spaces

Banach Besov Fréchet Hilbert Hölder Nuclear Orlicz Schwartz Sobolev Topological vector
Properties	Barrelled Complete Dual (Algebraic / Topological) Locally convex Reflexive Separable

Theorems

Operators

Algebras

Open problems

Applications

Advanced topics

Category

Read other articles:

The Democrats (Gabon)

The Democrats Les DémocratesAbbreviationLDLeaderGuy Nzouba NdamaFounded2017HeadquartersLibrevilleNational affiliationCoalition for the New RepublicSloganUnité, Justice, ProgrèsNational Assembly10 / 143Senate0 / 102Politics of GabonPolitical partiesElections Political party in Gabon This article relies largely or entirely on a single source. Relevant discussion may be found on the talk page. Please help improve this article by introducing citations to additional sources.Find sources: T...

Ilan Halevi

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada November 2022. Ilan HaleviLahirGeorges Alain Albert(1943-10-12)12 Oktober 1943Lyon, PrancisMeninggal10 Juli 2013(2013-07-10) (umur 69)Clichy, Hauts-de-Seine, PrancisKebangsaanPrancis,Israel (sejak 1965)Nama lainAlain Albert; Alan Albert; Georges Levin[...

John Milton Glover

American politician Not to be confused with John Montgomery Glover. John Milton GloverSt. Louis Globe-Democrat, February 22, 1887Member of the United States House of RepresentativesIn officeMarch 4, 1885 – March 3, 1889Preceded byJames BroadheadSucceeded byNathan FrankConstituencyMissouri's 9th congressional district Personal detailsBorn(1852-06-23)June 23, 1852St. Louis, Missouri, U.S.DiedOctober 20, 1929(1929-10-20) (aged 77)Pueblo, Colorado, U.S.Resting placeBellefontaine C...

Jean-Louis Touraine

Pour l’article ayant un titre homophone, voir Jean-Louis Tourenne. Pour les articles homonymes, voir Touraine (homonymie). Jean-Louis Touraine Jean-Louis Touraine en 2017. Fonctions Député français 20 juin 2007 – 21 juin 2022(15 ans et 1 jour) Élection 17 juin 2007 Réélection 17 juin 201218 juin 2017 Circonscription 3e du Rhône Législature XIIIe, XIVe et XVe (Cinquième République) Groupe politique SRC (2007-2016)SER (2016-2017)LREM (2017-2022) Prédécesseur Jean-Mich...

Goniaea furcifera

Species of grasshopper Goniaea furcifera Scientific classification Domain: Eukaryota Kingdom: Animalia Phylum: Arthropoda Class: Insecta Order: Orthoptera Suborder: Caelifera Family: Acrididae Genus: Goniaea Species: G. furcifera Binomial name Goniaea furciferaWalker, 1870 Goniaea furcifera is a species of grasshopper in the family Acrididae. It was described by Francis Walker in 1870.[1] Synonyms The following are synonyms for this species:[1] Goniaea minipes Sjöstedt, ...

Peter Burt

Scottish businessman Sir Peter Alexander Burt FRSE (6 March 1944 – 28 November 2017)[1] was a Scottish businessman, former chief executive and later governor of the Bank of Scotland. Early life The grave of Sir Peter Burt, Whitekirk graveyard Burt was born in 1944 in East Africa, and educated in Scotland. He graduated from the University of St Andrews and the University of Pennsylvania's Wharton School and later worked in the computer industry in California and Scotland.[2] ...

Animals (Martin Garrix song)

2013 song by Martin Garrix AnimalsSingle by Martin Garrixfrom the EP Gold Skies Released17 June 2013 (2013-06-17)Recorded2013GenreBig room house[1]Length 5:04 (original mix) 2:56 (US radio edit) 2:45 (UK radio edit) Label Spinnin' Stmpd (2023 reissue)[2] Songwriter(s) Martijn Garritsen [3]Producer(s) Martin Garrix Martin Garrix singles chronology Just Some Loops (2013) Animals (2013) Wizard (2013) Alternative UK and US cover Music videoAnimals on YouTube...

Alexander O. Smith

American translator and author Alexander O. SmithEducationBachelor of Arts, Dartmouth College/Keio University, 1995Master of Arts, Harvard University, 1998Occupation(s)Translator, authorYears active1997–presentNotable workVagrant Story, Phoenix Wright: Ace Attorney, Final Fantasy XII Alexander O. Smith is a professional Japanese to English translator and author. While his output covers many areas such as adaptation of Japanese novels, manga, song lyrics, anime scripts, and various acad...

Operation Panther (2013)

Operation PantherPart of Northern Mali conflict and Operation ServalAdrar des IfoghasDate19 February – 25 March 2013(1 month and 6 days)LocationAdrar des Ifoghas, MaliResult French VictoryBelligerents France Chad AQIM Ansar DineCommanders and leaders Bernard Barrera Mahamat Idriss Déby Itno Abdelhamid Abou Zeid †Strength 1,200800 troops 400-500 terroristsCasualties and losses 3 killed120 wounded1 armoured vehicle destroyed27 killed67-71 wounded 200-250 kil...

2016年美國總統選舉

2016年美國總統選舉 ← 2012 2016年11月8日 2020 → 538個選舉人團席位獲勝需270票民意調查投票率55.7%[1][2] ▲ 0.8 % 获提名人唐納·川普希拉莉·克林頓政党共和黨民主党家鄉州紐約州紐約州竞选搭档迈克·彭斯蒂姆·凱恩选举人票 304[3][4][註 1] 227[5] 胜出州/省 30 + 緬-2 20 + DC 民選得票 62,984,828[6] 65,853,514[6]...

美国众议院

此條目需要补充更多来源。 (2021年7月4日)请协助補充多方面可靠来源以改善这篇条目，无法查证的内容可能會因為异议提出而被移除。致使用者：请搜索一下条目的标题（来源搜索：美国众议院 — 网页、新闻、书籍、学术、图像），以检查网络上是否存在该主题的更多可靠来源（判定指引）。美國眾議院 United States House of Representatives第118届美国国会众议院徽章众议院旗...

Karl Theodor Anton Maria von Dalberg

German archbishop of Mainz, later of Regensburg (1744–1817) This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Karl Theodor Anton Maria von Dalberg – news · newspapers · books · scholar · JSTOR (December 2018) (Learn how and when to remove this message) Karl Theodor Anton Maria von DalbergElector of MainzArch...

普通高等学校招生全国统一考试

关于“高考”的其他用法，请见「高考」。此條目需要补充更多来源。 (2022年6月7日)请协助補充多方面可靠来源以改善这篇条目，无法查证的内容可能會因為异议提出而被移除。致使用者：请搜索一下条目的标题（来源搜索：普通高等学校招生全国统一考试 — 网页、新闻、书籍、学术、图像），以检查网络上是否存在该主题的更多可靠来源（判定指引）。 2022�...

Abortion in Pennsylvania

Abortion in Pennsylvania is legal up to the 24th week of pregnancy. 51% of Pennsylvania adults said in a 2014 poll by the Pew Research Center that abortion should be legal and 44% said it should be illegal in all or most cases.[1] The number of abortion clinics in Pennsylvania has declined over the years, with 114 in 1982, 81 in 1992 and twenty in 2014. There were 32,126 legal abortions in 2014, and 31,818 in 2015. History Legislative history By the end of the 1800s, all states i...

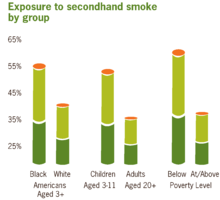
Passive smoking

Inhalation of tobacco smoke by persons other than the intended active smoker Second hand smoke redirects here. For the Sublime album, see Second-hand Smoke. Tobacco smoke in an Irish pub before a smoking ban came into effect on March 29, 2004 Passive smoking is the inhalation of tobacco smoke, called passive smoke, secondhand smoke (SHS) or environmental tobacco smoke (ETS), by individuals other than the active smoker. It occurs when tobacco smoke diffuses into the surrounding atmosphere as a...

Souvenir plot

Term for an online purchasable novelty item, does not entitle its purchaser to ownership See also: Laird and False titles of nobility Part of a series onScots law Administration Justice and Communities Directorate of the Scottish Government Cabinet Secretary for Justice Judicial Appointments Board Judicial Complaints Reviewer Parole Board for Scotland Legal Aid Board Courts & Tribunals Service College of Justice Office of the Public Guardian Scottish Sentencing Council Law Commission Crim...

2008 West Oxfordshire District Council election

2008 UK local government election Map of the results of the 2008 West Oxfordshire District Council election. Conservatives in blue and Liberal Democrats in yellow. Wards in dark grey were not contested in 2008. The 2008 West Oxfordshire District Council election took place on 1 May 2008 to elect members of West Oxfordshire District Council in Oxfordshire, England. One third of the council was up for election and the Conservative Party stayed in overall control of the council.[1] After...

3rd/4th Cavalry Regiment (Australia)

Regiment of the Australian Army 3rd/4th Cavalry RegimentCap badge of 3rd/4th Cavalry RegimentActive1981–20142017–PresentCountryAustraliaBranchArmyTypeTraining supportRolePersonnel, platform & logistic supportSizeOne squadronPart ofSchool of ArmourGarrison/HQPuckapunyalNickname(s)StingersMotto(s)Resolute/TenaciousMarchOld Comrades/Light CavalryEngagementsVietnam WarSomaliaRwandaEast TimorIraq WarWar in AfghanistanDecorationsUnit Citation for Gallantry (3CAV, ASQN)CommandersColonel...

Grand Prix F1 Hungaria 2022

Grand Prix Hungaria 2022 Lomba ke-13 dari 22 dalam Formula Satu musim 2022← Lomba sebelumnyaLomba berikutnya → Detail perlombaanTanggal 31 Juli 2022 (2022-07-31)Nama resmi Formula 1 Aramco Magyar Nagydíj 2022Lokasi Hungaroring Mogyoród, HungariaSirkuit Fasilitas balap permanenPanjang sirkuit 4.381 km (2.722 mi)Jarak tempuh 70 putaran, 306.630 km (190.531 mi)Rencana jarak tempuh 70 putaran, 306.630 km (190.531 mi)Cuaca Berawan dengan hujan ringanPosisi polePembal...

City of Greater Dandenong

For other uses of the name Dandenong, see Dandenong (disambiguation). Local government area in Victoria, AustraliaCity of Greater DandenongVictoriaThe City of Greater Dandenong within metropolitan MelbourneCoordinates38°01′0″S 145°20′38″E / 38.01667°S 145.34389°E / -38.01667; 145.34389Population166,094 (2018)[1] (43rd) • Density1,280/km2 (3,310/sq mi)Established1994Area130 km2 (50.2 sq mi)[1]MayorEden Fos...