Hilbert–Schmidt operator
|
Read other articles:

Peta menunjukkan lokasi Datu Saudi-Ampatuan Data sensus penduduk diDatu Saudi-Ampatuan Tahun Populasi Persentase 199523.640—200027.5093.31%200745.1267.07% Datu Saudi-Ampatuan adalah munisipalitas yang terletak di provinsi Maguindanao, Filipina. Pada tahun 2010, munisipalitas ini memiliki populasi sebesar 45.126 jiwa atau 5.134 rumah tangga. Pembagian wilayah Secara administratif Datu Saudi-Ampatuan terbagi menjadi 8 barangay, yaitu:[1] Dapiawan Elian Gawang Kabengi Kitango Kitapok M...

Chevrolet MalibuInformasiProdusenGeneral MotorsModel untuk tahun1964–19831997–sekarangBodi & rangkaKelasMedium Chevrolet Malibu (dinamai dari daerah Malibu, California) adalah mobil ukuran medium yang diproduksi oleh General Motors dengan merek Chevrolet. Pada awalnya Malibu merupakan salah satu trim Chevrolet Chevelle, tetapi kemudian mobil ini mendapat namanya sendiri mulai tahun 1978. Pada awalnya, mobil ini berpenggerak roda belakang, tetapi setelah kelahirannya kembali pada ...

Gul PanagPanag pada 2017Lahir3 Januari 1979 (umur 45)[1]Chandigarh, India[2]Nama lainGul Panag-Attari, Gulkirat Kaur PanagPekerjaanPemeran, pemeran suara, model, politisiTahun aktif2000–sekarangTinggi5 ft 6 in (1,68 m)Partai politikAam Aadmi PartySuami/istriRishi Attari (13 March 2011-)Situs webhttp://www.gulpanag.net Gul Panag (lahir Gulkirat Kaur Panag, 3 Januari 1979;[1] Chandigarh, India) adalah seorang pemeran, pengisi suara, model...

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (يوليو 2020) عاصفة أكتوبرOctober Gale (بالإنجليزية) ملصق فيلممعلومات عامةالصنف الفني فيلم إثارة تاريخ الصدور 2014 اللغة الأصلية الإنجليزية البلد كندا موقع التصوير أونتاريو ...

Indonesian cardinal His EminenceJulius Riyadi DarmaatmadjaSJCardinal, Archbishop Emeritus of JakartaChurchCathedral of the Assumption in JakartaArchdioceseJakartaProvinceJakartaMetropolisJakartaSeeJakartaAppointed11 January 1996Term ended28 June 2010PredecessorLeo SoekotoSuccessorIgnatius Suharyo HardjoatmodjoOther post(s)Cardinal-Priest of S. Cuore di MariaOrdersOrdination18 December 1969by Justinus Cardinal DarmojuwonoConsecration29 June 1983by Justinus Cardinal DarmojuwonoCreated...

2011 video game 2011 video gameForza Motorsport 4North American cover art featuring a Ferrari 458Developer(s)Turn 10 StudiosPublisher(s)Microsoft StudiosDirector(s)Dan GreenawaltProducer(s)Drew AngeloffDesigner(s)Scott CatlinWilliam GieseProgrammer(s)Daniel AdentChris TectorArtist(s)Rory ReichDerrick AynagaJon KnolesComposer(s)Lance HayesSeriesForzaPlatform(s)Xbox 360ReleaseNA: October 11, 2011AU: October 13, 2011EU: October 14, 2011Genre(s)RacingMode(s)Single-player, multiplayer Forza Motors...

Da RomanoStato Marca Trevigiana Marca di Verona Titoli Margravio della Marca Trevigiana Margravio della Marca di Verona Signore di: Angarano, Bassano, Brescia, Cartigliano, Conegliano, Godego, Onara, Padova, Parma, Romano, San Zenone, Trento, Treviso, Verona ed altri feudi Podestà di: Verona, Vicenza, Treviso Fondatore Arpone (leggendario) Ecelo (primo membro attestato) Ultimo sovranoAlberico da Romano[N 1] Data di fondazioneXI secolo Data di estinzione1260[N 2] Man...

Questa voce o sezione sull'argomento competizioni calcistiche non è ancora formattata secondo gli standard. Commento: Ogni utente deve seguire gli standard stabiliti dal Progetto Calcio, vedere il modello di voce ed applicarlo su tutta la pagina. I calendari, in particolare, possono essere difformi perché all'epoca non sempre erano disputate le partite di ritorno Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti del progetto di riferimento. Terza Cat...

Canadian $1 coin This article is about the current Canadian one-dollar coin. For the old dollar coin struck from 1935 to 1986, see Voyageur dollar. For the Canadian dollar as a currency, see Canadian dollar. For other uses, see Looney (disambiguation). This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article needs to be updated. Please help update this article to reflect recent events...

New Europe First editionAuthorMichael PalinCountryUnited KingdomLanguageEnglishSeriesMichael Palin's TripsGenreTravel literaturePublisherWeidenfeld & NicolsonPublication date2007Media typePrint (HardbackPages288ISBN978-0-297-84449-5OCLC141379962Preceded byHimalaya Followed byBrazil New Europe is the book that Michael Palin wrote to accompany the BBC television documentary series Michael Palin's New Europe. This description is that of the Hard Cover (Pictorial).I...

Pour les articles homonymes, voir Adams. Julie Adams Julie Adams en 1953. Données clés Nom de naissance Betty May Adams Surnom Betty Adams, Julia Adams, Julie Adams Naissance 17 octobre 1926Waterloo, Iowa(États-Unis) Nationalité Américaine Décès 3 février 2019 (à 92 ans)Los Angeles Profession Actrice Films notables L'Étrange Créature du lac noir modifier Julie Adams (nom de scène de Betty May Adams[1]) est une actrice américaine née le 17 octobre 1926 à Waterloo dans l'Io...
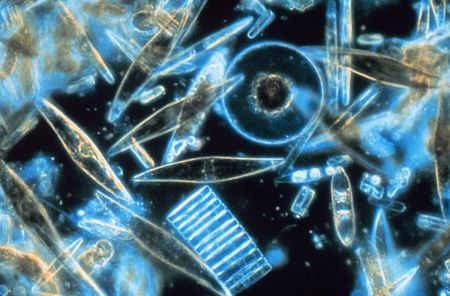
Paralytic shellfish toxin Saxitoxin Names IUPAC name [(3aS,4R,10aS)-10,10-dihydroxy-2,6-diiminooctahydro-1H,8H-pyrrolo[1,2-c]purin-4-yl]methyl carbamate Identifiers CAS Number 35523-89-8 Y 3D model (JSmol) Interactive image ChEBI CHEBI:34970 N ChEMBL ChEMBL501134 N ChemSpider 34106 N ECHA InfoCard 100.160.395 IUPHAR/BPS 2625 KEGG C13757 Y PubChem CID 37165 UNII Q0638E899B Y CompTox Dashboard (EPA) DTXSID3074313 InChI InChI=1S/C10H17N7O4/c11-6-15-5-4(3-21-8(13)18)...

On September 9, 1998, the Starr Report was released. The Starr Report, officially the Referral from Independent Counsel Kenneth W. Starr in Conformity with the Requirement of Title 28, United States Code, Section 595(c), is a United States federal government report by Independent Counsel Ken Starr concerning his investigation of President Bill Clinton. Delivered to the United States Congress on September 9, 1998, the allegations in the report led to the impeachment of Bill Clinton and the fiv...

Cet article est une ébauche concernant Saint-Paul de La Réunion. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Ravine Daniel Administration Pays France Région La Réunion Département La Réunion Commune Saint-Paul Géographie Coordonnées 21° 05′ 19,5″ sud, 55° 18′ 22,5″ est Localisation Géolocalisation sur la carte : La Réunion Ravine Daniel Géolocalisation ...

この項目には、一部のコンピュータや閲覧ソフトで表示できない文字が含まれています(詳細)。 数字の大字(だいじ)は、漢数字の一種。通常用いる単純な字形の漢数字(小字)の代わりに同じ音の別の漢字を用いるものである。 概要 壱万円日本銀行券(「壱」が大字) 弐千円日本銀行券(「弐」が大字) 漢数字には「一」「二」「三」と続く小字と、「壱」「�...

Le Labyrinthe De Pan Logo du titre du film Données clés Titre québécois Le Labyrinthe De Pan Titre original El Laberinto Del Fauno Réalisation Guillermo del Toro Scénario Guillermo del Toro Musique Javier Navarrete Acteurs principaux Ivana BaqueroDoug JonesSergi LópezAriadna GilMaribel Verdú Pays de production Espagne Mexique[1] Genre Fantaisie SombreDrameHorreurHistoriqueAventureThrillerGuerre Durée 118 minutes Sortie 2006 Pour plus de détails, voir Fiche technique et Distribution...

Questa voce sull'argomento calciatori seychellesi è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti del progetto di riferimento. Dine Suzette Nazionalità Seychelles Calcio Ruolo Attaccante Squadra Côte d'Or CarrieraSquadre di club1 2012- Côte d'Or? (?)Nazionale 2011-2017 Seychelles11 (2) 1 I due numeri indicano le presenze e le reti segnate, per le sole partite di campionato.Il simbolo → indica un trasferimento...

此條目可能包含不适用或被曲解的引用资料,部分内容的准确性无法被证實。 (2023年1月5日)请协助校核其中的错误以改善这篇条目。详情请参见条目的讨论页。 各国相关 主題列表 索引 国内生产总值 石油储量 国防预算 武装部队(军事) 官方语言 人口統計 人口密度 生育率 出生率 死亡率 自杀率 谋杀率 失业率 储蓄率 识字率 出口额 进口额 煤产量 发电量 监禁率 死刑 国债 ...

American supermarket chain in the southeast region For other uses, see Ingles (disambiguation). Ingles Markets, Inc.An Ingles location in Candler, NCFormerlyIngles Corner ShopCompany typePublicTraded asNasdaq: IMKTA (Class A)NASDAQIndustryRetail groceryFounded1963; 61 years ago (1963)Asheville, North Carolina, U.S.FounderRobert P. IngleHeadquartersBlack Mountain, North Carolina, U.S.Number of locations198[1]Areas servedAlabamaGeorgiaNorth CarolinaSouth CarolinaT...

For the 1928 American film, see Out of the Ruins (film). This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Out of the Ruins – news · newspapers · books · scholar · JSTOR (October 2010) (Learn how and when to remove this message) 1989 soundtrack album by Michael NymanOut of the Ruinsphotograph by Agnieszka ...














































