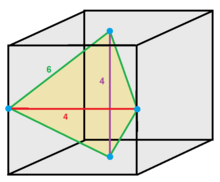
Disphenoid
|
Read other articles:

هناء ادور هنا إدور، 2017 معلومات شخصية الميلاد سنة 1946 (العمر 77–78 سنة)[1] البصرة مواطنة العراق الحياة العملية التعلّم كلية الحقوق جامعة بغداد المدرسة الأم جامعة بغداد المهنة سكرتيرة جمعية الأمل العراقية سبب الشهرة سعيها لإطلاق سراح النشطاء السياسيين الجو�...

Hussein bin AbdullahPutra Mahkota YordaniaPutra Mahkota Hussein pada tahun 2015.Kelahiran28 Juni 1994 (umur 29)Amman, YordaniaWangsaHashemiteAyahAbdullah IIIbuRania al-AbdullahPasanganRajwa Al Hussein (m. 2024)AgamaIslamAlmamaterKing's Academy, YordaniaUniversitas GeorgetownAkademi Militer Kerajaan Sandhurst Keluarga Kerajaan Yordania Baginda Sang RajaBaginda Sang Ratu Paduka Sang Putra Mahkota Paduka Putri Iman binti Abdullah Al - Hussein dari Yordania Pad...

Chemical compound EpiboxidineLegal statusLegal status Investigational Identifiers IUPAC name (1R,4S,6S)-6-(3-Methylisoxazol-5-yl)-7-azabicyclo[2.2.1]heptane CAS Number188895-96-7PubChem CID5747670ChemSpider4677635UNIIXI646L2ARJCompTox Dashboard (EPA)DTXSID20172258 Chemical and physical dataFormulaC10H14N2OMolar mass178.235 g·mol−13D model (JSmol)Interactive image SMILES CC1=NOC(=C1)[C@H]2C[C@@H]3CC[C@H]2N3 InChI InChI=1S/C10H14N2O/c1-6-4-10(13-12-6)8-5-7-2-3-9(8)11-7/h4,7-9,11H,2-3,5H...

Dominasi Tiongkok kedua di VietnamBắc thuộc lần thứ hai (北屬吝次二)43–544Map of the Liang dynasty in 502StatusDistrik dinasti Han Timur-Wu Timur-dinasti Jin-LiangIbu kotaJiaozhi (Vietnam: Giao Chỉ)Bahasa yang umum digunakanTionghoa LamaPemerintahanMonarkiKaisar • 43-57 Kaisar Guangwu dari Han (pertama)• 229-252 Sun Quan dari Wu Timur• 266-290 Kaisar Wu dari Jin• 420-422 Kaisar Wu dari Liu Song• 479-482 Kaisar Gao dari Qi Selatan�...

German architect and designer (1868–1940) For the Canadian writer, see Peter Behrens (writer). For the German musician and member of Trio, see Peter Behrens (musician). This article includes a list of general references, but it lacks sufficient corresponding inline citations. Please help to improve this article by introducing more precise citations. (November 2010) (Learn how and when to remove this template message) Peter BehrensPortrait of Peter Behrens by Max LiebermannBorn14 April 1868H...

City in California, United States This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Paramount, California – news · newspapers · books · scholar · JSTOR (November 2023) (Learn how and when to remove this template message) City in California, United StatesParamount, CaliforniaCityLocation of Paramount in Los Ang...
Baseball team Allentown Ambassadors Founded 1997 Ballpark Bicentennial Park Based in Allentown, Pennsylvania Team Colors Red, White, Blue (1997–2000) Red, White, Black (2001–2003) League Northeast League, 1997–1999, 2003 Northern League 2000–2002 Playoff Appearances 3: 1998, 1999, 2001 Owner Peter Karoly The Allentown Ambassadors were an independent baseball team that competed in the Northeast League and the Northern League from 1997 until 2003. They played their home games at Bicente...

La Lettera dei Sei (rumeno: Scrisoarea celor șase) è stata una lettera aperta firmata l'11 marzo 1989 da Silviu Brucan, insieme ad altri cinque dissidenti comunisti rumeni (Gheorghe Apostol, Alexandru Bârlădeanu, Grigore Răceanu, Corneliu Mănescu e Constantin Pârvulescu). Questa fu diffusa nei paesi occidentali e rappresentò uno dei più importanti documenti di dissenso interno al Partito Comunista Rumeno contro la dittatura di Nicolae Ceaușescu che, nove mesi dopo la pubblicazione d...

Gnathacmaeops Klasifikasi ilmiah Kerajaan: Animalia Filum: Arthropoda Kelas: Insecta Ordo: Coleoptera Famili: Cerambycidae Genus: Gnathacmaeops Gnathacmaeops adalah genus kumbang tanduk panjang yang tergolong famili Cerambycidae. Genus ini juga merupakan bagian dari ordo Coleoptera, kelas Insecta, filum Arthropoda, dan kingdom Animalia. Larva kumbang dalam genus ini biasanya mengebor ke dalam kayu dan dapat menyebabkan kerusakan pada batang kayu hidup atau kayu yang telah ditebang. Referensi...

Dokhtar-i-NoshirwanRoyal enthronement scene at Dokhtar i-Noshirwan.Shown within AfghanistanShow map of AfghanistanDokhtar-i-Noshirwan (Bactria)Show map of BactriaDokhtar-i-Noshirwan (West and Central Asia)Show map of West and Central AsiaDokhtar-i-Noshirwan (South Asia)Show map of South AsiaAlternative nameDokhtar-i-NoshirwanLocationSamangan, AfghanistanCoordinates35°45′33″N 67°52′34″E / 35.759179°N 67.876073°E / 35.759179; 67.876073TypeSettlementSite ...

1949 film ChampionTheatrical release posterDirected byMark RobsonScreenplay byCarl ForemanBased onthe story Championby Ring LardnerProduced byStanley KramerStarringKirk DouglasMarilyn MaxwellArthur KennedyCinematographyFranz PlanerEdited byHarry W. GerstadMusic byDimitri TiomkinProductioncompaniesScreen PlaysStanley Kramer ProductionsDistributed byUnited ArtistsRelease date May 20, 1949 (1949-05-20)[1] Running time99 minutesCountryUnited StatesLanguageEnglishBudget$570,...

Pour les articles homonymes, voir Kronecker. Leopold KroneckerLeopold Kronecker en 1865.BiographieNaissance 7 décembre 1823Legnica (royaume de Prusse)Décès 29 décembre 1891 (à 68 ans)BerlinSépulture Ancien cimetière Saint-MatthieuNationalité prussienneFormation Université Humboldt de Berlin (1841-1843)Université rhénane Frédéric-Guillaume de Bonn (jusqu'en 1843)Université de Wrocław (1843-1844)Activités Mathématicien, professeur d'universitéFratrie Hugo KroneckerAutres...

Sekelompok Wanita sedang menari Tari Pendet Akademi tari adalah sebuah lembaga pendidikan yang mempersiapkan seniman tari, pendidikan tari dan peneliti tari.[1] Ada beberapa lembaga tari di Indonesia, yaitu Akademi Tari di Lembaga Pendidikan Kesenian Jakarta (LPKJ) dan Institut Seni Indonesia Yogyakarta.[1] Sejarah Berdirinya akademi tari didorong kuat oleh para pecinta seni budaya Indonesia untuk mengembangkan apa yang dimilikinya.[1] Walaupun jauh sebelum itu pendidi...

يونس محمود معلومات شخصية الاسم الكامل يونس محمود خلف الميلاد 3 فبراير 1983 (العمر 41 سنة)[1]الدبس، كركوك، العراق الطول 1.85 م (6 قدم 1 بوصة) مركز اللعب مهاجم الجنسية العراق معلومات النادي النادي الحالي معتزل مسيرة الشباب سنوات فريق 1997–1999 كهرباء الدبس المسيرة الاحت�...

Town and municipality in Puerto Rico Town and Municipality in Puerto Rico, United StatesAguada Municipio de AguadaTown and MunicipalityFrom top, left to right: Main plaza and Iglesia San Francisco de Asís de la Aguada (Church of San Francisco de Asís); Ismael Chavalillo Delgado Aguada Multi-use Coliseum; Hacienda Caño Las Nasas (Caño Las Nasas Plantation) and Coloso sugarmill; and panoramic shoreline view FlagCoat of armsNicknames: La Villa de Sotomayor, Ciudad Del Descubrimiento, Vi...

Disambiguazione – Se stai cercando altri significati, vedi Montecchio (disambigua). Montecchiocomune Montecchio – Veduta LocalizzazioneStato Italia Regione Umbria Provincia Terni AmministrazioneSindacoFederico Gori dal 26-5-2014 (2º mandato dal 27-5-2019) Data di istituzione1948 TerritorioCoordinate42°39′44.42″N 12°17′16.91″E42°39′44.42″N, 12°17′16.91″E (Montecchio) Altitudine377 m s.l.m. Superficie49,22 km² Abitanti1 5...

This page is an archive of past discussions. Do not edit the contents of this page. If you wish to start a new discussion or revive an old one, please do so on the current talk page. Notablity of medical devices I've tagged some claims in Venowave, and I wonder if the subject is notable. Any guidance on the notability of medical devices? I don't know the particulars of this article, but does one randomized controlled trial on a device establish notability, for example? Biosthmors (talk) 20:18...

M47 パットン 性能諸元全長 8.51m全幅 3.51m全高 3.35m重量 46t懸架方式 トーションバー方式速度 48km/h行動距離 130km主砲 50口径 90mm M36副武装 12.7mm重機関銃M2×17.62mm重機関銃M1919A4×2(同軸1挺、車体正面右側1挺)装甲 101mmエンジン コンチネンタル AVDS-1790-5B4ストロークV型12気筒空冷ガソリンアリソン CD-850-4クロスドライブ式自動変速機(前進2段/後進1段)後輪駆動810hp(604kW)乗...

2009 2019 Élections européennes de 2014 en Italie 73 députés européens pour l'Italie 25 mai 2014 Corps électoral et résultats Population 60 626 442 Inscrits 50 662 460 Votants 28 991 258 57,22 % 7,8 Votes exprimés 27 448 906 Votes blancs 1 542 352 Parti démocrate – Matteo Renzi Voix 11 203 231 40,81 % 14,7 Sièges obtenus 31 10 Mouvement 5 étoiles – Beppe Gril...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada April 2016. Old PermicJangkauanU+10350..U+1037F(48 titik kode)BidangSMPAksaraPerm LamaAksara utamaAbjad Perm LamaTerpakai43 titik kodeTak terpakai5 titik kode kosongRiwayat versi Unicode7.043 (+43) Catatan: [1][2] Old Permic adalah blok Unicode yang ...