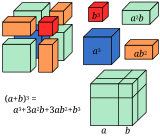
Hình học số học

Đường cong siêu ellip được xác định bởi chỉ có hữu hạn điểm hữu tỷ (chẳng hạn như các điểm và ) theo định lý Faltings.

Trong toán học, hình học số học đại khái là ứng dụng các kỹ thuật từ hình học đại số vào các vấn đề trong lý thuyết số.[1] Hình học số học tập trung vào hình học Diophantine, nghiên cứu các điểm hữu tỷ của các đa tạp đại số.[2][3]

Theo thuật ngữ trừu tượng hơn, hình học số học có thể được định nghĩa là nghiên cứu các sơ đồ loại hữu hạn trên phổ của vành số nguyên.

Tổng quan

Các đối tượng cổ điển được hình học số học đề cập đến là các điểm hữu tỷ: tập hợp nghiệm của một hệ phương trình đa thức trên các trường số, trường hữu hạn, trường p-adic hoặc trường hàm số đại số, tức là các trường không đóng đại số trừ các số thực. Điểm hữu tỷ có thể được đặc trưng trực tiếp bởi các [[hàm chiều cao]] đo độ phức tạp số học của chúng.[4]

Cấu trúc của các đa tạp đại số được xác định trên các trường không đại số đã trở thành một lĩnh vực quan tâm nảy sinh với sự phát triển trừu tượng hiện đại của hình học đại số. Trên các lĩnh vực hữu hạn, cohomology étale cung cấp các bất biến tôpô liên quan đến các đa tạp đại số.[5] Lý thuyết Hodge p-adic cung cấp các công cụ để kiểm tra khi các đặc tính chung của các đa tạp này trên các số phức mở rộng đến các trường trên các trường p-adic.[6]

Tham khảo

  1. ^ Sutherland, Andrew V. (ngày 5 tháng 9 năm 2013). “Introduction to Arithmetic Geometry” (PDF). Truy cập ngày 22 tháng 3 năm 2019.
  2. ^ Klarreich, Erica (ngày 28 tháng 6 năm 2016). “Peter Scholze and the Future of Arithmetic Geometry”. Truy cập ngày 22 tháng 3 năm 2019.
  3. ^ Poonen, Bjorn (2009). “Introduction to Arithmetic Geometry” (PDF). Truy cập ngày 22 tháng 3 năm 2019.
  4. ^ Lang, Serge (1997). Survey of Diophantine Geometry. Springer-Verlag. tr. 43–67. ISBN 3-540-61223-8. Zbl 0869.11051.
  5. ^ Grothendieck, Alexander (1960). “The cohomology theory of abstract algebraic varieties”. Proc. Internat. Congress Math. (Edinburgh, 1958). Cambridge University Press. tr. 103–118. MR 0130879.
  6. ^ Serre, Jean-Pierre (1967). “Résumé des cours, 1965–66”. Annuaire du Collège de France. Paris: 49–58.

Read other articles:

Konstantinus XI Palaiologos

Konstantin XI Paleolog Dragaš Κωνσταντῖνος ΙΑ' ΠαλαιολόγοςKaisar dari Kekaisaran BizantiumKonstantinus XI PalaiologosBerkuasa6 Januari 1449 – 29 Mei 1453Penobatan6 Januari 1449PendahuluIoannes VIII PalaiologosPenerusJabatan dihapuskanKelahiran(1404-02-08)8 Februari 1404Kematian29 Mei 1453(1453-05-29) (umur 48)AyahManuel II PalaiologosIbuHelena DragašPasanganTheodora ToccoCaterina GattilusioAnakTidak adaAgamaOrtodoks Yunani Konstantinus XI Palaiologos Dragaš...

 

Dysnomia (satelit)

DysnomiaDysnomia (kiri) di orbit sekitar Eris (tengah)(Teleskop Luar Angkasa Hubble)PenemuanDitemukan olehMichael E. Brown, M. A. van Dam, A. H. Bouchez, D. Le Mignant, R. D. Campbell, J. C. Y. Chin, A. Conrad, S. K. Hartman, E. M. Johansson, R. E. Lafon, D. L. Rabinowitz, P. J. Stomski Jr., D. M. Summers, C. A. Trujillo, and P. L. WizinowichTanggal penemuan10 September 2005PenamaanPenamaan(136199) Eris I DysnomiaNama alternatifS/2005 (136199) 1Kata sifat bahasa InggrisDysnomia...

 

Prananath College

College in Odisha Prananath College is a college in Odisha, located in the outskirts of the city of Bhubaneswar, India. The college was founded by Prananath Patnaik. The college is Affiliated to Utkal University. The college has autonomous status from UGC and holds NAAC A grade. [1] Prananath College (Autonomous)PN CollegeFormer nameKhordha (Degree) CollegeMottoutiṣṭhata jagrata varān prāpya nibodhata (ISO)Motto in EnglishArise, awake, and stop not till the goal is reached....

Referendum

referendum o referendo (gerundivo del verbo latino refero «riporto», «riferisco» dalla locuzione ad referendum «[convocazione] per riferire»[1]) è un istituto giuridico con cui si chiede all'elettorato di esprimersi con un voto diretto su una specifica proposta o domanda. Indice 1 Descrizione 1.1 Differenze con il plebiscito 2 Tipologia 3 Nel mondo 3.1 Croazia 3.2 Italia 3.3 Nuova Zelanda 3.4 Svizzera 3.5 San Marino 3.6 Taiwan 3.7 Unione europea 4 Note 5 Voci correlate 6 Altri ...

 

The Dance (song)

1990 single by Garth Brooks The DanceSingle by Garth Brooksfrom the album Garth Brooks B-sideIf Tomorrow Never ComesReleasedApril 30, 1990RecordedNovember 1, 1988GenreCountryLength3:40LabelCapitol NashvilleSongwriter(s)Tony ArataProducer(s)Allen ReynoldsGarth Brooks singles chronology Not Counting You (1990) The Dance (1990) Friends in Low Places (1990) The Dance is a song written by Tony Arata, and recorded by American country music singer Garth Brooks as the tenth and final track from his s...

 

Ben Verwaayen

Ben VerwaayenVerwaayen in 2009Born (1952-02-11) 11 February 1952 (age 72)Driebergen, Province of UtrechtNationalityDutchEducationDriebergen schoolAlma materUtrecht UniversityOccupationCEOEmployers ITT Corporation (1975-1988) PTT Telecom (1988-1997) Lucent Technologies (1997-2003) BT Group (2003-2008) Alcatel-Lucent (2008-2013) Political partyPeople's Party for Freedom and DemocracySpouseHelenaChildren2AwardsOfficier in de Orde van Oranje Nassau (Netherlands)Honorary Knight Commande...

Coloeus monedula

Come leggere il tassoboxTaccola Stato di conservazione Rischio minimo[1] Classificazione scientifica Dominio Eukaryota Regno Animalia Sottoregno Eumetazoa Superphylum Deuterostomia Phylum Chordata Subphylum Vertebrata Infraphylum Gnathostomata Superclasse Tetrapoda Classe Aves Sottoclasse Neornithes Superordine Neognathae Ordine Passeriformes Sottordine Oscines Infraordine Corvida Superfamiglia Corvoidea Famiglia Corvidae Genere Coloeus Specie C. monedula Nomenclatura binomiale Coloe...

 

Preet Bharara

American lawyer and former federal prosecutor (born 1968) Preet BhararaOfficial portrait, 2009United States Attorney for the Southern District of New YorkIn officeAugust 13, 2009 – March 11, 2017PresidentBarack ObamaDonald TrumpPreceded byMichael J. GarciaSucceeded byJoon Kim (acting) Personal detailsBornPreetinder Singh Bharara (1968-10-13) October 13, 1968 (age 55)Firozpur, Punjab, IndiaPolitical partyDemocraticSpouseDalya BhararaChildren3EducationHarvard University (BA)Colu...

 

Kalwan Assembly constituency

Constituency of the Maharashtra legislative assembly in India KalwanConstituency No. 117 for the Maharashtra Legislative AssemblyConstituency detailsCountryIndiaRegionWestern IndiaStateMaharashtraDistrictNashikLS constituencyDindoriReservationSTMember of Legislative Assembly14th Maharashtra Legislative AssemblyIncumbent Nitin Arjun Pawar PartyNationalist Congress Party (Ajit Pawar Faction)Elected year2019 Kalwan Assembly constituency is one of the 288 Vidhan Sabha (legislative assembly) const...

Drafting of the Universal Declaration of Human Rights

Process of UN project (1947–1948) The Universal Declaration of Human Rights was drafted between early 1947 and late 1948 by a committee formed by the United Nations Commission on Human Rights. Further discussion and amendments were made by the Commission on Human Rights, the Economic and Social Council and the General Assembly of the United Nations. Representatives of the UN Commission on the Status of Women participated in the meetings of the UN Commission on Human Rights, debating for a g...

 

约翰斯顿环礁

此條目可参照英語維基百科相應條目来扩充。 (2021年5月6日)若您熟悉来源语言和主题，请协助参考外语维基百科扩充条目。请勿直接提交机械翻译，也不要翻译不可靠、低品质内容。依版权协议，译文需在编辑摘要注明来源，或于讨论页顶部标记{{Translated page}}标签。 约翰斯顿环礁Kalama Atoll 美國本土外小島嶼 Johnston Atoll 旗幟颂歌：《星條旗》The Star-Spangled Banner約翰斯頓環礁�...

 

Новотитаровское сельское поселение

Сельское поселение России (МО 2-го уровня)Новотитаровское сельское поселение Флаг[d] Герб 45°14′09″ с. ш. 38°58′16″ в. д.HGЯO Страна  Россия Субъект РФ Краснодарский край Район Динской Включает 4 населённых пункта Адм. центр Новотитаровская Глава сельского пос�...

Хип-хоп

Хип-хоп Направление популярная музыка Истоки фанкдискоэлектронная музыкадабритм-энд-блюзреггидэнсхоллджаз[1]чтение нараспев[англ.]исполнение поэзииустная поэзияозначиваниедюжины[англ.]гриотыскэтразговорный блюз Время и место возникновения Начало 1970-х, Бронкс, Н...

 

List of Ryder Cup matches

Golf tournament List of Ryder Cup matchesTournament informationEstablished1927Tour(s)PGA Tour, European TourFormatMatch playCurrent champion Europe (in 2023) The Ryder Cup is a golf competition contested by teams from Europe and the United States. The competition was originally contested between Great Britain and the United States; players from the Channel Islands also appeared on the British team, Republic of Ireland golfers were added to the British team in 1953 (although the team name was...

 

إسفدن

إسفدن اسفدن city الاسم الرسمي Esfeden الإحداثيات 33°38′42″N 59°46′41″E / 33.64500°N 59.77806°E / 33.64500; 59.77806 تقسيم إداري  الدولة  إيران  المحافظة خراسان جنوبي  المقاطعة قائنات  الناحية Central عدد السكان (2006)  المجموع 3٬145  عدد الذكور 1791 (2016)[1]   عدد الإناث 1807 (2...

KNHC

This article is about the Seattle radio station. For the NWS facility that uses the code KNHC, see National Hurricane Center. Radio station in Seattle, WashingtonKNHCSeattle, WashingtonBroadcast areaSeattle, Washington and surrounding areaFrequency89.5 MHz (HD Radio)BrandingC89.5ProgrammingFormatDance hitsSubchannelsHD2: New wave C89.5 Save The WaveHD3: Downtempo Café ChillOwnershipOwnerSeattle Public SchoolsHistoryFirst air dateDecember 1969Former frequencies1210 kHz (1969–1971)Call ...

 

Daegu

Metropolitan City in Yeongnam, South Korea This article is about the city. For other uses, see Daegu (disambiguation). Metropolitan city in Yeongnam, South KoreaDaegu 대구Metropolitan city  transcription(s) • Hangul대구광역시 • Hanja大邱廣域市 • Revised RomanizationDaegu-gwangyeoksi • McCune-ReischauerTaegu-kwangyŏksiTop: Beomeo Jct, Second: a portrait in Kim Gwangseok Street in Bangcheon Market, Daegu 83 Tower, ARC cultur...

 

The Demon Headmaster (1996 TV series)

British television series (1996–1998) For the 2019 television series, see The Demon Headmaster (2019 TV series). The Demon HeadmasterGenreScience fictionHorrorCreated byGillian CrossStarringTerrence HardimanFrances AmeyGunnar Atli CautheryThomas SzekeresCountry of originUnited KingdomNo. of series3No. of episodes19ProductionRunning time25 minutesOriginal releaseNetworkBBC1Release2 January 1996 (1996-01-02) –22 January 1998 (1998-01-22) The Demon Headmaster is a British tele...

منتخب كوريا الجنوبية لكرة القاعدة

منتخب كوريا الجنوبية الوطني لكرة القاعدة البلد  كوريا الجنوبية الاتحاد اتحاد كوريا الجنوبية لكرة القاعدة الاتحاد القاري اتحاد آسيا لكرة القاعدة مركز آي بي أي إف مركز 8 تعديل مصدري - تعديل   منتخب كوريا الجنوبية الوطني لكرة القاعدة هو الفريق الذي يمثل كوريا الجنوبية ف�...

 

Fungsi zeta Riemann

Fungsi zeta Riemann ζ(z) digambarkan dengan pewarnaan domain.[1] Pole di z = 1 {\displaystyle z=1} dan dua akar di garis kritis. Fungsi zeta Riemann atau fungsi zeta Euler–Riemann adalah fungsi variabel kompleks, dilambangkan dengan huruf Yunani ζ {\displaystyle \zeta } (zeta), yang dirumuskan sebagai berikut ζ ( s ) = ∑ n = 1 ∞ 1 n s = 1 + 1 2 s + 1 3 s + ⋯ {\displaystyle \zeta (s)=\sum _{n=1}^{\infty }{\frac {1}{n^{s}}}=1+{\frac {1}{2^{s}}}+{\fr...