鬼六流ドッカン飛車

持駒 –
987654321 
香桂 金 金銀桂香一
 飛 銀  王角 二
歩 歩歩歩歩 歩歩三
      歩  四
 歩       五
  歩      六
歩歩 歩歩歩歩歩歩七
 角  飛銀金玉 八
香桂銀金   桂香九
持駒 –
Lady Killer Wham-Bam Rook

女殺しドッカン飛車（おんなごろしどっかんびしゃ、英：Lady Killer Wham-Bam Rook or Lady Killer Rushing Roo）または鬼六流ドッカン飛車もしくは団鬼六流どっかん中飛車（おにろくりゅうどっかんびしゃ、だんおにろくりゅうどっかんなかびしゃ、英：Oniroku Dan Dokkan Rook）は、将棋の戦法のひとつ。

BDSMポルノ作家の団鬼六が女性プロ棋士を倒すために使用したサプライズ将棋戦法として知られる[1]。

基本的に、居飛車の相手に使用する振り飛車戦法であり、戦略は角交換の脅威を伴う向かい飛車ポジションへの変化を計画している。

初期配置

1.1。 ▲5八飛。出だしは、初手から飛車をすぐ5筋へといった珍しい移動から開始する。

1.1。 △３四歩。後手が3筋の歩を進めることによって角を斜めに開くと、先手は鬼六流戦法を試みる可能性がある。

2.2。 ▲３八金。先手の2手目の動きは、3筋のへの右金のまれな動き。後にゴキゲン中飛車でも使用されていく相手の角交換からの△4五角打ちなどに対処している手。

さらなる発展

2.2。△８四歩。後手は、飛車先歩を突くことで飛車もアクティブにし始める。つまり、居飛車の位置を選択。

3.3。▲４九玉。先手は玉を盤の左側から遠ざけ始める。

ここでの目的は、玉を金美濃囲いにすばやく配置すること。

3.3。 △８五歩。後手は歩交換を目指して飛車先の歩を進める。

4.4。 ▲７六歩。後手の歩突きに応じて、先手は角道を開き、角の脱出ルートを確保。

4.4。 △６二銀、5。 ▲４八銀。後手は右の銀を中央に向かって動かす。これは、振り飛車の対戦相手に対する典型的な反応。先手も同様。

5.5。△４二玉、6。 ▲３九玉。対局者は双方、駒組み段階で玉を盤の右側に移動。

後手は囲いを築く。これは、振り飛車の対戦相手に対して配置された居飛車側に典型的な指し方。

7。△３二玉 ▲２八玉。 13手目までに先手は玉を金美濃に移動し終えた。

これは、飛車が8筋に移動させる前の鬼六流の基本的な位置。

バリアントの順序

上記の位置には、他の転置を介して到達。

攻撃シーケンス

7。 △８六歩。後手は、8筋で歩交換を開始。

8.8。 ▲同歩△同飛。歩交換。

9.9。▲２二角成△同銀。先手によって始された角交換。

角が8八から外れると、先手は向かい飛車をプレイできるようになる。

10.10。 ▲８八飛。先手は飛車を8筋に振り返り、向かい飛車のポジションを獲得。

後手の飛車は8六地点で保護されていないため、後手は先手の脅威に対応する必要がある。後手が８六歩、同歩、同飛と来れば、そこで角交換して、８八飛とするのが鬼六流の格子で、この手筋は1970年代の升田流から2010年代からはゴキゲン中飛車田村式でおなじみの手順となっている。

10.10。 △８七歩。後手は、先手の飛車上に歩を打ち、それを捕まえると脅して攻撃を続ける。

△同飛成と後手が飛車交換に応じるのは後手が８筋に打ち込むスキが残るため、囲い堅さでも先手に分があるとされる。

本筋としては飛車交換拒否の△８七歩であるが、以下▲９八飛 △８八角▲７八金△７九角成▲同金 △８八銀▲同金△同歩成▲７七角と進むと先手の堅さが生き、飛車と金を降ろされても▲３九銀打で容易には崩されない。以下△９八と▲８六角△８八飛▲８三飛△７一金などの進行が予想される。

関連項目

参考文献

  1. ^ 湯川 2015, p. 75–84, 女殺しドッカン飛車.

参考文献

  • 湯川, 博士 [Hiroshi Yukawa] (1989). 奇襲大全. マイナビ出版. ISBN 978-4-83995-787-2 
  • 飯塚祐紀、奇襲振り飛車戦法～その狙いと対策～、マイナビ将棋BOOKS、 第8章　鬼六流どっかん飛車、マイナビ出版：2014年12月、ISBN：978-4-8399-5372-0
居飛車
相居飛車
矢倉
相掛かり
角換わり
横歩取り
対振り飛車
舟囲い急戦
振り飛車
中飛車
四間飛車
三間飛車
向かい飛車
その他
囲い
居飛車
相居飛車
対振り飛車
振り飛車
対居飛車
相振り飛車
その他
カテゴリ カテゴリ

Read other articles:

Cimetière des Rois

Jalur tengah, pemakaman Plainpalais Cimetière des Rois (bahasa Prancis untuk Pemakaman para Raja) (resminya Cimetière de Plainpalais), adalah sebuah pemakaman di Jenewa, Swiss. Pemakaman ini biasanya disebut berdasarkan rue des Rois (bahasa Prancis untuk Jalan Para Raja) yang berada di dekat letaknya.[1] Pemakaman ini didirikan pada tahun 1482 untuk orang-orang yang meninggal karena wabah penyakit pada Abad Pertengahan, selama pandemi wabah kedua.[1] Tokoh yang dimakamkan Ha...

 

 

SDIT Al Iman

Artikel ini perlu diwikifikasi agar memenuhi standar kualitas Wikipedia. Anda dapat memberikan bantuan berupa penambahan pranala dalam, atau dengan merapikan tata letak dari artikel ini. Untuk keterangan lebih lanjut, klik [tampil] di bagian kanan. Mengganti markah HTML dengan markah wiki bila dimungkinkan. Tambahkan pranala wiki. Bila dirasa perlu, buatlah pautan ke artikel wiki lainnya dengan cara menambahkan [[ dan ]] pada kata yang bersangkutan (lihat WP:LINK untuk keterangan lebih lanjut...

 

 

1964 United States Senate election in Arizona

For related races, see 1964 United States Senate elections. 1964 United States Senate election in Arizona ← 1958 November 3, 1964 1970 →   Nominee Paul Fannin Roy Elson Party Republican Democratic Popular vote 241,089 227,712 Percentage 51.43% 48.57% County resultsFannin:      50–60% Elson:      50–60%      60–70%      70–80% U.S. senator before election...

Abbé Pierre

Abbé PierreL’abbé Pierre photographié en 1999 par le Studio Harcourt.FonctionDéputé françaisMeurthe-et-Moselle21 octobre 1945 - 4 juillet 1951BiographieNaissance 5 août 19124e arrondissement de LyonDécès 22 janvier 2007 (à 94 ans)5e arrondissement de ParisSépulture EstevilleNom de naissance Marie Joseph Henry GrouèsSurnoms Castor méditatif, Le père, l'Abbé PierreNationalité françaiseFormation Couvent des Capucins de CrestActivités Prêtre catholique, homme politique, ...

 

 

نادي أرسنال

  هذه المقالة عن نادي كرة القدم الإنجليزي المعروف باسم أرسنال. لالفريق الأرجنتيني، طالع أرسنال ساراندي. لهنا، طالع نادي أرسنال (توضيح). أرسنال الاسم الكامل نادي أرسنال لكرة القدم اللقب The Gunners (المدفعجية) The Arsenal (الترسانة) الاسم المختصر ARS تأسس عام 1886 (منذ 138 سنة) باسم دايل...

 

 

Sand devil

Species of shark Sand devil Conservation status Least Concern  (IUCN 3.1)[1] Scientific classification Domain: Eukaryota Kingdom: Animalia Phylum: Chordata Class: Chondrichthyes Subclass: Elasmobranchii Subdivision: Selachimorpha Order: Squatiniformes Family: Squatinidae Genus: Squatina Species: S. dumeril Binomial name Squatina dumerilLesueur, 1818 Range of the sand devil[1] The sand devil or Atlantic angel shark (Squatina dumeril) is a species of angelshark, family...

穆斯林世界

  「伊斯兰国家」重定向至此。關於一种政权形式，請見「伊斯兰国 (政权形式)」。 此條目需要补充更多来源。 (2019年2月22日)请协助補充多方面可靠来源以改善这篇条目，无法查证的内容可能會因為异议提出而被移除。致使用者：请搜索一下条目的标题（来源搜索：穆斯林世界 — 网页、新闻、书籍、学术、图像），以检查网络上是否存在该主题的更多可靠来源（...

 

 

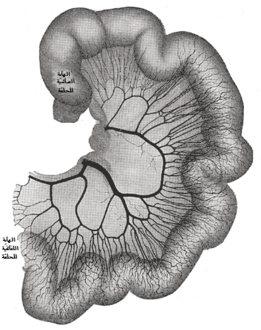
قوساء شريانية

هذه المقالة تحتاج للمزيد من الوصلات للمقالات الأخرى للمساعدة في ترابط مقالات الموسوعة. فضلًا ساعد في تحسين هذه المقالة بإضافة وصلات إلى المقالات المتعلقة بها الموجودة في النص الحالي. (يونيو 2018) قوساء شريانية لفائف الأمعاء الدقيقة، وتظهر توزيع الشرايين المعوية تفاصيل معرف...

 

 

مؤسسة محمد السادس للعلماء الأفارقة

مؤسسة محمد السادس للعلماء الأفارقة مقر مؤسسة محمد السادس للعلماء الأفارقة في فاس الاختصار (بالفرنسية: FM6OA)‏  البلد المغرب  المقر الرئيسي فاس، المغرب تاريخ التأسيس 13 يوليو 2015 الاهتمامات توحيد وتنسيق جهود العلماء المسلمين في مجال التعريف بقيم الإسلام السمحة وترسيخها. ...

نادي الحمادة

نادي الحمادة شعار نادي الحمادة الألوان الأزرق ، الأبيض ، الأسود تأسس عام 1391هـ [1] البلد السعودية  الإدارة المالك الهيئة العامة للرياضة بندر بن صخر السديري تعديل مصدري - تعديل   نادي الحمادة، هو نادٍ سعودي لكرة القدم تأسس عام 1971. ومقره في الغاط.[2] تاريخ صعد الناد�...

 

 

Neighborhoods in Spokane, Washington

Main article: Spokane, Washington Panoramic map of Spokane, 1905 Neighborhoods in Spokane, Washington are officially grouped by the Spokane City Council into three main city council districts: 1, 2, and 3. Each city council district contains multiple, official neighborhoods that are recognized with a neighborhood council. Informally, neighborhoods are colloquially grouped by local geographical, geological, cultural, or historical features (such as South Hill, North Side, Five Mile, Hillyard, ...

 

 

Victoria Gap

Mountain pass in Hong Kong Victoria GapTraditional Chinese爐峰峽Simplified Chinese炉峰峡TranscriptionsStandard MandarinHanyu PinyinLúfēngxiáYue: CantoneseJyutpinglou4 fung1 haap6 The Lions Pavilion at Victoria Gap, with the Peak Tower in the background The Peak Tower viewed from The Peak Galleria at Victoria Gap The road junction at Victoria Gap, next to the Peak Tower. From left to right: Peak Road, the Peak Lookout Restaurant, Harlech Road (with street vendor), Mount Aust...

A3 motorway (Serbia)

Road in Serbia This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: A3 motorway Serbia – news · newspapers · books · scholar · JSTOR (August 2016) (Learn how and when to remove this message) Autoput A 3Serbian: Autoput A3Serbian Cyrillic: Аутопут А3Route informationPart of E70 Maintained by JP Putevi...

 

 

Myanmar at the 2024 Summer Olympics

Sporting event delegationMyanmar at the2024 Summer OlympicsFlag of MyanmarIOC codeMYANOCMyanmar Olympic CommitteeWebsitewww.myasoc.org (in Burmese)in Paris, FranceJuly 26, 2024 (2024-07-26) – August 11, 2024 (2024-08-11)Competitors1 in 1 sportMedals Gold 0 Silver 0 Bronze 0 Total 0 Summer Olympics appearances (overview)19481952195619601964196819721976198019841988199219962000200420082012201620202024 Myanmar is scheduled to compete at the 2024 Summer Ol...

 

 

2036年夏季奥林匹克运动会

此條目可参照英語維基百科相應條目来扩充。 (2021年10月12日)若您熟悉来源语言和主题，请协助参考外语维基百科扩充条目。请勿直接提交机械翻译，也不要翻译不可靠、低品质内容。依版权协议，译文需在编辑摘要注明来源，或于讨论页顶部标记{{Translated page}}标签。 此條目有关未來已定或預計會發生的事件。未有可靠来源的臆測內容可能會被移除，隨事件临近而有更多可�...

Additive white Gaussian noise

Basic noise model used in information theory This article relies largely or entirely on a single source. Relevant discussion may be found on the talk page. Please help improve this article by introducing citations to additional sources.Find sources: Additive white Gaussian noise – news · newspapers · books · scholar · JSTOR (February 2020) Additive white Gaussian noise (AWGN) is a basic noise model used in information theory to mimic the effect of many...

 

 

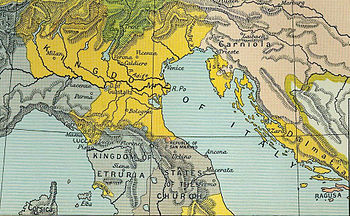
Kingdom of Italy (Napoleonic)

State in northern Italy, 1805 to 1814 For other kingdoms of Italy, see Kingdom of Italy (disambiguation). Kingdom of ItalyRegno d'Italia (Italian)Royaume d'Italie (French)1805–1814 Flag Coat of arms The Kingdom of Italy in 1812StatusClient state of the French EmpireCapitalMilanCommon languagesFrenchItalianLombardVenetianEmilianRomagnolFriulianReligion CatholicDemonym(s)ItalianGovernmentUnitary absolute monarchyKing • 1805–1814 Napoleon I Viceroy • 18...

 

 

Shmuel Sambursky

Professor Sambursky (1935), lecturing in the Institute of Physics, The Hebrew University in Jerusalem. Shmuel Sambursky, or Samuel Sambursky (1900-1990) Hebrew: שמואל סמבורסקי was a German, Palestinian, and Israeli physicist, professor, and author during the respective epochs of his country —Germany, Mandatory Palestine, and Israel.[1] Early life and migration Sambursky was born in Königsberg, Germany 30 October 1900. Educated in Germany,[2] Dr. Sambursky emigr...

Wisława Szymborska

Wisława SzymborskaBiographieNaissance 2 juillet 1923Prowent (pl) (Grande-Pologne)Décès 1er février 2012 (à 88 ans)Cracovie (Pologne)Sépulture Cimetière RakowickiNom de naissance Maria Wisława Anna SzymborskaSurnom Wisława SzymborskaNationalité PologneFormation Université JagellonneActivités Poétesse, mécanicienne, critique littéraire, essayiste, écrivaine, traductrice, critiquePère Wincenty Szymborski (d)Mère Anna Szymborska (d)Conjoint Adam Włodek (en) (de 1948 �...

 

 

Yogi Bear

American animated television and film character This article is about the character. For the 2010 film of this name, see Yogi Bear (film). For the original show and all other works featuring the character, see Yogi Bear (disambiguation). Jellystone redirects here. For the animated HBO Max series of the same name, see Jellystone! Not to be confused with Yogi Berra. Fictional character Yogi BearThe Yogi Bear Show characterFirst appearanceYogi Bear's Big Break (The Huckleberry Hound Show, 1958)C...