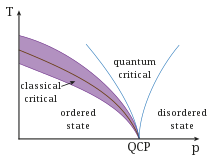
朝永–ラッティンジャー液体
|
Read other articles:
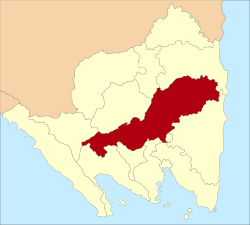
Peta Lokasi Kabupaten Lampung Tengah di Lampung Berikut ini adalah daftar kecamatan dan kelurahan/desa di kabupaten Lampung Tengah, Provinsi Lampung, Indonesia. Kabupaten Lampung Tengah terdiri dari 28 kecamatan, 10 kelurahan, dan 301 desa. Pada tahun 2017, jumlah penduduknya mencapai 1.468.875 jiwa dengan luas wilayah 3.802,68 km² dan sebaran penduduk 386 jiwa/km².[1][2] Daftar kecamatan dan kelurahan di Kabupaten Lampung Tengah, adalah sebagai berikut: Kode Kemendagri Keca...

British film industry award BAFTA Award for Best ActorThe 2023 recipient: Cillian MurphyAwarded forBest Performance by an Actor in a Leading RoleLocationUnited KingdomPresented byBritish Academy of Film and Television ArtsCurrently held byCillian Murphy for Oppenheimer (2023)Websitehttp://www.bafta.org/ Best Actor in a Leading Role is a British Academy Film Award presented annually by the British Academy of Film and Television Arts (BAFTA) to recognize an actor who has delivered an outstandin...

Gabriel Mercado Informasi pribadiNama lengkap Gabriel Iván Mercado[1]Tanggal lahir 18 Maret 1987 (umur 37)Tempat lahir Puerto Madryn, ArgentinaTinggi 181 cm (5 ft 11 in)Posisi bermain BekInformasi klubKlub saat ini SevillaNomor 25Karier junior Racing ClubKarier senior*Tahun Tim Tampil (Gol)2006–2010 Racing Club 96 (1)2010–2012 Estudiantes LP 66 (10)2012–2016 River Plate 94 (5)2016– Sevilla 40 (3)Tim nasional2010– Argentina 22 (3) * Penampilan dan gol di...

State railway of the German Democratic Republic (1945–1993) This article is about the state railway of the former post-war East Germany. For its pan-Germany predecessor during the inter-war years, see Deutsche Reichsbahn. This article includes a list of references, related reading, or external links, but its sources remain unclear because it lacks inline citations. Please help improve this article by introducing more precise citations. (June 2020) (Learn how and when to remove this template...

Impact of the COVID-19 pandemic on basketball This article needs to be updated. Please help update this article to reflect recent events or newly available information. (April 2022) Part of a series on theCOVID-19 pandemicScientifically accurate atomic model of the external structure of SARS-CoV-2. Each ball is an atom. COVID-19 (disease) SARS-CoV-2 (virus) Cases Deaths Timeline 2019 2020 January responses February responses March responses April responses May responses June responses July re...

Organ system of the reproductive organs and the urinary system This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Genitourinary system – news · newspapers · books · scholar · JSTOR (April 2008) (Learn how and when to remove this template message) Genitourinary systemSome components of the female and male genito...

1981 1988 Élections législatives de 1986 dans l'Ardèche 3 sièges de députés à l'Assemblée nationale 16 mars 1986 Corps électoral et résultats Population 267 970 Inscrits 200 920 Votants 160 996 80,13 % 8,6 Votes exprimés 152 546 Parti socialiste – Robert Chapuis Voix 50 634 33,19 % 3,4 Députés élus 1 1 Rassemblement pour la République – Régis Perbet Voix 40 239 26,38 % &...

Questa voce o sezione sull'argomento edizioni di competizioni calcistiche non cita le fonti necessarie o quelle presenti sono insufficienti. Puoi migliorare questa voce aggiungendo citazioni da fonti attendibili secondo le linee guida sull'uso delle fonti. Segui i suggerimenti del progetto di riferimento. Premier League Malti 2007-2008 Competizione Premier League Malti Sport Calcio Edizione 93ª Organizzatore MFA Luogo Malta Partecipanti 10 Formula 1 girone all'italiana Risultati ...

Living-quarters for soldiers For other uses, see Billet (disambiguation). This article includes a list of general references, but it lacks sufficient corresponding inline citations. Please help to improve this article by introducing more precise citations. (September 2009) (Learn how and when to remove this template message) A billet scene. Painting from 1898 by Vilhelm Rosenstand. A billet is a living-quarters to which a soldier is assigned to sleep. Historically, a billet was a private dwel...

Immigration Act of 1882Long titleAn Act to regulate Immigration.Enacted bythe 47th United States CongressEffectiveAugust 3, 1882CitationsPublic lawPub. L.Tooltip Public Law (United States) 47–376Statutes at Large22 Stat. 214Legislative historySigned into law by President Chester A. Arthur on August 3, 1882 The Immigration Act of 1882 was a United States federal law signed by President Chester A. Arthur on August 3, 1882. It imposed a head tax on non-citizens of the Unit...

Egyptian pharaoh Mersekhemre InedMersekhemre Neferhotep II ?Statue of Mersekhemre Neferhotep II, who could be the same person as Mersekhemre Ined. Discovered in the Karnak cachette, now on display in the Egyptian Museum, CG 42024.PharaohReign3 years, 1 to 4 months and 1 dayPredecessorSankhenre SewadjtuSuccessorSewadjkare HoriRoyal titulary Prenomen (Praenomen) Mersekhemre[1][2] Mr-sḫm-Rˁ He who loves the power of Ra Turin canon: Mersekhemre Ined Mr.j-sḫm-Rˁ-in...

Cave and archaeological site in East Timor Lene HaraPaintings in Lene Hara caveLocation in East TimorShow map of East TimorLene Hara cave (Asia)Show map of AsiaLocationLautém DistrictRegioneastern tip of East TimorCoordinates8°23′32″S 127°17′25″E / 8.39222°S 127.29028°E / -8.39222; 127.29028 A carved face The Lena Hara cave is the main cave of a system of solutional caves in the Lautém District at the eastern tip of East Timor (Timor-Leste), close to the ...

Royal Navy Fleet Air Arm Squadron 744 Naval Air SquadronSquadron badgeActive1 March 1943 - 1 June 19446 March 1944 - 1 December 194720 July 1951 - 1 March 19541 March 1954 - 31 October 195614 November 2018 - presentCountry United KingdomBranch Royal NavyTypeFleet Air Arm Second Line SquadronRole Air Gunner Training Squadron Merchant Aircraft Carrier and Anti-Submarine training Anti-Submarine Trials and Development Unit Mission Systems and Armament Test and Evaluation Squadron Part&#...

Pour les articles homonymes, voir Etchepare. Cet article est une ébauche concernant les Pyrénées-Atlantiques et un écrivain français. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Bernard d'EtchepareLinguæ Vasconum PrimitiæBiographieNaissance 1480Bussunarits-SarrasquetteDécès 1545Activités Écrivain, prêtre catholique, ministre du culteAutres informationsGenre artistique Poésiemodifier - modifier l...

Relief yaksini berdiri di bawah pohon asoka, dibuat pada zaman kerajaan Sunga, abad ke-2 SM. Yaksini atau yaksi (Dewanagari: यक्षिणी; ,IAST: yakṣiṇī,; bahasa Pali: yakkhiṇī atau yakkhī) adalah kelompok makhluk gaib berjenis kelamin perempuan dalam kepercayaan agama Hindu, Buddha, dan Jain, yang berbeda dengan dewa, asura, gandarwa, atau apsari (bidadari). Yaksini dan makhluk serupa yang berjenis kelamin jantan yaitu yaksa, merupakan salah satu makhluk...

此條目可参照英語維基百科相應條目来扩充。 (2021年5月6日)若您熟悉来源语言和主题,请协助参考外语维基百科扩充条目。请勿直接提交机械翻译,也不要翻译不可靠、低品质内容。依版权协议,译文需在编辑摘要注明来源,或于讨论页顶部标记{{Translated page}}标签。 约翰斯顿环礁Kalama Atoll 美國本土外小島嶼 Johnston Atoll 旗幟颂歌:《星條旗》The Star-Spangled Banner約翰斯頓環礁�...

此条目序言章节没有充分总结全文内容要点。 (2019年3月21日)请考虑扩充序言,清晰概述条目所有重點。请在条目的讨论页讨论此问题。 哈萨克斯坦總統哈薩克總統旗現任Қасым-Жомарт Кемелұлы Тоқаев卡瑟姆若马尔特·托卡耶夫自2019年3月20日在任任期7年首任努尔苏丹·纳扎尔巴耶夫设立1990年4月24日(哈薩克蘇維埃社會主義共和國總統) 哈萨克斯坦 哈萨克斯坦政府...

1966 EuropeanAthletics ChampionshipsTrack events100 mmenwomen200 mmenwomen400 mmenwomen800 mmenwomen1500 mmen5000 mmen10,000 mmen80 m hurdleswomen110 m hurdlesmen400 m hurdlesmen3000 msteeplechasemen4×100 m relaymenwomen4×400 m relaymenRoad eventsMarathonmen20 km walkmen50 km walkmenField eventsHigh jumpmenwomenPole vaultmenLong jumpmenwomenTriple jumpmenShot putmenwomenDiscus throwmenwomenHammer throwmenJavelin throwmenwomenCombined eventsPentathlonwomenDecathlonmenvte The women's 4 x 100...

Viceroy of India from 1888 to 1895 His Excellency The Most HonourableThe Marquess of LansdowneKG GCSI GCMG GCIE PCMinister without PortfolioIn office25 May 1915 – 10 December 1916MonarchGeorge VPrime MinisterH. H. AsquithPreceded byMichael Hicks BeachSucceeded byArthur HendersonLeader of the House of LordsIn office13 October 1903 – 4 December 1905MonarchEdward VIIPrime MinisterArthur BalfourPreceded byThe Duke of DevonshireSucceeded byThe Marquess of RiponSecretary of St...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Oktober 2022. Engravir yang menggambarkan Maria Prophetissima dari buku Michael Maier Symbola Aurea Mensae Duodecim Nationum (1617). Maria Orang Yahudi (bahasa Latin: Maria Prophetissima), atau Maria sang Nabiah, adalah seorang alkemis awal yang dikenal dari kar...