Xeometría de Riemann
|
Read other articles:
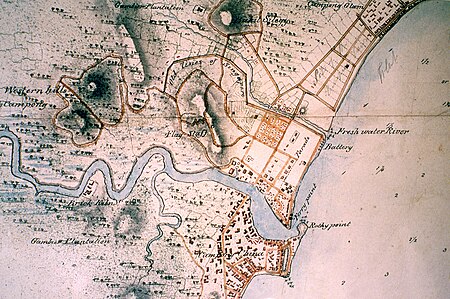
Sungai SingapuraSungai Singapura yang mengalir melalui kawasan pusat bisnis di SingapuraPeta Sungai Singapura pada awal abad ke-19LokasiCountry SingapuraCiri-ciri fisikHulu sungai - lokasiKanal Alexandra Muara sungai - lokasiMarina ChannelPanjang32 km (20 mi) Sungai Singapura (Melayu: Sungei Singapuracode: ms is deprecated , Hanzi: 新加坡河) adalah sebuah sungai kecil yang mempunyai nilai historis yang tinggi karena merupakan awal mula pe...

American politician (1896-1960) Herbert O'ConorUnited States Senatorfrom MarylandIn officeJanuary 3, 1947 – January 3, 1953Preceded byGeorge L. P. RadcliffeSucceeded byJames Glenn BeallChair of the National Governors AssociationIn officeJune 21, 1942 – June 20, 1943Preceded byHarold StassenSucceeded byLeverett Saltonstall51st Governor of MarylandIn officeJanuary 11, 1939 – January 3, 1947Preceded byHarry NiceSucceeded byWilliam Preston Lane Jr.Attorney General...

American politician (born 1931) Connie MorellaUnited States Ambassador to the Organisation for Economic Co-operation and DevelopmentIn officeAugust 1, 2003 – August 6, 2007PresidentGeorge W. BushPreceded byJeanne PhillipsSucceeded byChristopher EganMember of the U.S. House of Representativesfrom Maryland's 8th districtIn officeJanuary 3, 1987 – January 3, 2003Preceded byMichael BarnesSucceeded byChris Van HollenMember of the Maryland House of Dele...

Grand Prix Jef Scherens 2017 GénéralitésCourse51e Tour de Louvain-Mémorial Jef ScherensCompétitionsUCI Europe Tour 2017 1.1Coupe de Belgique de cyclisme sur route 2017Date20 août 2017Distance178,6 kmPays BelgiqueLieu de départLouvainLieu d'arrivéeLouvainÉquipes20Site officielSite officielRésultatsVainqueur Timothy Dupont (Verandas Willems-Crelan)Deuxième Kenny Dehaes (Wanty-Groupe Gobert)Troisième Justin Jules (WB-Veranclassic-Aqua Protect) ◀20162018▶Documentation Le...

Cet article est une ébauche concernant le Concours Eurovision de la chanson et la Suède. Vous pouvez partager vos connaissances en l’améliorant (comment ?) ; pour plus d’indications, visitez le projet Eurovision. Suèdeau Concours Eurovision 2011 Données clés Pays Suède Chanson « Popular » Interprète Eric Saade Compositeur Fredrik Kempe Parolier Fredrik Kempe Langue Anglais Sélection nationale Type de sélection Émission téléviséeMelodifestivalen D...

Prime Minister of Australia from 2015 to 2018 The HonourableMalcolm TurnbullACOfficial portrait, 201529th Prime Minister of AustraliaIn office15 September 2015 – 24 August 2018MonarchElizabeth IIGovernor GeneralSir Peter CosgroveDeputyWarren TrussBarnaby JoyceMichael McCormackPreceded byTony AbbottSucceeded byScott MorrisonLeader of the Liberal PartyIn office14 September 2015 – 24 August 2018DeputyJulie BishopPreceded byTony AbbottSucceeded byScott MorrisonIn office16 Se...

American venture capital firm Sequoia Capital Operations, LLCCompany typePrivateIndustryVenture capitalFounded1972; 52 years ago (1972)FounderDon ValentineHeadquartersMenlo Park, California, U.S.Area servedUnited States, Southeast Asia, India, China, IsraelKey peopleMichael MoritzDouglas LeoneJim GoetzRoelof BothaProductsInvestmentsAUMUS$85 billion (2022)Websitesequoiacap.com Sequoia Capital is an American venture capital firm headquartered in Menlo Park, California which sp...

此條目可参照英語維基百科相應條目来扩充。 (2021年5月6日)若您熟悉来源语言和主题,请协助参考外语维基百科扩充条目。请勿直接提交机械翻译,也不要翻译不可靠、低品质内容。依版权协议,译文需在编辑摘要注明来源,或于讨论页顶部标记{{Translated page}}标签。 约翰斯顿环礁Kalama Atoll 美國本土外小島嶼 Johnston Atoll 旗幟颂歌:《星條旗》The Star-Spangled Banner約翰斯頓環礁�...

Irish private coach operator This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: JJ Kavanagh and Sons – news · newspapers · books · scholar · JSTOR (April 2024) (Learn how and when to remove this message) JJ Kavanagh and SonsFounded1919HeadquartersUrlingford, County Kilkenny, IrelandService areaIrelandUnited Kin...

تشيرنوبل (بالأوكرانية: Чорнобиль) تشيرنوبل تاريخ التأسيس 1970 تقسيم إداري البلد أوكرانيا (1991–) [1] عاصمة لـ منطقة تشرنوبل المحظورة خصائص جغرافية إحداثيات 51°16′00″N 30°13′00″E / 51.266666666667°N 30.216666666667°E / 51.266666666667; 30.216666666667 المساحة 25 كيلومتر مربع[...

Irish-American song The original sheet music Too-Ra-Loo-Ra-Loo-Ral (That's an Irish Lullaby) is a classic Irish-American song that was written in 1913 by composer James Royce Shannon (1881–1946) for the Tin Pan Alley musical Shameen Dhu. The original recording of the song, by Chauncey Olcott, peaked at #1 on the music charts. The song was brought back to prominence by Bing Crosby's performance in 1944's Going My Way. Crosby's single sold over a million copies and peaked at #4 on the Billboa...

English painter Henry Hetherington EmmersonBorn(1831-11-11)11 November 1831Chester-le-Street, County Durham, EnglandDied28 August 1895(1895-08-28) (aged 63)Cullercoats, EnglandNationalityEnglishKnown forPainting Henry Hetherington Emmerson (11 November 1831 – 28 August 1895), commonly known by his initials H. H. Emmerson, was an English painter and illustrator. Emmerson (née Hetherington).[1] At the age of 13, he went to Newcastle, where he studied painting and engraving ...

Cámara de Castilla Tipo consejoFundación 1518Fundador Carlos I de EspañaJuana I de CastillaDisolución 1834[editar datos en Wikidata] La Cámara de Castilla era un consejo del régimen polisinodial de la Monarquía española, encargado de aconsejar al rey en los nombramientos, la administración de la gracia y mercedes reales, concepto jurídico propio del poder que ejercen los reyes por su mera voluntad.[1][2] Historia y funciones Fue fundado en 1518 en época del ...
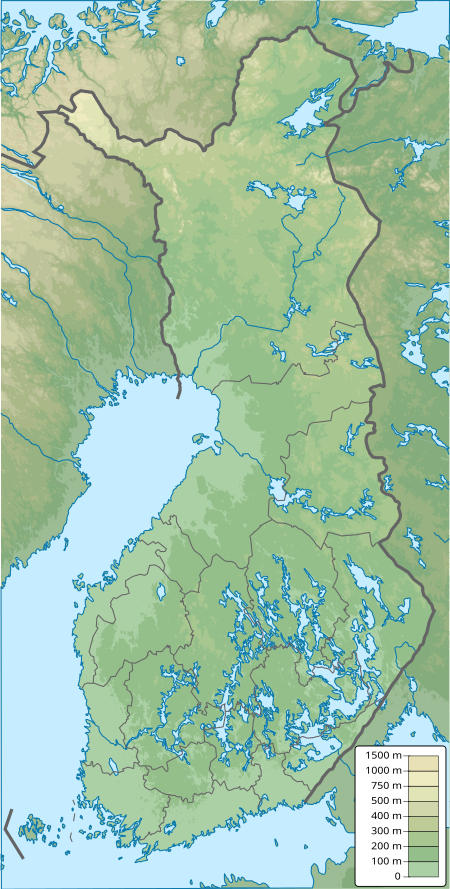
Lokasi Laut Bothnia. Laut Bothnia (Swedia: Bottenhavet, Finlandia: Selkämeri), atau Laut Rauma, menghubungkan Teluk Bothnia dengan Laut Baltik. Kvarken terletak antara keduanya. Bersama, Laut Bothnian dan Teluk Bothnia membentuk sebuah daerah yang lebih besar, Teluk Bothnia. Teluk Bothnia terletak antara Swedia, di Barat, Finlandia, di Timur, dan Laut Åland, Quark Utara, dan Laut Kepulauan di selatan. Luas permukaan Laut Bothnia sekitar 79.000 km ². Kota-kota pesisir terbesar, dari se...

This article is about the British Indian title. For other uses, see Khan (disambiguation). AwardKhan Sahib MedalTitle Badge for Khan SahibTypeCivil decorationAwarded forPublic servicePresented byViceroy & Governor-General of India on behalf of the British Indian GovernmentEligibilityMuslim, Parsi and Jewish Commonwealth subjects of British IndiaStatusDiscontinued since 1947Last awarded1947PrecedenceNext (higher)Khan Bahadur (title)EquivalentRai Sahib (for Hindus)Next (lower)Khan...

Japanese cattle ranch Ito RanchSky view of Ito Ranch in 2023.CountryJapanCoordinates34°39′50″N 136°25′21″E / 34.6638°N 136.4224°E / 34.6638; 136.4224Established1953OwnerHiroki ItoWebsiteOfficial website Ito Ranch (伊藤牧場) is a Matsusaka beef farm located near the city of Tsu, Mie Prefecture, Japan, dedicated to raising kuroge wagyū or Japanese Black beef. Founded in 1953, as of 2024[update] it is owned by Hiroki Ito (伊藤浩基), and is cla...

Este artículo o sección necesita referencias que aparezcan en una publicación acreditada. Busca fuentes: «Supermodelo (programa de televisión)» – noticias · libros · académico · imágenesEste aviso fue puesto el 16 de mayo de 2013. Supermodelos Serie de televisiónGénero Reality showCreado por Tyra BanksDirigido por Floren Abad (2006-2007)Pablo del Pozo Gómez (2007-2008)Presentado por Judit Mascó (2006-2007)Eloisa González (2008)Protagonistas Judit MascóJu...

المنظور الجديد حول بولس هو نقلة نوعية في الطريقة التي يفسر بها العلماء، وخاصة البروتسانت، كتابات الرسول بولس.[1][2][3] فمنذ الإصلاح البروتستانتي، كانت دراسات كتابات بولس متأثرة جداً بآراء الكنائس اللوثرية والمصلحة (المنظور القديم) التي تعزو بعض السمات السلبية ا...

Magnano komune di Italia Tempat Negara berdaulatItaliaDaerah di ItaliaPiemonteProvinsi di ItaliaProvinsi Biella NegaraItalia Ibu kotaMagnano PendudukTotal354 (2023 )GeografiLuas wilayah10,56 km² [convert: unit tak dikenal]Ketinggian543 m Berbatasan denganBollengo Cerrione Palazzo Canavese Piverone Torrazzo Zimone Zubiena SejarahSanto pelindungSecundus of Victimulum (en) Informasi tambahanKode pos13887 Zona waktuUTC+1 UTC+2 Kode telepon015 ID ISTAT096030 Kode kadaster ItaliaE821 La...

Technology involving the manufacturing and innovation of clothing materials This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Clothing technology – news · newspapers · books · scholar · JSTOR (April 2022) (Learn how and when to remove this message) A Gore-Tex raincoat. Clothing technology describes advances in...



