吠檀多不二論
|
Read other articles:

Chertan Classe spettraleA2V Distanza dal Sole165 anni luce CostellazioneLeone Coordinate(all'epoca J2000) Ascensione retta11h 14m 14,41s Declinazione15° 25′ 46,45″ Dati fisiciRaggio medio4,3[1] R⊙ Massa2,93[2] M⊙ Velocità di rotazione23 Temperaturasuperficiale9320 (media) Luminosità120 L⊙ Indice di colore (B-V)0,01 Età stimata450 milioni Dati osservativiMagnitudine app.+3,33 Magnitudine ass.-0,19[3] Velocità radiale-7.6 km...

American lawyer and politician (1837–1916) Harrison E. HavensMember of the U.S. House of Representativesfrom MissouriIn officeMarch 4, 1871 – March 3, 1875Preceded byAbram ComingoSucceeded byRobert Anthony HatcherConstituency4th district (1871–1873)6th district (1873–1875) Personal detailsBorn(1837-12-15)December 15, 1837Franklin County, Ohio, USDiedAugust 16, 1916(1916-08-16) (aged 78)Havana, CubaResting placeColon Cemetery, HavanaPolitical partyRepublican H...

Main article: 1916 United States presidential election 1916 United States presidential election in North Carolina ← 1912 November 7, 1916 1920 → All 12 North Carolina votes to the Electoral College Nominee Woodrow Wilson Charles Evans Hughes Party Democratic Republican Home state New Jersey New York Running mate Thomas R. Marshall Charles W. Fairbanks Electoral vote 12 0 Popular vote 168,383 120,890 Percentage 58.10% 41.71% County Results Wils...

Questa voce sull'argomento edizioni di competizioni calcistiche è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti del progetto di riferimento. Coppa delle Alpi 1981 Competizione Coppa delle Alpi Sport Calcio Edizione 21ª Organizzatore FFF, ASF Luogo Svizzera Francia Partecipanti 8 Risultati Vincitore Basilea(3º titolo) Secondo Sochaux Statistiche Incontri disputati 17 Gol segnati 56 (3,29 per incontro)...

2014 soundtrack album by John PowellHow to Train Your Dragon 2Soundtrack album by John PowellReleasedJune 13, 2014Recorded2012–2014GenreFilm scoreLength1:11:23LabelRelativity Music GroupJohn Powell chronology Rio 2(2014) How to Train Your Dragon 2(2014) Pan(2015) Singles from How to Train Your Dragon 2 Into a FantasyReleased: June 9, 2014 How to Train Your Dragon 2: Music from the Motion Picture is a soundtrack album to the 2014 film How to Train Your Dragon 2, and was released by ...

Canadian ice hockey player Ice hockey player Wade Skolney Born (1981-06-24) June 24, 1981 (age 42)Humboldt, Saskatchewan, CanadaHeight 6 ft 0 in (183 cm)Weight 195 lb (88 kg; 13 st 13 lb)Position DefenceShot RightPlayed for Philadelphia PhantomsPhiladelphia FlyersWilkes-Barre Scranton PenguinsStraubing TigersAcroni JeseniceNHL draft UndraftedPlaying career 2002–2011 Wade Skolney (born June 24, 1981) is a Canadian former professional ice hockey pla...

عمر كمال عبد الواحد عمر كمال مع مصر في كأس الأمم الإفريقية 2021 معلومات شخصية الاسم الكامل عمر كمال سيد عبد الواحد[1] الميلاد 29 سبتمبر 1993 (العمر 30 سنة)مصر الطول 1.86 م (6 قدم 1 بوصة) مركز اللعب ظهير أيمن الجنسية مصر معلومات النادي النادي الحالي الأهلي الرقم 29 مسيرة ا...

Organized environmental movement in the US 1970s US postage stamp block People's Climate March (2017) The organized environmental movement is represented by a wide range of non-governmental organizations or NGOs that seek to address environmental issues in the United States. They operate on local, national, and international scales. Environmental NGOs vary widely in political views and in the ways they seek to influence the environmental policy of the United States and other governments. The ...

Commanding officer of the Russian Navy Commander-in-Chief of the Russian NavyГлавнокомандующий ВМФFlag of the Commander-in-Chief of the Russian NavyIncumbentAdmiral Aleksandr MoiseyevActing since March 2024 Russian NavyMember ofGeneral Staff of the Armed ForcesReports toChief of the General StaffAppointerPresident of RussiaFormation8 September 1802 (historical)19 August 1992 (current form)WebsiteOfficial website Navies of Russia Imperial Russian Navy Wrangel...

2021 video game and its franchise Blue ArchiveKey visual of the seriesブルーアーカイブ(Burūākaibu)Created byNexon GamesYostar Video gameDeveloperNexon GamesPublisherCN/JP: Yostar [zh; ja]WW: NexonDirected byKim Yong-ha[1]Music byMitsukiyoKarutNorGenreAction RPGReal-time strategyVisual novelEngineUnityPlatformAndroid, iOSReleasedJP: February 4, 2021WW: November 8, 2021CN: August 3, 2023 Anime television seriesBlue Archive The AnimationDirected ...

US federal government agency Bureau of Economic AnalysisLogo of the Bureau of Economic AnalysisAgency overviewFormedJanuary 1, 1972; 52 years ago (1972-01-01)Preceding agencyOffice of Business EconomicsHeadquartersSuitland, MDEmployees500Annual budget$101 million (FY2019)Agency executivesVipin Arora, DirectorPatricia Abaroa, Deputy Director;Parent departmentDepartment of CommerceWebsitewww.bea.gov The Bureau of Economic Analysis (BEA) of the United States Department of Comme...

Akwa Group S.A.Company typeSociété anonymeFounded1932 (1932)FounderAhmed Ouldhadj AkhannouchHaj Ahmed WakrimHeadquartersCasablanca, MoroccoKey peopleAziz AkhannouchAli WakrimJamal WakrimRevenue $3 billionOwnersAkhannouch and WakrimWebsitewww.akwagroup.com Akwa Group S.A. is a conglomerate company headquartered in Casablanca. The company is active in oil and gas (Afriquia, Afriquia Gaz, Tissir Gaz, Maghreb Oxygène), retail (Mini-Brahim), tourism (Société d’Aménagement et de Promot...

Si ce bandeau n'est plus pertinent, retirez-le. Cliquez ici pour en savoir plus. Certaines informations figurant dans cet article ou cette section devraient être mieux reliées aux sources mentionnées dans les sections « Bibliographie », « Sources » ou « Liens externes » (mai 2015). Vous pouvez améliorer la vérifiabilité en associant ces informations à des références à l'aide d'appels de notes. PsychiatrieDr. Philippe Pinel à l'hôpital de la Salp...

Russian major general This article is about the Russian general. For ice hockey player, see Roman Berdnikov (ice hockey). In this name that follows Eastern Slavic naming customs, the patronymic is Borisovich and the family name is Berdnikov. Roman Borisovich BerdnikovNative nameРоман Борисович БердниковBorn (1974-08-31) 31 August 1974 (age 49)Kamen-na-Obi, Russian SFSR, Soviet UnionAllegiance RussiaYears of service1995–presentRankLieutenant generalC...

Norristown The BoroughMunisipalitas peraturan rumah tanggaKursi KabupatenKotamadya NorristownDistrik Bersejarah Pusat NorristownLokasi Norristown di Montgomery County, Pennsylvania.NorristownLokasi Norristown di PennsylvaniaTampilkan peta PennsylvaniaNorristownNorristown (Amerika Serikat)Tampilkan peta Amerika SerikatKoordinat: 40°07′12″N 75°20′30″W / 40.12000°N 75.34167°W / 40.12000; -75.34167Negara United StatesNegara Bagian AS PennsylvaniaCount...
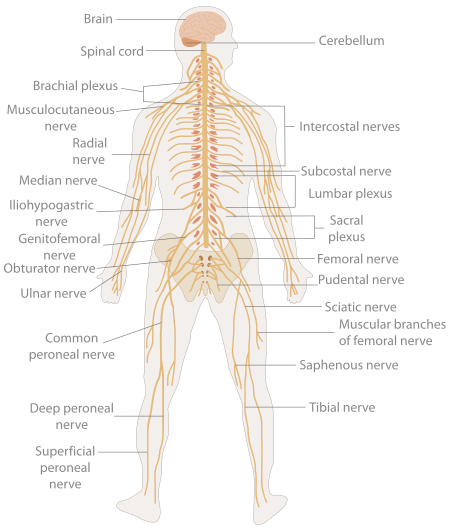
Complex network which connects several biologically relevant entities A biological system is a complex network which connects several biologically relevant entities. Biological organization spans several scales and are determined based different structures depending on what the system is.[1] Examples of biological systems at the macro scale are populations of organisms. On the organ and tissue scale in mammals and other animals, examples include the circulatory system, the respiratory...

Species of endospore forming bacterium Clostridium botulinum Clostridium botulinum stained with gentian violet. Scientific classification Domain: Bacteria Phylum: Bacillota Class: Clostridia Order: Eubacteriales Family: Clostridiaceae Genus: Clostridium Species: C. botulinum Binomial name Clostridium botulinumvan Ermengem, 1896 Clostridium botulinum is a gram-positive,[1] rod-shaped, anaerobic, spore-forming, motile bacterium with the ability to produce botulinum toxin, which is ...

U.S. House district for Indiana Indiana's 6th congressional districtIndiana's 6th congressional district since January 3, 2023RepresentativeGreg PenceR–ColumbusArea5,550.4 sq mi (14,375 km2)Distribution59.23% urban40.77% ruralPopulation (2022)758,725Median householdincome$69,426[1]Ethnicity81.0% White5.3% Hispanic5.3% Asian4.0% Black3.9% Two or more races0.6% otherCook PVIR+19[2] Indiana's 6th congressional district is a congressional district in the U.S. stat...

Japanese football club Football clubMinebea Mitsumi FC ミネベアミツミFCFull nameMinebea Mitsumi Football ClubFounded1964; 60 years ago (1964) as Honda Lock SCStadiumMiyazaki Ikime no Mori Ivy StadiumMiyazakiCapacity11,000OwnerMinebeaMitsumi, Inc.ManagerYosuke MiyajiLeagueJapan Football League202314th of 15WebsiteClub website Home colours Away colours Minebea Mitsumi FC (ミネベアミツミFC, Minebea Mitsumi Efu Shī), formerly Honda Lock SC (ホンダロックSC, H...

Centered figurate number that represents a nonagon with a dot in the center A centered nonagonal number (or centered enneagonal number) is a centered figurate number that represents a nonagon with a dot in the center and all other dots surrounding the center dot in successive nonagonal layers. The centered nonagonal number for n layers is given by the formula[1] N c ( n ) = ( 3 n − 2 ) ( 3 n − 1 ) 2 . {\displaystyle Nc(n)={\frac {(3n-2)(3n-1)}{2}}.} Multiplying the (n - ...


