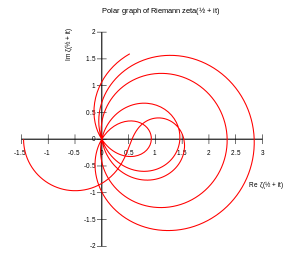
Hàm zeta Riemann
| |||||||||||||||||||||||||||
Read other articles:

Borsa İstanbulJenisBursa efekLokasiSarıyer, Istanbul, TurkiDidirikan26 Desember 1985 (1985-12-26) (sebagai İMKB) 5 April 2013Tokoh pentingTuncay Dinç (CEO)Mata uangLira TurkiEmiten tercatat371 [1]Kapitalisasi pasar$220.620 miliar (2014)[2][3]Volume$1.292 triliun(2012)IndeksBIST 30BIST 50BIST 100Situs webborsaistanbul.com Ekonomi Turki Sejarah ekonomi Turki Uang Lira Turki Bank Sentral Republik Turki Bursa Efek Istanbul Stock Exchange Perusahaan ...

American legislative district Map of Massachusetts Senate's 3rd Essex district, based on the 2010 United States census. Massachusetts Senate's 3rd Essex district in the United States is one of 40 legislative districts of the Massachusetts Senate.[1] It covers portions of Essex county.[2] Democrat Brendan Crighton of Lynn has represented the district since 2018.[3] Locales represented The district includes the following localities:[2] Lynn Lynnfield Marblehead N...

Administration of an estate on death This article is about administration of an estate on death. For other usages, see Administration (disambiguation). This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) The examples and perspective in this article may not represent a worldwide view of the subject. You may improve this article, discuss the issue on the talk page, or create a new article, as a...

Final Piala Liga Inggris 1970TurnamenPiala Liga Inggris 1969–1970 Manchester City West Bromwich Albion 2 1 setelah perpanjangan waktuTanggal7 Maret 1970StadionStadion Wembley, LondonWasitV. James (York)Penonton97.963← 1969 1971 → Final Piala Liga Inggris 1970 adalah pertandingan final ke-10 dari turnamen sepak bola Piala Liga Inggris untuk menentukan juara musim 1969–1970. Pertandingan ini diselenggarakan pada 7 Maret 1970 di Stadion Wembley. Manchester City memenangkan pertan...

Gerakan Angkatan Muda Kristen IndonesiaTanggal pendirian4 November 1945TipeOrganisasi Kemasyarakatan PemudaKantor pusatJL. Salemba Raya 10 Flat. 21 Jakarta 10430, Tlp.: (021) 32726884PendiriPersatuan Pemuda Kristen Indonesia, Majelis Pemuda Kristen Oikumenis & Komisi Pemuda Dewan Gereja IndonesiaSitus webhttp://www.gamki.or.id Gerakan Angkatan Muda Kristen Indonesia atau biasa disingkat GAMKI adalah organisasi pengkaderan yang mempersiapkan anggotanya dalam berbagai bidang pelayanan (pend...

City in Chūbu, JapanŌgaki 大垣市CityFunamachi Port on the Suimon River FlagSealLocation of Ōgaki in Gifu PrefectureŌgaki Coordinates: 35°21′33.7″N 136°36′46.3″E / 35.359361°N 136.612861°E / 35.359361; 136.612861CountryJapanRegionChūbuPrefectureGifuArea • Total206.57 km2 (79.76 sq mi)Population (December 31, 2018) • Total161,539 • Density780/km2 (2,000/sq mi)Time zoneUTC+9 (Japan Stand...

Bandar Udara AlburyTampilan luar aula keberangkatanIATA: ABXICAO: YMAYInformasiJenisPublicPengelolaDewan Kota AlburyMelayaniAlbury, New South Wales, Wodonga, VictoriaKetinggian dpl mdplKoordinat36°04′06″S 146°57′30″E / 36.06833°S 146.95833°E / -36.06833; 146.95833Koordinat: 36°04′06″S 146°57′30″E / 36.06833°S 146.95833°E / -36.06833; 146.95833Situs webSitus web resmiPetaYMAYLokasi di New South WalesLandasan pacu...

Cagliari CalcioCalcio Casteddu, Rossoblù Segni distintivi Uniformi di gara Casa Trasferta Terza divisa Colori sociali Rosso, blu Simboli Quattro mori Inno Tifo Cagliari e boh!!Paolo Migani Dati societari Città Cagliari Nazione Italia Confederazione UEFA Federazione FIGC Campionato Serie A Fondazione 1920 Rifondazione1935 Proprietario Fluorsid Group Presidente Tommaso Giulini Allenatore Claudio Ranieri Stadio Unipol Domus(16 416 posti) Sito web www.cagliaricalcio.com Palmarès Sc...

Texts composed in the Bengali language Bengali literature বাংলা সাহিত্যBengali literatureBy category Bengali languageBengali language authorsChronological list – Alphabetic ListBengali writersWriters – Novelists – PoetsFormsNovel – Poetry – Science FictionInstitutions and awardsLiterary Institutions Literary PrizesRelated Portals Literature Portal India Portal Bangladesh Portalvte This article contains Bengali text. Without proper rendering support, you may s...

State education agency for the U.S. state of Washington Old Capitol building; home to OSPI The Washington State Office of Superintendent of Public Instruction, or OSPI, is the state education agency for the State of Washington. The agency is bound by the Washington State Legislature to implement state laws regarding education, including the 1993 education reform act which mandated the controversial WASL standards based assessment. The Superintendent of Public Instruction is sixth (behind the ...

Velodrome in Rio de Janeiro, Brazil Not to be confused with Barra Velodrome. This article needs to be updated. Please help update this article to reflect recent events or newly available information. (June 2021) Velódromo Municipal do RioAerial view of the Rio Olympic VelodromeLocationBarra da Tijuca, Rio de Janeiro, BrazilCoordinates22°58′28.93″S 43°23′32.04″W / 22.9747028°S 43.3922333°W / -22.9747028; -43.3922333Capacity5,000ConstructionOpened25 July 201...

Questa voce sull'argomento politici francesi è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti del progetto di riferimento. Emmanuel Crétet Ministro dell'interno del Primo Impero FranceseDurata mandato11 agosto 1807 –29 giugno 1809 PredecessoreJean-Baptiste Nompère de Champagny SuccessoreJoseph Fouché Governatore della Banca di Francia del Primo Impero FranceseDurata mandato25 aprile 1806 –9 agosto...

Train carriage Ferdinand Foch, second from right, pictured outside the carriage in Compiègne after agreeing to the armistice that ended World War I. The carriage was later chosen by Nazi Germany as the symbolic setting of Pétain's June 1940 armistice.[1] Left to right: Joachim von Ribbentrop, Wilhelm Keitel, Hermann Göring, Rudolf Hess, Adolf Hitler, Erich Raeder partially obscured and Walther von Brauchitsch in front of the Armistice carriage The Compiègne Wagon was the train car...

City in South Dakota, United StatesCanton, South DakotaCityMotto: Can Live. Can Dream. Can Do!Location in Lincoln County and the state of South DakotaCoordinates: 43°18′12″N 96°35′06″W / 43.30333°N 96.58500°W / 43.30333; -96.58500CountryUnited StatesStateSouth DakotaCountyLincolnIncorporated1881[1]Government • TypeCouncil-ManagerArea[2] • Total3.29 sq mi (8.51 km2) • Land3.27 sq&#...

Building in New York City, New YorkJudge BuildingFormer namesGoelet BuildingGeneral informationLocation110 Fifth AvenueManhattan, New York City, New YorkCoordinates40°44′15″N 73°59′34″W / 40.73750°N 73.99278°W / 40.73750; -73.99278Completed1888Height147 feet (45 m)Technical detailsFloor count10Design and constructionArchitect(s)McKim, Mead & WhiteReferences[1] The Judge Building, originally the Goelet Building, is a ten-story edifice built...

Peter CushingOBECushing sebagai Victor Frankenstein dalam The Revenge of FrankensteinLahir(1913-05-26)26 Mei 1913Kenley, Surrey, InggrisMeninggal11 Agustus 1994(1994-08-11) (umur 81)Canterbury, Kent, InggrisPendidikanShoreham CollegeAlmamaterGuildhall School of Music and DramaPekerjaanAktorTahun aktif1935–1991Suami/istriViolet Helene Beck (m. 1943; wafat 1971)PenghargaanBritish Academy Television Award for Best Actor (1956) Pete...

Questa voce sull'argomento competizioni ciclistiche è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti del progetto di riferimento. Il podio dell'edizione 1979: da sinistra, Jan Jankiewicz, Gianni Giacomini e Bernd Drogan La gara in linea maschile Dilettanti era una delle prove disputare durante i campionati del mondo di ciclismo su strada. Riservata ai ciclisti non professionisti, si svolse dal 1921 fino al 1995, risultando per ques...

Bank in China This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Bank of Communications – news · newspapers · books · scholar · JSTOR (November 2007) (Learn how and when to remove this message) Bank of Communications Co., Ltd.Bocom Financial Towers, the bank's head office in ShanghaiNative name交通银行股�...

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (أبريل 2018) Phospholipase A2 receptor 1, 180kDaالمعرفاتالرمز، (أو الرموز) PLA2R1; CLEC13C; PLA2-R; PLA2G1R; PLA2IR; PLA2Rمعرفات خارجية OMIM: 604939 MGI: 102468 هومولوجين: 32016 بطاقات الجينات: PLA2R1 Gene علم الوجود ا...

لويك دوفال (بالفرنسية: Loïc Duval) معلومات شخصية الميلاد 12 يونيو 1982 (42 سنة) شارتر مواطنة فرنسا الحياة العملية المهنة سائق سيارة سباق[1] اللغات الفرنسية الرياضة سباقات السيارات المواقع الموقع الموقع الرسمي تعديل مصدري - تعديل لويك دوفال (با...