Mínimos cadrados lineais
|
Read other articles:

Koordinat: 8°05′12″S 115°08′00″E / 8.086699°S 115.133276°E / -8.086699; 115.133276 SangsitDesaTentara kolonial Belanda di Sangsit pada masa Perang Bali gambar oleh G. Kepper (G.Kepper: Wapenfeiten van het Nederlandsch-Indisch leger)Negara IndonesiaProvinsiBaliKabupatenBulelengKecamatanSawanKode pos81171Kode Kemendagri51.08.07.2012Luas3,60 km²[1]Jumlah penduduk10.077 jiwa(2000) 7.726 jiwa (2010)[2]Kepadatan2.146 jiwa/km² (2010)Jumlah R...

TembelangKecamatanPeta lokasi Kecamatan TembelangNegara IndonesiaProvinsiJawa TimurKabupatenJombangPemerintahan • CamatAgus SantosoPopulasi • Total50,794 jiwaKode Kemendagri35.17.13 Kode BPS3517150 Luas32,94 km²Desa/kelurahan15 Tembelang adalah sebuah kecamatan di Kabupaten Jombang, Jawa Timur, Indonesia. Pusat pemerintahan Kecamatan Tembelang saat ini terletak di desa Sentul. Sebelumnya, pusat pemerintahan terletak di desa Pesantren yang kini telah beralih fungs...

Terminal BunderTerminal penumpang tipe BKenampakan unit bus Trans Jatim dan beberapa unit bus antarkota terparkir di peron Terminal Bunder (2022).LokasiJalan Raya Lamongan Bunder, Dusun Banjai Sari, Desa Banjarsari, Kecamatan Cerme, Kabupaten Gresik, Provinsi Jawa Timur, Kodepos 61171 IndonesiaKoordinat7°10′12″S 112°35′4″E / 7.17000°S 112.58444°E / -7.17000; 112.58444Koordinat: 7°10′12″S 112°35′4″E / 7.17000°S 112.58444°E&#x...

Palais Schaumburg di Bonn Pemandangan daerah Palais Schaumburg Palais Schaumburg Palais Schaumburg adalah sebuah bangunan bergaya neoklasik di Bonn, Jerman yang menjadi pusat sekunder Kantor Kanselir Federal Jerman dan pusat utama resmi Kanselir Republik Federal Jerman dari tahun 1949 sampai tahun 1999. Sebagai pusat dari Kekanseliran Federal, Palais secara ringkas dikenal sebagai Rumah Kanselir Federal (Jerman: Haus des Bundeskanzlerscode: de is deprecated ). Sejak tahun 1999, Palais Schaumb...

Kakatua filipina Cacatua haematuropygia In Palawan, PhilippinesStatus konservasiTerancam kritisIUCN22684795 TaksonomiKerajaanAnimaliaFilumChordataKelasAvesOrdoPsittaciformesFamiliCacatuidaeGenusCacatuaSpesiesCacatua haematuropygia DistribusiEndemikFilipina lbs Kakatua Filipina atau Red-vented cocktaoo dengan nama ilmiah Cacatua haematuropygia merupakan spesies kakatua endemik di Filipina yang paling terancam populasinya. Sekilas bentuk dan ukuran burung ini mirip dengan Kakatua Tanimbar (Caca...

Синелобый амазон Научная классификация Домен:ЭукариотыЦарство:ЖивотныеПодцарство:ЭуметазоиБез ранга:Двусторонне-симметричныеБез ранга:ВторичноротыеТип:ХордовыеПодтип:ПозвоночныеИнфратип:ЧелюстноротыеНадкласс:ЧетвероногиеКлада:АмниотыКлада:ЗавропсидыКласс:Пт�...

Gempa bumi Taiwan 20242024年4月花莲地震Bangunan sembilan lantai roboh di Kota HualienWaktu UTC2024-04-02 23:58:11ISC637103828USGS-ANSSComCatTanggal setempat3 April 2024Waktu setempat07:58:11 NSTKekuatan7.4 MwKedalaman34,8 km (22 mi)Episentrum23°49′08″N 121°33′43″E / 23.819°N 121.562°E / 23.819; 121.562dekat Kota Hualien, Hualien, TaiwanJenisSesar NaikWilayah bencanaTaiwanIntensitas maks.VIII (Parah)CWB 6+ TsunamiYa (45 cm di I...

Ini adalah nama Korea; marganya adalah Kim. Kim Hae-sookKim Hae-sook bulan Maret 2019Lahir30 Desember 1955 (umur 68)Busan, Korea SelatanAlmamaterUniversitas Kyung HeePekerjaanAktrisTahun aktif1975–sekarangNama KoreaHangul김해숙 Hanja金海淑 Alih AksaraGim Hae-sukMcCune–ReischauerKim Hae-suk Kim Hae-sook (Hangul: 김해숙; lahir 30 Desember 1955) merupakan seorang aktris Korea Selatan.[1] Ia dikenal sebagai aktris dalam film My Brother (2004), Open City (20...

Pertempuran-pertempuran BergiselBagian dari Perang Koalisi KelimaPatung Andreas Hofer di dekat Bergisel di InnsbruckTanggal12 April – 1 November 1809LokasiBergisel, selatan Innsbruck, AustriaHasil Pada akhirnya dimenangkan Prancis/BayernPihak terlibat Pemberontak Tirol Kerajaan Bayern PrancisTokoh dan pemimpin Andreas Hofer Josef Speckbacher Peter Mayr Joachim Haspinger Martin Teimer François Lefebvre Bernhard Deroy Karl von Wrede General Rechberg Jean Drouet d'ErlonKekuatan 5.000 5....

A Simple Response to an Elemental MessageThe logo of A Simple ResponseDate20:00 UTC, 10 October 2016Duration434 light year journeyLocationCebreros Station (DSA 2)Coordinates40°27′9.68″N 4°22′3.18″W / 40.4526889°N 4.3675500°W / 40.4526889; -4.3675500Typeinterstellar transmissionOrganised byUniversity of Edinburgh, UK European Space Agency, DE UK Astronomy Technology Centre; Royal Observatory Edinburgh, UK European Southern Observatory, DE Johns Hopkins Unive...

For the television station licensed to Kodiak, Alaska, KMXT-LP, see AlaskaOne. This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: KMXT FM – news · newspapers · books · scholar · JSTOR (March 2024) (Learn how and when to remove this message) Radio station in Kodiak, AlaskaKMXTKodiak, AlaskaBroadcast areaSou...

Johannesburg is the economic capital of South Africa. This list of tallest buildings in Johannesburg ranks all completed buildings by height in the city of Johannesburg, which is the largest city in South Africa. Johannesburg is classified as a megacity,[1] and is one of the 100 largest urban areas in the world. Tallest buildings The Southern Life Centre. This list ranks Johannesburg buildings that stand at least 100 m (328 ft) tall, based on standard height measurement. Th...

إرفين ويلهلم مولر (بالألمانية: Erwin Wilhelm Müller) معلومات شخصية الميلاد 13 يونيو 1911 [1][2] برلين[3] الوفاة 17 مايو 1977 (65 سنة) [1][2] واشنطن العاصمة[3] مواطنة ألمانيا الولايات المتحدة (1962–)[4] عضو في الأكاديمية الوطنية للعلوم، والأكاديم�...

For other places with the same name, see Valencia (disambiguation). Place in Carabobo, VenezuelaValenciaNuestra Señora de la Asunción de la Nueva Valencia del ReyTop to bottom, left to right:Panoramic View of Valencia; Urb Prebo Park; Bolívar Square of Valencia; Panoramic View of the south of Valencia; Street in Valencia; Municipal Theatre of Valencia; Arch of Carabobo; Metrópolis Mall FlagEtymology: Valencia de Don JuanNickname(s): Capital industrial de Venezuela,La pequeña Detroit...

Leading member of the Dominican Order BlessedRaymond of CapuaO.P.Master General of the Order of PreachersBornca. 1303[1]Capua, Kingdom of NaplesDied5 October 1399 (aged 96)Nuremberg, Holy Roman EmpireVenerated inRoman Catholic ChurchBeatified15 May 1899, Saint Peter's Basilica, Kingdom of Italy by Pope Leo XIIIMajor shrineChurch of San Domenico Maggiore, Naples, ItalyFeast5 OctoberAttributesDominican habit Legenda maior sanctae Catharinae Senensis, 1477 La vita di Santa Caterina ...

Disambiguazione – Se stai cercando il gruppo musicale, vedi Matrioska (gruppo musicale). Matrioska aperta La matrioska[1][2][3] o matriosca[1][2][4] (in russo матрёшка?, matrëška ascoltaⓘ; al plurale matrioske o matriosche) è un insieme di bambole cave in legno di grandezza variabile, ciascuna delle quali è inseribile in quella immediatamente più grande. Uno tra i più iconici elementi della cultura popolare della Russia, ...

Gargantua and Pantagruel redirect here. For other uses, see Gargantua (disambiguation) and Pantagruel (ensemble). 16th-century novels by François Rabelais The Five Books of the Lives and Deeds of Gargantua and PantagruelTitle-page of a c. 1532 edition of PantagruelPantagruel (c. 1532)Gargantua (1534)The Third Book of Pantagruel (1546)The Fourth Book of Pantagruel (1552)The Fifth Book of Pantagruel (c. 1564)AuthorFrançois Rabelais (Alcofribas Nasier)Original titleLes Cinq l...

Wahlkreis 10: Favoriten Staat Österreich Bundesland Wien Wahlkreisnummer 10 Anzahl der Mandate 10 Einwohner 220.324 (1. Jänner 2024)[1] Wahlberechtigte 110.710 (2015)[2] Wahldatum 11. Oktober 2015 Wahlbeteiligung 70,27 % (2015)[2] Abgeordnete Stefan Berger (FPÖ) Nemanja Damnjanovic (FPÖ) Peter Florianschütz (SPÖ) Christian Hursky (SPÖ) Günter Koderhold (FPÖ) Martina Ludwig-Faymann (SPÖ) Michael Stumpf (...
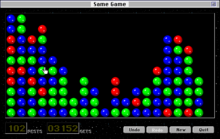
Video game genre For the video game named Puzzle, see Puzzle (American Video Entertainment). A 1994 Mac version of the 1985 tile-matching puzzle video game Chain Shot! Part of a series onPuzzles Types Guessing Riddle Situation Logic Dissection Induction Logic grid Self-reference Mechanical Combination Construction Disentanglement Lock Go problems Folding Stick Tiling Tour Sliding Chess Maze (Logic maze) Word and Number Crossword Sudoku Puzzle video games Mazes Metapuzzles Topics Brain te...

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (سبتمبر 2023) الحسينيون أو حسينيون هم فرع من العلويين الذين ينحدرون من نسل الحسين بن علي، حفيد النبي محمد. وهم يشكلون، �...

![{\displaystyle \|A\mathbf {x} -\mathbf {b} \|^{2}=\left([A\mathbf {x} ]_{1}-\mathbf {b} _{1}\right)^{2}+\left([A\mathbf {x} ]_{2}-\mathbf {b} _{2}\right)^{2}+\dots +\left([A\mathbf {x} ]_{n}-\mathbf {b} _{n}\right)^{2},}](https://wikimedia.org/api/rest_v1/media/math/render/svg/92ab91bdfcfe0feefd14528407064aa40df4bdd0)

















