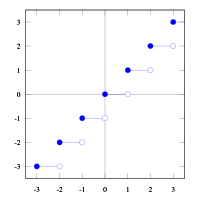
Funcións chan e teito
|
Read other articles:

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada November 2022. Jill Bolte TaylorJill Bolte Taylor berbicara di TED pada 27 Februari 2008Lahir1959Louisville, KentuckyTempat tinggal Bloomington, IndianaAlmamaterSMA Terre Haute South Vigo, B.A. Universitas Indiana, Ph.D. Universitas Negeri Indiana, Postdoktoral di S...

Any additive color space based on the RGB color model This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: RGB color spaces – news · newspapers · books · scholar · JSTOR (December 2014) (Learn how and when to remove this template message) 1931 CIE chromaticity diagram showing some RGB color spaces as defined by t...

العلاقات المالديفية الدومينيكية جزر المالديف دومينيكا المالديف دومينيكا تعديل مصدري - تعديل العلاقات المالديفية الدومينيكية هي العلاقات الثنائية التي تجمع بين المالديف ودومينيكا.[1][2][3][4][5] مقارنة بين البلدين هذه مقارنة عامة ومرجعي�...

كلينتوندال الإحداثيات 41°41′40″N 74°02′48″W / 41.694548°N 74.046639°W / 41.694548; -74.046639 [1] تقسيم إداري البلد الولايات المتحدة[2] خصائص جغرافية المساحة 14.534144 كيلومتر مربع14.534136 كيلومتر مربع (1 أبريل 2010)[3] ارتفاع 166 متر عدد السكان عدد السكا�...

Partai Liberal Kanada Parti libéral du CanadaSingkatanLPC (bahasa Inggris)PLC (bahasa Prancis)Ketua umumJustin TrudeauPresidenSuzanne CowanDibentuk1861; 162 tahun lalu (1861)[a]IdeologiLiberalisme (Kanada)Liberalisme sosial[1]Afiliasi internasionalLiberal InternationalSitus webliberal.ca Partai Liberal Kanada (bahasa Inggris: Liberal Party of Canada, bahasa Prancis: Parti libéral du Canada) adalah sebuah partai politik beraliran tengah sampai kiri tengah di Kanada....

Species of virus Human metapneumovirus Human metapneumovirus (hMPV) structure and genome Virus classification (unranked): Virus Realm: Riboviria Kingdom: Orthornavirae Phylum: Negarnaviricota Class: Monjiviricetes Order: Mononegavirales Family: Pneumoviridae Genus: Metapneumovirus Species: Human metapneumovirus Human metapneumovirus (HMPV or hMPV) is a negative-sense single-stranded RNA virus of the family Pneumoviridae[1] and is closely related to the Avian metapneumovirus (AMPV) sub...

Hardy HartonoLahir16 November 1983 (umur 40)Barnet, London, InggrisKebangsaanIndonesiaAlmamaterUniversitas TrisaktiPekerjaanPemeranTahun aktif2006—sekarang Hardy Hartono (lahir 16 November 1983) adalah seorang pemeran Indonesia.[1][2] Kehidupan awal Hardy lahir di London, Inggris dan tumbuh serta besar di kota Barnet. Ia kemudian pindah ke Jakarta, Indonesia. Hardy merupakan lulusan SMU Negeri 34 Jakarta dan selanjutnya menempuh kuliah di Universitas Trisakti.[...

American admiral and diplomat (1925–2007) This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: William J. Crowe – news · newspapers · books · scholar · JSTOR (November 2022) (Learn how and when to remove this template message) William Crowe60th United States Ambassador to the United Kingdom In officeJune 2, 199...

1936 American film directed by Fred Guiol Silly BilliesDirected byFred GuiolScreenplay byAl BoasbergJack TownleyStory byThomas LennonFred GuiolProduced byLee MarcusStarringBert WheelerRobert WoolseyDorothy LeeCinematographyNick MusuracaJ. Roy HuntEdited byJohn LockertMusic byRoy WebbProductioncompanyRKO Radio PicturesDistributed byRKO Radio PicturesRelease date March 20, 1936 (1936-03-20)[1] Running time65 minutesCountryUnited StatesLanguageEnglish Silly Billies is a 19...

For other uses of Finger Lake, see Finger Lake (disambiguation). Group of lakes in New York, United States Finger LakesSatellite view of the Finger Lakes region in late fall. Lake Ontario at the top left.LocationNew YorkCoordinates42°40′N 76°50′W / 42.667°N 76.833°W / 42.667; -76.833TypeFinger lakesPart ofLake Ontario BasinPrimary outflowsOswego RiverBasin countriesUnited StatesSettlementsIthaca, Geneva, Canandaigua The eleven Finger Lakes of New York...

Study of the nature of deities and religious beliefs Not to be confused with Religious studies. This article is about theology as a science. For Sinéad O'Connor's album, see Theology (album). For the academic journal, see Theology (journal). Part of a series onTheism Types of faith Agnosticism Apatheism Atheism Classical theism Deism Henotheism Ietsism Ignosticism Monotheism Monism Dualism Monolatry Kathenotheism Omnism Pandeism Panentheism Pantheism Polytheism Transtheism Specific conceptio...

For broader coverage of this topic, see Plate theory. Deformation of a thin plate highlighting the displacement, the mid-surface (red) and the normal to the mid-surface (blue) The Kirchhoff–Love theory of plates is a two-dimensional mathematical model that is used to determine the stresses and deformations in thin plates subjected to forces and moments. This theory is an extension of Euler-Bernoulli beam theory and was developed in 1888 by Love[1] using assumptions proposed by Kirch...

American media website about technology and consumer electronics News.com redirects here. For the Australian news website, see news.com.au. CNETScreenshotType of businessSubsidiaryType of siteTechnology, newsAvailable inEnglish, French, JapaneseCreated by Halsey Minor Shelby Bonnie Editor Lindsey Turrentine Connie Guglielmo IndustryJournalismParentCBS Interactive (2008–2020)Red Ventures (2020–present)URLcnet.comCommercialYesRegistrationOptionalLaunched 1992; 32 year...

Iranian poet and scholar This article includes a list of general references, but it lacks sufficient corresponding inline citations. Please help to improve this article by introducing more precise citations. (May 2017) (Learn how and when to remove this message) Mohammad-Taqi Baharمحمدتقی بهارBorn10 December 1886Mashhad, IranDied22 April 1951(1951-04-22) (aged 64)Tehran, IranOccupationPoet, politician and journalistLiterary movementPersian literatureNotable worksTārikh-e Sist...

محمد عبد الله حسن محمد معلومات شخصية الميلاد 2 ديسمبر 1978 (46 سنة) دبي مواطنة الإمارات العربية المتحدة الحياة العملية المهنة حكم كرة قدم الرياضة كرة القدم[1] بلد الرياضة الإمارات العربية المتحدة تعديل مصدري - تعديل محمد عبد الله حسن محمد (مواليد ...

لمعانٍ أخرى، طالع فيرفيلد (توضيح). فيرفيلد الإحداثيات 44°48′52″N 72°55′35″W / 44.814444444444°N 72.926388888889°W / 44.814444444444; -72.926388888889 [1] تقسيم إداري البلد الولايات المتحدة[2] التقسيم الأعلى مقاطعة فرانكلين خصائص جغرافية المساحة 177.4 كيلومتر م...

Arjen RobbenRobben con il Bayern Monaco nel 2019Nazionalità Paesi Bassi Altezza180 cm Peso80 kg Calcio RuoloAllenatore (ex attaccante) Termine carriera15 luglio 2021 - giocatore CarrieraGiovanili 1989-1996 VV Bedum1996-2000 Groningen Squadre di club1 2000-2002 Groningen46 (8)2002-2004 PSV56 (17)2004-2007 Chelsea67 (15)2007-2009 Real Madrid50 (11)2009-2019 Bayern Monaco201 (99)2020-2021 Groningen6 (0)[1] Nazionale 1999 Paesi Bassi U-151 (0)1999-...

Second Battle of TabascoPart of Mexican–American WarAmerican landing in San Juan Bautista (Villahermosa today) during the Second Battle of TabascoDateJune 15–16, 1847LocationVillahermosa, TabascoResult American victoryBelligerents United States MexicoCommanders and leaders Matthew C. Perry David D. Porter Domingo Echagaray Claro HidalgoStrength 8 vessels1,050 U.S. Marines[1]: 340 7 field artillery pieces 600+ Soldiers[1]: 341 Casualtie...

Payments by Haiti to France in compensation for property lost in the Haitian Revolution The Baron de Mackau of France presenting demands to Jean-Pierre Boyer, President of Haiti, in 1825 The Haiti Independence Debt involves an 1825 agreement between Haiti and France that included France demanding an indemnity of 150 million francs to be paid by Haiti in claims over property – including Haitian slaves – that was lost through the Haitian Revolution in return for diplomatic recognition, with...

Военные награды Италии — награды Италии предназначенные для награждения военнослужащих Италии и иных государств. Содержание 1 Военный орден Италии 2 Медали «За воинскую доблесть» 3 Медаль «За гражданскую доблесть» 4 Кресты «За воинскую доблесть» 5 Медаль «За гражданс...






![{\displaystyle {\begin{aligned}\lfloor x\rfloor +\lfloor y\rfloor &\leq \lfloor x+y\rfloor \leq \lfloor x\rfloor +\lfloor y\rfloor +1,\\[3mu]\lceil x\rceil +\lceil y\rceil -1&\leq \lceil x+y\rceil \leq \lceil x\rceil +\lceil y\rceil .\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c51c4db96365020b998169d24d9df06a4db2b74d)

















