W Series 2020
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Read other articles:

Aleksei Ionov Ionov berseragam Dynamo Moscow pada tahun 2013Informasi pribadiNama lengkap Aleksei Sergeyevich IonovTanggal lahir 18 Februari 1989 (umur 35)Tempat lahir Kingisepp, RusiaTinggi 1,77 m (5 ft 9+1⁄2 in)Posisi bermain GelandangInformasi klubKlub saat ini Dynamo MoscowNomor 99Karier junior–2007 Zenit St. PetersburgKarier senior*Tahun Tim Tampil (Gol)2007–2011 Zenit St. Petersburg 57 (8)2012–2013 Kuban Krasnodar 37 (3)2013 Anzhi Makhachkala 6 (0)2013�...

Tamara DudaNama asalТамара ДудаLahir5 Januari 1976 (umur 48)Kyiv, RSS UkrainaWarga negaraUkrainaAlmamaterTaras Shevchenko University of KyivPekerjaanPenulisKarier menulisNama penaTamara Horikha ZernyaBahasaBahasa UkrainaGenreFiksiKarya terkenalDaughter (Доця)PenghargaanShevchenko National PrizeTahun aktif2019-sekarangPasanganSvyatoslav BoykoAnak3 Tamara Anatoliyivna Duda (Ukrainian: Тамара Анатоліївна Дудаcode: uk is deprecated ; la...

Lambang kota La Louvière La Louvière merupakan sebuah kota di Belgia. Kota ini letaknya di bagian selatan. Tepatnya di region Walonia. Pada tahun 2010, kota ini memiliki jumlah penduduk sebesar 78.071 jiwa dan memiliki luas wilayah 64,24 km². Kota ini memiliki angka kepadatan penduduk 1.200 jiwa/km². Pranala luar Wikimedia Commons memiliki media mengenai La Louvière. Situs resmi Site of the Centre region Diarsipkan 2007-01-18 di Wayback Machine., in French Photographies of the Duferco st...

Voce principale: Rovigo Calcio. Rovigo CalcioStagione 2006-2007Sport calcio Squadra Rovigo Allenatore Carmine Parlato Presidente Francesco Scerra Serie C26º posto nel girone B Coppa Italia Serie CPrimo turno Maggiori presenzeCampionato: Rizzi (32) Miglior marcatoreCampionato: Gasparello (13) StadioStadio Francesco Gabrielli 1949-1950 2007-2008 Si invita a seguire il modello di voce Questa pagina raccoglie le informazioni riguardanti il Rovigo Calcio nelle competizioni ufficiali della s...

Irene AngelinaIrene dan Philipp, di dalam sebuah lukisan dinding yang berasal dari abad ke-16 di Gereja Biara LorchPermaisuri SisiliaBerkuasa1193Permaisuri JermanBerkuasa1198–1208Informasi pribadiKelahiranskt. 1181Konstantinopel, Kekaisaran Romawi TimurKematian27 August 1208 – 1181; umur -28–-27 tahunKastil Hohenstaufen, Kekaisaran Romawi SuciPemakamanBiara LorchWangsaWangsa AngelosAyahIsaakius II AngelusPasanganRuggero III, Raja SisiliaPhilipp, Raja JermanAnakBeatrixMariaK...

Azis Mugni Inspektur Jenderal Departemen PerhubunganMasa jabatanAgustus 1986 – 1 November 1991MenteriRusmin NuryadinAzwar AnasPendahuluMoerwaniPenggantiMuslim Massewa Karier militerPihak IndonesiaDinas/cabang TNI Angkatan DaratMasa dinas1956—?Pangkat Mayor Jenderal TNINRP18320SatuanZeni (CZI)Sunting kotak info • L • B Mayor Jenderal TNI (Purn.) Abdul Azis Mugni adalah seorang perwira angkatan darat dan birokrat dari Indonesia. Ia menjabat sebagai Inspektur...

Stannocene Names IUPAC name StannoceneBis(η5-cyclopentadienyl)tin(II) Other names Bis(cyclopentadienyl)tinDi(cyclopentadienyl)tin Identifiers CAS Number 1294-75-3[1] 3D model (JSmol) Interactive image InChI InChI=1S/2C5H5.Sn/c2*1-2-4-5-3-1;/h2*1-5H;/q2*-1;+2Key: CRQFNSCGLAXRLM-UHFFFAOYSA-N SMILES [cH-]1cccc1.[cH-]1cccc1.[Sn+2] Properties Chemical formula C10H10Sn Molar mass 248.900 g·mol−1 Structure[2] Crystal structure orthorhombic Space group Pbcm, No.5...

Asfar ibn Shiruya (Gilaki/Persian: اسفار بن شیرویه: died 931) was an Iranian military leader of Gilaki origin, active in northern Iran (esp. Tabaristan and Jibal) in the early 10th century. He played a major role in the succession disputes of the Alids of Tabaristan, and managed to establish himself as the ruler of Tabaristan and northern Jibal briefly from 928 to 930. Name Asfār is a local Caspian form of Middle Persian aswār, which means rider, cavalryman. The New Persian for...

American daily newspaper The neutrality of this article is disputed. Relevant discussion may be found on the talk page. Please do not remove this message until conditions to do so are met. (October 2014) (Learn how and when to remove this message) The Herald-SunTypeDaily newspaperFormatBroadsheetOwner(s)The McClatchy Company[1]PublisherSara Glines[2]EditorRobyn Tomlin[2]Founded1853: The Durham Morning Herald[3] 1889: The Durham Sun[4][5]1991: Th...

American streaming news channel Television channel CBS News 24/7CountryUnited StatesBroadcast areaWorldwideNetworkCBS NewsHeadquartersCBS Broadcast Center Manhattan, New York City, New York, United StatesProgrammingLanguage(s)EnglishPicture format1080i (HD)Downscaled to:540p360p144pOwnershipOwnerParamount GlobalParentParamount StreamingSister channels List Nickelodeon Nick Jr. Channel Nicktoons TeenNick NickMusic CBS CBS Sports Network CBS Sports HQ CBS Sports Golazo Network MTV MTV2 MTV Tres...

For the Indian Telugu-language comedy film, see Pelli Sandadi (1959 film). For the Indian Telugu-language musical romantic comedy, see Pelli SandaD. 1996 Indian filmPelli SandadiDVD CoverDirected byK. Raghavendra RaoWritten byK. Raghavendra RaoSatyanand (dialogue)Produced byC. Aswani DuttAllu AravindStarringSrikanthRavaliDeepti BhatnagarCinematographyV. JayaramEdited byMarthand K. VenkateshMusic byM. M. KeeravaniProductioncompanySri Raghavendra Movie CorporationDistributed byGeetha ArtsReleas...

Mt Gravatt Bus ServiceMt Gravatt Bus Service Custom Coaches bodied Volvo B7RLE in January 2015Service areaSouth East BrisbaneService typeBus & coach operatorRoutes3HubsWestfield Mt GravattDepotsBurbankUnderwoodFleet48 (December 2022)[1]Websitewww.mtgcoach.com.au Mt Gravatt Bus Service is an Australian operator of bus services in South East Brisbane. It operates three services under contract to the Queensland Government under the Translink banner. History Originally named Burbank ...

Julie Williams redirects here. For the scientist, see Julie Williams (scientist). Soap opera character Julie Olson WilliamsDays of Our Lives characterSusan Seaforth Hayes as Julie Olson WilliamsPortrayed by Charla Doherty (1965–1966) Catherine Dunn (1967) Catherine Ferrar (1967–1968) Susan Seaforth Hayes (1968–present) Duration 1965–1984 1990–1994 1996 1999–present First appearanceNovember 8, 1965 (November 8, 1965)ClassificationPresent; recurringCreated byPegg...

محمد طاھر القادري (بالإنجليزية: Tahir Qadri) معلومات شخصية الميلاد 19 فبراير 1951جھنگ باکستان الإقامة كندا (2006–) مواطنة باكستان[1][2] كندا[3] العقيدة مسلم الحياة العملية الحقبة النصف الثانى من القرن الواحد والعشرين حتى الان المدرسة الأم جامعة البنجاب المه...
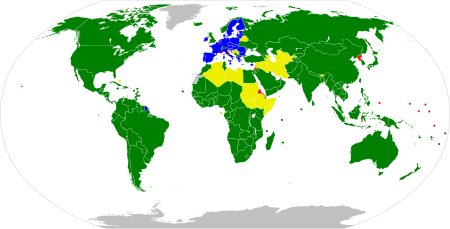
منظمة التجارية العالميةWorld Trade Organization منظمة التجارة العالمية أعضاء أعضاء ممثلين بشكل مزدوج من قبل الاتحاد الأوروبي مراقبون دول غير مشاركة الاختصار WTO البلد سويسرا المقر الرئيسي جنيف، سويسرا تاريخ التأسيس 1 كانون الثاني / يناير 1995 الوض...

New Zealand politician This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Clem Simich – news · newspapers · books · scholar · JSTOR (March 2024) (Learn how and when to remove this message) The HonourableClem SimichQSOSimich in 2009Member of the New Zealand Parliamentfor TāmakiIn office1992–2005Preceded bySir...

Women's VL2 at the 2019 ICF Canoe SprintWorld ChampionshipsVenueOlympic Centre of SzegedLocationSzeged, HungaryDates21–22 AugustCompetitors11 from 9 nationsWinning time56.10Medalists Emma Wiggs Great Britain Susan Seipel Australia Maria Nikiforova Russia← 20182021 → 2019 ICF Canoe SprintWorld ChampionshipsCanadian eventsC-1 200mmenwomenC-1 500mmenwomenC-1 1000mmenC-1 5000mmenwomenC-2 200mmenw...

Surface drawn by a moving line passing through a fixed point An elliptic cone, a special case of a conical surface In geometry, a conical surface is a three-dimensional surface formed from the union of lines that pass through a fixed point and a space curve. Definitions A (general) conical surface is the unbounded surface formed by the union of all the straight lines that pass through a fixed point — the apex or vertex — and any point of some fixed space curve — the directri...

Big tent political party in Brazil This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Brazilian Democratic Movement – news · newspapers · books · scholar · JSTOR (November 2012) (Learn how and when to remove this message) Brazilian Democratic Movement Movimento Democrático BrasileiroAbbreviationMDBPresidentBal...
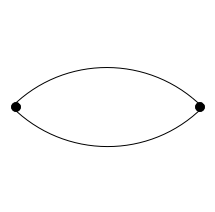
In graph theory, edges incident/directed between the same vertices Multiple edges joining two vertices. In graph theory, multiple edges (also called parallel edges or a multi-edge), are, in an undirected graph, two or more edges that are incident to the same two vertices, or in a directed graph, two or more edges with both the same tail vertex and the same head vertex. A simple graph has no multiple edges and no loops. Depending on the context, a graph may be defined so as to either allow or ...