Read other articles:

Nyi Hadjar Dewantara Nyi Hajar Dewantara, Nyi Hadjar Dewantara atau Nyi Hajar Dewantoro (14 September 1890 – 16 April 1971)[1] adalah seorang pegiat pendidikan dan pergerakan perempuan Indonesia. Ia bernama lahir Raden Ajeng Sutartinah sebagai putri keenam dari pasangan Kanjeng Pangerah Haryo (KPH) Sosroningrat putra K.P.A.A Pakualam III dan R.A.Y. Mutmainah yang setelah bersuami bernama B.R.A.Y. Sosroningrat putri dari K.R.T. Mertonegoro II.[1] R.A. Sutartinah...

Chiang Ching-kuo 蔣經國 Presiden Republik TiongkokMasa jabatan20 Mei 1978 – 13 Januari 1988Wakil PresidenHsieh Tung-ming (1978 - 1984) Lee Teng-hui (1984 - 1988) PendahuluYen Chia-kanPenggantiLee Teng-huiPerdana Menteri Republik ChinaMasa jabatan29 Mei 1972 – 20 Mei 1978PresidenChiang Kai-shekYen Chia-kan PendahuluYen Chia-kanPenggantiSun Yun-suanKetua Kuomintang ke-1Masa jabatan5 April 1975 – 13 Januari 1988 PendahuluChiang Kai-shek (Direktur-Jenderal Kuom...

Untuk kegunaan lain, lihat Osaka. Prefektur Osaka 大阪府PrefekturTranskripsi Jepang • Jepang大阪府 • RōmajiŌsaka-fu BenderaLambangKoordinat: 34°41′11″N 135°31′12″E / 34.68639°N 135.52000°E / 34.68639; 135.52000Koordinat: 34°41′11″N 135°31′12″E / 34.68639°N 135.52000°E / 34.68639; 135.52000NegaraJepangWilayahKansaiPulauHonshuIbu kotaOsakaPemerintahan • GubernurHirohumi Yoshi...

PT Zurich Topas LifeJenisJasa keuanganDidirikanJakarta, Indonesia (1986)Kantorpusat Jakarta, IndonesiaTokohkunciChristopher Franz Bendl Presiden DirekturPemilikZurich Insurance Group Mayapada GroupSitus webwww.zurich.co.id Zurich Topas Life atau biasa disebut dengan Zurich Life Indonesia atau Zurich Life adalah perusahaan asuransi jiwa yang berdiri sejak 1986 dan berkantor pusat di Jakarta. Perusahaan sebelumnya bernama Century Lifindo Perdana sejak berdiri hingga tahun 2009. K...

Camila MendesMendes di San Diego Comic-Con 2019LahirCamila Carraro Mendes29 Juni 1994 (umur 29)[1]Charlottesville, Virginia, Amerika SerikatAlmamaterUniversitas New YorkPekerjaanAktrispenyanyiTahun aktif2016–sekarang Camila Carraro Mendes[2] (lahir 29 Juni 1994)[3] adalah seorang aktris asal Amerika Serikat. Dia memulai kariernya dengan memerankan Veronica Lodge di serial drama remaja CW, Riverdale (2017–sekarang), di mana dia memenangkan Teen Choice Awa...
Canadian journalist Christie BlatchfordBlatchford on November 21, 2008BornChristie Marie Blatchford[1](1951-05-20)May 20, 1951[2]Rouyn-Noranda, Quebec, CanadaDiedFebruary 12, 2020(2020-02-12) (aged 68)Toronto, Ontario, CanadaAlma materRyerson UniversityOccupation(s)newspaper columnist, writer and broadcasterYears active1972-2019Notable credit(s)Toronto Sun, National Post, The Globe and MailSpouses Jim Oreto (m. 1977; div. ...

Canale di WellandStato Canada Divisioni censuarie Ontario MunicipalitàPort Colborne, St. Catharines, Thorold, Welland Lunghezza43,5 km Mappa del fiume Modifica dati su Wikidata · Manuale Il canale di Welland è attraversato dal viadotto Garden City e dal ponte mobile Homer Una chiusa del secondo canale di Welland Chiusa abbandonata del terzo canale La nave MS Isa mentre attraversa la chiusa 7 Il canale di Welland (in inglese: Welland Canal) è un canale artificiale navigabile...

Запрос «Пугачёва» перенаправляется сюда; см. также другие значения. Алла Пугачёва На фестивале «Славянский базар в Витебске», 2016 год Основная информация Полное имя Алла Борисовна Пугачёва Дата рождения 15 апреля 1949(1949-04-15) (75 лет) Место рождения Москва, СССР[1]...

French artist (1885–1979) This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Sonia Delaunay – news · newspapers · books · scholar · JSTOR (August 2021) (Learn how and when to remove this message) Sonia DelaunaySonia Terk, c.1912BornSarah Elievna Shtern[citation needed](1885-11-14)14 November 1885Hrady...

A.C. VaredoCalcio Segni distintiviUniformi di gara Casa Trasferta Colori sociali Giallo, blu Dati societariCittàVaredo Nazione Italia ConfederazioneUEFA Federazione FIGC Fondazione1947 Scioglimento198?StadioComunale(250 posti) PalmarèsSi invita a seguire il modello di voce Il Football Club Lilion Snia Varedo, chiamato in passato anche Lilion Snia o semplicemente Varedo, è stata una società calcistica italiana con sede nella città di Varedo, in provincia di Monza. Disputò vari campi...

土库曼斯坦总统土库曼斯坦国徽土库曼斯坦总统旗現任谢尔达尔·别尔德穆哈梅多夫自2022年3月19日官邸阿什哈巴德总统府(Oguzkhan Presidential Palace)機關所在地阿什哈巴德任命者直接选举任期7年,可连选连任首任萨帕尔穆拉特·尼亚佐夫设立1991年10月27日 土库曼斯坦土库曼斯坦政府与政治 国家政府 土库曼斯坦宪法 国旗 国徽 国歌 立法機關(英语:National Council of Turkmenistan) ...
2020年夏季奥林匹克运动会波兰代表團波兰国旗IOC編碼POLNOC波蘭奧林匹克委員會網站olimpijski.pl(英文)(波兰文)2020年夏季奥林匹克运动会(東京)2021年7月23日至8月8日(受2019冠状病毒病疫情影响推迟,但仍保留原定名称)運動員206參賽項目24个大项旗手开幕式:帕维尔·科热尼奥夫斯基(游泳)和马娅·沃什乔夫斯卡(自行车)[1]闭幕式:卡罗利娜·纳亚(皮划艇)&#...

French-Tunisian architect (1920–2008) Olivier-Clément CacoubAmphi Weil, Grenoble UniversityBorn(1920-04-14)April 14, 1920Tunis, TunisiaDiedApril 27, 2008(2008-04-27) (aged 88)Paris, FranceNationalityFrenchOccupationArchitectAwardsGrand Prix de Rome (1953) Olivier-Clément Cacoub, (born April 14, 1920, in Tunis, and died April 27, 2008, in Paris), was a French architect of Tunisian-Jewish origin,[1] known for working in the International Style and Brutalism. Career He studied a...

Line of southernmost latitude at which the Sun can be directly overhead This article is about the circle of latitude. For other uses, see Tropic of Capricorn (disambiguation). World map showing the Tropic of Capricorn Relationship of Earth's axial tilt (ε) to the tropical and polar circles The Tropic of Capricorn (or the Southern Tropic) is the circle of latitude that contains the subsolar point at the December (or southern) solstice. It is thus the southernmost latitude where the Sun can be...

Science fiction book series by Martha Wells The Murderbot DiariesFirst edition cover of All Systems Red Novels: All Systems Red (2017) Artificial Condition (2018) Rogue Protocol (2018) Exit Strategy (2018) Network Effect (2020) Fugitive Telemetry (2021) System Collapse (2023) Short stories: Compulsory (2018) Obsolescence (2019) Home: Habitat, Range, Niche, Territory (2021) AuthorMartha WellsCover artistJaime JonesCountryUnited StatesLanguageEnglishGenreScience fictionPublisherTor Books (US)Me...

Vladislaus I Vladislaus I (Bahasa Ceska: Vladislav) (1065–1125), adipati Bohemia dari tahun 1109 - 1117 dan dari tahun 1120 sampai 12 April, 1125. Vladislav I merupakan Adipati, kemudian Raja, Vratislav II dari Bohemia oleh istri keduanya Swatawa, putri Casimir I dari Polandia. Bersama dengan sepupunya Svatopluk, Vladislav mengusir saudaranya Bořivoj II dari Bohemia pada tahun 1107. Pada tahun 1109 Svatopluk wafat, dan Vladislav I menggantikannya sebagai adipati Bohemia. Bořivoj II kembal...

Questa voce sugli argomenti competizioni calcistiche e sport in Giappone è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti del progetto di riferimento. Coppa dell'ImperatoreSport Calcio TipoSquadre di club FederazioneJFA Paese Giappone OrganizzatoreFederazione calcistica del Giappone CadenzaAnnuale Aperturasettembre Chiusuragennaio Partecipanti88 FormulaEliminazione diretta Sito Internetwww.jfa.or.jp/ StoriaFondazione1921 Deten...

Sign of National Youth National Youth (Swedish: Nationell ungdom) was a Swedish neofascist and openly racist organisation. National Youth was closely linked with the Swedish Resistance Movement. SÄPO classified it as a white power organisation[1] and Expo classified it as a Nazi organisation.[2] The group became famous in the summer of 1998 for tearing down photographs of nude boys at the Swedish Historical Museum. The reason behind the action was to protest against paedophil...
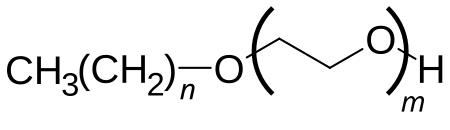
C12-15 pareth-12 n = 11-14, m = 12 Names Other names C12-15 pareth-12Ethoxylated C12-15 alcohols Identifiers CAS Number 68131-39-5 Y UNII 131316X18L Y Except where otherwise noted, data are given for materials in their standard state (at 25 °C [77 °F], 100 kPa). Infobox references Chemical compound C12-15 pareth-12 (INCI name) is an emulsifier and surfactant commonly used in cosmetics formulations. It is a polyethylene glycol ether formed by combining synthetic C12...
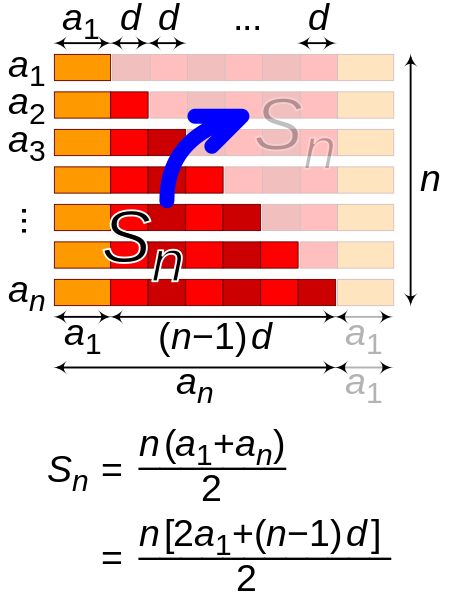
Sequence of equally spaced numbers Proof without words of the arithmetic progression formulas using a rotated copy of the blocks An arithmetic progression or arithmetic sequence is a sequence of numbers such that the difference from any succeeding term to its preceding term remains constant throughout the sequence. The constant difference is called common difference of that arithmetic progression. For instance, the sequence 5, 7, 9, 11, 13, 15, . . . is an arithmetic progression with a common...