Probability of success
|
Read other articles:

artikel ini perlu dirapikan agar memenuhi standar Wikipedia. Tidak ada alasan yang diberikan. Silakan kembangkan artikel ini semampu Anda. Merapikan artikel dapat dilakukan dengan wikifikasi atau membagi artikel ke paragraf-paragraf. Jika sudah dirapikan, silakan hapus templat ini. (Pelajari cara dan kapan saatnya untuk menghapus pesan templat ini) VillejuifNegaraPrancisArondisemenL'Haÿ-les-RosesAntarkomuneCommunautéd'agglomérationde Val de BièvreKode INSEE/pos94076 / Villejuif meru...

AdventHealth 400NASCAR Cup SeriesTempatKansas SpeedwayLokasiKansas City, Kansas, U.S.Perusahaan sponsorAdventHealth[1]Lomba pertama2011Jarak tempuh4.005 mil (6.445,423 km)Jumlah putaran267Stages 1/2: 80 eachFinal stage: 107Nama sebelumnyaSTP 400 (2011–2013) 5-Hour Energy 400 (2014) SpongeBob SquarePants 400 (2015) Go Bowling 400 (2016–2017) KC Masterpiece 400 (2018) Digital Ally 400 (2019) Super Start Batteries 400 presented by O'Reilly Auto Parts (2020) Buschy McBusch Race 4...

Sports stadium in New York City Ohio FieldOhio FieldLocation within New York CityShow map of New York CityOhio FieldOhio Field (New York)Show map of New YorkLocationThe Bronx, New YorkCoordinates40°51′28″N 73°54′40″W / 40.85778°N 73.91111°W / 40.85778; -73.91111OwnerBronx Community CollegeSurfacegrassTenantsBronx Broncos NYU Violets (former) Ohio Field is the main outdoor athletic facility at Bronx Community College in the University Heights neighborhood of...

Sensus Amerika Serikat Ke-22, dikenal sebagai Sensus 2000 dan dilaksanakan oleh Biro Sensus. Biro Sensus menetapkan jumlah penduduk Amerika Serikat pada 1 April 2000 sebanyak 281.421.906 jiwa, peningkatan 13.2% dibanding 248.709.873 jiwa pada Sensus 1990.[1] Ini merupakan sensus federal ke-22 dan pada waktu itu menjadi usaha administratif sipil masa damai terbesar di Amerika Serikat.[2] Sekitar 16 persen rumah tangga menerima formulir panjang pada sensus 2000 yang berisi lebih...

British actor (born 1978) For the South Australian softgoods retailer, see Matthew Goode (merchant). Matthew GoodeGoode at the 2014 Toronto Film FestivalBorn (1978-04-03) 3 April 1978 (age 46)Exeter, Devon, England[1]Alma materUniversity of BirminghamOccupationActorYears active2002–presentSpouseSophie DymokeChildren3RelativesSally Meen (half-sister) Matthew William Goode (born 3 April 1978) is a British actor.[2] Goode made his screen debut in 2002 with ABC's ...

Type of Filipino stilt house This article is about the Filipino house. For the folk song, see Bahay Kubo (folk song). Part of a series on theCulture of the Philippines Society History Language sign language People ethnic groups indigenous peoples Religion Value system Kinship Honorifics Arts and literature Architecture Arts Comics Dance Fashion and clothing Literature Music Other Cuisine Cultural Properties Folklore Historical markers Media newspapers radio cinema TV Internet Mythology Public...

École des Ponts ParisTech École nationale des ponts et chaussées adalah lembaga pendidikan tinggi dan penelitian tingkat universitas di bidang sains, teknik, dan teknologi. Didirikan pada tahun 1747 oleh Daniel-Charles Trudaine, ini adalah salah satu Grandes écoles Prancis tertua dan paling bergengsi.[1] Secara historis, misi utamanya adalah untuk melatih pejabat teknik dan insinyur sipil tetapi sekolah tersebut sekarang menawarkan pendidikan yang luas termasuk ilmu komputer, mate...

Kanojo mo KanojoSampul volume tankōbon pertama, menampilkan Saki Sakiカノジョも彼女(Kanojo mo Kanojo)GenreKomedi romantis[1] MangaPengarangHiroyukiPenerbitKodanshaPenerbit bahasa InggrisNA Kodansha USA (digital)MajalahWeekly Shōnen MagazineDemografiShōnenTerbit4 Maret 2020 – 24 Mei 2023Volume16 Seri animeSutradaraSatoshi KuwabaraSkenarioKeiichirō ŌchiMusikMiki SakuraiTatsuhiko SaikiStudioTezuka ProductionsPelisensiCrunchyroll SA/SEA Muse CommunicationSaluranasliJNN (MBS,...

烏克蘭總理Прем'єр-міністр України烏克蘭國徽現任杰尼斯·什米加尔自2020年3月4日任命者烏克蘭總統任期總統任命首任維托爾德·福金设立1991年11月后继职位無网站www.kmu.gov.ua/control/en/(英文) 乌克兰 乌克兰政府与政治系列条目 宪法 政府 总统 弗拉基米尔·泽连斯基 總統辦公室 国家安全与国防事务委员会 总统代表(英语:Representatives of the President of Ukraine) 总...

Alfons FrylandLahir(1888-05-01)1 Mei 1888Wina, Austria-Hungaria (kini Austria)Meninggal29 November 1953(1953-11-29) (umur 65)Graz, AustriaPekerjaanPemeranTahun aktif1921–1933 Alfons Fryland (1 Mei 1888 – 29 November 1953) adalah seorang pemeran film asal Austria.[1] Ia tampil dalam 47 film antara 1921 dan 1933. Filmografi pilihan Labyrinth des Grauens (1921) The Eternal Struggle (1921) Kean (1921) Frau Dorothys Bekenntnis (1921) Herzogin Satanella (1921) M...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Maret 2016. SMP Negeri 1 MamujuInformasiRentang kelasVII, VIII, IXKurikulumKurikulum Tingkat Satuan PendidikanAlamatLokasiJl. Hasanuddin 132, Mamuju, Sulawesi BaratMoto SMP Negeri (SMPN) 1 Mamuju, merupakan salah satu Sekolah Menengah Pertama Negeri yang ada di Mamu...

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (يناير 2022) المتغير في علم الإحصاء هو الخاصية أو السمة التي تأخذ قيما أو مستويات مختلفة من فرد إلى آخر (وتكون من قيمتين...

Political party in Quebec Coalition Avenir Québec AbbreviationCAQLeaderFrançois LegaultPresidentSarah BeaumierFoundersFrançois LegaultCharles SiroisFounded4 November 2011 (2011-11-04)[1]Split fromParti QuébécoisHeadquarters1260 Rue Mill, Montreal, QuebecH3K 2B4Membership (2022) 50,000[2]IdeologyQuebec nationalismQuebec autonomismConservatism (Canadian)PopulismPolitical positionCentre-right[3][4][5][6][7]Colo...

ADO Den Haag 2007/2008 Plaats Den Haag Stadion ADO Den Haag Stadion Capaciteit 15.000 Voorzitter Henk Jagersma Algemeen directeur Piet van der Pol Trainer Wiljan Vloet Assistent Henk Fraser Marco Gentile Topscorer(s) Hans van de Haar (9 goals) (Hoofd)sponsor Stad Holland Zorgverzekeraar Competitie Jupiler League Tenue Geldig voor 2007/08 Portaal Voetbal ADO Den Haag speelde in het seizoen 2007/08 in de Jupiler League. Na de degradatie een seizoen hiervoor eindigde ADO in de Eers...

Polygon with an infinite number of sides For the novel, see Apeirogon (novel). The regular apeirogonEdges and vertices∞Schläfli symbol{∞}Coxeter–Dynkin diagramsInternal angle (degrees)180°Dual polygonSelf-dual A partition of the Euclidean line into infinitely many equal-length segments can be understood as a regular apeirogon. In geometry, an apeirogon (from Ancient Greek ἄπειρος apeiros 'infinite, boundless', and γωνία gonia 'angle') or infini...
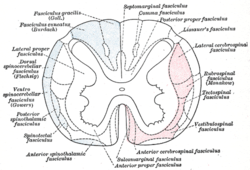
Not to be confused with spinotectal tract. Tectospinal tractDiagram showing possible connection of long descending fibers from higher centers with the motor cells of the ventral column through association fibers. (Tectospinal fasciculus labeled at center right.)Diagram of the principal fasciculi of the spinal cord. (Tectospinal fasciculus labeled at center right, in red.)DetailsIdentifiersLatintractus tectospinalisMeSHD065844NeuroLex IDbirnlex_759TA98A14.1.02.211 A14.1.04.112TA26119FMA72620An...

Bandar Udara Internasional Penglai Yantai烟台蓬莱国际机场IATA: YNTICAO: ZSYTInformasiJenisPublikPengelolaYantai International Airport GroupMelayaniYantai, ShandongLokasiChaoshui Town, Penglai, ShandongDibuka28 Mei 2015Maskapai utama China Eastern Airlines Shandong Airlines Zona waktuCST (UTC+08:00)Ketinggian dpl47 mdplKoordinat37°39′26″N 120°59′14″E / 37.65722°N 120.98722°E / 37.65722; 120.98722Situs webwww.ytairport.com.cnPetaCAAC airport...

Nepicastat Names Preferred IUPAC name 5-(Aminomethyl)-1-[(2S)-5,7-difluoro-1,2,3,4-tetrahydronaphthalen-2-yl]-1,3-dihydro-2H-imidazole-2-thione Other names SYN-117 Identifiers CAS Number 173997-05-2 Y 3D model (JSmol) Interactive image ChEBI CHEBI:139334 ChemSpider 7971947 Y IUPHAR/BPS 6630 MeSH Nepicastat PubChem CID 9796181 UNII VPG12K4540 Y CompTox Dashboard (EPA) DTXSID80169740 InChI InChI=1S/C14H15F2N3S/c15-9-3-8-4-10(1-2-12(8)13(16)5-9)19-11(6-17)7-18-14(19)20/h3,5,7,10H...

Roman Catholic church in Ilocos Sur, Philippines Church in Ilocos Sur, PhilippinesMagsingal ChurchSaint William the Hermit Parish ChurchSan Guillermo Hermitanio Parish ChurchChurch facade in 2011Magsingal ChurchLocation in LuzonShow map of LuzonMagsingal ChurchLocation in the PhilippinesShow map of Philippines17°41′05″N 120°25′32″E / 17.68459°N 120.42555°E / 17.68459; 120.42555LocationMagsingal, Ilocos SurCountryPhilippinesDenominationRoman CatholicHistoryF...

Mininera & District Football League General Information Founded 1925 Records Highest Score 515 – Great Western 79.41.515(v Ararat Eagles 1.0.6) – 2019 Most goals in a game 28 – Jason Mifsud – Caramut (v Dunkeld) – 1994 Most goals in a season 205 – Jason Mifsud – Caramut – 1994 Most wins in a row 36 – Tatyoon – 2006–08 Most losses in a row 43 – Dunkeld – 1993–96 Most flags in a row 4 – Lake Bolac – 1954–1957 The Mininera & District Football League is ...