Apeirogon
| |||||||||||||||
Read other articles:

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Maret 2024. Aosta Airport(Corrado Gex Airport)Aeroporto regionale della Valle d'Aosta Corrado GexAéroport régional de la Vallée d'Aoste Corrado GexIATA: AOTICAO: LIMWInformasiJenisPublicPengelolaGovernmentMelayaniAostaLokasiSaint-Christophe, ItalyKetinggian ...

Musical feast given by the cardinal de La Rochefoucauld in the Teatro Argentina in Rome in 1747 Lists of operas cover operas, a form of theatre in which music is essential, and the roles are portrayed by singers. There are general lists and lists by theme, country, medium and venue. General List of operas by composer List of prominent operas List of operas by title By theme List of operettas List of Christmas operas List of operas set in the Crusades List of Orphean operas Science fiction op...

Township in Illinois, United StatesMelrose TownshipTownshipLocation in Adams CountyAdams County's location in IllinoisCoordinates: 39°53′07″N 91°20′40″W / 39.88528°N 91.34444°W / 39.88528; -91.34444CountryUnited StatesStateIllinoisCountyAdamsEstablishedNovember 6, 1849Area • Total46.55 sq mi (120.6 km2) • Land44.57 sq mi (115.4 km2) • Water1.98 sq mi (5.1 km2) 4.25%Eleva...

French-born prelate The Most ReverendHenry Damian JunckerBishop of AltonSeeDiocese of AltonInstalledApril 26, 1857Term endedOctober 2, 1868PredecessornoneSuccessorPeter Joseph BaltesOrdersOrdinationMarch 16, 1834by John Baptist PurcellConsecrationApril 26, 1857by John Baptist PurcellPersonal detailsBorn(1809-08-22)August 22, 1809Fénétrange, Moselle, FranceDiedOctober 2, 1868(1868-10-02) (aged 59)Alton, Illinois, USA Henry Damian Juncker (August 22, 1809 – October 2, 1868) w...

Синелобый амазон Научная классификация Домен:ЭукариотыЦарство:ЖивотныеПодцарство:ЭуметазоиБез ранга:Двусторонне-симметричныеБез ранга:ВторичноротыеТип:ХордовыеПодтип:ПозвоночныеИнфратип:ЧелюстноротыеНадкласс:ЧетвероногиеКлада:АмниотыКлада:ЗавропсидыКласс:Пт�...

† Человек прямоходящий Научная классификация Домен:ЭукариотыЦарство:ЖивотныеПодцарство:ЭуметазоиБез ранга:Двусторонне-симметричныеБез ранга:ВторичноротыеТип:ХордовыеПодтип:ПозвоночныеИнфратип:ЧелюстноротыеНадкласс:ЧетвероногиеКлада:АмниотыКлада:Синапсиды�...

Antonio Pergreffi Nazionalità Italia Altezza 189 cm Calcio Ruolo Difensore Squadra Modena Carriera Giovanili ???? AlbinoLeffe Squadre di club1 2006-2007 Ardens Cene? (3)2007-2009 AlzanoCene? (3)2009-2010 Scanzorosciate? (1)2010-2011 Brusaporto? (4)2011-2014 Scanzorosciate? (10)2014-2015 Pontisola31 (1)2015-2016 Lecco36 (5)[1]2016-2020 Piacenza127 (10)[2]2020- Modena115 (7)[3] 1 I due numeri indicano le presenze...

Mario Van Peebles Mario Cain Van Peebles (Città del Messico, 15 gennaio 1957) è un attore, regista, sceneggiatore, produttore cinematografico e produttore televisivo statunitense. Come regista ha diretto film che narrano dei problemi della droga e della violenza nella comunità afroamericana (New Jack City) e delle Pantere Nere (Panther). Nel 1991 è stato inserito dalla rivista People tra i 50 uomini più belli del mondo.[1] Indice 1 Biografia 2 Filmografia parziale 2.1 Regista 2.1...

Association football club in Suez, Egypt This article does not cite any sources. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Suez Cement SC – news · newspapers · books · scholar · JSTOR (February 2019) (Learn how and when to remove this message) Football clubSuez CementFull nameAsmant el-SuweisFounded1995; 29 years ago (1995)GroundSuez Stadium...

Not to be confused with Albert Speer. American judge Albert Spear, Justice of the Maine Supreme Court Albert Moore Spear (March 17, 1852 – January 31, 1929) was the Chief Justice of the Maine Supreme Judicial Court and President of the Maine Senate. Spear was born in Madison, Maine in 1852. He graduated from Bates College in Lewiston, Maine in 1875. Spear then studied law and was admitted to the Maine bar in 1878. Spear went on to practice law and served as a city solicitor, mayor and s...

土库曼斯坦总统土库曼斯坦国徽土库曼斯坦总统旗現任谢尔达尔·别尔德穆哈梅多夫自2022年3月19日官邸阿什哈巴德总统府(Oguzkhan Presidential Palace)機關所在地阿什哈巴德任命者直接选举任期7年,可连选连任首任萨帕尔穆拉特·尼亚佐夫设立1991年10月27日 土库曼斯坦土库曼斯坦政府与政治 国家政府 土库曼斯坦宪法 国旗 国徽 国歌 立法機關(英语:National Council of Turkmenistan) ...
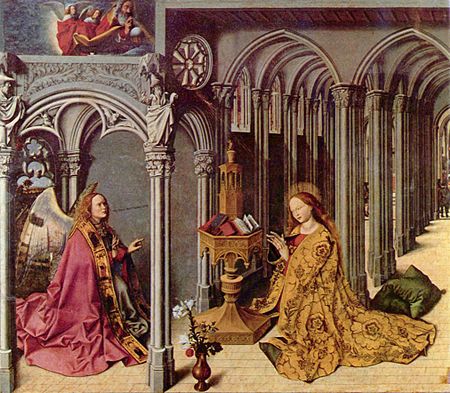
Subject in art The Virgin shrinks back in reluctance in the Annunciation with Sts. Margaret and Ansanus, by Simone Martini and Lippo Memmi, 1333 Aix Annunciation, generally attributed to Barthélemy d'Eyck, c. 1443–1445 Domenico Beccafumi, 1545 The Annunciation has been one of the most frequent subjects of Christian art.[1][2] Depictions of the Annunciation go back to early Christianity, with the Priscilla catacomb in Rome including the oldest known fresco of the Annunciatio...

Quarterly magazine in print since 1994 This article relies excessively on references to primary sources. Please improve this article by adding secondary or tertiary sources. Find sources: Palestine–Israel Journal – news · newspapers · books · scholar · JSTOR (July 2012) (Learn how and when to remove this message) Palestine–Israel JournalFrequencyQuarterlyPublisherMiddle East PublicationsFounded1994Based inJerusalemLanguageEnglish, Hebrew, ArabicWeb...

Mackenzie Arnold Datos personalesNombre completo Mackenzie Elizabeth ArnoldNacimiento Gold Coast, Queensland, Australia 25 de febrero de 1994 (30 años)Nacionalidad(es) AustralianaAltura 1,79 metrosCarrera deportivaDeporte FútbolClub profesionalClub West Ham UnitedPosición PorteraDorsal(es) 1Selección nacionalSelección Selección de AustraliaDebut 2012Part. (goles) 28 (0)[editar datos en Wikidata] Mackenzie Elizabeth Arnold (Gold Coast, Queensland, Australia;...

Greek deity of the Moon Herm of Aphroditus at the Nationalmuseum in Stockholm Greek terracotta figurine, late 4th century BCE, National Museum of Magna Grecia Bronze statuette, Roman imperial, 1st-3rd century CE, British Museum[1] Aphroditus or Aphroditos (Greek: Ἀφρόδιτος, Aphróditos, [apʰróditos]) was a male Aphrodite originating from Amathus on the island of Cyprus and celebrated in Athens. Aphroditus was portrayed as having a female shape and clothing like Aph...

Sudden overwhelming sensation of fear For the psychological condition, see Panic attack. For other uses, see Panic (disambiguation). Illustration in Charles Darwin's The Expression of the Emotions in Man and Animals Part of a series onEmotions Affect Classification In animals Emotional intelligence Mood Regulation Interpersonal Dysregulation Valence Emotions Acceptance Admiration Affection Amusement Anger Angst Anguish Annoyance Anticipation Anxiety Apathy Arousal Awe Belongingness Boredom Co...

Shiseido Company, LimitedLogo Stato Giappone ISINJP3351600006 Fondazione1872 Fondata daArinobu Fukuhara Sede principaleTokyo Persone chiaveMasahiko Uotani CEO Settorebeni di consumo Prodotticosmetici, lozioni, profumi Fatturato7,4 miliardi di €, circa 1.000 miliardi di yen (2017) Dipendenti33.356 (2013) Sito webwww.shiseido.co.jp/com/ Modifica dati su Wikidata · Manuale Shiseido Company, Limited (株式会社資生堂?, Kabushiki-gaisha Shiseidō) è la maggiore azienda giappones...

Pour les articles homonymes, voir MMA. Arts martiaux mixtes Autres noms Mixed martial arts (MMA)Combat libreCage fightFree-fight Sport olympique non Fédération mondiale IMMAF[1] modifier Les arts martiaux mixtes, souvent désignés par le sigle anglais MMA pour mixed martial arts, anciennement appelés combat libre ou free-fight, sont un sport de combat de percussion-préhension, combinant les techniques de percussion telles que coups de pied, de poing, de genou et de coude, mais aus...

Uprising against British Company rule Sepoy Mutiny redirects here. For other uses, see Sepoy Mutiny (disambiguation). Indian War of Independence redirects here. For other uses, see The Indian War of Independence (disambiguation). Indian Rebellion of 1857A 1912 map of Northern India, showing the centres of the rebellion.Date10 May 1857 (1857-05-10) – 1 November 1858 (1858-11-01)(1 year and 6 months)LocationIndiaResult British victoryTerritorialchanges ...

American cartoonist Bob Bindig (right) in 1982. Robert K. Bindig (December 21, 1920 – November 6, 2007) was a cartoonist and comics historian who worked on the Adventures of the Big Boy comic book from 1985 to 1995.[1] Education In the late 1930s, Bindig attended the Buffalo Technical High School where he took a number of art classes.[1] Personal life Bindig was born in Buffalo, New York, but eventually moved to Orchard Park. He married Doris Krull in 1941, with whom he had ...



