Einstein manifold
|
Read other articles:

I Promise You (I.P.U.)Lagu oleh Wanna Onedari album 0+1=1 (I Promise You)Dirilis5 Maret 2018 (2018-03-05)FormatDigital downloadGenrePopDance-popK-popDurasi3:40LabelYMC EntertainmentCJ E&M MusicPenciptaGalactikaRobinAthenaVideo musikI Promise You (I.P.U.) di YouTube I Promise You (I.P.U.) adalah lagu dari boy band asal Korea Selatan Wanna One. Lagu ini menjadi lagu pra-singel dari album mini kedua mereka, 0+1=1 (I Promise You). Tangga lagu Tanggal lagu mingguan Tangga lagu (2018) Posi...

Gabriele FaernoBiografiKelahiran1510 Cremona Kematian17 November 1561 (50/51 tahun)Roma KegiatanPekerjaanPenulis dan penyair AliranHumanisme Sebuah cetakan karya Thomas Augustinus Vairani dari patung dada Gabriele Faerno di Capitoline Museum, 1772 Gabriele Faerno adalah seorang cendekiawan humanis. Ia juga dikenal dengan nama Latin Faernus Cremonensis. Ia lahir di Cremona pada sekitar tahun 1510 dan meninggal di Roma pada 17 November 1561. Ia adalah penyinting dan penyair Latin yang kini dike...

Military governor of Mari Ishtup-Ilum𒅖𒁾𒀭Military governor of MariStatue of Ishtup-Ilum.Reignc.2147–2136 BCEPredecessorNûr-MêrSuccessorIshgum-AdduDynastyShakkanakku dynasty Mariclass=notpageimage| Location of Mari, where Ishtup-Ilum ruled. Ishtup-Ilum, also Ishtup-El (𒅖𒁾𒀭, Ish-dub-ilum, c. 2147–2136 BCE)[1] was a ruler of the city of Mari, one of the military governors known as Shakkanakku in northern Mesopotamia, after the fall of the Akkadian Empire.[2]...

Football leagueOberliga HamburgFounded1945CountryGermanyStateHamburgNumber of teams19Level on pyramidLevel 5Promotion toRegionalliga NordRelegation to Landesliga Hamburg-Hammonia Landesliga Hamburg-Hansa Current championsTSV Sasel (2022–23) The Oberliga Hamburg, sometimes referred to as Hamburg-Liga, is the highest league in the German state of Hamburg, incorporating some of its surrounding districts. It is one of fourteen Oberligen in German football, the fifth tier of the German football ...

Untuk keju, lihat Quark (keju). Quark Sebuah proton, tersusun atas 2 up quark dan satu down quark (Pewarnaan Muatan warna pada tiap quark tidak begitu penting, hanya ketiga warna tersebut diwakilkan.) Komposisi: Partikel dasar Generasi: Ke-1, ke-2, ke-3 Interaksi: Elektromagnetik, Gravitasi, Interaksi Kuat, Interaksi Lemah Simbol: q Antipartikel: Antiquark (q) Penggagas: Murray Gell-Mann (1964) George Zweig (1964) Penemu: SLAC (~1968) Tipe: 6 (up, down, strange, charm, bottom, and top) Muatan...

Manulife Financial CorporationKantor pusat Manulife di Bloor Street di Downtown TorontoSebelumnyaThe Manufacturers Life Insurance CompanyJenisPublikKode emitenTSX: MFCNYSE: MFCSEHK: 945PSE: MFCIndustriJasa keuanganDidirikan23 Juni 1887; 136 tahun lalu (1887-06-23)Kantorpusat200 Bloor Street EastToronto, OntarioM4W 1E5TokohkunciRoy Gori (Presiden dan CEO) John Cassaday (Chairman dewan direksi)ProdukManajemen aset, perbankan komersial, kredit pemilikan properti, perbankan konsume...

Mid-20th-century American futurist architectural style Googie redirects here. For the British actress, see Googie Withers. For the American percussionist, see Arthur Googy. Not to be confused with Google. Norms Restaurants location on La Cienega Boulevard in Los Angeles Googie architecture (/ˈɡuːɡi/ GOO-ghee[1]) is a type of futurist architecture influenced by car culture, jets, the Atomic Age and the Space Age.[2] It originated in Southern California from the Streamline M...

Questa voce sull'argomento stagioni delle società calcistiche italiane è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti del progetto di riferimento. Voce principale: S.E.F. Torres 1903. S.E.F. TorresStagione 1964-1965 Sport calcio Squadra Torres Allenatore Federico Allasio Presidente Nino Costa Serie C9º posto nel girone B. Maggiori presenzeCampionato: Biagi, Grottola (34) Miglior marcatoreCampionato: G. Currarini, Di Stefan...

Nicolas WalterBornNicolas Hardy Walter(1934-11-22)22 November 1934South London, EnglandDied7 March 2000(2000-03-07) (aged 65)Milton Keynes, EnglandEducation Rendcomb College Exeter College, Oxford Occupations Writer Journalist Movement Anarchism Anti-war Humanism Spouses Ruth Oppenheim (m. 1962; div. 1982) Christine Morris (m. 1987) Children2, including Natasha WalterParentWilliam Grey Walter (father)Rela...

Anjung Seni Idrus TintinInformasi umumGaya arsitekturMelayu RiauLokasiPekanbaru, Riau, IndonesiaAlamatKompleks Bandar Seni Raja Ali Haji (Bandar Serai), PekanbaruKoordinat0°28′41″N 101°27′21″E / 0.4779809°N 101.4558773°E / 0.4779809; 101.4558773Koordinat: 0°28′41″N 101°27′21″E / 0.4779809°N 101.4558773°E / 0.4779809; 101.4558773Diresmikan11 Agustus 2007[2]PemilikUnit Pelaksana Teknis (UPT) Bandar Serai, di bawah p...

1987 anime film Neo TokyoJapanese theatrical posterJapanese nameKanji迷宮物語TranscriptionsRevised HepburnMeikyū Monogatari Directed by Rintaro (Labyrinth Labyrinthos) Yoshiaki Kawajiri (The Running Man) Katsuhiro Otomo (Construction Cancellation Order) Written by Rintaro (Labyrinth Labyrinthos) Yoshiaki Kawajiri (The Running Man) Katsuhiro Otomo (Construction Cancellation Order) Based onMeikyū Monogatariby Taku MayumuraProduced byMasao MaruyamaRintaroStarring Hideko Yoshida Masane Tsuk...

American author (1927–2020) For the Michigan politician, see Roger Kahn (politician). For the American musician and composer, see Roger Wolfe Kahn. Not to be confused with Roger Angell. Roger KahnBorn(1927-10-31)October 31, 1927New York City, U.S.DiedFebruary 6, 2020(2020-02-06) (aged 92)Mamaroneck, New York, U.S.OccupationAuthorNationalityAmericanNotable worksThe Boys of Summer Roger Kahn (October 31, 1927 – February 6, 2020) was an American author, best known for his 1972 baseball ...

Judgments of the Constitutional Courtof South Africa 1995 1996 1997 1998 1999 2000 2001 2002 2003 2004 2005 2006 2007 2008 2009 2010 2011 2012 2013 2014 2015 2016 2017 2018 2019 2020 2021 2022 2023 2024 vte The table below lists the judgments of the Constitutional Court of South Africa delivered in 2000. The members of the court during 2000 were President Arthur Chaskalson, Deputy President Pius Langa, and judges Lourens Ackermann, Richard Goldstone, Johann Kriegler, Tholie Madala, Yvonne Mo...

Raya Airways IATA ICAO Kode panggil TH RMY Raya Express Didirikan1993; 31 tahun lalu (1993) (sebagai Transmile Air Services)PenghubungBandar Udara Sultan Abdul Aziz ShahArmada5Tujuan9SloganDelivering Trust And ValueKantor pusatBandar Udara Sultan Abdul Aziz ShahSubang, MalaysiaSitus webrayaairways.com Raya Airways Sdn Bhd adalah maskapai penerbangan kargo dengan kantor pusat di Raya Airways Centre di Kompleks Kargo di Bandar Udara Sultan Abdul Aziz Shah di Subang, Selangor, Malaysia.[...

French politician This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: André Joseph Abrial – news · newspapers · books · scholar · JSTOR (March 2018) (Learn how and when to remove this message) André Joseph Abrial André Joseph Abrial (19 March 1750, Annonay, Ardèche – 13 November 1828)[1] was a ...
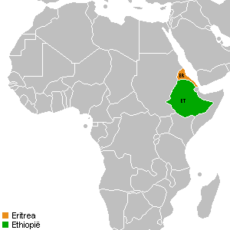
United Nations resolution adopted in 2005 UN Security CouncilResolution 1640Ethiopia (green) and Eritrea (orange)Date23 November 2005Meeting no.5,308CodeS/RES/1640 (Document)SubjectThe situation between Eritrea and EthiopiaVoting summary15 voted forNone voted againstNone abstainedResultAdoptedSecurity Council compositionPermanent members China France Russia United Kingdom United StatesNon-permanent members Algeria Argentina Benin Brazil ...

Voce principale: Associazione Calcio Savoia 1908. Unione Sportiva SavoiaStagione 1939-1940Sport calcio Squadra Savoia Allenatore Osvaldo Sacchi Presidente Bruno Salzano Serie C9º posto Coppa ItaliaSedicesimi di finale Maggiori presenzeCampionato: Di Reda, Risorti (28)Totale: Di Reda, Risorti (32) Miglior marcatoreCampionato: Marcer (9)Totale: Abatematteo, Marcer (10) StadioCampo Formisano (5.000) 1938-1939 1940-1941 Si invita a seguire il modello di voce Questa voce raccoglie le inform...

Geneva Open 1988Sport Tennis Data19 settembre – 26 settembre Edizione9a SuperficieTerra rossa CampioniSingolare Marián Vajda Doppio Mansour Bahrami / Tomáš Šmíd 1987 1989 Il Geneva Open 1988 è stato un torneo di tennis giocato sulla terra rossa. È stata la 9ª edizione del torneo, che fa parte del Nabisco Grand Prix 1988. Si è giocato a Ginevra in Svizzera dal 19 al 26 settembre 1988. Indice 1 Campioni 1.1 Singolare maschile 1.2 Doppio maschile 2 Collegamenti esterni Campioni Singol...

لي غراند الإحداثيات 42°00′22″N 92°46′35″W / 42.006111111111°N 92.776388888889°W / 42.006111111111; -92.776388888889 [1] تقسيم إداري البلد الولايات المتحدة[2] التقسيم الأعلى آيوا خصائص جغرافية المساحة 2.718944 كيلومتر مربع2.717487 كيلومتر مربع (1 أبريل 2010) ارتفاع 284 متر&#...

Short story by L. Sprague de CampLet's Have FunShort story by L. Sprague de CampIllustration of thestory in Science Fiction QuarterlyCountryUnited StatesLanguageEnglishGenre(s)Science fictionPublicationPublished inScience Fiction QuarterlyPublisherColumbia Publications, Inc.Media typePrint (Magazine)Publication dateMay 1957 Let's Have Fun is a science fiction short story by L. Sprague de Camp. It was first published in the magazine Science Fiction Quarterly for May, 1957.[1][2...














