Deconvolution
|
Read other articles:

This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article possibly contains original research. Please improve it by verifying the claims made and adding inline citations. Statements consisting only of original research should be removed. (June 2023) (Learn how and when to remove this template message) This article needs additional citations for verification. Please help improve this a...

القوات المسلحة الأردنية شعار القوات المسلحة الأردنية الدولة الأردن التأسيس 22 أكتوبر 1920 الاسم الأصلي الجيش العربي ألقاب الجيش المصطفوي اسم آخر القوات المسلحة الملكية الأردنية الفروع القوات البرية سلاح الجو القوة البحرية المقر عمّان القيادة القائد الأعلى المشير المل�...

Maskanan Informasi pribadiHubunganMashuri Saleh (saudara kandung)Karier militerDinas/cabang TNI Angkatan DaratMasa dinas1945—1975Pangkat Brigadir Jenderal TNINRP10171SatuanInfanteriSunting kotak info • L • B Brigadir Jenderal TNI (Purn.) Maskanan merupakan seorang perwira tinggi Angkatan Darat. Dan pernah menjabat Kepala Jawatan Keuangan Angkatan Darat pada 5 September 1975. Pendidikan Seskoad, 1964[1] Kursus Reguler Angkatan 3, Lemhannas, 2 Juni 1969 sampai 24 De...

This article is about the current branch of One Nation in New South Wales. For the former political party, see One Nation NSW. Political party in Australia One Nation One Nation – New South WalesAbbreviationPHONLeaderTania MihailukHeadquarters3/36 Vincent Street, Cessnock NSW 2325Ideology Australian nationalism Hansonism National conservatism Right-wing populism Political positionRight-wing to far-rightNational affiliationPauline Hanson's One NationColours OrangeLegislative ...

1907-1927 American film industry magazine The Moving Picture WorldJanuary 4, 1913 cover, featuring a scene from A Sicilian Heroine, an Italian filmCategoriesFilmEntertainmentFirst issue1907 (116 years ago)Final issueDecember 1927 (95 years ago)CountryUnited StatesBased inNew York CityLanguageEnglishOCLC1717051 The Moving Picture World was an influential early trade journal for the American film industry, from 1907 to 1927.[1] An industry powerhouse at its height, Moving Picture World ...

2020 documentary by Dawn Porter John Lewis: Good TroubleFilm posterDirected byDawn PorterProduced byLaura MichalchyshynDawn PorterErika AlexanderBen ArnonCinematographyTony HardmonKeith WalkerStefan WiesenEdited byJessica CongdonMusic byTamar-kaliProductioncompaniesCNN FilmsAGC StudiosTIME StudiosDistributed byMagnolia PicturesParticipantRelease dates June 19, 2020 (2020-06-19) (Tulsa) July 3, 2020 (2020-07-03) (United States) Running time96 minutesCountr...
Lambang Peta Data dasar Negara bagian: Hessen Regierungsbezirk: Gießen Ibu kota: Marburg Wilayah: 1.262,56 km² Penduduk: 252.792 (30 September 2005) Kepadatan penduduk: 200 jiwa per km² Nomor pelat kendaraan bermotor: MR Pembagian administratif: 9 kota13 kotamadya Alamat kantor bupati: Im Lichtenholz 6035043 Marburg Situs web resmi: www.marburg-biedenkopf.de Bupati: Robert Fischbach (CDU) Peta Marburg-Biedenkopf adalah sebuah distrik (Landkreis) di Hessen, Jerman. Marburg-Biedenkopf berba...

2008 novel by John Green Paper Towns The two[1] first edition coversAuthorJohn GreenCountryUnited StatesLanguageEnglishGenreYoung adult, MysteryPublisherDutton BooksPublication dateOctober 16, 2008Media typePrint (Hardback, Paperback)Pages305ISBN978-0-525-47818-8OCLC202483793LC ClassPZ7.G8233 Pap 2008 Paper Towns is a novel written by John Green, published on October 16, 2008, by Dutton Books.[2] The novel is about the coming-of-age of the protagonist, Quentin Q Jaco...

Halo, Nara Fortice. Selamat datang di Wikipedia bahasa Indonesia! Memulai Bacalah halaman Pengantar Wikipedia terlebih dahulu. Baca juga informasi tentang berkontribusi di Wikipedia. Lihat pula aturan yang disederhanakan sebelum melanjutkan. Tips Selalu tanda tangani pertanyaan Anda di Warung Kopi atau halaman pembicaraan dengan mengetikkan ~~~~ pada akhir kalimat Anda. Jangan takut! Anda tidak perlu takut salah ketika menyunting atau membuat halaman baru, menambahkan, atau menghapus kalimat...

ДостопримечательностьДом Пауля Лёбе Дом Пауля Лёбе. Западный фасад. Вид из здания Ведомства федерального канцлера через площадь Бюргерфорум 52°31′13″ с. ш. 13°22′29″ в. д.HGЯO Страна Германия[1] Местоположение Митте Архитектурный стиль архитектура постмоде�...

Symbol of an alleged conspiratorial military takeover or presence of UFOs For other uses, see Black helicopter (disambiguation). Unmarked black helicopters have been described in conspiracy theories since the 1970s. The black helicopter is a symbol of an alleged conspiratorial military takeover of the United States in the American militia movement, and has also been associated with UFOs,[1] especially in the UK,[2] men in black, and similar conspiracy theories.[2][...

Plateau in southwestern United States A map of the Colorado Plateau. The Four Corners region and the Colorado Plateau. The Colorado Plateau, also known as the Colorado Plateau Province,[1] is a physiographic and desert region of the Intermontane Plateaus, roughly centered on the Four Corners region of the southwestern United States. This province covers an area of 336,700 km2 (130,000 mi2) within western Colorado, northwestern New Mexico, southern and eastern Utah, northern ...
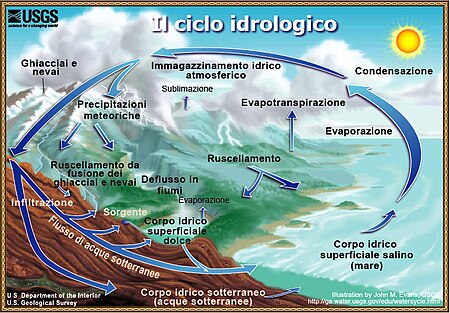
Questa voce o sezione sull'argomento geografia è priva o carente di note e riferimenti bibliografici puntuali. Sebbene vi siano una bibliografia e/o dei collegamenti esterni, manca la contestualizzazione delle fonti con note a piè di pagina o altri riferimenti precisi che indichino puntualmente la provenienza delle informazioni. Puoi migliorare questa voce citando le fonti più precisamente. Segui i suggerimenti del progetto di riferimento. Per idrosfera si intende l'insieme delle acq...

Aleksandr Dovbnya Krasnodar - SKA-Khabarovsk, 2017Informasi pribadiNama lengkap Aleksandr Vyacheslavovich DovbnyaTanggal lahir 14 April 1987 (umur 37)Tinggi 1,89 m (6 ft 2+1⁄2 in)Posisi bermain Penjaga gawangInformasi klubKlub saat ini FC Torpedo MoscowNomor 56Karier senior*Tahun Tim Tampil (Gol)2007–2008 FC Haka 52 (0)2009 Sibir 0 (0)2010 FC Nizhny Novgorod 0 (0)2011 FC Podolye Podolsky district 24 (0)2012– FC Torpedo Moscow 3 (0) * Penampilan dan gol di klub s...

Kitab Injil atau Evangeliarium (bahasa Yunani: Εὐαγγέλιον, Evanggélion; bahasa Latin: Evangeliarium) adalah kodeks atau buku jilid yang memuat satu atau lebih Injil dari Kitab Suci Perjanjian Baru. Lazimnya sebuah kitab Injil memuat seluruh Injil yang terdapat di dalam Kitab Suci Perjanjian Baru, yakni keempat versi riwayat hidup Yesus dari Nazaret yang merupakan akar iman Kristen. Istilah kitab Injil dapat pula berarti buku liturgi yang disebut evangeliarium, yakni kumpu...

يرقات البعوض في المياه الراكدة المياه الراكدة[1] تحدث عندما تتوقف المياه عن التدفق، وتعتبر المياه الراكدة أحد المخاطر البيئية.[2][3][4] الأخطار تعتبر الملاريا وحمى الضنك من أكبر أخطار المياه الراكدة، والتي يمكن أن تُصبح مرتعا للبعوض الناقل لهذه الأمراض. المي�...

العلاقات الباربادوسية التشادية باربادوس تشاد باربادوس تشاد تعديل مصدري - تعديل العلاقات الباربادوسية التشادية هي العلاقات الثنائية التي تجمع بين باربادوس وتشاد.[1][2][3][4][5] مقارنة بين البلدين هذه مقارنة عامة ومرجعية للدولتين: وجه المق...

NW Mystery Racer The NW-1 before going to Selfridge Field, Michigan, 1922 Role Air racingType of aircraft National origin United States Manufacturer Wright Aeronautical Corporation Designer Rex Beisel[1] First flight 11 October 1922[2] Status Both aircraft destroyed Primary user US Navy Number built 2 (2 NW-1, 1 NW-2 (rebuilt from the original NW-1) The Navy-Wright NW series, also called the Mystery Racer were racing aircraft built by Wright Aeronautical Corporation at t...

The remaining nuclide left over from radioactive decay This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Decay product – news · newspapers · books · scholar · JSTOR (December 2007) (Learn how and when to remove this message) Nuclear physics Nucleus Nucleons p n Nuclear matter Nuclear force Nuclear structure Nu...

Art of persuasion For the work by Aristotle, see Rhetoric (Aristotle). Part of a series onRhetoric History Ancient Greece Asianism Atticism Attic orators Calliope Sophists Ancient India Ancient Rome The age of Cicero Second Sophistic Middle Ages Byzantine rhetoric Trivium Renaissance Studia humanitatis Modern period Concepts Captatio benevolentiae Chironomia Decorum Delectare Docere Device Eloquence Eloquentia perfecta Eunoia Enthymeme Facilitas Fallacy Informal Figure of speech Scheme Trope ...













