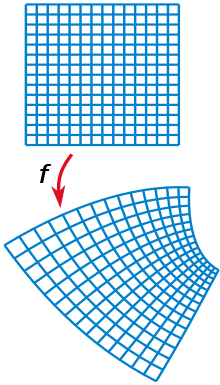
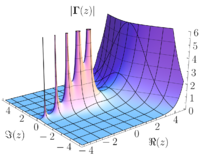
Conformal map
|
Read other articles:

Provinsi Ise (伊勢国code: ja is deprecated , ise no kuni) atau dikenal sebagai Seishu (勢州code: ja is deprecated , seishū) adalah nama provinsi lama Jepang yang menempati hampir seluruh wilayah prefektur Mie sekarang. Ise berbatasan dengan provinsi Iga, Kii, Mino, Omi, Owari, Shima, dan provinsi Yamato. Ibu kota berada di Suzuka. Kota istana yang terbesar adalah kota yang sekarang dikenal sebagai Tsu, walaupun ada juga kota sekeliling istana yang berkembang di tempat-tempat yang memil...

I Do Not Hook UpSingel oleh Kelly Clarksondari album All I Ever WantedDirilis31 Maret 2009 (2009-03-31)Direkam2008GenreDance-rockpower popDurasi3:20LabelRCAPenciptaKara DioGuardiGreg WellsKaty PerryProduserHoward BensonKronologi singel Kelly Clarkson My Life Would Suck Without You (2009) I Do Not Hook Up (2009) Already Gone (2009) Video musikI Do Not Hook Up di YouTube I Do Not Hook Up adalah lagu yang direkam oleh penyanyi asal Amerika Serikat Kelly Clarkson untuk album studio keempatny...

Anna Q. NilssonNilsson, c. 1920LahirAnna Quirentia Nilsson(1888-03-30)30 Maret 1888Ystad, SwediaMeninggal11 Februari 1974(1974-02-11) (umur 85)Sun City, California, A.S.PekerjaanAktrisTahun aktif1911–1954Suami/istriGuy Coombs (m. 1916; c. 1917) J. Marshall Gunnerson (m. 1922; c. 1925) Anna Quirentia Nilsson (30 Maret 1888 – 11 Februari 1974) adalah seorang aktri...

Voce principale: Promozione 1969-1970. Promozione Lombardia 1969-1970 Competizione Promozione Sport Calcio Edizione 14ª Organizzatore FIGC - LNDComitato Regionale Lombardo Luogo Italia Cronologia della competizione 1968-1969 1970-1971 Manuale Nella stagione 1969-1970 la Promozione era il quinto livello del calcio italiano (il massimo livello regionale). Qui vi sono le statistiche relative al campionato in Lombardia. Il campionato di Promozione Lombarda 1969-70 è stato il 14º campion...

Untuk kegunaan lain, lihat Sumatra (disambiguasi). SumatraNama lokal: سومترا (Jawi)Topografi Pulau SumatraPulau Sumatra di IndonesiaGeografiLokasiAsia TenggaraKoordinat0°00′N 102°00′E / 0.000°N 102.000°E / 0.000; 102.000KepulauanKepulauan Sunda BesarLuas473.481 km2Peringkat luaske-6Titik tertinggiGunung Kerinci (3.805 m)PemerintahanNegara IndonesiaProvinsi Aceh Sumatera Utara Sumatera Barat Riau Jambi ...

John NewlandsLahir26 November 1837 (1837-11-26)Lambeth, Surrey, Inggris,Britania RayaMeninggal29 Juli 1898 (1898-07-28UTC16) (aged 60)Lower Clapton, Middlesex, Inggris, Britania RayaKebangsaanInggrisWarga negaraBritaniaAlmamaterRoyal College of ChemistryDikenal atasTabel periodik, hukum oktafPenghargaanDavy Medal (1887)Karier ilmiahBidangKimia analisis Tempat lahir Newlands di West Square, Lambeth. John Alexander Reina Newlands (26 November 1837 – 29 Juli 1898) adalah seoran...

Questa voce o sezione sull'argomento centri abitati della Spagna non cita le fonti necessarie o quelle presenti sono insufficienti. Puoi migliorare questa voce aggiungendo citazioni da fonti attendibili secondo le linee guida sull'uso delle fonti. Segui i suggerimenti del progetto di riferimento. Pedrafita do Cebreirocomune Pedrafita do Cebreiro – Veduta LocalizzazioneStato Spagna Comunità autonoma Galizia Provincia Lugo TerritorioCoordinate42°44′N 7°01′W /...

Kementerian Pendidikan, Kebudayaan, dan Ilmu PengetahuanMinisterie van Onderwijs, Cultuur en WetenschapLambang BelandaKementerian Pendidikan, Budaya dan Ilmu PengetahuanInformasi KementerianDibentuk9 September 1918; 105 tahun lalu (1918-09-09)Wilayah hukumKerajaan BelandaKantor pusatRijnstraat 50, Den Haag, BelandaAnggaran tahunan€31,4 miliar (2013)[1]MenteriRobbert Dijkgraaf, Menteri Pendidikan, Kebudayaan, dan Ilmu PengetahuanMariëlle Paul, MenteriWakil Menterilowong, Sekret...

American public health advocate (1862–1954) This article may require cleanup to meet Wikipedia's quality standards. The specific problem is: Family section is significantly too long for encyclopedic purposes. Please help improve this article if you can. (August 2022) (Learn how and when to remove this message) Newspaper photo of Dr. Crumbine, published in 1921 Brick - Don't Spit on the Sidewalk in the collection of the National Museum of Health and Medicine Samuel Jay Crumbine (September 17...

Manuscript fragments from 32BC–640AD found in an Egyptian rubbish dump Grenfell (left) and Hunt (right) in about 1896 Oxyrhynchusclass=notpageimage| Site where the Oxyrhynchus Papyri were discovered Excavations at Oxyrhynchus 1, c. 1903. The Oxyrhynchus Papyri are a group of manuscripts discovered during the late nineteenth and early twentieth centuries by papyrologists Bernard Pyne Grenfell and Arthur Surridge Hunt at an ancient rubbish dump near Oxyrhynchus in Egypt (28°32′N 30°...

Komando Distrik Militer 0813/BojonegoroNegara IndonesiaAliansiKorem 082/CPYJCabang TNI Angkatan DaratTipe unitKodimPeranSatuan TeritorialBagian dariKodam V/BrawijayaMakodimBojonegoroPelindungTentara Nasional IndonesiaBaret H I J A U Situs webwww.kodim0813bojonegoro.mil.id KOMANDO DISTRIK MILITER 0813 BOJONEGORO atau Kodim 0813/Bojonegoro adalah Kodim yang berada di bawah Korem 082/Citra Panca Yudha Jaya. Markas Kodim 0813/Bojonegoro terletak di Jalan Hos Cokroaminoto, Bojonegor...

This article does not cite any sources. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Moldavian-Muntenian Carpathians – news · newspapers · books · scholar · JSTOR (August 2021) (Learn how and when to remove this message) Moldavian-Muntenian Carpathians, marked in red and labeled with D See also: Romanian Carpathians The Moldavian-Muntenian Carpathians are a grou...

Сельское поселение России (МО 2-го уровня)Новотитаровское сельское поселение Флаг[d] Герб 45°14′09″ с. ш. 38°58′16″ в. д.HGЯO Страна Россия Субъект РФ Краснодарский край Район Динской Включает 4 населённых пункта Адм. центр Новотитаровская Глава сельского пос�...

The State Intelligence Agency (SIA) (Bulgarian: Държавна агенция Разузнаване, romanized: Dǎržavna Agencija Razuznavane) is a Bulgarian foreign intelligence service, which obtains, processes, analyzes and provides the state leadership with intelligence, assessments, analyses and prognoses, related to the national security, interests and priorities of the Republic of Bulgaria.[1] Seal of The State Intelligence Agency History The history of intelligence a...

Disambiguazione – Se stai cercando l'omonimo videogioco, vedi Dragster (videogioco). Un dragster della Caterpillar Il dragster è un tipo di veicolo da competizione utilizzato per gare di accelerazione. Ha un motore scoperto nel quale il carburante viene iniettato nei cilindri tramite iniezione meccanica continua, ed è dotato di singola o doppia accensione, a seconda della classe.[1] Indice 1 Descrizione 2 Note 3 Voci correlate 4 Altri progetti 5 Collegamenti esterni Descrizione L...

本條目存在以下問題,請協助改善本條目或在討論頁針對議題發表看法。 此條目没有列出任何参考或来源。 (2010年12月8日)維基百科所有的內容都應該可供查證。请协助補充可靠来源以改善这篇条目。无法查证的內容可能會因為異議提出而被移除。 此條目需要擴充。 (2010年12月8日)请協助改善这篇條目,更進一步的信息可能會在討論頁或扩充请求中找到。请在擴充條目後將此�...

Avianca Penerbangan 052Puing-puing dari pesawat tersebut di sisi bukit Cove NeckRingkasan kecelakaanTanggal25 Januari 1990RingkasanKehabisan bahan bakarLokasiCove Neck, New York, Amerika Serikat 40°52′48″N 073°29′43″W / 40.88000°N 73.49528°W / 40.88000; -73.49528Penumpang149Awak9Cedera85Tewas73Selamat85Jenis pesawatBoeing 707-321BOperatorAviancaRegistrasiHK-2016AsalBandar Udara Internasional El DoradoPerhentianBandar Udara José María Córdova Int'lTu...

1989 film by R. Sundarrajan Rajadhi RajaTheatrical release posterDirected byR. SundarrajanScreenplay byPanchu ArunachalamStory byR. SundarrajanProduced byR. D. BhaskarStarringRajinikanthNadhiyaRadhaCinematographyRajarajanEdited byB. LeninV. T. VijayanMusic byIlaiyaraajaProductioncompanyPavalar CreationsRelease date 4 March 1989 (1989-03-04) CountryIndiaLanguageTamil Rajadhi Raja (transl. Superior King) is a 1989 Indian Tamil-language masala film directed by R. Sundarrajan...

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (سبتمبر 2021) أسماء جميل رشيد معلومات شخصية مواطنة عراقية الحياة العملية المدرسة الأم جامعة بغداد المهنة أستاذة جامعية اللغات العربية بوابة الأدب تعديل مصدري - تعديل ...

Chairman of Taiwan Statebuilding Party Wang Hsing-huan王興煥2nd Chairperson of the Taiwan Statebuilding PartyIncumbentAssumed office 17 January 2023Preceded byChen Yi-chi2nd Secretary-General of the Taiwan Statebuilding PartyIn office25 March 2020 – 17 January 2023Preceded byChen Hsin-yu [zh]Succeeded byVacant Personal detailsPolitical partyTaiwan Statebuilding Party Wang Hsing-huan (Chinese and Taiwanese: 王興煥, Pe̍h-ōe-jī: Ông Heng-hoàn) is a Taiwanes...