Koeffizienten für Differenzenquotienten
|
Read other articles:

Royal Secret AgentPoster promosiNama alternatifBlade of the Phantom Master New Secret Royal Agent New AmbassadorHangul암행어사: 조선비밀수사단 Hanja暗行御史: 朝鮮秘密搜查團 GenreSejarahKomediDetektifPembuatKBS Drama HeadquartersDitulis olehKang Min-sunPark Sung-hoonSutradaraKim Jung-minPemeranKim Myung-sooKwon NaraLee Yi-kyungLee Tae-hwanJo Soo-minPenata musikLee Ji-yongNegara asalKorea SelatanBahasa asliKoreaJmlh. episode16ProduksiProduser eksekutifYoon Jae-hyukP...

New Zealand political candidatesin the MMP era 1996 party lists by electorate 1999 party lists by electorate 2002 party lists by electorate 2005 party lists by electorate 2008 party lists by electorate 2011 party lists by electorate 2014 party lists by electorate 2017 party lists by electorate 2020 party lists by electorate 2023 party lists by electorate...

Alleged UFO incident in the United States Artist impression of the Maury Island UFO incident The Maury Island incident refers to claims made by Fred Crisman and Harold Dahl of falling debris and threats by men in black following sightings of unidentified flying objects in the sky over Maury Island in Puget Sound. The pair would later claim the events had occurred on June 21, 1947. Background Main articles: 1947 flying disc craze, Kenneth Arnold UFO sighting, and Flight 105 UFO sighting 1947 ...

French rugby league player (born 1934) George AillèresGeorge Aillères playing against Great Britain in Wigan, 1967Personal informationBorn (1934-12-03) 3 December 1934 (age 89)Poucharramet, Haute-Garonne, FrancePlaying informationRugby unionPositionProp, Lock Club Years Team Pld T G FG P 19??–19?? Rieumes 19?–19?? TOEC Total 0 0 0 0 0 Rugby leaguePositionProp, Second-row, Loose forward Club Years Team Pld T G FG P Toulouse Olympique Lézignan Sangliers Toulouse Olympique Total 0 0 ...
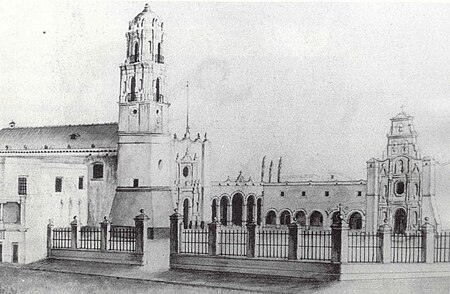
Katedral TolucaKatedral Santo Yosef dari Nazarethbahasa Spanyol: Catedral de TolucaKatedral TolucaLokasiTolucaNegaraMeksikoDenominasiGereja Katolik RomaSejarahTanggal dedikasi11 April 1976 (1976-04-11)ArsitekturStatusKatedralStatus fungsionalAktifPeletakan batu pertama12 Mei 1867Selesai1978AdministrasiDivisiKeuskupan Agung Toluca Katedral Toluca, secara resmi bernama Katedral Santo Yusuf dari Nazareth (bahasa Spanyol: Catedral de San José de Nazaret)[1] adalah sebuah ger...

本表是動態列表,或許永遠不會完結。歡迎您參考可靠來源來查漏補缺。 潛伏於中華民國國軍中的中共間諜列表收錄根據公開資料來源,曾潛伏於中華民國國軍、被中國共產黨聲稱或承認,或者遭中華民國政府調查審判,為中華人民共和國和中國人民解放軍進行間諜行為的人物。以下列表以現今可查知時間為準,正確的間諜活動或洩漏機密時間可能早於或晚於以下所歸�...

Pemisahan gas terkompresi menjadi aliran panas dan aliran dingin. Tabung vorteks, atau dikenal juga sebagai tabung vorteks Ranque-Hilsch, adalah alat mekanis yang memisahkan gas terkompresi menjadi aliran panas dan aliran dingin. Gas yang muncul dari ujung panas dapat mencapai suhu 200 °C, dan gas yang muncul dari ujung dingin bisa mencapai −50 °C.[1] Alat ini bekerja tanpa menggunakan komponen yang bergerak sehingga memiliki keunggulan tidak akan terjadi kerusakan akibat ges...

For the musical term, see Canso (song). This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Canzo – news · newspapers · books · scholar · JSTOR (August 2019) (Learn how and when to remove this message) Comune in Lombardy, ItalyCanzo Canz (Lombard)ComuneComune di CanzoClockwise from top: Canzo skyline; an hi...

2011 studio album by Jon StevensChanging TimesStudio album by Jon StevensReleased9 September 2011GenreRock music, Soul music, R&BLabelCircle Music, Universal Music AustraliaJon Stevens chronology The Works(2005) Changing Times(2011) Testify!(2011) Singles from Changing Times Just a ManReleased: August 2011 Changing Times is the seventh studio album by Australian singer-songwriter, Jon Stevens. The album was released digitally only on 9 September 2011. It was later released on CD ...

Metal or wooden device used with muzzleloading firearms For other uses, see Ramrod (disambiguation). This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Ramrod – news · newspapers · books · scholar · JSTOR (May 2016) (Learn how and when to remove this message) A countersunk ramrod, used in the 19th century for c...

20th quadrennial U.S. presidential election 1864 United States presidential election ← 1860 November 8, 1864 1868 → 234 members[a] (+17 invalidated)[b] of the Electoral College118 electoral votes needed to winTurnout73.8%[3] 7.4 pp Nominee Abraham Lincoln George B. McClellan Party National Union Democratic Alliance Parties Republican War Democrats Unconditional Union Home state Illinois New Jersey Running mate Andrew Johnson Georg...

Questa voce sull'argomento Siracusa è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti del progetto di riferimento. Questa voce o sezione sull'argomento provincia di Siracusa non cita le fonti necessarie o quelle presenti sono insufficienti. Puoi migliorare questa voce aggiungendo citazioni da fonti attendibili secondo le linee guida sull'uso delle fonti. Fontane BiancheLa spiaggia di Fontane Bianche Stato Italia Regione Si...

American TV series or program George Burns Comedy WeekPresented byGeorge BurnsCountry of originUnited StatesNo. of seasons1No. of episodes13ProductionExecutive producers Carl Gottlieb Steve Martin Running time30 min.Production companies 40 Share Productions Universal Television Original releaseNetworkCBSReleaseSeptember 18 (1985-09-18) –December 25, 1985 (1985-12-25) George Burns Comedy Week is a comedy anthology television series broadcast in the United States by CBS as par...

لمعانٍ أخرى، طالع العر (توضيح). العر (محلة) تقسيم إداري البلد اليمن المحافظة محافظة إب المديرية مديرية العدين العزلة عزلة جبل بحري القرية قرية وادي رباد السكان التعداد السكاني 2004 السكان 35 • الذكور 12 • الإناث 23 • عدد الأسر 6 • عدد المساكن 6 معلومات أخ...

كأس روسيا لكرة القدم 1996–97 تفاصيل الموسم كأس روسيا النسخة 5 البلد روسيا التاريخ بداية:17 أبريل 1996 نهاية:11 يونيو 1997 المنظم الاتحاد الروسي لكرة القدم البطل لوكوموتيف موسكو مباريات ملعوبة 285 عدد المشاركين 158 كأس روسيا لكرة القدم 1995–96 كأس روسيا ...

Government actions See also: U.S. military response during the September 11 attacks U.S. President Bush speaks with New York governor George Pataki and New York City mayor Rudy Giuliani two days following the September 11 attacks, on September 13, 2001 After the September 11, 2001 attacks, the United States government responded by commencing immediate rescue operations at the World Trade Center site, grounding civilian aircraft, and beginning a long-term response that included official invest...

Form of personal adornment For other uses, see Jewellery (disambiguation). Various examples of jewellery throughout history Jewellery (or jewelry in American English) consists of decorative items worn for personal adornment, such as brooches, rings, necklaces, earrings, pendants, bracelets, and cufflinks. Jewellery may be attached to the body or the clothes. From a western perspective, the term is restricted to durable ornaments, excluding flowers for example. For many centuries metal such as...

此條目可能包含原创研究。 (2013年2月17日)请协助補充参考资料、添加相关内联标签和删除原创研究内容以改善这篇条目。详细情况请参见讨论页。 此條目没有列出任何参考或来源。 (2013年2月17日)維基百科所有的內容都應該可供查證。请协助補充可靠来源以改善这篇条目。无法查证的內容可能會因為異議提出而被移除。 諺文優越主義,出現於韓國,認為諺文是世界上最優越�...

Act of Parliament in New Zealand Historic Places Act 1993New Zealand Parliament Long title An Act—(a) To promote the identification, protection, preservation, and conservation of the historical and cultural heritage of New Zealand; and(b) To continue the New Zealand Historic Places Trust and the New Zealand Historic Places Board of Trustees with the functions and powers necessary for the full and proper attainment of the objectives of this Act; and(c) To establish the Maori Heritage Council...

Timo Gebhart Gebhart dengan 1. FC Nürnberg di tahun 2013Informasi pribadiNama lengkap Timo GebhartTanggal lahir 12 April 1989 (umur 35)Tempat lahir Memmingen, JermanTinggi 1,82 m (5 ft 11+1⁄2 in)Posisi bermain GelandangKarier junior1994-2001 FC Memmingen2001-2004 BSC Memmingen2004–2007 1860 MunichKarier senior*Tahun Tim Tampil (Gol)2007–2008 1860 Munich II 5 (1)2007–2009 1860 Munich 37 (5)2009–2012 VfB Stuttgart 76 (4)2012–2016 1. FC Nürnberg 32 (2)2016 ...
























![{\displaystyle s=[-3,-2,-1,0,1]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/0755a78dd45f6b4c6e64c8fc2f2d15a68dee9893)



