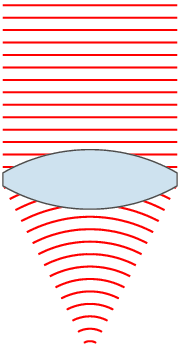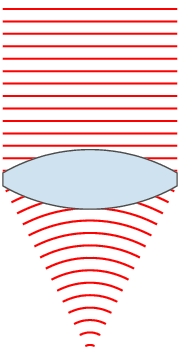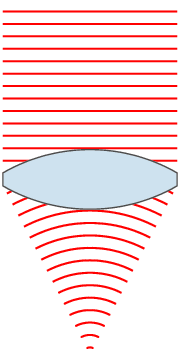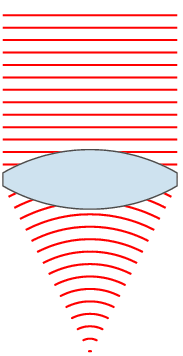
Wavefront
|
Read other articles:

Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: Ahmad Sukardja – berita · surat kabar · buku · cendekiawan · JSTOR Yang Mulia Prof. Dr.Ahmad SukardjaS.H., M.A.Hakim Agung IndonesiaMasa jabatan18 Juni 2003 – 16 September 2012Ditunjuk olehMeg...

Variety of grape Madeleine RoyaleGrape (Vitis)Handbuch der Tafeltraubenkultur, Berlin, Paul Parey, 1894SpeciesVitis viniferaAlso calledKönigliche Magdalenentraube (more)OriginFranceNotable winesTable/ornamental grapeVIVC number7068 Madeleine Royale is a variety of white grape. It is mostly grown for table grapes or ornamental purposes, but is notable as a parent of Müller-Thurgau and Madeleine Angevine. It ripens extremely early, in some cases by the 22 July, the feast day of Mary Magdalene...

Маленков Георгій Максиміліановичрос. Георгий Максимилианович Маленков Народився 8 січня 1902(1902-01-08)[1][2][…]Оренбург, Оренбурзька губернія, Російська імперіяПомер 14 січня 1988(1988-01-14)[4][5][…] (86 років)Москва, СРСР[3]Поховання Кунцевське кладовищеКраїна Р�...

Republik Yamanالجمهورية اليمنيةAl-Jumhūrīyah al-Yamanīyah (Arab) Bendera Lambang Semboyan: الله، الوَطَن، الثَورة، الوَحدة Allah, al-Waṭan, ats-Tsaurah, al-Waḥdah(Arab: Allah, Negara, Revolusi, Persatuan)Lagu kebangsaan: الجمهورية المتحدة Al-Jumhūrīyah al-Muttaḥidâh (Indonesia: Republik Persatuan) Perlihatkan BumiPerlihatkan peta BenderaIbu kota(dan kota terbesar)Sana'a15°17′N 44°24′E / 15...

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (أبريل 2016) البنك الروماني للتطويرالشعارمعلومات عامةالجنسية رومانيا التأسيس 19 يونيو 1923 النوع عمل تجاري — مؤسسة الائتمان — بنك — مؤسسة مالية المقر الرئيسي بوخارست �...

لاري بلوك معلومات شخصية اسم الولادة (بالإنجليزية: Lawrence Joel Block) الميلاد 30 أكتوبر 1942(1942-10-30)نيويورك، نيويورك الوفاة 7 أكتوبر 2012 (69 سنة)نيويورك مواطنة الولايات المتحدة الحياة العملية المهنة ممثل اللغة الأم الإنجليزية اللغات الإنجليزية سنوات النشاط 1971-2012 المواقع I...

Vlaardingen WestRotterdam Metro stationGeneral informationLocationNetherlandsCoordinates51°54′14″N 4°18′51″E / 51.90389°N 4.31417°E / 51.90389; 4.31417Line(s)Schiedam–Hoek van Holland railwayPlatforms1 island platform 1 side platformTracks3HistoryOpened1 June 1969Closed1 April 2017Rebuilt30 September 2019Services Preceding station Rotterdam Metro Following station From January 2019 Terminus Line ANot on evenings and early weekend mornings Vlaardingen Cent...

Island of Croatia This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Male Srakane – news · newspapers · books · scholar · JSTOR (December 2009) (Learn how and when to remove this template message) This article is about the island and the eponymous village. For the village near Čavle, see Ilovik, Čavle. Male S...

This article is an orphan, as no other articles link to it. Please introduce links to this page from related articles; try the Find link tool for suggestions. (March 2016) Schools in West Bengal, IndiaGoutam Smriti Satpati Binapani Vidyamandir G. S. Satpati Binapani VidyamandirSchoolsSatpati High SchoolGoutam Smriti Satpati Binapani VidyamandirLocation in West Bengal, IndiaCoordinates: 22°35′N 87°03′E / 22.58°N 87.05°E / 22.58; 87.05Country IndiaStateWest ...

لويسبرغ الإحداثيات 37°48′15″N 80°26′25″W / 37.804166666667°N 80.440277777778°W / 37.804166666667; -80.440277777778 تاريخ التأسيس 1782 تقسيم إداري البلد الولايات المتحدة[1][2] التقسيم الأعلى مقاطعة غرينبريه عاصمة لـ مقاطعة غرينبريه خصائص جغرافية المساحة 9.866313 �...

قرية رهقة - قرية - تقسيم إداري البلد اليمن المحافظة محافظة المحويت المديرية مديرية ملحان العزلة عزلة الشمارية السكان التعداد السكاني 2004 السكان 961 • الذكور 492 • الإناث 469 • عدد الأسر 98 • عدد المساكن 99 معلومات أخرى التوقيت توقيت اليمن (+3 غرينيتش)...

Papua New Guinea educator DameRose KekedoDBEfrom her biography by Eric JohnsBornRosalina Violet Kekedo1942Abau, Central Province, Papua New GuineaDied25 February 2005Port MoresbyOccupation(s)Teacher, lecturer, administratorKnown forFirst woman Chancellor of the University of Papua New Guinea Dame Rosalina Violet Kekedo DBE (1942 – 25 February 2005), better known as Rose Kekedo, was a leading educator in Papua New Guinea (PNG) and the first woman to be chancellor of the University o...

Ini adalah nama Tionghoa; marganya adalah Lai. Lai Feng-wei賴峰偉Magistrat Kabupaten PenghuPetahanaMulai menjabat 25 Desember 2018PendahuluChen Kuang-fuMasa jabatan20 Desember 1997 – 20 Desember 2005PendahuluJohn Lieh ChengPenggantiWang Chien-faMenteri EksaminasiMasa jabatan29 Juli 2010 – 10 Februari 2012PendahuluYang Chao-hsiangPenggantiTung Pao-cheng Informasi pribadiLahirPenghu, Taiwan20 September 1953 (umur 70)KebangsaanRepublik TiongkokPartai politikKuom...

В Википедии есть статьи о других людях с фамилией Деникин. Иван Ефимович Деникин майор пограничной стражи Иван Ефимович Деникин Дата рождения 1807(1807) Место рождения д. Ореховка (Саратовская губерния) Дата смерти 1885(1885) Принадлежность Российская империя Род войск �...

For the horror film, see Hello Mary Lou: Prom Night II. 1961 single by Ricky NelsonHello Mary LouSingle by Ricky Nelsonfrom the album Rick Is 21 A-sideTravelin' ManReleasedMay 1961Recorded1960−61GenreRockabilly[1]Length2:17LabelImperial Records #5741Songwriter(s)Gene Pitney, Cayet MangiaracinaRicky Nelson singles chronology You Are the Only One (1960) Hello Mary Lou (1961) A Wonder Like You/Everlovin' (1961) Official audioHello Mary Lou (Remastered) on YouTube Hello Mary Lou is a so...

Hospital near Birmingham, England Hospital in EnglandSandwell General HospitalSandwell and West Birmingham Hospitals NHS TrustSandwell General Hospital's main block, August 2016Shown in West MidlandsGeographyLocationSandwell, West Midlands, England, United KingdomCoordinates52°31′40″N 1°59′20″W / 52.5277°N 1.9889°W / 52.5277; -1.9889OrganisationCare systemPublic NHSTypeDistrict GeneralAffiliated university University of Birmingham Aston University Birmingha...

History of the US state of Michigan For the magazine, see Michigan History (magazine). The Great Seal of the State of Michigan History of Michigan By year Pre-statehood U.S. Civil War Since 1900 Topics: Cities - Politics Michigan portalvteThe history of human activity in Michigan, a U.S. state in the Great Lakes, began with settlement of the western Great Lakes region by Paleo-Indians perhaps as early as 11,000 B.C.E. One early technology they developed was the use of native copper,...

Suburb of Melbourne, Victoria, AustraliaChirnside ParkMelbourne, VictoriaEdward RoadChirnside ParkCoordinates37°45′14″S 145°19′37″E / 37.754°S 145.327°E / -37.754; 145.327Population11,779 (2021 census)[1] • Density528.2/km2 (1,368.0/sq mi)Postcode(s)3116Elevation116 m (381 ft)Area22.3 km2 (8.6 sq mi)Location 38 km (24 mi) NE of Melbourne CBD (Central Melbourne) 4 km (2 mi) NW of Lily...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada November 2022. Franck OhandzaInformasi pribadiNama lengkap Franck Ohandza ZoaTanggal lahir 28 September 1991 (umur 32)Tempat lahir Ngong, KamerunTinggi 5 ft 9,25 in (1,76 m)Posisi bermain PenyerangInformasi klubKlub saat ini Greuther Fürth (pinj...

アンドラCF(英語版)とは異なります。 FCアンドラ原語表記 Futbol Club Andorra愛称 Els Tricolorsクラブカラー 青・ 黄・ 赤創設年 1942年所属リーグ セグンダ・ディビシオン所属ディビジョン 2部(2022-23)ホームタウン アンドラ・ラ・ベリャホームスタジアム エスタディ・ナシオナル収容人数 3,306代表者 ジェラール・ピケ (90%) グルー�...