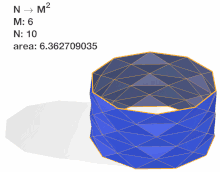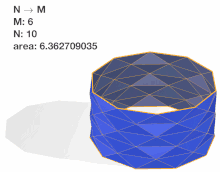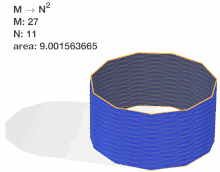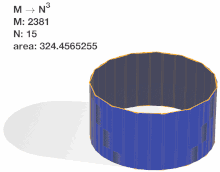
Surface area
|
Read other articles:

Le Grand-Saconnexcittà Le Grand-Saconnex – Veduta LocalizzazioneStato Svizzera Cantone Ginevra DistrettoNon presente AmministrazioneLingue ufficialifrancese TerritorioCoordinate46°14′00″N 6°08′00″E / 46.233333°N 6.133333°E46.233333; 6.133333 (Le Grand-Saconnex)Coordinate: 46°14′00″N 6°08′00″E / 46.233333°N 6.133333°E46.233333; 6.133333 (Le Grand-Saconnex) Altitudine445 m s.l.m. Superficie4,38 km² Abitanti12&#...

Cari artikel bahasa Cari berdasarkan kode ISO 639 (Uji coba) Kolom pencarian ini hanya didukung oleh beberapa antarmuka Halaman bahasa acak Bahasa Oirat ᡆᡕᡅᠷᠠᡑ ᡘᡄᠯᡄᠨ, Oirad kelen ᠮᠣᠩᠭᠣᠯ ᠬᠡᠯᠡᠨ ᠦ ᠣᠶᠢᠷᠠᠳ ᠠᠶᠠᠯᠭᠤMongγol kelen-ü Oyirad ayalγu Dituturkan diMongolia, Rusia, Tiongkok, Kyrgyzstan[1]WilayahKhovd, Uvs,[2] Bayan-Ölgii,[3] Kalmykia, Xinjiang, Gansu, QinghaiEtnisSuku Oirat (6...

Letak Haute-Marne di Prancis. Haute-Marne adalah sebuah departemen yang terletak di region Champagne-Ardenne, Prancis. Beribukotakan Chaumont, departemen ini berpenduduk 194.873 jiwa (1999). Ada 3 arrondissement, 32 kanton, dan 432 kotamadya di Haute-Marne. Wikimedia Commons memiliki media mengenai Haute-Marne. lbsDepartemen di Prancis 01 Ain 02 Aisne 03 Allier 04 Alpes-de-Haute-Provence 05 Hautes-Alpes 06 Alpes-Maritimes 07 Ardèche 08 Ardennes 09 Ariège 10 Aube 11 Aude 12 Aveyron 13 Bouche...

العلاقات الألمانية الكازاخستانية ألمانيا كازاخستان ألمانيا كازاخستان تعديل مصدري - تعديل العلاقات الألمانية الكازاخستانية هي العلاقات الثنائية التي تجمع بين ألمانيا وكازاخستان.[1][2][3][4][5] مقارنة بين البلدين هذه مقارنة عامة ومرجعية �...

Si ce bandeau n'est plus pertinent, retirez-le. Cliquez ici pour en savoir plus. Cet article sur le sport doit être recyclé (février 2022). Une réorganisation et une clarification du contenu paraissent nécessaires. Améliorez-le, discutez des points à améliorer ou précisez les sections à recycler en utilisant {{section à recycler}}. SnowboardPlanche à neige Données clés Sport olympique depuis 1998 modifier Un snowboardeur qui pratique le Jib Le snowboard, surf des neiges, planche...

Research and analysis division of Economist Group Economist Intelligence Unit LimitedCompany typeBusiness unitFounded1946HeadquartersLondon, EnglandProductsForecasting, economic research and analysisParentEconomist GroupSubsidiariesBazian, Clearstate, Canback ConsultingWebsitewww.eiu.com The Economist Intelligence Unit (EIU) is the research and analysis division of the Economist Group, providing forecasting and advisory services through research and analysis, such as monthly country reports, ...

Mokhatat Al-HaramainLingkunganNegaraArab SaudiProvinsiProvinsi MakkahPemerintahan • Wali kotaHani Abu Ras[1] • Gubernur kotaMish'al Al-SaudKetinggian12 m (39 ft)Zona waktuUTC+3 (AST) • Musim panas (DST)ASTKode pos(5 kode digit dimulai dari 23; e.g. 23434)Kode area telepon+966-12Situs webwww.jeddah.gov.sa/english/index.php Mokhatat Al-Haramain adalah sebuah permukiman padat penduduk di kota Jeddah di Provinsi Makkah, tepatnya di sebelah bar...

For the tributary of the Vilyuy, see Markha (Vilyuy). RiverMarkhaМархаMouth of the Markha in the Lena Sentinel-2 image.Mouth location in Yakutia, RussiaShow map of Sakha RepublicMarkha (Lena) (Russia)Show map of RussiaPhysical characteristicsSource • locationLena Plateau • coordinates61°59′44″N 122°00′16″E / 61.99556°N 122.00444°E / 61.99556; 122.00444 MouthLena • coordinates60°35′12″N 123...

本條目存在以下問題,請協助改善本條目或在討論頁針對議題發表看法。 此條目需要补充更多来源。 (2017年12月19日)请协助補充多方面可靠来源以改善这篇条目,无法查证的内容可能會因為异议提出而被移除。致使用者:请搜索一下条目的标题(来源搜索:若望保祿二世 — 网页、新闻、书籍、学术、图像),以检查网络上是否存在该主题的更多可靠来源(判定指引)。 �...

本條目存在以下問題,請協助改善本條目或在討論頁針對議題發表看法。 此條目的语调或风格或許不合百科全書。 (2024年1月29日)請根據指南協助改善这篇条目,並在讨论页討論問題所在,加以改善。 此生者传记条目需要补充更多可供查證的来源。 (2024年1月29日)请协助補充可靠来源,无法查证的在世人物内容将被立即移除。 此条目页的主題是中华人民共和国现任国...

西維珍尼亞 美國联邦州State of West Virginia 州旗州徽綽號:豪华之州地图中高亮部分为西維珍尼亞坐标:37°10'N-40°40'N, 77°40'W-82°40'W国家 美國加入聯邦1863年6月20日(第35个加入联邦)首府(最大城市)查爾斯頓政府 • 州长(英语:List of Governors of {{{Name}}}]]) • 副州长(英语:List of lieutenant governors of {{{Name}}}]])吉姆·賈斯蒂斯(R)米奇·卡邁克爾(...

Questa voce sull'argomento centri abitati della provincia di Buenos Aires è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Disambiguazione – Se stai cercando la città francese, vedi Boulogne-sur-Mer. Boulogne Sur MercittàBoulogne Sur Mer – Veduta LocalizzazioneStato Argentina Provincia Buenos Aires DipartimentoSan Isidro TerritorioCoordinate34°30′S 58°34′W / 34.5°S 58.566667°W-34.5; -58.566667 (Boulogne Sur Mer...

Medical conditionEpithelioid HemangioendotheliomaMicrograph of an epithelioid hemangioendothelioma of the liver.SpecialtyOncology Epithelioid hemangioendothelioma (EHE) is a rare tumor, first characterized by Sharon Weiss and Franz Enzinger in 1982[1] that both clinically and histologically is intermediate between angiosarcoma and hemangioma. However, a distinct, disease-defining genetic alteration recently described for EHE indicates that it is an entirely separate entity from both a...

1900年美國總統選舉 ← 1896 1900年11月6日 1904 → 447張選舉人票獲勝需224張選舉人票投票率73.2%[1] ▼ 6.1 % 获提名人 威廉·麥金利 威廉·詹寧斯·布賴恩 政党 共和黨 民主党 家鄉州 俄亥俄州 內布拉斯加州 竞选搭档 西奧多·羅斯福 阿德萊·史蒂文森一世 选举人票 292 155 胜出州/省 28 17 民選得票 7,228,864 6,370,932 得票率 51.6% 45.5% 總統選舉結果地圖,紅色代表�...

Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: John Bowes-Lyon – berita · surat kabar · buku · cendekiawan · JSTOR John Herbert Jock Bowes-Lyon (1 April 1886–7 Februari 1930) adalah putra kedua dari Pangeran Strathmore dan Kinghorne ke-14 dan Putri...

Nepali politician Jeevan Ram Shresthaजीवन राम श्रेष्ठMinister of Culture, Tourism and Civil AviationIn office27 June 2022 – 26 December 2022PresidentBidhya Devi BhandariPrime MinisterSher Bahadur DeubaPreceded byPrem AleSucceeded bySudan KiratiMember of Parliament, Pratinidhi SabhaIn office4 March 2018 – 18 September 2022Preceded byNabindra Raj JoshiSucceeded byBiraj Bhakta ShresthaConstituencyKathmandu 8Chair of Nepal Olympic CommitteeIncumbe...

Death penalty abolition advocate SisterHelen PrejeanCSJPrejean in 2006Born (1939-04-21) April 21, 1939 (age 85)Baton Rouge, Louisiana, U.S.EducationSt. Mary's Dominican CollegeSaint Paul UniversityOccupation(s)Sister, spiritual adviser, author, anti-death penalty activist Helen Prejean CSJ (/preɪˈʒɑːn/ pray-ZHAHN;[1] born April 21, 1939) is a Catholic religious sister and a leading American advocate for the abolition of the death penalty. She is known for her best-selling bo...

جائزة إسبانيا الكبرى 1989 (بالإسبانية: XXXI Gran Premio Tio Pepe de España) السباق 14 من أصل 16 في بطولة العالم لسباقات الفورمولا واحد موسم 1989 السلسلة بطولة العالم لسباقات فورمولا 1 موسم 1989 البلد إسبانيا التاريخ 1 أكتوبر 1989 مكان التنظيم شريش، إسبانيا طول المسار 4.218 كيلومتر (2.6209 م�...

State beach in Monterey and Santa Cruz counties, California, United States Zmudowski State BeachZmudowski State BeachShow map of CaliforniaShow map of the United StatesLocationMonterey County, United StatesNearest cityMoss LandingCoordinates36°50′36″N 121°48′17″W / 36.84333°N 121.80472°W / 36.84333; -121.80472Governing bodyCalifornia Department of Parks and Recreation Zmudowski State Beach is located on Monterey Bay, in Moss Landing, Monterey Coun...

Bugie, baci, bambole & bastardiSean Penn in una scena del filmTitolo originaleHurlyburly Paese di produzioneStati Uniti d'America Anno1998 Durata122 min Generecommedia, drammatico RegiaAnthony Drazan SceneggiaturaDavid Rabe ProduttoreAnthony Drazan, Richard N. Gladstein, David Hamburger FotografiaGu Chang Wei MontaggioDylan Tichenor MusicheDavid Baerwald, Steve Lindsey ScenografiaMichael D. Haller CostumiMary Claire Hannan Interpreti e personaggi Sean Penn: Eddie Kevin Spacey: Mickey ...