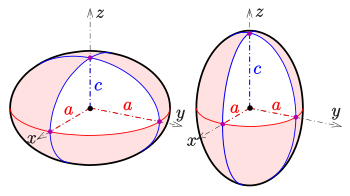
Spheroid
| |||||||
Read other articles:

Consejería de Hacienda, Economía y Administración Pública de la Generalidad Valenciana Palacio del Almirante de Valencia, sede de la Consejería de Hacienda, Economía y Administración PúblicaLocalizaciónPaís EspañaInformación generalJurisdicción Comunidad ValencianaTipo ConsejeríaSede Calle del Palau, 1446003 Valencia (España)OrganizaciónDirección Ruth Merino (Consejera)Depende de Consejo de la Generalidad ValencianaDependencias SubsecretaríaIntervención General de la G...
Diagram yang menunjukkan penataan kamera tunggal. Kamera tunggal atau penataan kamera tunggal (Inggris: single-camera setupcode: en is deprecated atau single-camera mode of production), juga dikenal sebagai portable single camera, adalah metode pembuatan film dan produksi video. Penataan kamera tunggal awalnya dikembangkan semasa kemunculan sinema Hollywood klasik pada tahun 1910-an dan tetap menjadi mode produksi standar untuk sinema. Dalam produksi televisi, metode kamera tunggal dan multi ...

Gaya atau nada penulisan artikel ini tidak mengikuti gaya dan nada penulisan ensiklopedis yang diberlakukan di Wikipedia. Bantulah memperbaikinya berdasarkan panduan penulisan artikel. (Pelajari cara dan kapan saatnya untuk menghapus pesan templat ini) Eyang Hasan Maulani Lengkong adalah ahli sufi yang hidup sekitar tahun 1800 an, beliau adalah penasihat Imam Bonjol dan ditangkap serta diasingkan oleh belanda serta meninggal di Menado sulawesi Tenggara. Beliau adalah tokoh berpengaruh dan men...

City in California, United States This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Menifee, California – news · newspapers · books · scholar · JSTOR (November 2023) (Learn how and when to remove this template message) City in California, United StatesMenifee, CaliforniaCityCity of MenifeeNewport Road looking ...

Синелобый амазон Научная классификация Домен:ЭукариотыЦарство:ЖивотныеПодцарство:ЭуметазоиБез ранга:Двусторонне-симметричныеБез ранга:ВторичноротыеТип:ХордовыеПодтип:ПозвоночныеИнфратип:ЧелюстноротыеНадкласс:ЧетвероногиеКлада:АмниотыКлада:ЗавропсидыКласс:Пт�...

Pour les articles homonymes, voir Heidelberg (homonymie). Heidelberg Vue sur Heidelberg. Armoiries Drapeau Administration Pays Allemagne Land Bade-Wurtemberg District(Regierungsbezirk) Karlsruhe Arrondissement(Landkreis) Heidelberg (ville-arrondissement) Nombre de quartiers(Ortsteile) 15 Bourgmestre(Bürgermeister) Eckart Würzner Code postal 69115-69126 Code communal(Gemeindeschlüssel) 08 2 21 000 Indicatif téléphonique 06221 Immatriculation HD Démographie Gentilé Heidelbergeois Popula...

Pour les articles homonymes, voir Volonté. Ne doit pas être confondu avec volition. Si ce bandeau n'est plus pertinent, retirez-le. Cliquez ici pour en savoir plus. Cet article ne cite pas suffisamment ses sources (octobre 2021). Si vous disposez d'ouvrages ou d'articles de référence ou si vous connaissez des sites web de qualité traitant du thème abordé ici, merci de compléter l'article en donnant les références utiles à sa vérifiabilité et en les liant à la section « No...

Fatehpuri MosqueReligionAffiliationSunni IslamDistrictCentral DelhiLocationLocationChandni Chowk, Old DelhiStateDelhiCountryIndiaLocation in Delhi, IndiaShow map of DelhiFatehpuri Mosque (India)Show map of IndiaGeographic coordinates28°39′24.0″N 77°13′21.4″E / 28.656667°N 77.222611°E / 28.656667; 77.222611ArchitectureTypeMosqueStyleMughal architectureCreatorFatehpuri Begum (wife of Shahjahan)Date established1650; 374 years ago (1650) Hist...

1958 Japanese filmThe White Snake EnchantressTheatrical posterDirected byTaiji Yabushita [ja]Screenplay byTaiji YabushitaStory byShin UeharaBased onLegend of the White SnakeProduced byHiroshi ÔkawaStarringHisaya MorishigeMariko MiyagiNarrated byHisaya MorishigeCinematographyTakamitsu TsukaharaEdited byShinataro MiyamotoMusic byChuji KinoshitaHajime KaburagiMasayoshi IkedaProductioncompanyToei DogaDistributed byToei CompanyRelease date October 22, 1958 (1958-10-22)...

Food delivery and restaurant company Hello Curry Pvt. Ltd.IndustryFood delivery and restaurantFoundedEarly 2014FoundersSandeep Penumatsa & Raju BhupathiHeadquartersHyderabad, IndiaWebsitewww.hellocurry.com/index.php[dead link] Hello Curry Pvt. Ltd. is a food delivery and restaurant company based in Hyderabad, India.[1][2][3] The company was founded in early 2014, and in November 2015 had 32 outlets for its food delivery operations.[4][5] Far...

Sekolah Tinggi Agama Buddha NalandaDidirikanMei 1979KetuaDr. Sutrisno, S.IP., M.Si.AlamatJl. Pulo Gebang No.107, RT.13/RW.4, Pulo Gebang, Kec. Cakung, Kota Jakarta Timur, Daerah Khusus Ibukota Jakarta, Jakarta, DKI JakartaSitus webhttps://stabnalanda.ac.id https://nalanda.ac.id Sekolah Tinggi Agama Buddha Nalanda atau STAB Nalanda adalah sekolah tinggi agama Buddha yang pertama di Indonesia. Beralamatkan di Jl. Pulo Gebang No.107, RT.13/RW.4, Pulo Gebang, Kec. Cakung, Kota Jakarta Timur, Daer...

Fictional character from the British soap opera EastEnders Soap opera character Mel OwenEastEnders characterPortrayed byTamzin OuthwaiteDuration1998–2002, 2018–2019First appearanceEpisode 168319 October 1998 (1998-10-19)Last appearanceEpisode 602114 November 2019 (2019-11-14)ClassificationFormer; regularIntroduced byMatthew Robinson (1998)John Yorke (2018)Book appearancesSteve Owen: Still Waters (2001)Spin-offappearancesEastEnders: Th...

خريطة توضح أعداد المسيحيين اللاطائفيين في 2010 أكثر من 10 مليون أكثر من 1 مليون مسيحية لا طائفية (بالإنجليزية: Nondenominational Christianity) هُم المسيحيون الذين يصرحون بمسيحيتهم لكن يرفضون أن ينتموا إلى طائفة ويصنفون أيضاً كبروتستانت ويقولون أنهم مسيحيين بطريقتهم الخاصة م�...

16th-century BC royal cemetery in southern Greece Grave Circle ANative name Greek: Ταφικός περίβολος A'Grave Circle A (left) and the main entrance of the citadel (right)LocationMycenaeCoordinates37°43′49″N 22°45′22″E / 37.73028°N 22.75611°E / 37.73028; 22.75611AreaArgolis, GreeceFormed16th century BCBuilt forResting place of the Mycenaean ruling familiesLocation of Grave Circle A in Greece Grave Circle A is a 16th-century BC royal cemetery s...

Politeknik Pelayaran BantenNama sebelumnyaBalai Pendidikan dan Pelatihan Ilmu Pelayaran (BP2IP) TangerangMotoPatria Sapta Bahari BhaktiMoto dalam bahasa IndonesiaBakti Seorang Pelaut Yang TangguhDidirikan27 Oktober 2002Lembaga indukKementerian Perhubungan Republik IndonesiaAlamatJalan Raya Karang Serang No.1, Tangerang, Banten, IndonesiaSitus webpoltekpel-banten.ac.id Politeknik Pelayaran Banten[1] adalah institusi perguruan tinggi kedinasan di bidang maritim dan transportasi lau...

This article relies excessively on references to primary sources. Please improve this article by adding secondary or tertiary sources. Find sources: Homosexuality in the militaries of ancient Greece – news · newspapers · books · scholar · JSTOR (June 2015) (Learn how and when to remove this message) Homosexuality in the militaries of ancient Greece was a significant aspect across the ancient Greek city-states, ranging from being a core part of militar...

Sedimentary rock exposure in Colorado and Utah, U.S. For AMD's APU codename, see AMD Raven Ridge. For the place in Washington state, see Raven Ridge (Washington). Raven Ridge. NASA satellite photo, 2008.[1] Raven Ridge is a starkly visible sedimentary rock exposure located in Rio Blanco County, Colorado and Uintah County, Utah, USA. It is managed by the Bureau of Land Management. The ridge contains a diverse selection of rare plants unique to the state of Colorado. Geology The Raven R...

Provinsi Badakhshan بدخشانProvinsiSuasan beberapa distrik di Provinsi BadakhshanPeta Afghanistan dengan Dataran tinggi BadakhshanKoordinat: 38°0′N 71°0′E / 38.000°N 71.000°E / 38.000; 71.000Negara AfghanistanIbukotaFayzabadPemerintahan • GubernurMaulvi Abdul Ghani Faiq[1] • Wakil GubernurNisar Ahmad Ahmadi[2]Luas[3] • Total44.059 km2 (17,011 sq mi)Populasi (2021)[4 ...

العلاقات الفيتنامية النيجيرية فيتنام نيجيريا فيتنام نيجيريا تعديل مصدري - تعديل العلاقات الفيتنامية النيجيرية هي العلاقات الثنائية التي تجمع بين فيتنام ونيجيريا.[1][2][3][4][5] مقارنة بين البلدين هذه مقارنة عامة ومرجعية للدولتين: وجه الم...

Paul Hodes Paul William Hodes[1] (lahir 21 Maret 1951) adalah seorang pengacara, musisi dan mantan anggota DPR Amerika Serikat, yang menjabat dari 2007 sampai 2011. Ia adalah anggota Partai Demokrat. Referensi ^ Paul William Hodes (D). The Washington Post. Diarsipkan dari versi asli tanggal 2012-07-13. Parameter |url-status= yang tidak diketahui akan diabaikan (bantuan) Pranala luar Wikimedia Commons memiliki media mengenai Paul Hodes. Paul Hodes for US Senate official camp...





![{\displaystyle C_{\text{v}}=2{\sqrt[{3}]{a^{2}c}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/271e21c03f9e1f8e341ed781367399f38cf3ec78)













