P-adic L-function
|
Read other articles:

Cultural center in Columbus, Ohio Ohio State Arsenal redirects here. For the federal arsenal in Columbus, see Fort Hayes. Priscilla R. TysonCultural Arts CenterFormer nameOhio State ArsenalEstablished1976Location139 West Main Street Columbus, Ohio 43215 USATypeCultural centerPublic transit access 3, 6, 9 CoGoWebsiteOfficial website Ohio State ArsenalU.S. National Register of Historic PlacesColumbus Register of Historic Properties The art center among Scioto Mile parksCoordinates39°57′19″...
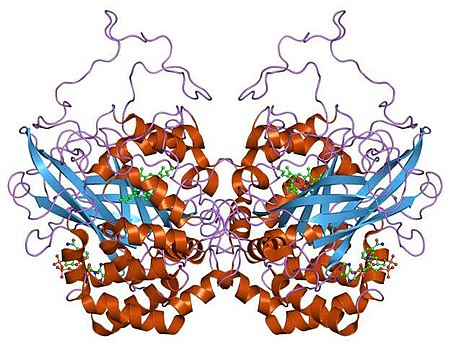
KatalaseIdentifikasiSimbolCatalasePfamPF00199InterProIPR011614PROSITEPDOC00395SCOP7catSUPERFAMILY7catOPM superfamily435OPM protein3e4wCDDcd00328Struktur protein yang tersedia:PfamstrukturPDBRCSB PDB; PDBe; PDBjPDBsumringkasan struktur CatalasePengidentifikasiNomor EC1.11.1.6Nomor CAS9001-05-2 Basis dataIntEnztinjauan IntEnzBRENDAentri BRENDAExPASytinjauan NiceZymeKEGGentri KEGGMetaCycjalur metabolikPRIAMprofilStruktur PDBRCSB PDB PDBe PDBsumOntologi GenAmiGO / EGO PencarianPMCartikelPubMedart...

サン・マウリーツィオ・カナヴェーゼSan Maurizio Canavese行政国 イタリア州 ピエモンテ県/大都市 トリノCAP(郵便番号) 10077市外局番 011ISTATコード 001248識別コード I024分離集落 Ceretta, Malanghero隣接コムーネ #隣接コムーネ参照地震分類 zona 4 (sismicità molto bassa)気候分類 zona E, 2830 GG公式サイト リンク人口人口 10,279 [1] 人 (2020-01-01)人口密度 587 人/km2文化住民の呼称 sanmauri...

808s and Heartbreak Album de Kanye West Sortie 24 novembre 2008 Enregistré 2008• Glenwood Studios, Burbank, Californie)• Avex Recording Studio Honolulu, Hawaï Durée 52:01 Genre Pop, synthpop, electropop, electronica, RnB Format CD Producteur Kanye West, Jeff Bhasker, Plain Pat, No I.D., Mr Hudson Label Roc-A-Fella, Island Def Jam Albums de Kanye West Graduation(2007) VH1 Storytellers(2010)Singles Love LockdownSortie : 18 septembre 2008 HeartlessSortie : 4 novembre 2...

У Вікіпедії є статті про інші значення цього терміна: Струтинка. село Струтинка Країна Україна Область Одеська область Район Подільський район Громада Савранська селищна громада Код КАТОТТГ UA51120210200061388 Облікова картка Струтинка (Савранська селищна громада) Ос�...

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (يونيو 2019) موريس باريت معلومات شخصية الميلاد 17 فبراير 1956 مونتريال تاريخ الوفاة 30 سبتمبر 2018 (62 سنة) [1] مواطنة كندا الوزن 150 رطل الحياة العملية المهنة �...

Seorang pelayan kopi, dari wilayah Utsmaniyah di Kairo, Mesir pada tahun 1857. Sebuah rumah kopi di Damaskus. Sebuah Rumah kopi Wina Les Deux Magots di Paris, sempat menjadi tempat perkumpulan para intelektual Prancis yang terkenal Sebuah kafe kopi di Melbourne. Kota tersebut dikatakan memiliki beberapa kopi terbaik di dunia. Budaya kopi mendeskripsikan atmosfer sosial atau serangkaian perilaku sosial terkait yang sangat bergantung pada kopi, sebagian besar sebagai sebuah lubrikan sosial. Ist...

Christian Feest (2015) in San Cristobal de las Casas, Mexico Christian Feest (born July 20, 1945) is an Austrian ethnologist and ethnohistorian. Biography Feest was born on July 20, 1945, in Broumov. He specializes in the Native Americans of eastern North America and the Northeastern United States and their material culture, ethnological image research and Native American anthropology of art. He is widely acknowledged for his pioneering research and publications on the early European-Native A...

Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: Daftar Ketua Umum Partai Kebangkitan Bangsa – berita · surat kabar · buku · cendekiawan · JSTOR Ketua Umum Partai Kebangkitan BangsaPetahanaMuhaimin Iskandarsejak 25 Mei 2005KediamanGedung DPP PKBMa...

Citrus fruit and plant This article is about the citrus fruit and plant. For other uses, see Yuzu (disambiguation). This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Yuzu – news · newspapers · books · scholar · JSTOR (July 2020) (Learn how and when to remove this template message) Yuzu Scientific classificatio...

Prominent person or group who commands some degree of public fascination and appears in the media For other uses, see Celebrity (disambiguation). Television personalities redirects here. For the band, see Television Personalities. Star (person) redirects here. For people named Star, see Star (name). Celebrity is a condition of fame and broad public recognition of a person or group as a result of the attention given to them by mass media. An individual may attain a celebrity status from having...

This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Star Trek: The Next Generation 1993 video game – news · newspapers · books · scholar · JSTOR (July 2008) (Learn how and when to remove this template message) 1993 video gameStar Trek: The Next GenerationNorth American cover artDeveloper(s)Imagineering Inc....

This article includes a list of general references, but it lacks sufficient corresponding inline citations. Please help to improve this article by introducing more precise citations. (October 2021) (Learn how and when to remove this template message) Vox Mando-Guitar An octave twelve is a type of 12-string guitar fitted with a short-scale neck (15.5 inches) and a small solid body. It is tuned one octave higher than a standard guitar, giving it the tonal range of a mandolin and enabling a guit...

Building in Mong Kok, Hong Kong 22°19′25″N 114°10′08″E / 22.323475°N 114.168815°E / 22.323475; 114.168815 Not to be confused with Union Square (Hong Kong). For the building in San Diego, California, see First Allied Plaza. Allied PlazaChinese: 聯合廣場Allied Plaza (full view)General informationTypeShopping centreLocationIntersection of Nathan Road and Nullah Road in Mong Kok, Yau Tsim Mong DistrictAddress760 Nathan RoadCountry Hong Kong Allied ...

Lac ChambonLac ChambonLocationPuy-de-DômeCoordinates45°34′13″N 2°55′18″E / 45.57028°N 2.92167°E / 45.57028; 2.92167Primary outflowsCouze ChambonBasin countriesFranceSurface area0.6 km2 (0.23 sq mi)Max. depth4 m (13 ft)Surface elevation877 m (2,877 ft) This article does not cite any sources. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find ...

Barus UtaraKecamatanPeta lokasi Kecamatan Barus UtaraNegara IndonesiaProvinsiSumatera UtaraKabupatenTapanuli TengahPemerintahan • CamatTodo Marihot SipahutarPopulasi (2020)[1] • Total4.958 jiwa • Kepadatan78,67/km2 (203,8/sq mi)Kode Kemendagri12.01.17 Kode BPS1204073 Luas63,02 km²Desa/kelurahan6 Desa Barus Utara adalah sebuah kecamatan yang berada di kabupaten Tapanuli Tengah, provinsi Sumatera Utara, Indonesia. Ibu kota kecamatan in...

This article relies excessively on references to primary sources. Please improve this article by adding secondary or tertiary sources. Find sources: MasterChef Croatia – news · newspapers · books · scholar · JSTOR (April 2011) (Learn how and when to remove this template message) Croatian TV series or program MasterChef CroatiaGenreCookeryJudgesTomislav GretićMate JankovićRadovan MarčićCountry of originCroatiaOriginal languageCroatianNo. of sea...

Artikel ini berisi konten yang ditulis dengan gaya sebuah iklan. Bantulah memperbaiki artikel ini dengan menghapus konten yang dianggap sebagai spam dan pranala luar yang tidak sesuai, dan tambahkan konten ensiklopedis yang ditulis dari sudut pandang netral dan sesuai dengan kebijakan Wikipedia. Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari ...

Den här artikeln har skapats av Lsjbot, ett program (en robot) för automatisk redigering. (2016-10)Artikeln kan innehålla fakta- eller språkfel, eller ett märkligt urval av fakta, källor eller bilder. Mallen kan avlägsnas efter en kontroll av innehållet (vidare information) Ambo Village Ort Land Kiribati Ögrupp Gilbertöarna Öråd Tarawa Höjdläge 13 m ö.h. Koordinater 1°21′11″N 173°02′33″Ö / 1.35317°N 173.04259°Ö / 1.35317; 1...
Paghimo ni bot Lsjbot. Alang sa ubang mga dapit sa mao gihapon nga ngalan, tan-awa ang Cedar Ridge. 38°03′57″N 120°16′37″W / 38.06576°N 120.27686°W / 38.06576; -120.27686 Cedar Ridge Lungsod Nasod Tinipong Bansa Estado California Kondado Tuolumne County Gitas-on 1,133 m (3,717 ft) Tiganos 38°03′57″N 120°16′37″W / 38.06576°N 120.27686°W / 38.06576; -120.27686 Population 1,132 (2011-05-14) [1] Timezon...




