Nicomachus
| |||||||||||||||||||||
Read other articles:

Bleed It OutSingel oleh Linkin Parkdari album Minutes to MidnightDirilis20 Agustus 2007Direkam2007GenreRapcoreAlternative metalDurasi2:44LabelWarner Bros. RecordsPenciptaMike ShinodaChester BenningtonLinkin ParkProduserRick RubinMike Shinoda Bleed It Out adalah singel kedua Linkin Park dari album Minutes to Midnight. Lagu ini, selain Hands Held High, adalah lagu yang mengandung nyanyian rap Mike Shinoda. Lagu ini menempati urutan ke-4 di album Minutes to Midnight ini dan dirilis sebagai singe...

Musabaqah Tilawatil Quran (disingkat MTQ) adalah sebuah festival pemuliaan kitab suci umat Islam (Al-quran) yang diselenggarakan secara puncaknya pada tingkat nasional. Berikut ini adalah daftar Pembaca Pria Terbaik (Qari') sejak MTQ pertama yang dilaksanakan di Kota Makassar pada tahun 1968.[1] Tahun 1960-an 1968: K.Q. Ahmad Syahid - Jawa Barat 1969: Abdul Hakim Muslim - Jawa Tengah Tahun 1970-an Qori H. Muammar Z.A 1970: Salafuddin - Jawa Tengah 1971: Hasan Basri - Sumatera Utara 19...

Ethnic group of India For the language, see Shompen language. Shompen Keyet Kalay Mixed group of Shompen in 1886Total population229 (2011 census);[1] or 200-300 (2015 est.)[2]Regions with significant populations IndiaAndaman and Nicobar IslandsLanguagesShompenRelated ethnic groupsNicobarese people Austroasiatic people The Shompen or Shom Pen are the indigenous people of the interior of Great Nicobar Island, part of the Indian union territory of Andaman and Nicobar Islands...

Вільяр-дель-ІнфантадоVillar del InfantadoМуніципалітетКраїна ІспаніяАвтономна спільнота Кастилія-Ла-МанчаПровінція КуенкаКоординати 40°27′22″ пн. ш. 2°28′44″ зх. д. / 40.456° пн. ш. 2.479° зх. д. / 40.456; -2.479Координати: 40°27′22″ пн. ш. 2°28′44″ зх. �...

43°44′05″N 7°25′17″E / 43.73465°N 7.42133333°E / 43.73465; 7.42133333 جائزة موناكو الكبرى 1956 (بالفرنسية: XIV Grand Prix Automobile de Monaco) السباق 2 من أصل 8 في بطولة العالم لسباقات الفورمولا واحد موسم 1956 السلسلة بطولة العالم لسباقات فورمولا 1 موسم 1956 البلد موناكو التاريخ 13 مايو 1956 مكان ا

Elena Caffarena Morice Elena Caffarena 1938 Elena Caffarena (* 23. März 1903 in Iquique, Chile; † 19. Juli 2003 in Santiago de Chile, Chile) war eine chilenische Anwältin und Frauenrechtlerin. Sie war Gründerin der Bewegung für die Emanzipation chilenischer Frauen (Memch), der chilenischen Föderation der Fraueninstitutionen (Fechif) und der Stiftung zum Schutz von Kindern. Inhaltsverzeichnis 1 Leben und Werk 2 FECHIF und MEMCh 3 Veröffentlichungen (Auswahl) 4 Literatur 5 Weblinks 6 Ei...

De loop van een 240mm houwitser. Getrokken loop met spiraalsgewijs verlopende trekken van een kanon 10cm brons.De loop van een vuurwapen (ook wel schietbuis genoemd) is een buis, waardoor, nadat na een gecontroleerde ontsteking een afvuurlading zeer snel ontbrandt (en bijna explodeert), een projectiel met hoge snelheid afgevuurd wordt. De buis is tegenwoordig in veruit de meeste gevallen gemaakt van staal. Constructie Moderne lopen van vuurwapens worden gekenmerkt door een speciale opbouw en ...

العلاقات المكسيكية الليسوتوية المكسيك ليسوتو المكسيك ليسوتو تعديل مصدري - تعديل العلاقات المكسيكية الليسوتوية هي العلاقات الثنائية التي تجمع بين المكسيك وليسوتو.[1][2][3][4][5] مقارنة بين البلدين هذه مقارنة عامة ومرجعية للدولتين: وجه الم�...

Large flightless seabird endemic to Antarctica This article is about the bird. For the band, see Emperor Penguin (music). Emperor penguin Adults with a chick on Snow Hill Island, Antarctic Peninsula Conservation status Near Threatened (IUCN 3.1)[1] Scientific classification Domain: Eukaryota Kingdom: Animalia Phylum: Chordata Class: Aves Order: Sphenisciformes Family: Spheniscidae Genus: Aptenodytes Species: A. forsteri Binomial name Aptenodytes forsteriGray, 1844 Emperor pe...

American television series For the 1980 film, see Stir Crazy (film). Stir CrazyGenreSitcomAdventureDeveloped byLarry TuckerLarry RosenWritten byMichael RussnowDirected byPeter H. Hunt Christian I. Nyby IIStarringJoe Guzaldo Larry Riley Marc Silver Jeannie WilsonOpening themeStir It UpCountry of originUSAOriginal languageEnglishNo. of seasons1No. of episodes9ProductionProducerDavid J. LattRunning time60 min.Production companiesLarry/Larry ProductionsColumbia Pictures TelevisionOriginal release...

Canonical structure within the Catholic Church Part of a series on theCanon law of theCatholic Church Ius vigens (current law) 1983 Code of Canon Law Omnium in mentem Magnum principium Code of Canons of the Eastern Churches Ad tuendam fidem Ex corde Ecclesiae Indulgentiarum Doctrina Praedicate evangelium Veritatis gaudium Custom Matrimonial nullity trial reforms of Pope Francis Documents of the Second Vatican Council Christus Dominus Lumen gentium Optatam totius Orientalium ecclesiarum Presby...

Список середньовічних соборів Англії: Назва Зовнішній вигляд Інтер'єр Дата Сайт Бристольський соборангл. Bristol Cathedral 1140-1888 http://www.bristol-cathedral.co.uk/ [Архівовано 25 грудня 2003 у Wayback Machine.] Вінчестерський соборангл. Winchester Cathedral 1079-1521 http://www.winchester-cathedral.org.uk/ [Архівовано 18 квітня 2021 у Wayback ...

IUCN (International union of conservation of Nature) Wildlife of India Biodiversity Flora Fauna Lists Amphibians Ants Birds Butterflies Fish Mammals Molluscs Moths Odonates Tiger beetles Reptiles Spiders Endangered animals Protected areas Biosphere reserves Wildlife sanctuaries Conservation areas Private protected areas Reserved andprotected forests Conservation andcommunity reserves Communal forests Lists National parks Ramsar Sites Conservation Projects Tiger Elephant Acts of Parliament Ind...

Television station in Metro Davao, PhilippinesDXMJ-TV (GMA TV-5 Davao)Metro DavaoPhilippinesCityDavao CityChannelsAnalog: 5 (VHF)Digital: 37 (UHF) (ISDB-T) (test broadcast)Virtual: 5.01BrandingGMA TV-5 DavaoProgrammingSubchannelsSee listAffiliations 5.1: GMA 5.2: GTV 5.3: Heart of Asia 5.6: Hallypop 5.7: I Heart Movies 5.8: Pinoy Hits OwnershipOwnerGMA Network Inc.Sister stations DXRA-TV (GTV) GMA Super Radyo DXGM 1125 Davao Barangay FM 103.5 Davao HistoryFoundedDecember 25, 1962Former call s...

American media executive, political commentator, and conspiracy theorist Solomon speaking at the Conservative Political Action Conference in Washington, D.C. John F. Solomon is an American journalist who was a contributor to Fox News until late 2020.[1][2][3][4] He was formerly an executive and editor-in-chief at The Washington Times.[5] Although he won a number of awards (including the 2008 Robert F. Kennedy Journalism Award) for his investigative jour...

Indian talk show Memu SaithamSeason 2 posterమేము సైతంGenreReality talk showPresented byLakshmi ManchuCountry of originIndiaOriginal languageTeluguNo. of seasons2No. of episodes66ProductionProducerLakshmi ManchuProduction locationHyderabadCamera setupMulti-cameraRunning timeapprox. 40-45 minutes per episodeProduction companyManchu TelefilmsOriginal releaseNetworkGemini TVRelease2 April 2016 (2016-04-02) –12 August 2018 (2018-08-12)RelatedSun Naam Oruvar Sada Ni...

هذه مقالة غير مراجعة. ينبغي أن يزال هذا القالب بعد أن يراجعها محرر مغاير للذي أنشأها؛ إذا لزم الأمر فيجب أن توسم المقالة بقوالب الصيانة المناسبة. يمكن أيضاً تقديم طلب لمراجعة المقالة في الصفحة المخصصة لذلك. (يوليو 2021) ستار لاند فوكال باند النوع بوب شركة الإنتاج تسجيل�...
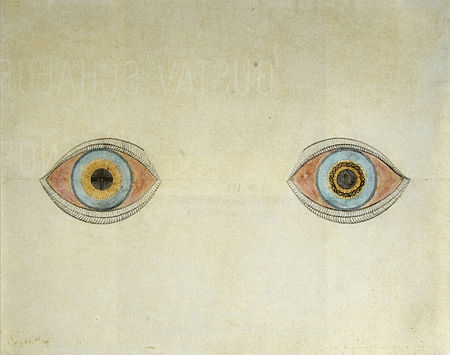
الفصامقماش مطرز أنجزه أحد الأشخاص المصابين بالفُصاممعلومات عامةالاختصاص طب نفسي — علم النفس السريري من أنواع اضطراب نفسياضطراب مجال الفُصاممرض الأسبابالارتباط الجيني القائمة ... Cav1.2[1]TSNARE1 (en) [2]SLCO6A1 (en) [3]ZEB2 (en) [4]SDCCAG8[5]NOTCH4 (en) [6]BRD1 (en) [7]FBXO11[8&...

Otto Porter Otto Porter con los Wizards en 2019.Datos personalesNombre completo Otto Porter, Jr.Apodo(s) BubbaNacimiento San Luis, Misuri Estados Unidos6 de marzo de 1993 (31 años)Nacionalidad(es) EstadounidenseAltura 2,03 m (6′ 8″)Peso 90 kg (198 lb)Carrera deportivaDeporte BaloncestoEquipo universitario Georgetown (2011–2013)Club profesionalDraft de la NBA 1.ª ronda (puesto 3), 2013 por Washington Wizards[1]Club RetiradoLiga NBAPosición AleroTrayect...

Location of New Kent County in Virginia This is a list of the National Register of Historic Places listings in New Kent County, Virginia. This is intended to be a complete list of the properties and districts on the National Register of Historic Places in New Kent County, Virginia, United States. The locations of National Register properties and districts for which the latitude and longitude coordinates are included below, may be seen in an online map.[1] There are 18 properties and ...





