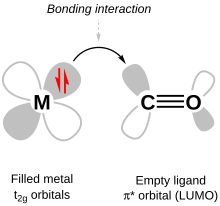
Ligand field theory
|
Read other articles:

Archaeological period This article is about the historical/archaeological period known as the Iron Age. For the mythological Iron Age, see Ages of Man. Part of a series on theIron Age ↑ Bronze Age By region Ancient Near East (1200–550 BC) Bronze Age collapse (1200–1150 BC) Anatolia, Caucasus, Levant Europe Aegean (1200–700 BC) Italy (1100–700 BC) Balkans (1100 BC – 150 AD) Eastern Europe (900 – 650 BC) Central Europe (800 – 50 BC) Great Britain (800 BC – 100 AD) Northern Eur...

Nama ini menggunakan cara penamaan Spanyol: nama keluarga pertama atau paternalnya adalah Ortega dan nama keluarga kedua atau maternalnya adalah Gaona. Amancio OrtegaBerkas:Amancio Ortega 2015 Photograph.jpgAmancio Ortega, Oktober 2013LahirAmancio Ortega Gaona28 Maret 1936 (umur 88)Busdongo de Arbás, León, SpanyolTempat tinggalA Coruña, SpanyolKebangsaanSpanyolPekerjaanPebisnisDikenal atasPendiri Inditex GroupKekayaan bersih US$79,5 miliar (7 September 2016)[1]Anggot...

Emily Donelson Ibu Negara Amerika Serikat ke-7Masa jabatan4 Maret 1829 – 19 Desember 1836 PendahuluLouisa AdamsPenggantiSarah Yorke Jackson Informasi pribadiLahir(1807-06-01)1 Juni 1807Donelson, TennesseeMeninggal19 Desember 1836(1836-12-19) (umur 29)Nashville, TennesseeSuami/istriAndrew Jackson A.J. DonelsonHubunganJohn Donelson (father)Rachel Donelson Jackson (aunt)PekerjaanIbu Negara Amerika SerikatSunting kotak info • L • B Emily Tennessee Donelson (1 Juni 18...

List of events ← 2015 2014 2013 2016 in Japan → 2017 2018 2019 Decades: 1990s 2000s 2010s 2020s See also:Other events of 2016History of Japan • Timeline • Years The following lists events that happened during 2016 in Japan. Year: Heisei 28 Incumbents Emperor: Akihito[1] Prime Minister: Shinzō Abe (L–Yamaguchi) Chief Cabinet Secretary: Yoshihide Suga (L–Kanagawa) Chief Justice of the Supreme Court: Itsurō Terada President of the House...

Street in St. Petersburg, Russia 59°55′44″N 30°18′30″E / 59.9288°N 30.3082°E / 59.9288; 30.3082 View to the beginning of the avenue from No. 11 Voznesensky Prospekt (Russian: Вознесенский проспект) is a 1.8 km long street in Admiralteysky District of Saint Petersburg, Russia. Crossing Saint Isaac's Square, the Moika (Blue Bridge) and Griboyedov Canal (Voznesensky Bridge), the street spans from Admiralteysky Prospekt to Izmaylovsky B...

この項目には、一部のコンピュータや閲覧ソフトで表示できない文字が含まれています(詳細)。 数字の大字(だいじ)は、漢数字の一種。通常用いる単純な字形の漢数字(小字)の代わりに同じ音の別の漢字を用いるものである。 概要 壱万円日本銀行券(「壱」が大字) 弐千円日本銀行券(「弐」が大字) 漢数字には「一」「二」「三」と続く小字と、「壱」「�...

Cycling race 2018 Gent–Wevelgem (women's race)2018 UCI Women's World Tour, race 5 of 23Race detailsDates25 March 2018Stages1Distance142.6 km (88.61 mi)Winning time3h 41' 00Results Winner Marta Bastianelli (ITA) (Alé–Cipollini) Second Jolien D'Hoore (BEL) (Mitchelton–Scott) Third Lisa Klein (GER) (Canyon–SRAM)← 2017 2019 → The seventh running of Gent–Wevelgem's women's race (also known as Gent-Wevelge...

Map of Zimbabwe This is a list of cities, towns and villages in Zimbabwe. See also: Place names in Zimbabwe. Cities This section does not cite any sources. Please help improve this section by adding citations to reliable sources. Unsourced material may be challenged and removed. (September 2017) (Learn how and when to remove this message) Harare Bulawayo Mutare Kwekwe Kadoma Cities in Zimbabwe[1] City Province Census 1982 Census 1992 Census 2002 Census 2012 Census 2022 Harare Harare ...

Mongolie, pays-zone exempte d'arme nucléaire En 1992, le président de la Mongolie Punsalmaagiyn Ochirbat a annoncé que son pays envisageait de devenir un « pays-zone exempte d'arme nucléaire ». Les changements géopolitiques engendrés par la chute de l'Union soviétique et, en particulier pour le pays, le retrait des troupes russes de son territoire, ont donné à Oulan-Bator l'opportunité de proclamer sa neutralité politique. Bien que peu orthodoxe, l'initiative mongole de...

King of Ephyra in Greek mythology For other uses, see Sisyphus (disambiguation). Not to be confused with Syphilis. Persephone supervising Sisyphus in the Underworld, Attic black-figure amphora, c. 530 BC, Staatliche Antikensammlungen[1] Sisyphus and Amphiaraus, copy of mural in François Tomb from Vulci made in 4th century BC In Greek mythology, Sisyphus or Sisyphos (/ˈsɪsɪfəs/; Ancient Greek: Σίσυφος Sísyphos) was the founder and king of Ephyra (now known as Corinth...

Ayub 33Kitab Ayub lengkap pada Kodeks Leningrad, dibuat tahun 1008.KitabKitab AyubKategoriKetuvimBagian Alkitab KristenPerjanjian LamaUrutan dalamKitab Kristen18← pasal 32 pasal 34 → Ayub 33 (disingkat Ayb 33) adalah bagian dari Kitab Ayub di Alkitab Ibrani dan Perjanjian Lama dalam Alkitab Kristen. Kitab ini menceritakan riwayat Ayub, seorang yang saleh, dan pencobaan yang dialaminya.[1][2] Teks Naskah sumber utama: Masoretik, Septuaginta dan Naskah Laut Mati. Pas...

Disambiguazione – Se stai cercando altri significati, vedi Unione europea (disambigua). Disambiguazione – UE rimanda qui. Se stai cercando altri significati, vedi Ue (disambigua). Unione europeavedi i nomi nelle lingue ufficiali Bandiera dell'Europa AbbreviazioneUE, Ue TipoUnione sovranazionale[1][2] Fondazione CEE: trattato di Roma, 25 marzo 1957 UE: trattato di Maastricht, 7 febbraio 1992 Scopoesercizio di parte della sovranità nazionale degli Stati membri...

Untuk daftar Direktur Jenderal Belanda yang berkuasa di New Amsterdam sebagai bagian dari New Netherland antara 1625 dan 1664, lihat: Direktur Jenderal New Netherland. Wali kota New York City adalah kepala eksekutif pemerintah New York City, sebagaimana ditetapkan oleh Charter City of New York. Daftar ini hingga tahun 1897 mencakup wali kota dari (lebih kurang) wilayah yang kini dianggap sebagai Manhattan dan bagian-bagian dari The Bronx saja. Kelima borough ini dikonsolidasikan pada 1898. Li...

「加東康一」、「加藤公一」、「加藤幸一」、あるいは「加藤浩一」とは別人です。 日本の政治家加藤 紘一かとう こういち 1985年、首相官邸にて生年月日 (1939-06-17) 1939年6月17日出生地 日本、愛知県名古屋市[1]没年月日 (2016-09-09) 2016年9月9日(77歳没)死没地 日本、東京都出身校 東京大学法学部第3類東京大学法学部第2類国立台湾大学大学院ハーバード大学大学院...

Pour les articles homonymes, voir Gautier de Brienne. Gautier IV de BrienneFonctionsComte de BrienneComte de Jaffa et d'AscalonTitres de noblesseComte de Brienne1205-1246Prédécesseur Gautier III de BrienneSuccesseur Jean de BrienneComte de Jaffa1221-1246Successeur Jean d'IbelinBiographieNaissance 1205Décès 1244 ou 1246Le CaireActivité FeudataireFamille Maison de BriennePère Gautier III de BrienneMère Elvire de SicileFratrie Marguerite de Reynel (d)Conjoint Marie de Lusi...

Head of the district council of Masterton, New Zealand Mayor of MastertonIncumbentGary Caffellsince 9 October 2022StyleHis/Her WorshipTerm lengthThree yearsInaugural holderR.G WilliamsFormation1877 The Mayor of Masterton is the head of the municipal government of Masterton, New Zealand, and presides over the Masterton District Council. The Mayor is directly elected using First Past the Post.The Mayor is assisted by the Deputy Mayor of Masterton. The current Mayor is Gary Caffell, elected...
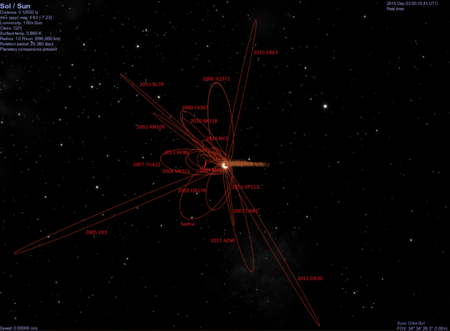
(308933) 2006 SQ372Астероид Открытие Первооткрыватель SDSS Место обнаружения Обсерватория Апачи-Пойнт[1] Дата обнаружения 27 сентября 2006 Альтернативные обозначения 2006 SQ372 Категория объект рассеянного диска Орбитальные характеристики Эпоха 27 августа 2011 Эксцентриситет (e) 0,9749394...

Championnat sud-américain de 1956 Généralités Sport Football Organisateur(s) CONMEBOL Édition 24e Lieu(x) Uruguay Date du 21 janvier 1956au 15 février 1956 Participants 6 Matchs joués 15 Affluence 568 000 spectateurs Site(s) Stade Centenario(Montevideo) Palmarès Tenant du titre Argentine Vainqueur Uruguay Deuxième Chili Troisième Argentine Buts 38 (2,5 par match) Meilleur(s) buteur(s) Hormazábal (4) Navigation Chili 1955 Pérou 1957 modifier Le Championnat sud-américain de f...

British politician (born 1951) The Right Honourable SirJohn RedwoodOfficial portrait, 2020Shadow Secretary of State for DeregulationIn office6 May 2005 – 5 December 2005LeaderMichael HowardPreceded byPosition establishedSucceeded byPosition abolishedShadow Secretary of State for Environment, Transport and the RegionsIn office15 June 1999 – 2 February 2000LeaderWilliam HaguePreceded byGillian ShephardSucceeded byArchie NormanShadow Secretary of State for Trade and Industr...

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (أغسطس 2024) علم سكون الموائعصنف فرعي من ميكانيكا الموائع جزء من hydromechanics (en) — سكونيات تعديل - تعديل مصدري - تعديل ويكي ب...