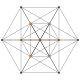
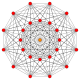
2 22 honeycomb
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Read other articles:

Artikel ini membahas mengenai bangunan, struktur, infrastruktur, atau kawasan terencana yang sedang dibangun atau akan segera selesai. Informasi di halaman ini bisa berubah setiap saat (tidak jarang perubahan yang besar) seiring dengan penyelesaiannya. Rolex TowerInformasi umumLokasiDubai, Uni Emirat ArabPerkiraan rampung2009Data teknisJumlah lantai63Desain dan konstruksiArsitekSkidmore, Owings & Merrill (London) LLP Rolex Tower merupakan sebuah menara bertingkat 63 di Dubai, Uni Emirat A...

Voce principale: Associazione Calcio Savoia 1908. Football Club Savoia 1908Stagione 2006-2007Sport calcio Squadra Savoia Allenatore Paolo Anastasio (1ª-27ª) poi Massimo Agovino (28ª-34ª) Presidente Alessandro Farinelli Serie D5º posto Coppa Italia Serie DPrimo turno Maggiori presenzeCampionato: Romagnini (35)Totale: Romagnini (35) Miglior marcatoreCampionato: Pinto (10)Totale: Pinto (10) StadioAlfredo Giraud 2005-2006 2007-2008 Si invita a seguire il modello di voce Questa voce rac...

9Poster teaterIndeks kartuSutradaraShane AckerProtagonisElijah WoodJohn C. ReillyJennifer ConnellyChristopher PlummerCrispin GloverMartin LandauFred TatascioreProduksi seni pertunjukanTim BurtonTimur BekmambetovJim LemleyDana GinsburgNaskahShane Acker MusikDanny ElfmanDeborah LurieFotografiKevin R. Adams Penyuntingan filmNick Kenway Rumah produksiFocus Features, Relativity Media, Jam Filled Entertainment, Bazelevs Company dan Tim Burton Productions DistributorFocus FeaturesUniversal Pictures...

ロバート・デ・ニーロRobert De Niro 2011年のデ・ニーロ生年月日 (1943-08-17) 1943年8月17日(80歳)出生地 アメリカ合衆国・ニューヨーク州ニューヨーク市身長 177 cm職業 俳優、映画監督、映画プロデューサージャンル 映画、テレビドラマ活動期間 1963年 -配偶者 ダイアン・アボット(1976年 - 1988年)グレイス・ハイタワー(1997年 - )主な作品 『ミーン・ストリート』(1973年)...

نادي شافهاوزن تأسس عام 1896 البلد سويسرا الموقع الرسمي الموقع الرسمي تعديل مصدري - تعديل نادي شافهاوزن لكرة القدم (بالألمانية: FC Schaffhausen AG) نادي كرة قدم سويسري يلعب في دوري التحدي السويسري.[1][2][3] تم تأسيس النادي في عام 1896. مراجع ^ معلومات عن نادي شافهاو...

1787 painting by Jacques-Louis David This article is about the painting. For the event depicted, see Trial of Socrates. The Death of SocratesArtistJacques-Louis DavidYear1787 (1787)MediumOil on canvasMovementNeoclassicismDimensions129.5 cm × 196.2 cm (51.0 in × 77.2 in)LocationMetropolitan Museum of Art, New York The Death of Socrates (French: La Mort de Socrate) is an oil on canvas painted by French painter Jacques-Louis David in 1787. The pai...

Questa voce sull'argomento hockeisti su ghiaccio cechi è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Adam Svoboda Nazionalità Rep. Ceca Altezza 177 cm Peso 80 kg Hockey su ghiaccio Ruolo Portiere Palmarès Per maggiori dettagli vedi qui Modifica dati su Wikidata · Manuale Adam Svoboda (Brno, 26 giugno 1978 – Pardubice, 7 maggio 2019) è stato un hockeista su ghiaccio ceco. È stato trovato impiccato in casa sua nel maggio 2019 ...

Aoba 青葉区Distrik kotaKantor Distrik AobaLokasi Distrik Aoba di wilayah SendaiNegaraJepangWilayahTōhokuPrefektur MiyagiKotaSendaiDidirikan1 April 1989Luas[1] • Total302,24 km2 (116,70 sq mi)Populasi (1 Desember 2023) • Total314,947 • Kepadatan1.042,04/km2 (2,698,9/sq mi)Zona waktuUTC+09:00 (JST)Kode pos980-8701Alamat kantor distrik1-5-1 Kamisugi, Aoba-ku, Sendai-shi, Miyagi-kenNomor telefon022-225-7211Situs webwww....

Fijian American professional wrestler (1943–2017) For his son Jimmy Snuka Jr., see Deuce (wrestler). Jimmy SnukaSnuka in 2011Birth nameJames Wiley SmithBorn(1943-05-18)May 18, 1943Suva, Fiji[a]DiedJanuary 15, 2017(2017-01-15) (aged 73)Coral Springs, Florida, U.S.Spouse(s) Sharon Snuka (m. 1964; div. 1999) Carole Snuka (m. 2004)ChildrenDeuce, Liana Snuka and TaminaFamilyAnoaʻi[1]Professio...

Component parts of the UK since 1922 Countries of the United Kingdom England NorthernIreland Scotland Wales CategoryAdministrative divisionLocation United KingdomFound inLegal jurisdictionsNumber4Possible statusITL 1 region (3)Legal jurisdiction (3)Additional statusHome NationsGovernmentCentral government (1)Devolved government (3) Since 1922, the United Kingdom has been made up of four countries: England, Scotland, Wales (which collectively make up Great Britain) and Nort...

Mineral group, calcium phosphate Not to be confused with appetite. Apatite groupApatite (CaF) (fluorapatite) doubly-terminated crystal in calciteGeneralCategoryPhosphate mineralFormula(repeating unit)Ca5(PO4)3(F,Cl,OH)IMA symbolAp[1]Strunz classification8.BN.05Crystal systemHexagonalCrystal classDipyramidal (6/m) (same H-M symbol)[2]Space groupP63/m (no. 176)IdentificationColorTransparent to translucent, usually green, less often colorless, yellow, blue to violet, pink, brown....

Truncated order-4 octagonal tiling Poincaré disk model of the hyperbolic plane Type Hyperbolic uniform tiling Vertex configuration 4.16.16 Schläfli symbol t{8,4}tr{8,8} or t { 8 8 } {\displaystyle t{\begin{Bmatrix}8\\8\end{Bmatrix}}} Wythoff symbol 2 8 | 8 2 8 8 | Coxeter diagram or Symmetry group [8,4], (*842)[8,8], (*882) Dual Order-8 tetrakis square tiling Properties Vertex-transitive In geometry, the truncated order-4 octagonal tiling is a uniform tiling of the hyperbolic pla...

Una coppia omosessuale nel 2008 al Gay Pride DICO è una sigla che significa DIritti e doveri delle persone stabilmente COnviventi e viene riferita comunemente al disegno di legge, presentato dal Governo Prodi II l'8 febbraio 2007, finalizzato al riconoscimento nell'ordinamento giuridico italiano di taluni diritti e doveri discendenti dai rapporti di convivenza registrati all'anagrafe: l'iter legislativo si è di fatto concluso con la caduta del Governo Prodi II nel 2008. Indice 1 L'inquadram...
Bahraini activist Esra'a Al ShafeiBorn (1986-07-23) 23 July 1986 (age 37)NationalityBahrainiOrganizationMajal (Mideast Youth)Websitemajal.org Esra'a Al Shafei (Arabic: إسراء الشافعي ’Asrā’ ash-Shāfa’ī; born 23 July 1986)[1] is a Bahraini civil rights activist, blogger, and the founder and executive director of Majal (Mideast Youth) and its related projects, including CrowdVoice.org.[2] Al Shafei is a senior TED Fellow,[3] an Echoing Green fell...

De baltiska folkstammarnas utbredning kring år 1200, då korsfararna började bli en kraft i Baltikum. Kartan ger sannolikt också en grov bild av utbredning av språk och dialekter vid denna tidpunkt, från nordost till sydväst: Lettgalliska, Seloniska, Semgalliska, Kuriska, Litauiska, Sudoviska, Prusiska Baltiska språk Baltiska språk är en grupp indoeuropeiska språk, talade öster om Östersjön. Lettiska, litauiska och fornpreussiska tillhör denna grupp av språk. Numera finns bara ...

This article relies excessively on references to primary sources. Please improve this article by adding secondary or tertiary sources. Find sources: McLaren Health Care Corporation – news · newspapers · books · scholar · JSTOR (September 2023) (Learn how and when to remove this message) McLaren Health Care CorporationCompany typeNonprofitIndustryHealthcareFounded1914 HeadquartersGrand Blanc, Michigan, USAArea servedFlint/Tri-Cities,Central Michiga...

US Bank New York Community Bancorp, Inc.New York Community Bank headquarters,Hicksville, New York, U.S.FormerlyQueens County Savings Bank (1859–2000)Company typePublic companyTraded asNYSE: NYCBS&P 400 componentIndustryCommercial bankFoundedApril 14, 1859; 165 years ago (1859-04-14)HeadquartersHicksville, New York, U.S.Number of locations420 branchesKey people Joseph Otting (CEO),Sandro DiNello (Non-Executive Chairman of the Board)ProductsMulti-family loansCommerc...

Maria Wetterstrand Anggota Parlemen Swediauntuk Wilayah StockholmMasa jabatan2001–2011Pembicara Partai HijauMasa jabatan12 Mei 2002 – 21 Mei 2011Menjabat bersama Peter ErikssonPendahuluLotta HedströmMatz HammarströmPenggantiÅsa RomsonGustav Fridolin Informasi pribadiLahir2 Oktober 1973 (umur 50)Eskilstuna, SwediaPartai politikPartai HijauSuami/istriVille Niinistö(2004-2012; bercerai)AnakElias (l. 2004)Linnea (l. 2007)Alma materUniversitas GothenburgSunting kotak ...

См. также: Красный террор Эта статья о крымском терроре в 1917—1918 годах. О красном терроре в Крыму в 1920—1921 годах смотрите статью Красный террор в Крыму Тела казнённых во время «варфоломеевских ночей» в Евпатории, выброшенные на берег летом 1918 года Ма́ссовый терро́р в Крыму...

District in Sabah, MalaysiaSandakan District Daerah SandakanDistrictSandakan Municipal Council office. SealCoordinates: 5°50′20″N 118°6′55″E / 5.83889°N 118.11528°E / 5.83889; 118.11528Country MalaysiaState SabahDivisionSandakanCapitalSandakanGovernment • President (Sandakan Municipal Council)Benedict AsmatArea • Total2,266 km2 (875 sq mi)Population (2010) • Total396,290Websitemps.sabah.gov....