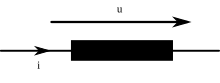
محاثة تبادلية
|
Read other articles:

Jagoan SilatGenre Drama Roman Fantasi Laga PembuatMD EntertainmentTerinspirasi olehKung Fu Pandaoleh Mark Osborne & John StevensonDitulis olehDono IndartoSkenarioDono IndartoSutradaraAi ManafPemeran Tommy Kaganangan Thoriq Hafidz Nana Mirdad Niken Anjani Lian Firman Bemby Putuanda Barry Prima George Rudy Ria Irawan Adi Pawitra Marsha Aruan Bintang Elian Nona Laboni Daniel Shivael Abthal Adams Timothy Fidealdo Argo Jimmy Oma Kucrut Amanda Rawles Bella Shania Samantha Dressler Ricky Martin...

Italian cyclist (born 1969) This article is about the Italian cyclist. For German soccer player, see Marco Villa (footballer). Marco VillaVilla in 2007Personal informationBorn (1969-02-08) February 8, 1969 (age 55)Abbiategrasso, ItalyTeam informationCurrent teamRetiredDisciplineTrackRoadRoleRiderProfessional teams1994–1995Amore & Vita–Galatron1996–1997Brescialat1998–1999Cantina Tollo–Alexia Alluminio2000–2002Alexia Alluminio2003–2004Formaggi Pinzolo Fiavè Medal re...

Pour les articles homonymes, voir Duparc. Pour les autres membres de la famille, voir Fouques Duparc. Cet article est une ébauche concernant un homme politique français. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Henri Fouques Duparc Fonctions Député français 8 décembre 1958 – 3 juillet 1962(3 ans, 6 mois et 25 jours) Élection 30 novembre 1958 Circonscription Oran Législature IIe (Q...

UFC MMA events in 2021 2021 in UFCInformationFirst dateJanuary 16, 2021 (2021-01-16)Last dateDecember 18, 2021 (2021-12-18)EventsTotal events43UFC13TUF Finale events1FightsTotal fights509Title fights19Chronology 2020 in UFC 2021 in UFC 2022 in UFC The year 2021 was the 28th year in the history of the Ultimate Fighting Championship (UFC), a mixed martial arts promotion based in the United States. Releases and retirements These fighters have either been released f...

This article needs to be updated. The reason given is: Hardware failure following a January 2021 spacewalk,[1] and updates on planned 2020 changes. Please help update this article to reflect recent events or newly available information. (January 2021) A student speaks to crew on the International Space Station using Amateur Radio equipment, provided free by volunteers of the ARISS program.. Astronaut Doug Wheelock operating ham radio from the ISS Amateur Radio on the International Sp...

Anti-Vivisection CoalitionTypePressure groupFocusOpposition to animal testingLocationLondon, United KingdomArea served UK wideKey peopleDanny Flies (Chairman) Sophie Kennerley (UK Coordinator) Dr Andre Menache (Scientific Consultant)Websitewww.stopvivisection.org.uk The Anti-Vivisection Coalition (AVC) is a United Kingdom-based pressure group which campaigns against animal testing. The AVC are described as 'main driver' of the Stop Vivisection Initiative, a petition launched in November 2012...

艾哈迈德·塞古·杜尔总统杜尔、代表几内亚共和国在美国马里兰访问华盛顿特区期间抵达安德鲁斯空军基地。 (1982年6月) 第一任几内亚总统任期1958年10月2日—1984年3月26日前任无,职务设立继任路易斯·兰萨纳·贝阿沃吉 个人资料出生(1922-01-09)1922年1月9日 法兰西第三共和国法属西非法拉纳逝世1984年3月26日(1984歲—03—26)(62歲) 美國克利夫兰, 俄亥俄州墓地科奈克里大清�...

Buk District 북구DistrikTranskripsi Korea • Hanja北區 • Alih Aksara yang DisempurnakanBuk-gu • McCune-ReischauerPuk-kuNegaraKorea SelatanWilayahYeongnamTingkat provinsiUlsanPembagian administratif27 administratif dongLuas • Total80,41 km2 (31,05 sq mi)Populasi (2006) • Total149.676 • Kepadatan1,900/km2 (4,800/sq mi) • DialekGyeongsangSitus webBuk District Office Distrik Buk (B...

районБокейординский районБөкей Орда ауданы 48°48′45″ с. ш. 46°45′49″ в. д.HGЯO Страна Казахстан Входит в Западно-Казахстанскую область Адм. центр село Сайхин Аким Даумов Нурлыбек Жаскайратович[1] История и география Площадь 19214,45[2] км² (3-е место) Часо...

National indifference is the status of lacking a strong and consistent national identity. The concept was originated by scholars of the Bohemian lands, where many inhabitants historically resisted classification as either Czechs or Germans, around 2000.[1] It was outlined by Tara Zahra in her 2010 paper published in Slavic Review, Imagined Noncommunities: National Indifference as a Category of Analysis.[2][3][4][5][6][7][8] In 20...

Art school in Manhattan, New York Art Students' League redirects here. For the building occupied by the organization, see American Fine Arts Society. For the school started by Thomas Eakins, see Art Students' League of Philadelphia. The American Fine Arts Society Building at 215 West 57th Street The Art Students League of New York is an art school in the American Fine Arts Society in Manhattan, New York City. The Arts Students League is known for its broad appeal to both amateurs and professi...

System of ditches and embankments The three sections of the Willow Palisade on an 1883 map Willow Palisade (Chinese: 柳條邊; pinyin: Liǔtiáo Biān; Manchu: ᠪᡳᡵᡝᡤᡝᠨ ᠵᠠᠰᡝ, Möllendorff: Biregen Jase) was a system of ditches and embankments that was planted with willows, was intended to restrict movement into Manchuria (including Northeast China and Outer Manchuria), and was built by the Qing dynasty of China during the late 17th century.[1] I...

City in Oregon, United StatesBarlow, OregonCityChurch in BarlowLocation in OregonCoordinates: 45°15′08″N 122°43′21″W / 45.25222°N 122.72250°W / 45.25222; -122.72250CountryUnited StatesStateOregonCountyClackamasIncorporated1903Government • MayorMichael Lundsten[1]Area[2] • Total0.05 sq mi (0.14 km2) • Land0.05 sq mi (0.14 km2) • Water0.00 sq mi (0.00 ...
Questa voce sull'argomento centri abitati del Guerrero è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Igualapacomune LocalizzazioneStato Messico Stato federato Guerrero TerritorioCoordinate16°45′19″N 98°29′01″W16°45′19″N, 98°29′01″W (Igualapa) Altitudine599 m s.l.m. Superficie266,70 km² Abitanti11 383[1] (2015) Densità42,68 ab./km² Altre informazioniFuso orarioUTC-6 CartografiaIgualapa Igu...

Íslandsmót - Sjóvá-Almennra1995 Généralités Sport Football Édition 84e Date du 23 mai 1995 au 23 septembre 1995 Participants 10 Palmarès Tenant du titre ÍA Akranes Promu(s) UMF GrindavíkLeiftur Olafsfjordur Navigation Saison précédente Saison suivante modifier La saison 1995 du Championnat d'Islande de football était la 84e édition de la première division islandaise. Les 10 clubs jouent les uns contre les autres lors de rencontres disputées en matchs aller et retour. L'...

Group whose operation is composition of permutations Algebraic structure → Group theoryGroup theory Basic notions Subgroup Normal subgroup Group action Quotient group (Semi-)direct product Direct sum Free product Wreath product Group homomorphisms kernel image simple finite infinite continuous multiplicative additive cyclic abelian dihedral nilpotent solvable Glossary of group theory List of group theory topics Finite groups Cyclic group Zn Symmetric group Sn Alternating group An Dihedral g...

1970 novel by Joan Didion For the Patti Scialfa album, see Play It as It Lays (album). Play It as It Lays First edition coverAuthorJoan DidionLanguageEnglishPublisherFarrar, Straus and GirouxPublication date1970Publication placeUnited StatesMedia typePrint (hardcover and paperback)Pages214ISBN0-374-52171-9OCLC312968389 Play It as It Lays is a 1970 novel by American writer Joan Didion. Time magazine included the novel in its list of the 100 Best English-Language Novels from 1923 to 2005.&...

Australian novelist Alice Grant Rosman, c.1935 Alice Grant Rosman (18 July 1882 — 20 August 1961) (born Alice Trevenen Rosman) was an Australian novelist.[1] Writing career Alice Rosman was born in Kapunda, South Australia. She had one sister called Mary and the two girls attended St Mary's Convent, Franklin Street, Adelaide until 1889.[1] In 1901, she started the Girl's Realm Guide in Adelaide. The Girls' Realm Guild published one of Rosman's books, The Young Queen.[2&#...

Reggina CalcioStagione 1992-1993Sport calcio Squadra Reggina Allenatore Gabriele Geretto (1ª-13ª) Enzo Ferrari (14ª-34ª) Presidente Pasquale Foti Serie C19º posto 1991-1992 1993-1994 Si invita a seguire il modello di voce Questa voce raccoglie le informazioni riguardanti la Reggina nelle competizioni ufficiali della stagione 1992-1993. Indice 1 Stagione 2 Divise e sponsor 3 Rosa 4 Risultati 4.1 Campionato 4.1.1 Girone di andata 4.1.2 Girone di ritorno 4.2 Coppa Italia Serie C 5 Stat...

Overview of marriage law worldwide Marital law redirects here. Not to be confused with Martial law. For the social and interpersonal aspects of marriage, see Marriage. This article needs to be updated. Please help update this article to reflect recent events or newly available information. (December 2023) Marriage law by country State recognizes civil marriages only State recognizes both civil and certain religious marriages State recognizes civil marriages...