.hack系列
| |||||||||||||||||
Read other articles:

This article is about the NHL recognition award. For the NHL All-Stars who play in the All-Star Game, see National Hockey League All-Star Game. Annual National Hockey League honor NHL All-Star TeamSportIce hockeyLeagueNational Hockey LeagueAwarded forBest players at each position as voted by the Professional Hockey Writers' AssociationHistoryFirst award1930–31 The National Hockey League All-Star teams were first named at the end of the 1930–31 NHL season, to honor the best performers over...

Cet article est une ébauche concernant l’Inde. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Un guerrier rajput. Les Rajputs (« fils de prince » de raja, prince, et putra, fils) forment la majorité des habitants du Rajasthan, correspondant approximativement à l'ancien Rajputana, et une partie de celle du Gujarat et du Penjab. Une partie du Sind, dans l'actuel Pakistan a également été contr�...

Defunct railroad branch in Massachusetts Whittenton BranchWhittenton Branch abutments at King Phillip StreetOverviewStatusAbandonedLocaleBristol County, MassachusettsTerminiWhittenton JunctionRaynhamFormer connectionsMiddleboro Secondary, Dighton and Somerset RailroadStations3ServiceOperator(s)Old Colony Railroad, New York, New Haven and Hartford RailroadHistoryOpened1882 (full length)Closed1966TechnicalLine length4.8 km (3.0 mi)Track gauge4 ft 8+1⁄2 in (1,435&#...

Phoenix Sky Harbor International Airport Bandara Internasional Phoenix Sky HarborIATA: PHXICAO: KPHXFAA LID: PHXInformasiJenisPublikPemilikKota PhoenixPengelolaPhoenix Airport SystemMelayaniPhoenix, ArizonaLokasiPhoenix, ArizonaMaskapai penghubung Ameriflight Great Lakes Airlines US Airways Ketinggian dpl346 mdplSitus webwww.skyharbor.comPetaFAA airport diagramPHXLokasi di ArizonaLandasan pacu Arah Panjang Permukaan kaki m 8/26 11,489 3,502C Beton 7L/25R 10,300 3,139 Beton 7R/25L 7,...

Overview of the languages spoken in Honduras This article does not cite any sources. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Languages of Honduras – news · newspapers · books · scholar · JSTOR (September 2023) (Learn how and when to remove this message) Languages of HondurasOfficialSpanishIndigenousAmerindian languagesMinorityGarifunaForeignEnglish There ar...

Japanese light novel series The Demon Sword Master of Excalibur AcademyFirst light novel volume cover聖剣学院の魔剣使い(Seiken Gakuin no Makentsukai) Light novelWritten byYū ShimizuIllustrated byAsagi TōsakaPublished byMedia FactoryEnglish publisherNA: Yen PressImprintMF Bunko JDemographicMaleOriginal runMay 25, 2019 – presentVolumes15 MangaWritten byYū ShimizuIllustrated byAsuka KeigenPublished byKadokawa ShotenEnglish publisherNA: Yen PressMagazin...

Senegalese professional footballer Dominique Badji Badji with FC Cincinnati in 2023Personal informationFull name Dominique Joel Badji[1]Date of birth (1992-10-16) 16 October 1992 (age 31)Place of birth Dakar, SenegalHeight 6 ft 0 in (1.83 m)[2]Position(s) ForwardTeam informationCurrent team BandırmasporNumber 14College careerYears Team Apps (Gls)2011–2014 Boston University Terriers 69 (26)Senior career*Years Team Apps (Gls)2014 Real Boston Rams 6 (4)2015...

Конкуренция уголовно-правовых норм имеет место в случаях, когда одно и то же деяние регулируется двумя нормами уголовного законодательства, из которых подлежит применению только одна. Содержание 1 Законодательные положения, касающиеся конкуренции уголовно-правовых но�...

43°22′33.24″N 80°17′8.57″W / 43.3759000°N 80.2857139°W / 43.3759000; -80.2857139 (Grand River Conservation Authority Head Office) Grand River Conservation AuthorityFounded1932FocusManage the water and other natural resources in the Grand River WatershedArea served Grand River WatershedWebsitewww.grandriver.ca The Grand River Conservation Authority (GRCA) is a conservation authority in Ontario, Canada. It operates under the Conservation Authorities A...

Jean Baptiste Joseph Fourier Jean Baptiste Joseph Fourier (Auxerre, 21 marzo 1768 – Parigi, 16 maggio 1830) è stato un matematico e fisico francese, conosciuto soprattutto per le sue famose serie e trasformata e per la sua legge sulla conduzione del calore. Indice 1 Biografia 2 Contributi 3 Riconoscimenti 4 Opere 5 Onorificenze 6 Voci correlate 7 Altri progetti 8 Collegamenti esterni Biografia Théorie analitique de la chaleur, 1888 La sua istruzione si compì dapprima dai Benedettini, poi...

List of links and links-style golf courses The following is a list of links golf courses; also included are many links-style courses and courses that share many of the features of links courses. Scotland Dumfries and Galloway Southerness Golf Club, Southerness, Dumfriesshire[1] Wigtownshire County Golf Club, Glenluce, Wigtownshire[1] Strathclyde Dundonald Links Golf Club, Irvine, Ayrshire[1] Gailes Links Golf Club, Irvine, Ayrshire[1] Irvine Golf Club, Irvine, ...

Kedutaan Besar Republik Indonesia di Seoul대사관은 인도네시아 공화국 서울시 Koordinat37°31′07″N 126°55′54″E / 37.518501°N 126.93158°E / 37.518501; 126.93158Lokasi Seoul, Republik KoreaAlamat380 Youidaebangro, YoudeungpokuSeoul 150895, Republik KoreaDuta BesarGandi Sulistiyanto SoehermanYurisdiksi Korea SelatanSitus webkemlu.go.id/seoul/id Kedutaan Besar Republik Indonesia di Seoul (KBRI Seoul) adalah misi diplomatik Republik Indonesia un...

County in South West England This article is about the county in England. For other uses, see Somerset (disambiguation). Non-metropolitan and ceremonial county in EnglandSomersetNon-metropolitan and ceremonial countyThe Somerset Levels from Glastonbury Tor; St Mary's Church, Bruton; and Pulteney Bridge, BathCoordinates: 51°06′N 2°54′W / 51.100°N 2.900°W / 51.100; -2.900Sovereign stateUnited KingdomConstituent countryEnglandRegionSouth West EnglandEstablishedAnc...

Data DAFIF mengenai diagram Bandar Udara Internasional Ottawa Macdonald-Cartier. DAFIF atau Digital Aeronautical Flight Information File (Bahasa Indonesia: Berkas Digital Informasi Penerbangan Aeronautika) adalah basis data aeronautika yang komprehensif dan terkini, termasuk didalamnya informasi mengenai data bandar udara, maskapai penerbangan, wilayah udara, navigasi dan fakta lain yang relevan untuk terbang di seluruh dunia, yang dikelola oleh National Geospatial-Intelligence Agency (NGA). ...

Marvel TelevisionJenisDivisiIndustriTelevisiGenrePahlawan superDidirikan28 Juni 2010; 13 tahun lalu (2010-06-28)KantorpusatBurbank, California[1], ASTokohkunciJeph Loeb (wakil presiden eksekutif)Dan Buckley (presiden animasi dan unit digital)ProdukSerial televisiPemilikMarvel Entertainment(The Walt Disney Company)AnakusahaMarvel Animation, Inc.[2]Situs webmarvel.com/tv Marvel Television adalah sebuah divisi dari Marvel Entertainment yang merupakan bagian dari The Walt Dis...

Hong Kong para-badminton player Badminton playerChan Ho Yuen陳浩源Personal informationCountry Hong KongBorn (1985-01-17) 17 January 1985 (age 39)Hong KongMen’s singles WH2Highest ranking2 (1 January 2019)Current ranking3 (8 November 2022) Medal record Men's para-badminton Representing Hong Kong Paralympic Games 2020 Tokyo Men's singles World Championships 2019 Basel Men's singles 2013 Dortmund Men's singles 2013 Dortmund Men's doubles 2015 Stoke Mandeville Men's...
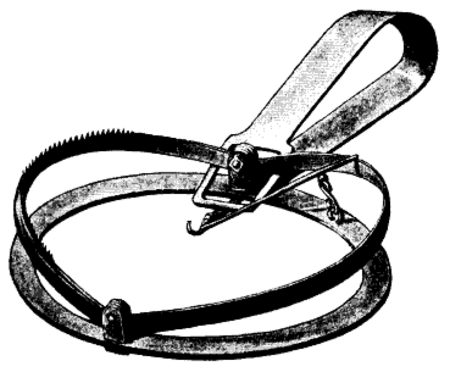
Trap for catching humans Two mantraps (one a humane type) and a spring gun A mantrap is a mechanical physical security device for catching poachers and other trespassers.[1][unreliable source?] They have taken many forms, the most usual being similar to a large foothold trap, the steel springs being armed with teeth which meet in the victim's leg. In 1827, they were made illegal in England, except in houses between sunset and sunrise as a defence against burglars.[2]&#...

Vous lisez un « bon article » labellisé en 2011. Il fait partie d'un « bon thème ». La dynastie Song (chinois : 宋朝 ; pinyin : Sòng cháo ; 960–1279) de Chine est une dynastie régnante qui contrôle la Chine historique et le Sud de la Chine du milieu du Xe siècle jusqu’au dernier quart du XIIIe siècle. Cette dynastie marque un tournant important dans le domaine des innovations en science et technologie de la Chine a...

1-я румынская добровольческая пехотная дивизия имени Тудора Владимирескурум. Divizia Tudor Vladimirescu Флаг Народной Республики Румынии, изображённый на знаке дивизии Годы существования 4 октября 1943—после 1947 Страна СССР Румыния Входит в 2-й Украинский фронт, 33-й стрелковый к�...

Continuous function on an interval takes on every value between its values at the ends Intermediate value theorem: Let f {\displaystyle f} be a continuous function defined on [ a , b ] {\displaystyle [a,b]} and let s {\displaystyle s} be a number with f ( a ) < s < f ( b ) {\displaystyle f(a)<s<f(b)} . Then there exists some x {\displaystyle x} between a {\displaystyle a} and b {\displaystyle b} such that f ( x ) = s {\displaystyle f(x)=s} . In mathematical analysis, the intermedi...
