Vụ Rách Lớn
|
Read other articles:

—— Permukiman di Uni Emirat Arab —— Al Barshaالبرشا Dubai Autodrome dan wilayah sekitar Al Barsha Negara Uni Emirat Arab Emirat Dubai Kota Dubai Didirikan 2000 Jumlah daerah 373-376, 671-673 Statistik permukiman Luas 38.1 km2 Jumlah penduduk 1,248[1] (2000) Kepadatan penduduk 32.75/km2 Permukiman sekitarnya Al Sufouh, Emirates Hills, Al Quoz Koordinat 25°06′40″N 55°11′43″E / 25.11111°N 55.19528°E / 25.11111; 55.19528 A...

Boetet Satidjah (EYD: Butet Satijah; Tapanuli Selatan, 1906 - tidak diketahui) adalah wartawati Indonesia pertama yang mendirikan surat kabar dan sekaligus menjadi editornya. Ia merupakan istri dari wartawan Parada Harahap. Boetet merupakan pendiri sekaligus editor surat kabar bulanan “Perempoean Bergerak” yang terbit di Medan tahun 1919 (De Preanger-bode, 19-06-1919), dengan motto ‘De beste stuurlui staan aan wal’ (Sahabat terbaik mampu melindungi).[1][2] Edisi pertam...

FC SüdtirolCalcio Rot-Weiß / Biancorossi, l'Alto, FCS Segni distintivi Uniformi di gara Casa Trasferta Colori sociali Bianco, rosso Inno FCSLukas Tait e altri[1] Dati societari Città Bolzano Nazione Italia Confederazione UEFA Federazione FIGC Campionato Serie B Fondazione 1974 Presidente Gerhard Comper Allenatore Federico Valente Stadio Druso(5 539 posti) Sito web www.fc-suedtirol.com Palmarès Stagione in corso Si invita a seguire il modello di voce Il Fussball Club S�...

Osaka Gas Co., Ltd.Nama asli大阪ガス株式会社大阪瓦斯株式会社 (with ateji)Nama latinŌsaka Gasu kabushiki gaishaJenisPerusahaan KKKode emitenTYO: 9532IndustriProduser EnergiDidirikanNishi-ku, Osaka, Jepang (15 April 1897 (1897-04-15))KantorpusatChuo-ku, Osaka, JapanTokohkunciHiroshi Ozaki (Presiden)ProdukGas alam cair, EnergiPendapatan¥1,294,781 million (2012)[* 1]¥1,187,142 million (2011)Laba operasi¥77,274 juta (2012)[* 1]¥88,584 j...

George Edwards George Edwards (3 April 1694 – 23 Juli 1773) adalah seorang naturalis dan ornithologis dari Inggris yang diketahui sebagai bapak ornitologi Inggris. Edwards lahir Stratford, Essex. Pada tahun-tahun awal, ia melakukan perjalanan melewati dataran Eropa untuk belajar sejarah alam dan memperoleh beberapa reputasi tentang penggambaran berwarna tentang hewan, terutama burung. Pada 1733, ia direkomendasikan Sir Hans Sloane sebagai pustakawan di Royal College of Physicians London. Il...

IIIe République Vincent Audren de Kerdrel, sénateur de 1876 à 1899 Hippolythe Thome de Keridec, sénateur de 1876 à 1878 Charles de La Monneraye sénateur de 1876 à 1894 Armand Fresneau sénateur de 1879 à 1900 Gustave de Lamarzelle, sénateur de 1894 à 1924 Charles Riou, sénateur de 1900 à 1920 Geoffroy de Goulaine, sénateur de 1901 à 1913 Roger Audren de Kerdrel, sénateur de 1909 à 1920 Jean Guilloteaux, sénateur de 1913 à 1924 Alfred Brard, sénateur de 1920 à 1940 Lo...

2014 Indian filmEndrendrumFilm posterDirected bySinish SreedharanWritten bySinish SreedharanProduced bySinish SreedharanStarringSathish Krishnan Priyanka ReddyEdited byT. S. SureshMusic byDharan KumarRelease date 7 March 2014 (2014-03-07) CountryIndiaLanguageTamilBudget1CBox office25L Endrendrum (lit. 'Everlasting') is a 2014 Tamil film written, produced, and directed by Ssinish Sreedharan. It started off as a short film, and over a period of time, it was developed i...

Coppa del Mondo di sci alpino 2012 Uomini Donne Vincitori Generale Marcel Hirscher Lindsey Vonn Discesa libera Klaus Kröll Lindsey Vonn Supergigante Aksel Lund Svindal Lindsey Vonn Slalom gigante Marcel Hirscher Viktoria Rebensburg Slalom speciale André Myhrer Marlies Schild Combinata Ivica Kostelić Lindsey Vonn Dati manifestazione Tappe 21 20 Gare individuali 44 37 Gare a squadre miste 1 Gare cancellate 1 3 2011 2013 La Coppa del Mondo di sci alpino 2012 fu la quarantaseiesima edizione d...

Demolished public building in Brussels, Belgium This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Maison du Peuple, Brussels – news · newspapers · books · scholar · JSTOR (December 2016) (Learn how and when to remove this message) Maison du Peuple (French)Volkshuis (Dutch)View of the Maison du Peuple...

Gereja Kristen Protestan MentawaiPenggolonganProtestanOrientasiLutheranModeratorPdt. P. Saguntung M.Si.WilayahIndonesiaDidirikan9 Juli 1916Jemaat51 jemaatUmat35.000 jiwaNama lainGKPM Gereja Kristen Protestan Mentawai (disingkat GKPM) ialah suatu kelompok gereja Kristen Protestan di Indonesia, khususnya di Kabupaten Kepulauan Mentawai, Sumatera Barat. Didirikan di Pulau Pagai Utara dan akhirnya menjadi anggota Persekutuan Gereja-gereja di Indonesia (PGI) yang ke 45. Sejarah GKPM didirikan pada...

Voce principale: Wigan Athletic Football Club. Wigan A.F.C.Stagione 2014-2015Sport calcio Squadra Wigan 2013-2014 2015-2016 Si invita a seguire il modello di voce Questa voce raccoglie le informazioni riguardanti il Wigan Athletic Football Club nelle competizioni ufficiali della stagione 2014-2015. Rosa N. Ruolo Calciatore 1 P Scott Carson 3 D Andrew Taylor 4 D Harry Maguire 5 D Gary Caldwell 7 C Chris McCann 10 A Billy McKay 11 A James McClean 14 C Emyr Huws 16 C Kim Bo-Kyung 17 D Emme...

هذه المقالة عن المجموعة العرقية الأتراك وليس عن من يحملون جنسية الجمهورية التركية أتراكTürkler (بالتركية) التعداد الكليالتعداد 70~83 مليون نسمةمناطق الوجود المميزةالبلد القائمة ... تركياألمانياسورياالعراقبلغارياالولايات المتحدةفرنساالمملكة المتحدةهولنداالنمساأسترالي�...

Protein-coding gene in the species Homo sapiens HLXIdentifiersAliasesHLX, HB24, HLX1, H2.0 like homeoboxExternal IDsOMIM: 142995 MGI: 96109 HomoloGene: 7363 GeneCards: HLX Gene location (Human)Chr.Chromosome 1 (human)[1]Band1q41Start220,879,431 bp[1]End220,885,059 bp[1]Gene location (Mouse)Chr.Chromosome 1 (mouse)[2]Band1 H5|1 88.97 cMStart184,459,337 bp[2]End184,464,816 bp[2]RNA expression patternBgeeHumanMouse (ortholog)Top expressed inst...

اقتصاد الصومالالبنك المركزي الصوماليعامالدولة الصومالعملة شلن صوماليالمنظمات الاتحاد الأفريقي، اتفاقية التجارة الحرة القارية الأفريقية (موقعة)، CAEU، تجمع دول الساحل والصحراء، الهيئة الحكومية الدولية المعنية بالتنميةالإحصائياتالناتج الإجمالي ▲ 5.218 مليار دولار (الاسمي...

Logo Goodwill Industries Goodwill Industries International Inc., yang dikenal sebagai Goodwill, adalah organisasi nirlaba Amerika 501(c)(3) yang menyediakan pelatihan kerja, layanan penempatan kerja, dan program berbasis komunitas lainnya untuk orang-orang yang menghadapi hambatan dalam pekerjaan mereka.[1] Menurut laporan tahunannya, Goodwill Industries juga mempekerjakan veteran dan individu yang tidak memiliki pengalaman kerja, pendidikan, atau menghadapi tantangan pekerjaan. Organ...

Developmental robotics (DevRob), sometimes called epigenetic robotics, is a scientific field which aims at studying the developmental mechanisms, architectures and constraints that allow lifelong and open-ended learning of new skills and new knowledge in embodied machines. As in human children, learning is expected to be cumulative and of progressively increasing complexity, and to result from self-exploration of the world in combination with social interaction. The typical methodological ap...

NCAA women's ice hockey postseason tournament Collegiate ice hockey tournament 2023 NCAA National Collegiate Women's ice hockey tournament2023 Women's Frozen Four logoTeams11Finals siteAMSOIL ArenaDuluth, MinnesotaChampionsWisconsin Badgers (7th title)Runner-upOhio State Buckeyes (2nd title game)SemifinalistsNortheastern Huskies (3rd Frozen Four)Minnesota Golden Gophers (14th Frozen Four)Winning coachMark Johnson (7th title)MOPCami Kronish (Wisconsin) ← 2022 NCAA women'...
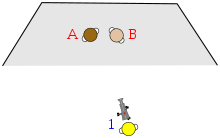
Film or video production principally using only one camera This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Single-camera setup – news · newspapers · books · scholar · JSTOR (June 2016) (Learn how and when to remove this message) Diagram showing a single-camera setup The single-camera setup, or single-camera ...

Collegiate summer baseball team in Massachusetts Cotuit KettleersInformationLeagueCape Cod Baseball League (West Division)LocationCotuit, MassachusettsBallparkLowell ParkFounded1947League championships1961, 1962, 1963, 1964, 1972, 1973, 1974, 1975, 1977, 1981, 1984, 1985, 1995, 1999, 2010, 2013, 2019ManagerMike RobertsGeneral ManagerBruce MurphyPresidentTerry MoranWebsitewww.capecodleague.com/cotuit/ The Cotuit Kettleers are a collegiate summer baseball team based in the village of Cotui...

Degree to which an optical system converges or diverges light For magnifying power, see Magnification. For the power of light, see Radiant flux and Luminous flux. Illustration of the relationship between optical power in diopters and focal length in meters. In optics, optical power (also referred to as dioptric power, refractive power, focusing power, or convergence power) is the degree to which a lens, mirror, or other optical system converges or diverges light. It is equal to the reciprocal...
