Дуглас Кельнер

Дуглас Кельнер
Народився31 травня 1943(1943-05-31)[1][2] (81 рік)
Вашингтон, США[3]
Країна США[4][5]
Діяльністьфілософ, викладач університету
Галузьфілософія культури[6], культурологія[d][6], критична теорія[6], постмодернізм[6] і медіаосвіта[6]
Знання мованглійська[7][6][8]
ЗакладКаліфорнійський університет в Лос-Анджелесі

Дуглас Кельнер (англ. Douglas Kellner) (нар. 1943[9]) — американський філософ і професор Каліфорнійського університету в Лос-Анджелесі. Він був редактором повного видання творів Герберта Маркузе[10] з 1998 року і відтак згадується як представник «третього покоління» Франкфуртської школи[11].

Кельнер отримав ступінь бакалавра в коледжі Доан у 1965 році і ступінь доктора філософії в Колумбійському університеті в 1973 році[12]. До цього він здійснив кілька навчальних поїздок до Європи, у тому числі з 1969 до 1971 року стажувався в Тюбінгенському університеті. Після закінчення докторської дисертації він був асистентом професора з 1973 до 1997 року, потім доцентом і, нарешті, з 1985 року, постійним професором Техаського університету в Остіні. З 1997 року він є професором філософії освіти в Каліфорнійському університеті в Лос-Анджелесі. Він є членом правління Міжнародного товариства Герберта Маркузе[13].

Вибрані публікації

  • Herbert Marcuse and the crisis of Marxism. University of California Press, Berkeley 1984, ISBN 0520051769.
  • Ernesto "Che" Guevara. Chelsea House Publishers, New York 1989, ISBN 1555468357.
  • Grand theft 2000. Media spectacle and a stolen election. Rowman & Littlefield, Lanham 2000, ISBN 0742521028.
  • From 9/11 to terror war. The dangers of the Bush legacy. Rowman & Littlefield, Lanham 2003, ISBN 0742526372.
  • Guys and guns amok. Domestic terrorism and school shootings from the Oklahoma City bombing to the Virginia Tech massacre. Paradigm Publishers, Boulder (Colorado) 2008, ISBN 9781594514920.

В Україні

Окремі статті публікувались у Журналі «Ї»[14]

Вебпосилання

Примітки

  1. ↑ Deutsche Nationalbibliothek Record #112859127 // Gemeinsame Normdatei — 2012—2016.
  2. ↑ SNAC — 2010.
  3. ↑ Бібліотека Конгресу — 1800.
  4. ↑ Bibliothèque nationale de France BNF: платформа відкритих даних — 2011.
  5. ↑ LIBRIS — Королівська бібліотека Швеції, 2004.
  6. ↑ а б в г д е Чеська національна авторитетна база даних
  7. ↑ Bibliothèque nationale de France BNF: платформа відкритих даних — 2011.
  8. ↑ CONOR.Sl
  9. ↑ Geburtsdatum laut WorldCat Identities. Архів оригіналу за 11 травня 2022. Процитовано 11 травня 2022.
  10. ↑ The Many Dimensions of Herbert Marcuse [Архівовано 22 жовтня 2020 у Wayback Machine.], Brandeis University
  11. ↑ Laureano Ralón und Justin Dowdall, Interview mit Douglas Kellner [Архівовано 2 грудня 2021 у Wayback Machine.], Figure Ground, 13 серпня 2012
  12. ↑ Biografische Angaben beruhen auf: Douglas Kellner, Vita (PDF) [Архівовано 12 вересня 2021 у Wayback Machine.]
  13. ↑ International Herbert Marcuse Society [Архівовано 11 травня 2022 у Wayback Machine.], About the society (Stand 2019).
  14. ↑ Дуглас Кельнер. Перша кібергенерація.//Журнал «Ї», Ч. 38, 2005. Архів оригіналу за 26 квітня 2022. Процитовано 11 травня 2022.

Read other articles:

Änne Bruck

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Februari 2023. Änne BruckLahir(1907-08-23)23 Agustus 1907Hamburg, JermanMeninggal13 Desember 1978(1978-12-13) (umur 71)Munich, JermanPekerjaanPemeranTahun aktif1936–1978 Änne Bruck (23 Agustus 1907 – 13 Desember 1978) adalah seorang pem...

 

 

Katak-tanduk jawa

Katak-tanduk Jawa Katak-tanduk jawa (Megophrys montana) di hutan Jabranti, Karangkancana, Kuningan Status konservasi Risiko Rendah (IUCN 2.3)[1] Klasifikasi ilmiah Kerajaan: Animalia Filum: Chordata Kelas: Amphibia Ordo: Anura Famili: Megophryidae Genus: Megophrys Spesies: M. montana Nama binomial Megophrys montanaKuhl & van Hasselt, 1822.[2]:102 Sinonim Megophrys monticola Kuhl and Van Hasselt, 1822 Megophrys Kuhlii Gray, 1825 Sumber: AMNH.ASW[3] Katak-...

 

 

Piala FA 1879–1880

Piala FA 1879–1880Negara InggrisJumlah peserta54Juara bertahanOld EtoniansJuaraClapham Rovers(gelar ke-1)Tempat keduaOxford University← 1878–1879 1880–1881 → Piala Challenge The Football Association 1879–1880 adalah edisi kesembilan dari penyelenggaraan Piala FA, turnamen tertua dalam sepak bola di Inggris. 54 tim mengikuti turnamen ini, di mana 5 dari 54 tim tidak memainkan satupun pertandingan. Edisi ini dimenangkan oleh Clapham Rovers setelah mengalahkan Oxford University ...

Muriel (given name)

MurielMuriel is an English name derived from Celtic elements meaning sea and brightPronunciation/ˈmjʊəriəl/ MURE-ee-əlGenderFeminineLanguage(s)EnglishOriginLanguage(s)CelticOther namesCognate(s)Muireall (Scottish Gaelic), Muirgheal (Irish) Muriel /ˈmjʊəriəl/ MURE-ee-əl[1] is a feminine given name in the English language. Origin/history The name is of Goidelic origin and was originally spelled as Muirgheal (muir sea, gheal bright) in Irish and Muireall in Scottish Gaelic. Var...

 

 

Yeong-ja's Heydays

1975 South Korean film directed by Kim Ho-sun Yeong-ja's HeydaysDirected byKim Ho-sunWritten byJo Seon-jakProduced byKim Tai-sooStarringYeom Bok-sun Song Jae-hoCinematographyJang Seok-junEdited byYoo Jae-wonMusic byJeong Sung-joRelease date February 11, 1975 (1975-02-11) Running time103 minutesCountrySouth KoreaLanguageKorean Yeong-ja's Heydays (Korean: 영자의 전성시대; RR: Yeongja-ui jeonseong sidae) is a 1975 South Korean film directed by Kim Ho-s...

 

 

T. O. Morrow

Comics character T. O. MorrowPublication informationPublisherDC ComicsFirst appearanceThe Flash #143 (March 1964)Created byGardner Fox (writer)Carmine Infantino (artist)In-story informationAlter egoTomek Ovadya MorahSpeciesHumanPlace of originPolandTeam affiliationsS.T.A.R. LabsScience SquadInjustice LeagueNotable aliasesThomas Oscar MorrowAbilities Genius-level intellect Proficient engineer, inventor, and roboticist T. O. Morrow is a supervillain appearing in American comic books published b...

Mount Assiniboine Provincial Park

Provincial park in British Columbia, Canada Mount Assiniboine Provincial ParkIUCN category II (national park)[1]Mount Assiniboine and Lake Magog at dawnLocation of Mount Assiniboine Provincial Park in British ColumbiaLocationEast Kootenay, British Columbia, CanadaNearest cityBanff, AlbertaCoordinates50°56′N 115°44′W / 50.94°N 115.74°W / 50.94; -115.74Area39,050 ha (150.8 sq mi)Established1922Governing bodyBC ParksWebsitebcparks....

 

 

David Brearley

American Founding Father and judge David BrearleyJudge of the United States District Court for the District of New JerseyIn officeSeptember 26, 1789 – August 16, 1790Appointed byGeorge WashingtonPreceded bySeat established by 1 Stat. 73Succeeded byRobert Morris Personal detailsBornDavid Brearley(1745-06-11)June 11, 1745Spring Grove, Maidenhead (now Lawrence Township), Hunterdon County,Province of New Jersey,British AmericaDiedAugust 16, 1790(1790-08-16) (aged 45)Trenton, New J...

 

 

Embassy Court

Historic site in East Sussex, United KingdomEmbassy CourtEmbassy Court seen from the southeastLocationKings Road, Brighton BN1 2PY, Brighton and Hove, East Sussex, United KingdomCoordinates50°49′23″N 0°09′23″W / 50.8230°N 0.1564°W / 50.8230; -0.1564Height110 feet (34 m)Built1934–35Built forMaddox PropertiesRestored2004–05Restored byConran and Partners; Makers LtdArchitectWells CoatesArchitectural style(s)Art Deco/ModernistOwnerBluestorm ...

Gilberto Freyre

Brazilian scholar, writer, and politician This article includes a list of general references, but it lacks sufficient corresponding inline citations. Please help to improve this article by introducing more precise citations. (June 2010) (Learn how and when to remove this message) Gilberto FreyreGilberto Freyre c. 1956BornGilberto de Mello Freyre(1900-03-15)March 15, 1900Recife, Pernambuco, BrazilDiedJuly 18, 1987(1987-07-18) (aged 87)Recife, Pernambuco, BrazilAlma materBaylor ...

 

 

Canon EF 28-80mm lens

Canon SLR EF mount zoom lens Canon EF 28-80mm f/3.5-5.6 USM. The EF 28–80mm f/3.5–5.6 is a zoom lens produced by Canon Inc. for their series of EOS single-lens reflex cameras. Canon produced eight iterations of the lens from 1989 to 1999. The other 28–80mm lens is the EF 28–80mm f/2.8–4L USM, which was an EF mount wide-to-normal zoom lens introduced by Canon in April 1989. Being of the L class, it is of completely different design and structure than the aforementioned 28–80mm f/3....

 

 

Chiesa (architettura)

Questa voce o sezione sull'argomento architettura ha problemi di struttura e di organizzazione delle informazioni. Motivo: migliorare le struttura dei capitoli (vedere pag di discussione. Ad es. vi è la sezione Storia con la sottosezione XIX e XX secolo, e subito dopo si torna indietro a I luoghi di riunione prima della pace della Chiesa. Risistema la struttura espositiva, logica e/o bibliografica dei contenuti. Nella discussione puoi collaborare con altri utenti alla risistemazione. S...

Charles X

Pour les articles homonymes, voir le roi de Suède Charles X Gustave et le cardinal Charles de Bourbon. Charles X « Charles X en habits de sacre » (François Gérard, 1825, huile sur toile, conservé au musée du Prado). Titre Roi de France et de Navarre 16 septembre 1824 – 2 août 1830(5 ans, 10 mois et 17 jours) Couronnement le 29 mai 1825 en la cathédrale de Reims Président du Conseil Joseph de VillèleJean-Baptiste de MartignacJules de PolignacCasimir-Louis...

 

 

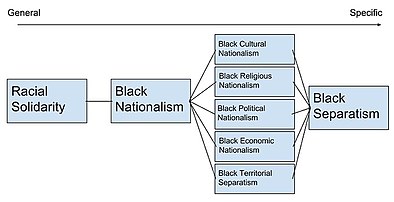
Black separatism

Movement for separate institutions This article is part of a series aboutBlack power History Black Arts Movement Black is beautiful Black power movement Black Power Revolution Kwanzaa Raised fist events of 1968 and 1972 Ideologies African socialism Africana womanism Afrocentrism Anti-Americanism Black anarchism Black feminism Black leftism Black nationalism Black pride Black separatism Black supremacy Intercommunalism Pan-Africanism Organizations African People's Socialist Party Assata's Daug...

 

 

Gelug

Patung Je Tsongkhapa, pendiri mazhab Gelugpa, pada sebuah altar di kuilnya (tempat kelahirannya) di biara Kumbum, dekat Xining, Qinghai (Amdo), Tiongkok. Foto diambil oleh Mario Biondi, 7 Juli 2006. Gelug atau Gelug-pa (atau dGe Lugs Pa, dge-lugs-pa, atau Dgelugspa; bahasa Mongol: Sharyn shashin, agama kuning), juga dikenal sebagai Sekte Topi Kuning, adalah mazhab Buddhisme yang didirikan oleh Je Tsongkhapa (1357—1419), seorang filsuf sekaligus pemimpin religius Tibet. Biara pertama yang di...

Ferrovia Retica

Ferrovia ReticaLogo Sede centrale Ferrovia Retica a Coira. Stato Svizzera Forma societariaAzienda pubblica ISINCH0002234372 Fondazione1885 a Coira Fondata daWillem-Jan Holsboer Sede principaleCoira Persone chiave Hans-Jürg Spillmann, presidente Heinz Dudli, vicepresidente SettoreTrasporto Prodottitrasporti ferroviari Fatturato308,7 milioni CHF (2007) Dipendenti1.348 (2008) Slogan«Die kleine Rote» Sito webwww.rhb.ch/ Modifica dati su Wikidata · Manuale La Ferrovia Retica (in&...

 

 

العلاقات الإستونية المالية

العلاقات الإستونية المالية إستونيا مالي   إستونيا   مالي تعديل مصدري - تعديل   العلاقات الإستونية المالية هي العلاقات الثنائية التي تجمع بين إستونيا ومالي.[1][2][3][4][5] مقارنة بين البلدين هذه مقارنة عامة ومرجعية للدولتين: وجه المقارنة إستوني�...

 

 

Gangnam Style

Gangnam StyleSingel oleh Psydari album Psy 6 (Six Rules), Part 1Dirilis15 Juli 2012 (2012-07-15)FormatCD single, unduh digitalDirekam2012GenreK-pop[1][2]Durasi3:39LabelYG, Universal Republic, School BoyPenciptaPark Jae-Sang, Yoo Gun-hyung[3]ProduserPark Jae-Sang, Yoo Gun Hyung[4] Karya seni kaset Psy 6 (Six Rules), Part 1 Karya seni kaset Psy 6 (Six Rules), Part 1 Video musikGangnam Style di YouTube Gangnam Style (bahasa Korea: 강남스타일, Penguca...

المسرح الأوروبي للحرب العالمية الثانية

المسرح الأوروبي للحرب العالمية الثانية جزء من الحرب العالمية الثانية  التاريخ وسيط property غير متوفر. بداية 1 سبتمبر 1939  نهاية 8 مايو 1945  الموقع أوروبا  تعديل مصدري - تعديل     هذه المقالة عن منطقة جغرافية وقعت في نطاق عمليات الحرب العالمية الثانية. لمقالات �...

 

 

Perrin number

Number sequence 3,0,2,3,2,5,5,7,10,... Spiral of equilateral triangles with side lengths equal to Perrin numbers. In mathematics, the Perrin numbers are a doubly infinite constant-recursive integer sequence with characteristic equation x3 = x + 1. The Perrin numbers bear the same relationship to the Padovan sequence as the Lucas numbers do to the Fibonacci sequence. Definition The Perrin numbers are defined by the recurrence relation P ( 0 ) = 3 , P ( 1 ) = 0 , P ( 2 ) = 2 , P ( n ) = P ( n &...