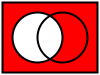
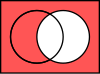
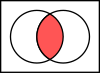
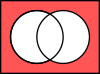
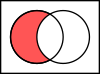
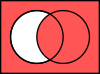
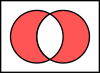
Função de verdade
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Read other articles:

本條目存在以下問題,請協助改善本條目或在討論頁針對議題發表看法。 此條目需要补充更多来源。 (2018年3月17日)请协助補充多方面可靠来源以改善这篇条目,无法查证的内容可能會因為异议提出而被移除。致使用者:请搜索一下条目的标题(来源搜索:羅生門 (電影) — 网页、新闻、书籍、学术、图像),以检查网络上是否存在该主题的更多可靠来源(判定指引)。 �...

Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: Jasa – berita · surat kabar · buku · cendekiawan · JSTOR Pemasaran Pemasaran Manajemen pemasaran Konsep inti Bauran pemasaran Produk Harga Distribusi Jasa Eceran Kelola merek Pemasaran berbasis akuntan E...

Chemical compound MedroxalolIdentifiers IUPAC name 5-(2-{[4-(1,3-Benzodioxol-5-yl)-2-butanyl]amino}-1-hydroxyethyl)-2-hydroxybenzamide CAS Number56290-94-9PubChem CID41835ChemSpider38173UNII7PX96289JACompTox Dashboard (EPA)DTXSID30866550 ECHA InfoCard100.054.618 Chemical and physical dataFormulaC20H24N2O5Molar mass372.421 g·mol−13D model (JSmol)Interactive image SMILES O=C(N)c1cc(ccc1O)C(O)CNC(C)CCc2ccc3OCOc3c2 InChI InChI=1S/C20H24N2O5/c1-12(2-3-13-4-7-18-19(8-13)27-11-26-18)22-10-17...

Provinsi Pomerania Barat provinsi di Polandia flag of West Pomeranian Voivodeship (en) coat of arms of the West Pomeranian Voivodeship (en) Dinamakan berdasarkanPomorze Zachodnie Tempat <mapframe>: Judul Poland/West Pomeranian.map .map bukan merupakan halaman data peta yang sah Negara berdaulatPolandia NegaraPolandia Ibu kotaSzczecin Pembagian administratifKamień County (en) Gryfino County (en) Gryfice County (en) Goleniów County (en) Powiat drawski (en) Choszczno County (en) Białoga...

Artikel ini tidak memiliki bagian pembuka yang sesuai dengan standar Wikipedia. Mohon tulis paragraf pembuka yang informatif sehingga pembaca dapat memahami maksud dari Toksikologi pestisida. Contoh paragraf pembuka Toksikologi pestisida adalah .... (Pelajari cara dan kapan saatnya untuk menghapus pesan templat ini) Artikel ini membutuhkan penyuntingan lebih lanjut mengenai tata bahasa, gaya penulisan, hubungan antarparagraf, nada penulisan, atau ejaan. Anda dapat membantu untuk menyuntingnya...

Quarterly investigative magazine on right-wing groups This article is about Public Eye Magazine. For the journal of the Swiss organisation, see Public Eye (organization). For other uses, see Public Eye. The Public Eye The Public Eye Magazine is published by Political Research Associates in Somerville, Massachusetts.[1] The magazine was founded in 1977 by the Public Eye Network.[2][3] It currently contains investigative articles about movements, institutions, and ideolo...

SuperDracoDue motori SuperDraco.Paese di origine Stati Uniti Principale costruttoreSpaceX Applicazionesistema di fuga e atterraggio verticale StatusIn servizio Motore a propellente liquidoPropellenteNTO / MMH PrestazioniSpinta (livello del mare)71 kN Raggio di regolazione100-20% Pressione camera6900 kPa Isp (livello del mare)235 s Tempo di accensione25 s Usato inDragon 2 Voce principale: Draco (motore a razzo). Il SuperDraco è un motore a razzo ipergolico progettato e assemblato da Spac...

German actress Knef redirects here. For her 1970 self-titled album, see Knef (album). You can help expand this article with text translated from the corresponding article in German. (August 2010) Click [show] for important translation instructions. View a machine-translated version of the German article. Machine translation, like DeepL or Google Translate, is a useful starting point for translations, but translators must revise errors as necessary and confirm that the translation is accu...

Sekolah Menengah Atas Santa Angela BandungInformasiDidirikan27 Februari 1951 dengan nama SMA SANTA ANGELAAkreditasiAKepala SekolahLucia Sri Istanti, S. SiModerator712 siswaJumlah kelas24 kelas (2017/2018)Rentang kelasMIA, IIS, dan IBAKurikulumX MIA, X IIS, XI MIA, XI IIS, XII MIA, XII IIS, XII IBAJumlah siswaKurikulum Tingkat Satuan PendidikanStatusSwastaNEM terendah21,50 (2007)NEM tertinggi27,96 (2007)Nilai masuk rata-rata26,55 (2007)AlamatLokasiJalan Merdeka 24, Kota Bandu...

Open air or skylight; architectural feature, courtyard in a Roman domus The Tucson High School Galleria and reflexive library (pictured) feature a modern atrium tetrastylum with four support columns and open roof In architecture, an atrium (pl.: atria or atriums)[1] is a large open-air or skylight-covered space surrounded by a building.[2] Atria were a common feature in Ancient Roman dwellings, providing light and ventilation to the interior. Modern atria, as developed in the ...

The Local Lore Museum of Goris The Local Lore Museum of Goris (arm. Գորիսի երկրագիտական թանգարան) is the first museum in the Syunik marz of Armenia. The museum opened in 1948 and was originally located in an abandoned church. Located in the center of the city of Goris, it houses a collection of over 5,000 ethnographic and archaeological objects that tell the story of the region's cultural heritage, history, and traditions. History The exhibition hall of the Local Lor...

History of former New York City baseball team This article is about the baseball team when in New York until 1957. For the football team, see New York Giants. For the history of the team from 1958 onward, see History of the San Francisco Giants. For information on the franchise in general, see San Francisco Giants. For the Players' League team, see New York Giants (Players' League). New York Gothams redirects here. For the basketball team, see New York Gothams (basketball). This article needs...

2007 film by James Foley Perfect StrangerTheatrical release posterDirected byJames FoleyScreenplay byTodd KomarnickiStory byJon BokenkampProduced by Elaine Goldsmith-Thomas Stephanie Langhoff Daniel A. Thomas Starring Halle Berry Bruce Willis Giovanni Ribisi Gary Dourdan CinematographyAnastas N. MichosEdited byChristopher TellefsenMusic byAntonio PintoProductioncompanies Columbia Pictures Revolution Studios Distributed bySony Pictures ReleasingRelease date April 13, 2007 (2007-...

Live album by Black Sabbath Cross Purposes LiveLive album by Black SabbathReleased13 March 1995[1]Recorded13 April 1994VenueHammersmith Apollo, LondonGenreHeavy metalLength70:09LabelI.R.S.ProducerBlack SabbathBlack Sabbath live albums chronology Live Evil(1982) Cross Purposes Live(1995) Reunion(1998) Cross Purposes LiveVideo by Black SabbathReleased13 March 1995Recorded13 April 1994VenueHammersmith Apollo, LondonGenreHeavy metalLength71 min (CD) 87 min. (VHS) LabelPMIDirectorD...

أوتو ملك اليونان (بالألمانية: Otto von Bayern) معلومات شخصية اسم الولادة (بالألمانية: Otto Friedrich Ludwig von Bayern) الميلاد 1 يونيو 1815(1815-06-01)سالزبورغ الوفاة 26 يوليو 1867 (52 سنة)بامبرغ مواطنة مملكة بافاريا مملكة اليونان الديانة الكنيسة الرومانية الكاثوليكية الزوجة أماليا أميرة أو�...

Альмагро Прозвища Tricolor Основан 1911 Стадион Альмагро,Буэнос-Айрес, Аргентина Вместимость 19 000 Президент Хулиан Ромеро Главный тренер Вальтер Перассо Сайт almagro.club (исп.) Соревнование Примера B Насьональ 2021 9-е в Зоне B Форма Основная Гостевая Резервная «Альма́гро» (исп. C...

ماندوراه الإحداثيات 32°31′44″S 115°43′23″E / 32.528888888889°S 115.72305555556°E / -32.528888888889; 115.72305555556 تقسيم إداري البلد أستراليا[1][2][3] التقسيم الأعلى أستراليا الغربية[1] خصائص جغرافية المساحة 106.6 كيلومتر مربع ارتفاع 2 متر معلومات أخرى 6210...

قصر هلال منظر عام وسط قصرهلال Official seal ofشعار الإحداثيات 35°39′N 10°54′E / 35.65°N 10.9°E / 35.65; 10.9 تقسيم إداري البلد تونس[1] التقسيم الأعلى ولاية المنستير عدد السكان عدد السكان 49376 (2014)[2] معلومات أخرى 5070 رمز جيونيمز 2468106 الموقع ال...

Proverbial idiom referring to an example of hypocrisy Charles H. Bennett's illustration of the saying (1860), with a coalman confronting a chimney sweep The pot calling the kettle black is a proverbial idiom that may be of Spanish origin, of which English versions began to appear in the first half of the 17th century. It means a situation in which somebody accuses someone else of a fault which the accuser shares, and therefore is an example of psychological projection,[1] or hypocrisy...

State in present-day northern Iran from 1349 to 1504 This article relies largely or entirely on a single source. Relevant discussion may be found on the talk page. Please help improve this article by introducing citations to additional sources.Find sources: Afrasiyab dynasty – news · newspapers · books · scholar · JSTOR (April 2017) Afrasiyab dynastyچلاویان1349–1504CapitalAmol(1349–1359 & 1393-1403)Firuzkuh (?)(1403–1504)Common la...